Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Quantum anomalous Hall effect with a permanent magnet defines a quantum resistance standard

Nature Physics volume 18, pages 25–29 (2022)Cite this article

Subjects

Abstract

The quantum anomalous Hall effect (QAHE)1,2,3,4,5,6 is a transport phenomenon where the Hall resistance is quantized to the von Klitzing constant due to the spontaneous magnetization of a ferromagnetic material even at zero magnetic field. Similar to the quantum Hall effect (QHE) under strong magnetic fields, the quantized Hall resistance of QAHE is supposed to be universal, independent of the details in the experimental realization7,8. However, the quantization accuracy of QAHE reported so far9,10,11 is much poorer than that of QHE. Here we demonstrate a precision of 10 parts per billion of Hall resistance quantization in QAHE. By directly comparing QAHE with QHE, we confirm that the quantization accuracy of QAHE satisfies the required level as a primary standard of electric resistance. We achieve this high accuracy of quantization by using a weak magnetic field supplied by a permanent disc magnet to align the magnetization domains. Our findings establish a milestone for developing a quantum resistance standard without strong magnetic fields.

This is a preview of subscription content, access via your institution

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Resistance standard based on QAHE with a permanent magnet.
Fig. 2: Electric transport properties.
Fig. 3: Direct comparison between QAHE with a permanent magnet and QHE in a strong field.
Fig. 4: Quantization accuracy of the QAHE-based resistance standard.

Data availability

Source data are provided with this paper. The data that support the findings of this study are available from the corresponding authors on reasonable request.

References

  1. Yu, R. et al. Quantized anomalous Hall effect in magnetic topological insulators. Science 329, 61–64 (2010).

    Article  ADS  Google Scholar 

  2. Chang, C.-Z. et al. Experimental observation of the quantum anomalous Hall effect in a magnetic topological insulator. Science 340, 167–170 (2013).

    Article  ADS  Google Scholar 

  3. Checkelsky, J. G. et al. Trajectory of the anomalous Hall effect towards the quantized state in a ferromagnetic topological insulator. Nat. Phys. 10, 731–736 (2014).

    Article  Google Scholar 

  4. Kou, X. et al. Scale-invariant quantum anomalous Hall effect in magnetic topological insulators beyond the two-dimensional limit. Phys. Rev. Lett. 113, 199901 (2014).

    Article  ADS  Google Scholar 

  5. Chang, C.-Z. et al. High-precision realization of robust quantum anomalous Hall state in a hard ferromagnetic topological insulator. Nat. Mater. 14, 473–477 (2015).

    Article  ADS  Google Scholar 

  6. Bestwick, A. J. et al. Precise quantization of the anomalous Hall effect near zero magnetic field. Phys. Rev. Lett. 114, 187201 (2015).

    Article  ADS  Google Scholar 

  7. Jeckelmann, B. & Jeanneret, B. The quantum Hall effect as an electrical resistance standard. Rep. Prog. Phys. 64, 1603–1655 (2001).

    Article  ADS  Google Scholar 

  8. Poirier, W. & Schopfer, F. Resistance metrology based on the quantum Hall effect. Eur. Phys. J. Spec. Top. 172, 207–245 (2009).

    Article  Google Scholar 

  9. Götz, M. et al. Precision measurement of the quantized anomalous Hall resistance at zero magnetic field. Appl. Phys. Lett. 112, 072102 (2018).

    Article  ADS  Google Scholar 

  10. Fox, E. J. et al. Part-per-million quantization and current-induced breakdown of the quantum anomalous Hall effect. Phys. Rev. B 98, 075145 (2018).

    Article  ADS  Google Scholar 

  11. Okazaki, Y. et al. Precise resistance measurement of quantum anomalous Hall effect in magnetic heterostructure film of topological insulator. Appl. Phys. Lett. 116, 143101 (2020).

    Article  ADS  Google Scholar 

  12. Stock, M., Davis, R., de Mirandés, E. & Milton, M. J. T. The revision of the SI—the result of three decades of progress in metrology. Metrologia 56, 022001 (2019).

    Article  ADS  Google Scholar 

  13. von Klitzing, K., Dorda, G. & Pepper, M. New method for high-accuracy determination of the fine-structure constant based on quantized Hall resistance. Phys. Rev. Lett. 45, 494–497 (1980).

    Article  ADS  Google Scholar 

  14. Thouless, D. J., Kohmoto, M., Nightingale, M. P. & den Nijs, M. Quantized Hall conductance in a two-dimensional periodic potential. Phys. Rev. Lett. 49, 405–408 (1982).

    Article  ADS  Google Scholar 

  15. Kohmoto, M. Topological invariant and the quantization of the Hall conductance. Ann. Phys. 160, 343–354 (1985).

    Article  ADS  MathSciNet  Google Scholar 

  16. Schopfer, F. & Poirier, W. Testing universality of the quantum Hall effect by means of the Wheatstone bridge. J. Appl. Phys. 102, 054903 (2007).

    Article  ADS  Google Scholar 

  17. Tzalenchuk, A. et al. Towards a quantum resistance standard based on epitaxial graphene. Nat. Nanotechnol. 5, 186–189 (2010).

    Article  ADS  Google Scholar 

  18. Ribeiro-Palau, R. et al. Quantum Hall resistance standard in graphene devices under relaxed experimental conditions. Nat. Nanotechnol. 10, 965–971 (2015).

    Article  ADS  Google Scholar 

  19. Lafont, F. et al. Quantum Hall resistance standards from graphene grown by chemical vapour deposition on silicon carbide. Nat. Commun. 6, 6806 (2015).

    Article  ADS  Google Scholar 

  20. Panna, A. R. et al. Graphene quantum Hall effect parallel resistance arrays. Phys. Rev. B 103, 075408 (2021).

    Article  ADS  Google Scholar 

  21. Scherer, H. & Camarota, B. Quantum metrology triangle experiments: a status review. Meas. Sci. Technol. 23, 124010 (2012).

    Article  ADS  Google Scholar 

  22. Kaneko, N.-H., Nakamura, S. & Okazaki, Y. A review of the quantum current standard. Meas. Sci. Technol. 27, 032001 (2016).

    Article  ADS  Google Scholar 

  23. Delahaye, F. & Jeckelmann, B. Revised technical guidelines for reliable d.c. measurements of the quantized Hall resistance. Metrologia 40, 217–223 (2003).

    Article  ADS  Google Scholar 

  24. Mogi, M. et al. Magnetic modulation doping in topological insulators toward higher-temperature quantum anomalous Hall effect. Appl. Phys. Lett. 107, 182401 (2015).

    Article  ADS  Google Scholar 

  25. Kawamura, M. et al. Current-driven instability of the quantum anomalous Hall effect in ferromagnetic topological insulators. Phys. Rev. Lett. 119, 016803 (2017).

    Article  ADS  Google Scholar 

  26. Pan, L. et al. Probing the low-temperature limit of the quantum anomalous Hall effect. Sci. Adv. 6, eaaz3595 (2020).

    Article  ADS  Google Scholar 

  27. Witt, T. J. Using the Allan variance and power spectral density to characterize d.c. nanovoltmeters. IEEE Trans. Instrum. Meas. 50, 445–448 (2001).

    Article  Google Scholar 

  28. Ou, Y. et al. Enhancing the quantum anomalous Hall effect by magnetic codoping in a topological insulator. Adv. Mater. 30, 1703062 (2018).

    Article  Google Scholar 

  29. Katmis, F. et al. A high-temperature ferromagnetic topological insulating phase by proximity coupling. Nature 533, 513–516 (2016).

    Article  ADS  Google Scholar 

  30. Mogi, M. et al. Large anomalous Hall effect in topological insulators with proximitized ferromagnetic insulators. Phys. Rev. Lett. 123, 016804 (2019).

    Article  ADS  Google Scholar 

  31. Watanabe, R. et al. Quantum anomalous Hall effect driven by magnetic proximity coupling in all-telluride based heterostructure. Appl. Phys. Lett. 115, 102403 (2019).

    Article  ADS  Google Scholar 

  32. Deng, Y. et al. Quantum anomalous Hall effect in intrinsic magnetic topological insulator MnBi2Te4. Science 367, 895–900 (2020).

    Article  ADS  Google Scholar 

  33. Liu, C. et al. Robust axion insulator and Chern insulator phases in a two-dimensional antiferromagnetic topological insulator. Nat. Mater. 19, 522–527 (2020).

    Article  ADS  Google Scholar 

  34. Sharpe, A. L. et al. Emergent ferromagnetism near three-quarters filling in twisted bilayer graphene. Science 365, 605–608 (2019).

    Article  ADS  Google Scholar 

  35. Serlin, M. et al. Intrinsic quantized anomalous Hall effect in a moiré heterostructure. Science 367, 900–903 (2019).

    Article  ADS  Google Scholar 

  36. BIPM Key Comparison Database. https://www.bipm.org/kcdb/comparison?id=430 (2021).

  37. Delahaye, F. Series and parallel connection of multiterminal quantum Hall-effect devices. J. Appl. Phys. 73, 7914–7920 (1993).

Download references

Acknowledgements

This research was supported by JST CREST (no. JPMJCR16F1 (M. Kawasaki)); MEXT/JSPS KAKENHI grant nos. JP15H05867 (M. Kawamura), JP15H05853 (A.T.), JP17H04846 (R.Y.), JP18H04229 (R.Y.), JP18H01155 (M. Kawamura), JP18H01156 (Y.O.) and JP18H05258 (N.-H.K.); and RIKEN-AIST Joint Research Fund (Y.O. and M. Kawamura).

Author information

Authors and Affiliations

  1. National Institute of Advanced Industrial Science and Technology (AIST), National Metrology Institute of Japan (NMIJ), Tsukuba, Ibaraki, Japan

    Yuma Okazaki, Takehiko Oe, Shuji Nakamura, Shintaro Takada & Nobu-Hisa Kaneko

  2. RIKEN Center for Emergent Matter Science (CEMS), Wako, Japan

    Minoru Kawamura, Ryutaro Yoshimi, Kei S. Takahashi, Masashi Kawasaki & Yoshinori Tokura

  3. Department of Applied Physics and Quantum-phase Electronics Center (QPEC), University of Tokyo, Tokyo, Japan

    Masataka Mogi, Masashi Kawasaki & Yoshinori Tokura

  4. Institute for Materials Research (IMR), Tohoku University, Sendai, Japan

    Atsushi Tsukazaki

  5. Tokyo College, University of Tokyo, Tokyo, Japan

    Yoshinori Tokura

Authors
  1. Yuma Okazaki
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Takehiko Oe
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Minoru Kawamura
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ryutaro Yoshimi
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Shuji Nakamura
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Shintaro Takada
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Masataka Mogi
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Kei S. Takahashi
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Atsushi Tsukazaki
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. Masashi Kawasaki
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Yoshinori Tokura
    View author publications

    You can also search for this author in PubMed Google Scholar

  12. Nobu-Hisa Kaneko
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Y.T. and N.-H.K. conceived the project. R.Y. and M. Kawamura fabricated the QAHE samples and characterized their general transport properties with the help of M.M., K.S.T., A.T. and M. Kawasaki. Y.O. conducted the precision measurements and data analysis with the assistance of T.O., S.N. and S.T. Y.O. and M. Kawamura wrote the draft. All the authors discussed the results and commented on the manuscript.

Corresponding authors

Correspondence to Yuma Okazaki or Minoru Kawamura.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks the anonymous reviewers for their contribution to the peer review of this work

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Extended data

Extended Data Fig. 1 Device Structure.

Photo-mask pattern of the mesa and the Ohmic contacts for the 800 μm-wide Hall bar device.

Extended Data Fig. 2 Transport properties of the FMTI film.

a, Optical microscope image of a 400 μm-wide Hall bar. S, D, and G denote source, drain contacts and a top-gate electrode, respectively. Vy was measured between the electrodes U2 and L2, and Vx was measured between L2 and L3. b, Gate voltage dependence of ρyx and ρxx with a measurement current I = 1.0 μA. The data were taken at B = 0 T after magnetizing the sample at B = 1.0 T. The value of ρxx was multiplied by 10 for clarity. c, d, Field- and zero-field cooling traces of ρyx and ρxx. Magnetic field B = 0.2 T in the field cooling measurement.

Extended Data Fig. 3 Gate dependence of ρxx.

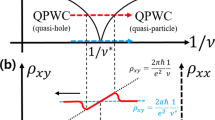
Gate voltage VG dependence of ρxx measured at I = 0.5 μA using the upper pair of the voltage contacts (U1-U5) of the 800 μm-wide Hall bar device. The values of ρxx are suppressed around − 2 V < VG < 0.5 V, indicating that the QAHE is well developed at VG = 0 V. Note that the measurement was performed below 0.5 V because a relatively large gate leakage was observed above 1.0 V.

Extended Data Fig. 4 One-to-one resistance bridge between the two QHEs.

a, b, Simplified circuit diagrams in the normal (a) and swapped (b) configurations. Here RX is RQAHE (RQHE1) and RS is RQHE1 + RQHE2(RQHE2) for the QAHE-QHE (QHE-QHE) comparison. c, Detailed circuit diagram of the resistance bridge for the comparison between QHE1 and QHE2.

Extended Data Fig. 5 Measurement sequence.

Single batch on-off sequence of the current I and the resulting measured voltage difference Vd. The current ramping rate was 0.16 μA/s. In the measurements of Fig. 4a, the single batch of the sequence took 45 s for I = 0.8 μA, and it took about 110 hours in total [45 s (single batch) × 2200 (number of points) × 2 (current polarity change) × 2 (circuit swapping)].

Extended Data Fig. 6 Uncertainty evaluation.

Relative uncertainty from possible source components in the normalized deviation ΔR/RK for the QAHE-QHE comparison with N = 2200 and I = 0.8 μA, and for the QHE-QHE comparison with N = 50 and I = 10 μA. In both cases, the dominant component of the uncertainty is dispersion from random effect.

Extended Data Fig. 7 Comparison of the relevant parameters.

Material: the materials and the layer structure of the FMTI film (the capping layer and the gate electrode are omitted for clarity). ΔR/RK: the normalized deviation in the values of the quantized anomalous Hall resistance. The current value I used for the precise measurement is also appended. (*Hall resistance measurements performed at I = 0.005 μA and 0.01 μA. **obtained by averaging the Hall resistance measurements for I < 0.09 μA.) W: Hall bar width. T0: activation energy (***measured in the 400 μm-wide Hall bar as described in Methods). ρxx: longitudinal resistivity. Method: experimental technique for the precision measurement of the Hall resistance.

Source data

Source Data Fig. 2

Numerical source data for Fig. 2.

Source Data Fig. 3

Numerical source data for Fig. 3c.

Source Data Fig. 4

Numerical source data for Fig. 4.

Rights and permissions

Reprints and Permissions

About this article

Verify currency and authenticity via CrossMark

Cite this article

Okazaki, Y., Oe, T., Kawamura, M. et al. Quantum anomalous Hall effect with a permanent magnet defines a quantum resistance standard. Nat. Phys. 18, 25–29 (2022). https://doi.org/10.1038/s41567-021-01424-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-021-01424-8

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing