Introduction

In the absence of disorder, the electrostatic force exerted by an electric field E on an electron fluid balances the Lorentz force from a magnetic field B so long as the fluid velocity v = cE × B/B2. Consequently, one expects the Hall conductance of a two dimensional electron gas to vary linearly with electron density, n, i.e., to exhibit the “classical” Hall effect: σxy = nec/B = (e2/h)ν, where the “filling factor” ν ≡ nϕ0/B and ϕ0 = hc/e is the quantum of flux. In contrast, where the quantum Hall effect arises, the Hall conductance remains constant, σxy = (e2/h)ν⋆, for a finite range of ν on either side of ν = ν⋆, where ν⋆ is a rational number corresponding to a quantized filling factor. Combining these observations, it is generally asserted that disorder is necessary for the quantum Hall effect. Instead, we show that the occurrence of a QPWC1,2,3,4,5 for sufficiently small ∣ν − ν⋆∣ results in a quantized Hall plateau, even in the absence of disorder. To make the considerations concrete, we derive the quantized Hall conductance both in a system with disorder, taking the limit as the disorder strength tends to zero and as the system size to infinity, and in a finite size system with no disorder but with a finite pinning potential at the edges. We also discuss the finite T phase diagram and how thermal melting of the QPWC restores the classical behavior.

Classical Hall effect

The derivation of the “classical” Hall effect typically invokes Galilean invariance6,7. Although higher-order corrections to effective mass theory do not have Galilean symmetry8, it is straightforward to show that translation invariance alone is sufficient for the Hall conductivity to take its classical value (See Supplementary Discussion). The observation of quantized Hall plateau, on the other hand, is commonly associated with the explicit breaking of translational symmetry by disorders9,10,11,12.

The occurrence of QPWC

In the absence of disorder, however, there is one other possible source of a quantum Hall plateau: spontaneous breaking of translational symmetry. Indeed, the presence of long-range Coulomb interactions makes this inevitable close to a quantized filling factor. Specifically, assuming the existence of a quantum Hall fluid at ν = ν⋆, for δν ≡ (ν − ν⋆) small, the system consists of a small density

$${n}_{{\rm{qp}}}=\left(\frac{B}{{\phi }_{0}}\right)\left|\frac{e}{{e}^{\star }}\right|| \delta \nu |$$
(1)

of quasi-particles or quasi-holes (depending on the sign of δν), where e* is the charge of a quasi-particle (i.e., \(\left|{e}^{\star }/e\right|=1\) for the integer quantum Hall effect and a suitable rational fraction for the fractional quantum Hall effect). Thus, for ∣δν∣ sufficiently small, the spacing between quasi-particles is large compared to the magnetic length, \(\ell =\sqrt{{\phi }_{0}/2\pi B}\), and they behave as classical particles with fixed guiding-centers. The result is that the quasi-particles form a Wigner crystal below a transition temperature,

$${T}_{{\rm{qpwc}}}={A}_{{\rm{wc}}}\,\frac{{({e}^{* })}^{2}}{4\pi \epsilon }{\left[\pi {n}_{qp}\right]}^{1/2} \sim | \delta \nu {| }^{1/2}\,,$$
(2)

where ϵ is the dielectric constant and Awc ≈ 1/12813,14,15. For T < Tqpwc the (QPWC) has quasi-long-range order and a finite shear modulus; it only has true long-range order at T = 0. The resulting phase diagram for the ‘ideal’ (i.e. zero disorder) quantum Hall system in the neighborhood of ν⋆ is thus as shown in Fig. 1a. Importantly, at low T in the crystalline state, the lowest lying current carrying excitations are interstitials, whose density is exponentially activated as

$${n}_{{\rm{int}}} \sim \exp [-{{\Delta }}/T]\,\,{\rm{with}}\,\,{{\Delta }}={A}_{{\rm{int}}}\,\frac{{({e}^{\star })}^{2}}{4\pi \epsilon }{\left[{n}_{{\rm{qp}}}\right]}^{1/2},$$
(3)

where Aint ≈ 0.13616,17.

Fig. 1: Finite T phase diagram of “ideal” quantum Hall effect.
figure 1

a Schematic phase diagram in the neighborhood of ν = ν⋆ (quantized filling factor) in the limit of vanishing disorder. b Resistivity tensor as a function of ν at three fixed temperatures, as indicated in panel a. At zero temperature (blue) the Hall resistivity is strictly quantized, whereas at high temperature (black) the QPWC has melted and the Hall resistivity takes its classical value. At intermediate temperatures (red), the Hall resistivity shows sharp crossovers from the classical value to the quantized one as ν moves away from ν*. Moreover, the longitudinal resistivity ρxx is very close to zero deep in the QPWC phase or near ν* but becomes finite away from those regions.

Full size image

The width of the QPWC phase can be estimated using the composite fermion approach18. In the absence of Landau level mixing, it was estimated in ref. 19 that an electron WC has lower energy than any quantum Hall liquid for ν < ν0 where 1/7 < ν0 < 1/5; Landau level mixing will generally tend to lead to a larger value of ν0. In the mean-field treatment of composite fermions with 2m flux quanta attached to each electron, an electron state with ν = (p + f)/[1 + 2m(p + f)] corresponds to a state with p fully filled and one f-filled Landau level of composite fermions. (m and p are integers and 0 < f < 1.) This reasoning leads to the conclusion that if there is a stable FQH liquid at ν⋆ = p/[1 + 2mp], there should be a stable QPWC for

$$\frac{p-{\nu }_{0}}{1+2m(p-{\nu }_{0})}\,<\,\nu \,<\,\frac{p+{\nu }_{0}}{1+2m(p+{\nu }_{0})}.$$
(4)

For instance, taking ν0 ≈ 1/719 the QPWC associated with the ν = 1/3 plateau would extend over the range 1/3 − 0.018 < ν < 1/3 + 0.014 in the absence of disorder.

The pinning of QPWC and quantum Hall effect

Because a Wigner crystal of electrons is insulating in the pinning regime20,21,22,23,24, the DC conductivity of the QPWC must come exclusively from the quantum Hall condensate. As in the case of any charge-density wave (CDW)25,26,27,28,29,30, the one subtlety concerns the possible contribution of a sliding state of the QPWC. We analyze this problem in two distinct ways:

Firstly, we consider a sequence of “real” systems with disorder strength, D, as D → 0. As is well known, disorder in two dimension both destroys long-range CDW order31—i.e., it rounds the transition at Tqpwc—and pins the CDW25,26. This means that for a QPWC with D ≠ 0, there is a quantized Hall response in a finite range of filling factors: σxy → (e2/h)ν⋆ as T → 0 for ν ∈ [ν* − δν−, ν* + δν+] where δν±(D) approach finite positive values deduced from Eq. (4) as D → 0. On the other hand, for field strengths greater than a critical field, E⋆, the QPWC depins (slides) and contributes to the current. The depinning field in the weak disorder limit can be estimated using the arguments of Lee and Rice25,27:

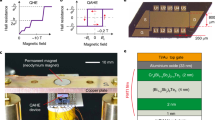
$${E}^{\star }(D)\approx \frac{\epsilon }{{({e}^{\star })}^{3}}\,{D}^{2},\,$$
(5)

where D2 is the mean square pinning energy per unit cell of the QPWC. In other words, for ever smaller D, in order to remain in the linear response regime, we must restrict measurements of the conductivity to an increasingly smaller range of field strengths, VH/L = E ≪ E⋆(D), where VH is the Hall voltage and L is the width of the device as in Fig. 2. We note that even though the critical current density \({j}_{x}^{* }={\rho }_{xy}{E}^{* }\) goes to 0 as D → 0, the critical current \({I}_{x}^{* } \sim L{D}^{2}\) could remain finite if we simultaneously take L → ∞ in an appropriate manner. To obtain a sense of the expected magnitude of the critical current, we make a crude estimate \({D}^{2} \sim {\left(\frac{e{e}^{* }}{4\pi \epsilon \ell }\right)}^{2}\times \left({n}_{{\rm{i}}}{\ell }^{2}\right)\) where ni is the density of impurities. In the cleanest two dimensional electron system as of today32 (in a d = 30 nm width GaAs quantum well) ni = 3 × 107 cm−2; for a 2D electron density n = 1 × 1011 cm−2 and B = 12T, which corresponds to ν = 1/3, this estimate yields a critical Hall voltage \({V}_{H}^{* } \sim 34\,\,{\rm{mV}}\) and correspondingly, for a sample with linear size Lx = 0.4 cm, to a critical current Ix ~ 0.43 μA.

Fig. 2: Schematic of a Hall bar.
figure 2

As the current flows through the current leads, the longitudinal and Hall voltages are measured at the voltage leads. L is the linear size of the sample.

Full size image

Alternatively, consider measuring the Hall response in an “ideal” (D = 0) sample of finite size as in Fig. 2. Here the current enters and leaves through current leads at either end, and the longitudinal and Hall voltages are measured at the voltage leads on the sides of the device. Because the QPWC has a finite shear modulus, it cannot smoothly flow through the current leads at either end of the device—it is pinned by the edges of the device. On the other hand, if the current exceeds a critical value, the crystal will be sheared, and a portion of the QPWC will begin to flow through the device. As in the disordered case, this means that the QPWC is pinned for small enough E. Now, assuming finite pinning potential at the edges, the critical field scales to 0 as E* ~ 1/L in the thermodynamic limit, but the critical Hall voltage and current remain finite even in the limit L → ∞: \({V}_{H}^{* }={\rho }_{xy}{I}_{x}^{* }={E}^{* }L\). Again, so long as measurements are carried out with \({I}_{x}\ll {I}_{x}^{* }\), a quantized Hall response will be observed (to an exponential accuracy with small corrections, \(\delta {\sigma }_{xy} \sim \exp [-L/\ell ]\)). Importantly, the width of the plateau, [δν− + δν+], approaches a constant as L → ∞.

The melting of QPWC

The role of the QPWC can (in principle) be unambiguously inferred from the thermal evolution even in the presence of weak disorder. For weak enough disorder, one expects a sharp crossover in conductivity at T = Tqpwc(δν): for T > Tqpwc, the Hall response should be close to its “classical” value, while for T < Tqpwc the quantum Hall effect should be seen up to exponentially small corrections33 proportional to the concentration of interstitials, nint. Since Tqpwc(δν) vanishes as δν → 0 as given in Eq. (2), the finite T Hall response should exhibit a peculiar reentrant behavior as a function of ν as shown in Fig. 1b. The temperature below which this reentrant behavior can be seen is evaluated to be Tqpwc ≈ 0.02K, where we take ν = 1/3 − 0.009( = 1/3 − δν−/2) and n = 1011 cm−2 in Eq. (2).