Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Tomonaga–Luttinger liquid in the edge channels of a quantum spin Hall insulator

Nature Physics volume 16, pages 47–51 (2020)Cite this article

Subjects

Abstract

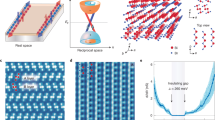
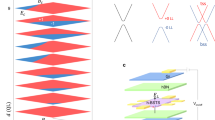
Quantum spin Hall insulators are two-dimensional materials that host conducting helical electron states strictly confined to the one-dimensional boundaries. These edge channels are protected by time-reversal symmetry against single-particle backscattering, opening new avenues for spin-based electronics and computation. However, the effect of the interelectronic Coulomb repulsion also has to be taken into account, as two-particle scattering is not impeded by topological protection and may strongly affect the edge state conductance. Here, we explore the impact of electronic correlations on highly localized edge states of the unique quantum spin Hall material bismuthene on SiC(0001) (ref. 1). Exploiting the advantage of having an accessible monolayer substrate system, we use STM/STS to visualize the close-to-perfect one-dimensional confinement of the edge channels and scrutinize their suppressed density of states at the Fermi level. On the basis of the observed spectral behaviour and its universal scaling with energy and temperature, we demonstrate the correspondence with a (helical) Tomonaga–Luttinger liquid. In particular, the extracted interaction parameter K is directly relevant to the fundamental question of the temperatures at which the quantized conductance (a hallmark of quantum spin Hall materials) will become obscured by correlations2.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Strongly confined 1D metallic channels at bismuthene armchair edges.
Fig. 2: Power-law suppression of the ZBA.
Fig. 3: Temperature dependence of the ZBA.
Fig. 4: Universal scaling of the ZBA as a hallmark of a TLL.

Data availability

The data that support the plots within this paper and other findings of this study are available from the corresponding author on reasonable request.

References

  1. Reis, F. et al. Bismuthene on a SiC substrate: a candidate for a high-temperature quantum spin Hall material. Science 357, 287–290 (2017).

    Article  ADS  MathSciNet  Google Scholar 

  2. Li, T. et al. Observation of a helical Luttinger liquid in InAs/GaSb quantum spin Hall edges. Phys. Rev. Lett. 115, 136804 (2015).

    Article  ADS  Google Scholar 

  3. Kane, C. L. & Mele, E. J. Quantum spin Hall effect in graphene. Phys. Rev. Lett. 95, 226801 (2005).

    Article  ADS  Google Scholar 

  4. Kane, C. L. & Mele, E. J. Z 2 topological order and the quantum spin Hall effect. Phys. Rev. Lett. 95, 146802 (2005).

    Article  ADS  Google Scholar 

  5. König, M. et al. Quantum spin Hall insulator state in HgTe quantum wells. Science 318, 766–770 (2007).

    Article  ADS  Google Scholar 

  6. Knez, I., Du, R.-R. & Sullivan, G. Evidence for helical edge modes in inverted InAs/GaSb quantum wells. Phys. Rev. Lett. 107, 136603 (2011).

    Article  ADS  Google Scholar 

  7. Wu, S. et al. Observation of the quantum spin Hall effect up to 100 Kelvin in a monolayer crystal. Science 359, 76–79 (2018).

    Article  ADS  MathSciNet  Google Scholar 

  8. Tang, S. et al. Quantum spin Hall state in monolayer 1T′-WTe2. Nat. Phys. 13, 683–687 (2017).

    Article  Google Scholar 

  9. Wu, C., Bernevig, B. A. & Zhang, S.-C. Helical liquid and the edge of quantum spin Hall systems. Phys. Rev. Lett. 96, 106401 (2006).

    Article  ADS  Google Scholar 

  10. Xu, C. & Moore, J. E. Stability of the quantum spin Hall effect: effects of interactions, disorder, and ℤ2 topology. Phys. Rev. B 73, 045322 (2006).

    Article  ADS  Google Scholar 

  11. Li, G. et al. Theoretical paradigm for the quantum spin Hall effect at high temperatures. Phys. Rev. B 98, 165146 (2018).

    Article  ADS  Google Scholar 

  12. Meyer, Chr, Klijn, J., Morgenstern, M. & Wiesendanger, R. Direct measurement of the local density of states of a disordered one-dimensional conductor. Phys. Rev. Lett. 91, 076803 (2003).

    Article  ADS  Google Scholar 

  13. Pauly, C. et al. Subnanometre-wide electron channels protected by topology. Nat. Phys. 11, 338–343 (2015).

    Article  Google Scholar 

  14. Voit, J. Charge–spin separation and the spectral properties of Luttinger liquids. Phys. Rev. B 47, 6740–6743 (1993).

    Article  ADS  Google Scholar 

  15. Haldane, F. D. M. ‘Luttinger liquid theory’ of one-dimensional quantum fluids. I. properties of the Luttinger model and their extension to the general 1D interacting spinless Fermi gas. J. Phys. C. Solid State 14, 2585–2609 (1981).

    Article  ADS  Google Scholar 

  16. Jompol, Y. et al. Probing spin–charge separation in a Tomonaga–Luttinger liquid. Science 325, 597–601 (2009).

    Article  ADS  Google Scholar 

  17. Bockrath, M. et al. Luttinger-liquid behaviour in carbon nanotubes. Nature 397, 598–601 (1999).

    Article  ADS  Google Scholar 

  18. Aleshin, A. N., Lee, H. J., Park, Y. W. & Akagi, K. One-dimensional transport in polymer nanofibers. Phys. Rev. Lett. 93, 196601 (2004).

    Article  ADS  Google Scholar 

  19. Blumenstein, C. et al. Atomically controlled quantum chains hosting a Tomonaga–Luttinger liquid. Nat. Phys. 7, 776–780 (2011).

    Article  Google Scholar 

  20. Chang, A. M., Pfeiffer, L. N. & West, K. W. Observation of chiral Luttinger behavior in electron tunneling into fractional quantum Hall edges. Phys. Rev. Lett. 77, 2538–2541 (1996).

    Article  ADS  Google Scholar 

  21. Hohenadler, M. & Assaad, F. F. Luttinger liquid physics and spin-flip scattering on helical edges. Phys. Rev. B 85, 081106 (2012).

    Article  ADS  Google Scholar 

  22. Braunecker, B., Bena, C. & Simon, P. Spectral properties of Luttinger liquids: a comparative analysis of regular, helical, and spiral Luttinger liquids. Phys. Rev. B 85, 035136 (2012).

    Article  ADS  Google Scholar 

  23. Eggert, S. Scanning tunneling microscopy of a Luttinger liquid. Phys. Rev. Lett. 84, 4413–4416 (2000).

    Article  ADS  Google Scholar 

  24. Fisher, M. P. A. & Dorsey, A. T. Dissipative quantum tunneling in a biased double-well system at finite temperatures. Phys. Rev. Lett. 54, 2647–2647 (1985).

    Article  ADS  Google Scholar 

  25. Bartosch, L. & Kopietz, P. Zero bias anomaly in the density of states of low-dimensional metals. Eur. Phys. J. B 28, 29–36 (2002).

    Article  ADS  Google Scholar 

  26. Hanna, A. E. & Tinkham, M. Variation of the Coulomb staircase in a two-junction system by fractional electron charge. Phys. Rev. B 44, 5919–5922 (1991).

    Article  ADS  Google Scholar 

  27. Devoret, M. H. et al. Effect of the electromagnetic environment on the Coulomb blockade in ultrasmall tunnel junctions. Phys. Rev. Lett. 64, 1824–1827 (1990).

    Article  ADS  Google Scholar 

  28. Ming, F., Smith, T. S., Johnston, S., Snijders, P. C. & Weitering, H. H. Zero-bias anomaly in nanoscale hole-doped mott insulators on a triangular silicon surface. Phys. Rev. B 97, 075403 (2018).

    Article  ADS  Google Scholar 

  29. Väyrynen, J. I., Geissler, F. & Glazman, L. I. Magnetic moments in a helical edge can make weak correlations seem strong. Phys. Rev. B 93, 241301 (2016).

    Article  ADS  Google Scholar 

  30. Teo, J. C. Y. & Kane, C. L. Critical behavior of a point contact in a quantum spin Hall insulator. Phys. Rev. B 79, 235321 (2009).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

We thank B. Trauzettel, M. Bode, A. Kowalewski and J. Maciejko for useful discussions. This work was supported by the DFG through the Würzburg-Dresden Cluster of Excellence on Complexity and Topology in Quantum Matter (EXC 2147, project 39085490), the Collaborative Research Center SFB 1170 ‘ToCoTronics’ in Würzburg and the SPP 1666 Priority Programme ‘Topological Insulators’. We are also grateful for support from the ERC through starting grant ERC-StG-Thomale-336012 ‘Topolectrics’.

Author information

Authors and Affiliations

  1. Physikalisches Institut and Würzburg-Dresden Cluster of Excellence ct.qmat, Universität Würzburg, Würzburg, Germany

    R. Stühler, F. Reis, J. Schäfer & R. Claessen

  2. Institut für Theoretische Physik und Astrophysik and Würzburg-Dresden Cluster of Excellence ct.qmat, Universität Würzburg, Würzburg, Germany

    T. Müller, T. Helbig, T. Schwemmer & R. Thomale

Authors
  1. R. Stühler
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Reis
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. T. Müller
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. T. Helbig
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. T. Schwemmer
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. R. Thomale
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. J. Schäfer
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. R. Claessen
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

R.S. and F.R. carried out the measurements. R.S. analysed the data and made the figures. T.M., T.H., T.S and R.T. developed the theory for the helical edge with dielectric screening. J.S. conceived the experiment. R.S., F.R., J.S. and R.C. wrote the text, and all authors contributed to critical discussion of the data.

Corresponding author

Correspondence to J. Schäfer.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information Nature Physics thanks Ward Plummer and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.

Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary Figs. 1–9, text and reference.

Supplementary Video

Continuous evolution of the DOS with energy and constant dI/dV scale.

Source data

Source data for Fig. 1c

Contains source data for Figure 1c.

Source data for Fig. 1d, left panel

Contains source data for Figure 1d left panel.

Source data for Fig. 1d, right panel

Contains source data for Figure 1d right panel.

Source data for Fig. 1d, right, Fourier filtered

Contains source data for Figure 1d right panel Fourier filtered.

Source data for Fig. 2a

Contains source data for Figure 2a.

Source data for Fig. 3a

Contains source data for Figure 3a.

Source data for Fig. 3b

Contains source data for Figure 3b.

Source data for Fig. 3c

Contains source data for Figure 3c.

Source data for Fig. 4

Contains source data for Figure 4.

Rights and permissions

Reprints and Permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Stühler, R., Reis, F., Müller, T. et al. Tomonaga–Luttinger liquid in the edge channels of a quantum spin Hall insulator. Nat. Phys. 16, 47–51 (2020). https://doi.org/10.1038/s41567-019-0697-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-019-0697-z

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing