Abstract
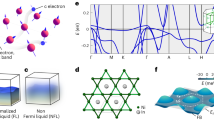
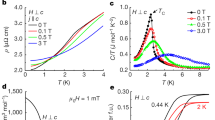
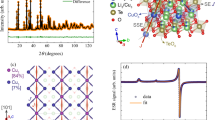
Strange-metal phenomena often develop at the border of antiferromagnetic order in strongly correlated metals1. Previous work established that they can originate from the fluctuations anchored by the quantum-critical point associated with a continuous quantum phase transition out of the antiferromagnetic order2,3,4. What is still unclear is how these phenomena can be associated with a potential new phase of matter at zero temperature. Here, we show that magnetic frustration of the 4f local moments in the distorted kagome intermetallic compound cerium palladium aluminium gives rise to such a paramagnetic quantum-critical phase. Our discovery motivates a design principle for strongly correlated metallic states with unconventional excitations.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding author P.S. upon reasonable request.
References
Keimer, B. & Moore, J. E. The physics of quantum materials. Nat. Phys. 13, 1045–1055 (2017).
Coleman, P. & Schofield, A. Quantum criticality. Nature 433, 226–229 (2005).
von Lőhneysen, H., Rosch, A., Vojta, M. & Wőlfle, P. Fermi-liquid instabilities at magnetic quantum phase transitions. Rev. Mod. Phys. 79, 1015–1075 (2007).
Gegenwart, P., Si, Q. & Steglich, F. Quantum criticality in heavy-fermion metals. Nat. Phys. 4, 186–197 (2008).
Balents, L. Spin liquids in frustrated magnets. Nature 464, 199–208 (2010).
Tokiwa, Y., Ishikawa, J. J., Nakatsuji, S. & Gegenwart, P. Quantum criticality in a metallic spin liquid. Nat. Mater. 13, 356–359 (2014).
Tokiwa, Y., Stingl, C., Kim, M.-S., Takabatake, T. & Gegenwart, P. Characteristic signatures of quantum criticality driven by geometrical frustration. Sci. Adv. 1, e1500001 (2015).
Si, Q. Global magnetic phase diagram and local quantum criticality in heavy fermion metals. Phys. B 378–380, 23–27 (2006).
Si, Q. Quantum criticality and global phase diagram of magnetic heavy fermions. Phys. Stat. Sol. B 247, 476–484 (2010).
Coleman, P. & Nevidomskyy, A. H. Frustration and the Kondo effect in heavy fermion materials. J. Low Temp. Phys. 161, 182–202 (2010).
Stewart, G. R. Non-Fermi-liquid behavior in d- and f-electron metals. Rev. Mod. Phys. 73, 797–855 (2001).
Doniach, S. Kondo lattice and weak antiferromagnetism. Phys. B 91, 231–234 (1977).
Friedemann, S. et al. Detaching the antiferromagnetic quantum critical point from the Fermi-surface reconstruction in YbRh2Si2. Nat. Phys. 5, 465–469 (2009).
Custers, J. et al. Evidence for a non-Fermi-liquid phase in Ge-substituted YbRh2Si2. Phys. Rev. Lett. 104, 186402 (2010).
Zhao, H. C. et al. Temperature-field phase diagram of geometrically frustrated CePdAl. Phys. Rev. B 94, 235131 (2016).
Lucas, S. et al. Entropy evolution in the magnetic phases of partially frustrated CePdAl. Phys. Rev. Lett. 118, 107204 (2017).
Dolores Núñez-Regueiro, M., Lacroix, C. & Canals, B. Magnetic ordering in the frustrated Kondo lattice compound CePdAl. Phys. C 282–287, 1885–1886 (1997).
Zhang, J. H. et al. Kondo destruction in a quantum paramagnet with magnetic frustration. Phys. Rev. B 97, 235117 (2018).
Goto, T., Hane, S., Umeo, K., Takabatake, T. & Isikawa, Y. Field-induced magnetic transitions and pressure-induced magnetic instability in CePdAl. J. Phys. Chem. Solids 63, 1159–1163 (2002).
Si, Q., Rabello, S., Ingersent, K. & Smith, J. L. Locally critical quantum phase transitions in strongly correlated metals. Nature 413, 804–808 (2001).
Coleman, P., Pepin, C., Si, Q. & Ramazashvili, R. How do Fermi liquids get heavy and die? J. Phys. Condens. Matter 13, 723–738 (2001).
Gegenwart, P. et al. Magnetic-field induced quantum critical point in YbRh2Si2. Phys. Rev. Lett. 89, 056402 (2002).
Fritsch, V. et al. Approaching quantum criticality in a partially geometrically frustrated heavy-fermion metal. Phys. Rev. B 89, 054416 (2014).
Sakai, A. et al. Signature of frustrated moments in quantum critical CePd1− xNixAl. Phys. Rev. B 94, 220405 (2016).
Tomita, T., Kuga, K., Uwatoko, Y., Coleman, P. & Nakatsuji, S. Strange metal without magnetic criticality. Science 349, 506–509 (2015).
Oike, H., Miyagawa, K., Taniguchi, H. & Kanoda, K. Pressure-induced Mott transition in an organic superconductor with a finite doping level. Phys. Rev. Lett. 114, 067002 (2015).
Paschen, S. et al. Hall-effect evolution across a heavy-fermion quantum critical point. Nature 432, 881–885 (2004).
Gegenwart, P. et al. Multiple energy scales at a quantum critical point. Science 315, 969–971 (2007).
Friedemann, S. et al. Fermi-surface collapse and dynamical scaling near a quantum-critical point. Proc. Natl Acad. Sci. USA 107, 14547–14551 (2010).
Custers, J. et al. Destruction of the Kondo effect in the cubic heavy-fermion compound Ce3Pd20Si6. Nat. Mater. 11, 189–194 (2012).
Senthil, T., Vojta, M. & Sachdev, S. Weak magnetism and non-Fermi liquids near heavy-fermion critical points. Phys. Rev. B 69, 035111 (2004).
Küchler, R. et al. Uniaxial stress tuning of geometrical frustration in a Kondo lattice. Phys. Rev. B 96, 241110 (2017).
Pixley, J. H., Yu, R. & Si, Q. Quantum phases of the Shastry–Sutherland Kondo lattice: implications for the global phase diagram of heavy-fermion metals. Phys. Rev. Lett. 113, 176402 (2014).
Ramires, A. & Coleman, P. Supersymmetric approach to heavy fermion systems. Phys. Rev. B 93, 035120 (2016).
Lee, P. A., Nagaosa, N. & Wen, X.-G. Doping a Mott insulator: physics of high-temperature superconductivity. Rev. Mod. Phys. 78, 17–85 (2006).
Acknowledgements
This work was supported by the Ministry of Science and Technology of China (grant nos 2017YFA0303100, 2015CB921303 and 2018YFA0305702), the National Natural Science Foundation of China (grant nos 11774404, 11474332, 11574377 and 11874400), the Chinese Academy of Sciences (grant nos XDB07020200, XDB25000000 and QYZDB-SSW-SLH013) and a fund from the Science and Technology on Surface Physics and Chemistry Laboratory (no. 01040117). Work at Augsburg was supported by the German Research Foundation (DFG) under the auspices of TRR 80 (no. 107745057), while work at Dresden was partly supported by the DFG Research Unit 960. The work at Rice University was supported in part by the NSF grant DMR-1920740 and the Robert A. Welch Foundation grant C-1411. Q.S. acknowledges the hospitality and support by a Ulam Scholarship from the Center for Nonlinear Studies at Los Alamos National Laboratory and the hospitality of the Aspen Center for Physics (NSF, PHY-1607611).
Author information
Authors and Affiliations
Contributions
P.S. and F.S. initiated the project; H.Z., J.Z., M.L. and P.S. performed the transport and susceptibility measurements under pressure; S.B., Y.T., P.G., S.Z. and G.C. performed the heat capacity measurements; J.C. calibrated the pressure cell and performed preliminary transport measurements under pressure; Y.I. prepared and oriented the single crystals; H.Z., J.Z., Y.Y., Q.S., F.S. and P.S. discussed the results and analysed the data; P.S., F.S. and Q.S. wrote the manuscript; all authors revised and approved the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Physics thanks Kazushi Kanoda, Gregory Stewart and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Figs. 1–9.
Rights and permissions
About this article
Cite this article
Zhao, H., Zhang, J., Lyu, M. et al. Quantum-critical phase from frustrated magnetism in a strongly correlated metal. Nat. Phys. 15, 1261–1266 (2019). https://doi.org/10.1038/s41567-019-0666-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-019-0666-6
This article is cited by
-
Hopping frustration-induced flat band and strange metallicity in a kagome metal
Nature Physics (2024)
-
Non-Fermi liquid behaviour in a correlated flat-band pyrochlore lattice
Nature Physics (2024)
-
Broadened quantum critical ground state in a disordered superconducting thin film
Nature Communications (2024)
-
The scaled-invariant Planckian metal and quantum criticality in Ce1−xNdxCoIn5
Nature Communications (2023)
-
Thermoelectric signature of quantum critical phase in a doped spin-liquid candidate
Nature Communications (2023)