Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Article
  • Published:

Topological chaos in active nematics

Nature Physics volume 15, pages 1033–1039 (2019)Cite this article

Subjects

Abstract

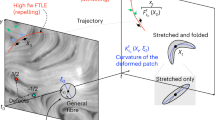
Active nematics are out-of-equilibrium fluids composed of rod-like subunits, which can generate large-scale, self-driven flows. We examine a microtubule-kinesin-based active nematic confined to two dimensions, exhibiting chaotic flows with moving topological defects. Applying tools from chaos theory, we investigate self-driven advection and mixing on different length scales. Local fluid stretching is quantified by the Lyapunov exponent. Global mixing is quantified by the topological entropy, calculated from both defect braiding and curve extension rates. We find excellent agreement between these independent measures of chaos, demonstrating that the extensile stretching between microtubules directly translates into macroscopic braiding of positive defects. Remarkably, increasing extensile activity (through ATP concentration) does not increase the dimensionless topological entropy. This study represents an application of chaotic advection to the emerging field of active nematics and quantification of the collective motion of an ensemble of defects (through topological entropy) in a liquid crystal.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Topological stirring of fluids.
Fig. 2: Measurement of separation rates in the active fluid.
Fig. 3: E-tec computation of topological entropy.
Fig. 4: Comparison of the four measures of chaos at 50 μM ATP concentration.
Fig. 5: Non-dimensionalized topological entropy and Lyapunov exponent are insensitive to motor activity.

Similar content being viewed by others

Data availability

The data that support the findings of this study are available from the corresponding authors on reasonable request.

Code availability

The MATLAB code for computing nematic director fields and topological defects is available on request from K.A.M. The E-tec code is available in Python from S.A.S. (smiths@mtholyoke.edu) on request.

References

  1. Toner, J. & Tu, Y. Long-range order in a two-dimensional dynamical XY model: how birds fly together. Phys. Rev. Lett. 75, 4326–4329 (1995).

    Article  ADS  Google Scholar 

  2. Katz, Y., Tunstrøm, K., Ioannou, C. C., Huepe, C. & Couzin, I. D. Inferring the structure and dynamics of interactions in schooling fish. Proc. Natl Acad. Sci. USA 108, 18720–18725 (2011).

    Article  ADS  Google Scholar 

  3. Buhl, J. et al. From disorder to order in marching locusts. Science 312, 1402–1406 (2006).

    Article  ADS  Google Scholar 

  4. Saw, T. B. et al. Topological defects in epithelia govern cell death and extrusion. Nature 544, 212–216 (2017).

    Article  ADS  Google Scholar 

  5. Kawaguchi, K., Kageyama, R. & Sano, M. Topological defects control collective dynamics in neural progenitor cell cultures. Nature 545, 327–331 (2017).

    Article  ADS  Google Scholar 

  6. Prost, J., Jülicher, F. & Joanny, J.-F. Active gel physics. Nat. Phys. 11, 111–117 (2015).

    Article  Google Scholar 

  7. Sokolov, A., Aranson, I. S., Kessler, J. O. & Goldstein, R. E. Concentration dependence of the collective dynamics of swimming bacteria. Phys. Rev. Lett. 98, 158102 (2007).

    Article  ADS  Google Scholar 

  8. Wensink, H. H. et al. Meso-scale turbulence in living fluids. Proc. Natl Acad. Sci. USA 109, 14308–14313 (2012).

    Article  ADS  Google Scholar 

  9. Dunkel, J. et al. Fluid dynamics of bacterial turbulence. Phys. Rev. Lett. 110, 228102 (2013).

    Article  ADS  Google Scholar 

  10. Palacci, J., Sacanna, S., Steinberg, A. P., Pine, D. J. & Chaikin, P. M. Living crystals of light-activated colloidal surfers. Science 339, 936–940 (2013).

    Article  ADS  Google Scholar 

  11. Yan, J. et al. Reconfiguring active particles by electrostatic imbalance. Nat. Mater. 15, 1095–1099 (2016).

    Article  ADS  Google Scholar 

  12. Narayan, V., Menon, N. & Ramaswamy, S. Nonequilibrium steady states in a vibrated-rod monolayer: tetratic, nematic, and smectic correlations. J. Stat. Mech. Theory Exp. 2006, P01005 (2006).

    Article  Google Scholar 

  13. Ndlec, F. J., Surrey, T., Maggs, A. C. & Leibler, S. Self-organization of microtubules and motors. Nature 389, 305–308 (1997).

    Article  ADS  Google Scholar 

  14. Schaller, V., Weber, C., Semmrich, C., Frey, E. & Bausch, A. R. Polar patterns of driven filaments. Nature 467, 73–77 (2010).

    Article  ADS  Google Scholar 

  15. Sanchez, T., Chen, D. T. N., DeCamp, S. J., Heymann, M. & Dogic, Z. Spontaneous motion in hierarchically assembled active matter. Nature 491, 431–434 (2012).

    Article  ADS  Google Scholar 

  16. Henkin, G., DeCamp, S. J., Chen, D. T. N., Sanchez, T. & Dogic, Z. Tunable dynamics of microtubule-based active isotropic gels. Phil. Trans. R. Soc. Lond. A 372, 20140142 (2014).

    Article  ADS  Google Scholar 

  17. Giomi, L. Geometry and topology of turbulence in active nematics. Phys. Rev. X 5, 031003 (2015).

    Google Scholar 

  18. DeCamp, S. J., Redner, G. S., Baskaran, A., Hagan, M. F. & Dogic, Z. Orientational order of motile defects in active nematics. Nat. Mater. 14, 1110–1115 (2015).

    Article  ADS  Google Scholar 

  19. Guillamat, P., Ignés-Mullol, J. & Sagués, F. Control of active liquid crystals with a magnetic field. Proc. Natl Acad. Sci. USA 113, 5498–5502 (2016).

    Article  ADS  Google Scholar 

  20. Doostmohammadi, A., Shendruk, T. N., Thijssen, K. & Yeomans, J. M. Onset of meso-scale turbulence in active nematics. Nat. Commun. 8, 15326 (2017).

    Article  ADS  Google Scholar 

  21. Guillamat, P., Ignés-Mullol, J. & Sagués, F. Taming active turbulence with patterned soft interfaces. Nat. Commun. 8, 564 (2017).

    Article  ADS  Google Scholar 

  22. Shendruk, T. N., Doostmohammadi, A., Thijssen, K. & Yeomans, J. M. Dancing disclinations in confined active nematics. Soft Matter 13, 3853–3862 (2017).

    Article  ADS  Google Scholar 

  23. Lemma, L. M., DeCamp, S. J., You, Z., Giomi, L. & Dogic, Z. Statistical properties of autonomous flows in 2D active nematics. Soft Matter 15, 3264–3272 (2019).

    Article  ADS  Google Scholar 

  24. Ramaswamy, S. The mechanics and statistics of active matter. Annu. Rev. Condens. Matter Phys. 1, 323–345 (2010).

    Article  ADS  Google Scholar 

  25. Marchetti, M. C. et al. Hydrodynamics of soft active matter. Rev. Mod. Phys. 85, 1143–1189 (2013).

    Article  ADS  Google Scholar 

  26. Aref, H. Stirring by chaotic advection. J. Fluid Mech. 143, 1–21 (1984).

    Article  ADS  MathSciNet  Google Scholar 

  27. Aref, H. et al. Frontiers of chaotic advection. Rev. Mod. Phys. 89, 025007 (2017).

    Article  ADS  MathSciNet  Google Scholar 

  28. Boyland, P. L., Aref, H. & Stremler, M. A. Topological fluid mechanics of stirring. J. Fluid Mech. 403, 277–304 (2000).

    Article  ADS  MathSciNet  Google Scholar 

  29. Muzzio, F. J., Swanson, P. D. & Ottino, J. M. The statistics of stretching and stirring in chaotic flows. Phys. Fluids A 3, 822–834 (1991).

    Article  ADS  MathSciNet  Google Scholar 

  30. Chaiken, J., Chevray, R., Tabor, M., Tan, Q. M. & Stuart, J. T. Experimental study of Lagrangian turbulence in a Stokes flow. Proc. R. Soc. Lond. A 408, 165–174 (1986).

    Article  ADS  Google Scholar 

  31. Aref, H. & Balachandar, S. Chaotic advection in a Stokes flow. Phys. Fluids 29, 3515–3521 (1986).

    Article  ADS  MathSciNet  Google Scholar 

  32. Vikhansky, A. Chaotic advection of finite-size bodies in a cavity flow. Phys. Fluids 15, 1830–1836 (2003).

    Article  ADS  Google Scholar 

  33. Gouillart, E., Thiffeault, J.-L. & Finn, M. D. Topological mixing with ghost rods. Phys. Rev. E 73, 036311 (2006).

    Article  ADS  MathSciNet  Google Scholar 

  34. Finn, M. D. & Thiffeault, J.-L. Topological optimization of rod-stirring devices. SIAM Rev. 53, 723–743 (2011).

    Article  MathSciNet  Google Scholar 

  35. Thiffeault, J.-L. Braids of entangled particle trajectories. Chaos 20, 017516 (2010).

    Article  ADS  MathSciNet  Google Scholar 

  36. Allshouse, M. R. & Thiffeault, J.-L. Detecting coherent structures using braids. Physica D 241, 95–105 (2012).

    Article  ADS  Google Scholar 

  37. Budišić, M. & Thiffeault, J.-L. Finite-time braiding exponents. Chaos 25, 087407 (2015).

    Article  ADS  MathSciNet  Google Scholar 

  38. Roberts, E., Sindi, S., Smith, S. A. & Mitchell, K. A. Ensemble-based topological entropy calculation (E-tec). Chaos 29, 013124 (2019).

    Article  ADS  MathSciNet  Google Scholar 

  39. Hirokawa, N., Noda, Y., Tanaka, Y. & Niwa, S. Kinesin superfamily motor proteins and intracellular transport. Nat. Rev. Mol. Cell Biol. 10, 682–696 (2009).

    Article  Google Scholar 

  40. Lu, W., Winding, M., Lakonishok, M., Wildonger, J. & Gelfand, V. I. Microtubule–microtubule sliding by kinesin-1 is essential for normal cytoplasmic streaming in Drosophila oocytes. Proc. Natl Acad. Sci. USA 113, E4995–E5004 (2016).

    Article  Google Scholar 

  41. Young, L.-S. in Entropy (eds Greven, A., Keller, G. & Warnecke, G.) 313–327 (Princeton Univ. Press, 2003).

  42. Visscher, K., Schnitzer, M. J. & Block, S. M. Single kinesin molecules studied with a molecular force clamp. Nature 400, 184–189 (1999).

    Article  ADS  Google Scholar 

  43. Lam, A. T., Curschellas, C., Krovvidi, D. & Hess, H. Controlling self-assembly of microtubule spools via kinesin motor density. Soft Matter 10, 8731–8736 (2014).

    Article  ADS  Google Scholar 

  44. Tan, A. J., Chapman, D. E., Hirst, L. S. & Xu, J. Understanding the role of transport velocity in biomotor-powered microtubule spool assembly. RSC Adv. 6, 79143–79146 (2016).

    Article  Google Scholar 

  45. Hemingway, E. J., Mishra, P., Marchetti, M. C. & Fielding, S. M. Correlation lengths in hydrodynamic models of active nematics. Soft Matter 12, 7943–7952 (2016).

    Article  ADS  Google Scholar 

  46. Thielicke, W. & Stamhuis, E. J. PIVlab—towards user-friendly, affordable and accurate digital particle image velocimetry in MATLAB. J. Open Res. Softw. 2, e30 (2014).

    Article  Google Scholar 

  47. Crocker, J. C. & Grier, D. G. Methods of digital video microscopy for colloidal studies. J. Colloid Interface Sci. 179, 298–310 (1996).

    Article  ADS  Google Scholar 

Download references

Acknowledgements

Our group is grateful to Z. Dogic for the generous contribution of microtubules and molecular motors and to L. Lemma for sample preparation. We also acknowledge useful discussions with S. Sindi. The authors acknowledge generous funding from the National Science Foundation, through several awards (DMR-1808926), NSF-CREST: Center for Cellular and Biomolecular Machines at UC Merced (HRD-1547848), and from the Brandeis Biomaterials facility MRSEC-1420382.

Author information

Authors and Affiliations

  1. Physics Department, University of California, Merced, CA, USA

    Amanda J. Tan, Ulyses Alvarado Olvera, Jorge Arteaga, Sam Fortini, Kevin A. Mitchell & Linda S. Hirst

  2. Applied Math Department, University of California, Merced, CA, USA

    Eric Roberts

  3. Department of Physics, Mount Holyoke College, South Hadley, MA, USA

    Spencer A. Smith

Authors
  1. Amanda J. Tan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Eric Roberts
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Spencer A. Smith
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ulyses Alvarado Olvera
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Jorge Arteaga
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Sam Fortini
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Kevin A. Mitchell
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Linda S. Hirst
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

L.S.H. and K.A.M. designed the study. A.J.T. carried out the experiments. A.J.T., E.R., S.A.S., U.A.O., J.A., S.F. and K.A.M. performed analysis. L.S.H., K.A.M. and A.J.T. wrote the paper.

Corresponding authors

Correspondence to Kevin A. Mitchell or Linda S. Hirst.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information: Nature Physics thanks Jordi Ignes-Mullol, Idan Tuval and Julia Yeomans for their contribution to the peer review of this work.

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary information

Supplementary Information

Supplementary material and Supplementary Figs. 1–6.

Reporting Summary

Supplementary Video 1

Fluorescence microscopy video of the microtubule/kinesin active nematic at 50 µM ATP concentration.

Supplementary Video 2

Fluorescence microscopy video of the active nematic with topological defects tracked at 50 µM ATP concentration. +1/2 defects are shown by white circles and –1/2 defects are shown by yellow triangles.

Supplementary Video 3

Optical microscopy bright-field video of the active nematic with tracked beads marked in blue and numbered. 50 µM ATP concentration.

Supplementary Video 4

Optical microscopy bright-field video of the active nematic at 50 µM ATP concentration with tracked beads marked and numbered. A nematic contour growing between a pair of tracked beads is shown in blue.

Supplementary Video 5

Fluorescence microscopy video of the active nematic at 50 µM ATP concentration with topological defects marked and tracked. +1/2 defects are shown by white circles and –1/2 defects are shown by yellow triangles. A nematic contour growing between a pair of tracked defects is shown in blue.

Supplementary Video 6

Braiding motion of the tracked +1/2 defects with E-tec triangulation showing the growth of the stretched mesh (red lines). 50 µM ATP concentration.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tan, A.J., Roberts, E., Smith, S.A. et al. Topological chaos in active nematics. Nat. Phys. 15, 1033–1039 (2019). https://doi.org/10.1038/s41567-019-0600-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s41567-019-0600-y

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing