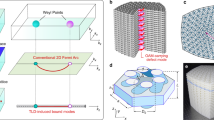
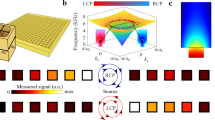
Abstract
Condensed-matter and other engineered systems, such as cold atoms1, photonic2 or phononic metamaterials3, have proved to be versatile platforms for the observation of low-energy counterparts of elementary particles from relativistic field theories. These include the celebrated Majorana modes4, as well as Dirac5,6 and Weyl fermions7,8,9. An intriguing feature of the Weyl equation10 is the chiral symmetry, where the two chiral sectors have an independent gauge freedom. Although this freedom leads to a quantum anomaly11,12,13,14,15, there is no corresponding axial background field coupling differently to opposite chiralities in quantum electrodynamics. Here, we provide the experimental characterization of the effect of such an axial field in an acoustic metamaterial. We implement the axial field through an inhomogeneous potential16 and observe the induced chiral Landau levels. From the metamaterials perspective these chiral channels open the possibility for the observation of non-local Weyl orbits17 and might enable unidirectional bulk transport in a time-reversal-invariant system18.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
Data availability
The data that support the plots within this paper and other findings of this study are available from the corresponding author upon reasonable request.
References
Roy, S., Kolodrubetz, M., Goldman, N. & Grushin, A. G. Tunable axial gauge fields in engineered Weyl semimetals: semiclassical analysis and optical lattice implementations. 2D Mater. 5, 024001 (2018).
Lu, L. et al. Experimental observation of Weyl points. Science 349, 622–624 (2015).
Li, F., Huang, X., Lu, J., Ma, J. & Liu, Z. Weyl points and Fermi arcs in a chiral phononic crystal. Nat. Phys. 14, 30–34 (2017).
Mourik, V. et al. Signatures of Majorana fermions in hybrid superconductor–semiconductor nanowire devices. Science 336, 1003–1007 (2012).
Borisenko, S. et al. Experimental realization of a three-dimensional Dirac semimetal. Phys. Rev. Lett. 113, 027603 (2014).
Liu, Z. K. et al. Discovery of a three-dimensional topological Dirac semimetal, Na3Bi. Science 343, 864–867 (2014).
Weng, H., Fang, C., Fang, Z., Bernevig, B. A. & Dai, X. Weyl semimetal phase in noncentrosymmetric transition-metal monophosphides. Phys. Rev. X 5, 011029 (2015).
Xu, S.-Y. et al. Discovery of a Weyl fermion semimetal and topological Fermi arcs. Science 349, 613–617 (2015).
Lv, B. et al. Experimental discovery of Weyl semimetal TaAs. Phys. Rev. X 5, 031013 (2015).
Weyl, H. Elektron und Gravitation. I. Z. Phys. 56, 330–352 (1929).
Adler, S. L. Axial-vector vertex in spinor electrodynamics. Phys. Rev. 177, 2426–2438 (1969).
Bell, J. S. & Jackiw, R. A PCAC puzzle: π 0 → γγ in the σ-model. Nuovo Cimento 60, 47–61 (1969).
Bertlmann, R. A. Anomalies in Quantum Field Theory (Oxford University Press, Oxford, 2000).
Landsteiner, K. Notes on anomaly induced transport. Acta Phys. Pol. B 47, 2617 (2016).
Gooth, J. et al. Experimental signatures of the mixed axial–gravitational anomaly in the Weyl semimetal NbP. Nature 547, 324–327 (2017).
Pikulin, D. I., Chen, A. & Franz, M. Chiral anomaly from strain-induced gauge fields in Dirac and Weyl semimetals. Phys. Rev. X 6, 041021 (2016).
Potter, A. C., Kimchi, I. & Vishwanath, A. Quantum oscillations from surface Fermi arcs in Weyl and Dirac semimetals. Nat. Commun. 5, 5161 (2014).
Huber, S. D. Topological mechanics. Nat. Phys. 12, 621–623 (2016).
Nielsen, H. & Ninomia, M. The Adler–Bell–Jackiw anomaly and Weyl fermions in a crystal. Phys. Lett. B 130, 389–396 (1983).
Bernevig, B. A. It’s been a Weyl coming. Nat. Phys. 11, 698–699 (2015).
Yang, L. et al. Weyl semimetal phase in the non-centrosymmetric compound TaAs. Nat. Phys. 11, 728–732 (2015).
Moll, P. J. W. et al. Transport evidence for Fermi-arc-mediated chirality transfer in the Dirac semimetal Cd3As2. Nature 535, 266–270 (2016).
v. Klitzing, K., Dorda, G. & Pepper, M. New method for high-accuracy determination of the fine-structure constant based on quantized Hall resistance. Phys. Rev. Lett. 45, 494–497 (1980).
Xiao, M., Chen, W.-J., He, W.-Y. & Chan, C. T. Synthetic gauge flux and Weyl points in acoustic systems. Nat. Phys. 11, 920–924 (2015).
He, H. et al. Topological negative refraction of surface acoustic waves in a Weyl phononic crystal. Nature 560, 61–64 (2018).
Vozmediano, M. A. H., Katsnelson, M. I. & Guinea, F. Gauge fields in graphene. Phys. Rep. 496, 109 (2010).
Liu, C.-X., Ye, P. & Qi, X.-L. Chiral gauge field and axial anomaly in a Weyl semimetal. Phys. Rev. B 87, 235306 (2013).
Cortjio, A., Ferreirós, Y., Landsteiner, K. & Vozmediano, M. A. H. Elastic gauge fields in Weyl semimetals. Phys. Rev. Lett. 115, 177202 (2015).
Grushin, A. G., Venderbros, J. W. F., Vishwanath, A. & Ilan, R. Inhomogeneous Weyl and Dirac semimetals: transport in axial magnetic fields and Fermi arc surface states from pseudo-Landau levels. Phys. Rev. X 6, 041046 (2016).
Sumiyoshi, H. & Fujimoto, S. Torsional chiral magnetic effect in a Weyl semimetal with a topological defect. Phys. Rev. Lett. 116, 166601 (2016).
Abbaszadeh, H., Souslov, A., Paulose, J., Schomerus, H. & Vitelli, V. Sonic Landau-level lasing and synthetic gauge fields in mechanical metamaterials. Phys. Rev. Lett. 119, 195502 (2017).
Levy, N. et al. Strain-induced pseudo–magnetic fields greater than 300 tesla in graphene nanobubbles. Science 329, 544–547 (2010).
Ni, X., Weiner, M., Alù, A. & Khanikaev, A. B. Observation of bulk polarization transitions and higher-order embedded topological eigenstates for sound. Preprint at https://arxiv.org/abs/1807.00896 (2018).
Xu, Y., Wang, S.-T. & Duan, L.-M. Weyl exceptional rings in a three-dimensional dissipative cold atomic gas. Phys. Rev. Lett. 118, 045701 (2017).
Yang, Z., Gao, Z., Yang, Y. & Zhang, B. Strain-induced gauge field and Landau levels in acoustic structures. Phys. Rev. Lett. 118, 194301 (2017).
Wen, X. et al. Observation of acoustic Landau quantization and quantum-Hall-like edge states. Preprint at https://arXiv.org/abs/1807.08454 (2018).
Fukushima, K., Kahrzeev, D. E. & Warringa, H. J. Chiral magnetic effect. Phys. Rev. D 78, 074033 (2008).
Liu, T., Pikulin, D. I. & Franz, M. Quantum oscillations without magnetic field. Phys. Rev. B 95, 041201(R) (2017).
Lee, C.-H. et al. Topolectrical circuits. Commun. Phys. 1, 39 (2018).
Fruchart, M. et al. Soft self-assembly of Weyl materials for light and sound. Proc. Natl Acad. Sci. USA 115, E3655 (2018).
Soluyanov, A. A. et al. Type-II Weyl semimetals. Nature 527, 495–498 (2015).
Acknowledgements
We acknowledge insightful discussions with D. Pikulin and A. Stern. We are grateful for financial support from the Swiss National Science Foundation, the NCCR QSIT. This work has received funding from the European Research Council under grant agreement no. 771503.
Author information
Authors and Affiliations
Contributions
S.D.H., R.I. and V.P. performed the theoretical part of this work. M.S.-G. and V.P. conducted the experiments. All authors contributed to the writing of the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary Text, Supplementary Figures 1–8 and Supplementary References.
Supplementary Video 1
Video showing the frequency response in momentum space as a function of excitation frequency.
Rights and permissions
About this article
Cite this article
Peri, V., Serra-Garcia, M., Ilan, R. et al. Axial-field-induced chiral channels in an acoustic Weyl system. Nat. Phys. 15, 357–361 (2019). https://doi.org/10.1038/s41567-019-0415-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-019-0415-x
This article is cited by
-
Observation of continuum Landau modes in non-Hermitian electric circuits
Nature Communications (2024)
-
Direct observation of Landau levels in silicon photonic crystals
Nature Photonics (2024)
-
Three-dimensional flat Landau levels in an inhomogeneous acoustic crystal
Nature Communications (2024)
-
Observation of Landau levels and chiral edge states in photonic crystals through pseudomagnetic fields induced by synthetic strain
Nature Photonics (2024)
-
Surface potential-adjusted surface states in 3D topological photonic crystals
Scientific Reports (2024)