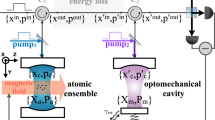
Abstract
Deterministic quantum interactions between single photons and single quantum emitters are a vital building block towards the distribution of quantum information between remote systems1,2,3,4. Deterministic photon–atom state transfer has previously been demonstrated with protocols that include active feedback or synchronized control pulses5,6,7,8,9,10. Here we demonstrate a passive swap operation between the states of a single photon and a single atom. The underlying mechanism is single-photon Raman interaction11,12,13,14,15—an interference-based scheme that leads to deterministic interaction between two photonic modes and the two ground states of a Λ-system. Using a nanofibre-coupled microsphere resonator coupled to single Rb atoms, we swap a photonic qubit into the atom and back, demonstrating fidelities exceeding the classical threshold of 2/3 in both directions. In this simultaneous write and read process, the returning photon, which carries the readout of the atomic qubit, also heralds the successful arrival of the write photon. Requiring no control fields, this single-step gate takes place automatically at the timescale of the atom’s cavity-enhanced spontaneous emission. Applicable to any waveguide-coupled Λ-system, this mechanism, which can also be harnessed to construct universal gates16,17, provides a versatile building block for the modular scaling up of quantum information systems.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Cirac, J. I., Zoller, P., Kimble, H. J. & Mabuchi, H. Quantum state transfer and entanglement distribution among distant nodes in a quantum network. Phys. Rev. Lett. 78, 3221–3224 (1997).
Monroe, C. Quantum information processing with atoms and photons. Nature 416, 238–246 (2002).
Kimble, H. J. The quantum internet. Nature 453, 1023–1030 (2008).
Monroe, C. et al. Large-scale modular quantum-computer architecture with atomic memory and photonic interconnects. Phys. Rev. A 89, 022317 (2014).
Duan, L.-M. & Kimble, H. J. Scalable photonic quantum computation through cavity-assisted interactions. Phys. Rev. Lett. 92, 127902 (2004).
Boozer, A. D., Boca, A., Miller, R., Northup, T. E. & Kimble, H. J. Reversible state transfer between light and a single trapped atom. Phys. Rev. Lett. 98, 193601 (2007).
Specht, H. P. et al. A single-atom quantum memory. Nature 473, 190–193 (2011).
Reiserer, A., Kalb, N., Rempe, G. & Ritter, S. A quantum gate between a flying optical photon and a single trapped atom. Nature 508, 237–240 (2014).
Tiecke, T. G. et al. Nanophotonic quantum phase switch with a single atom. Nature 508, 241–244 (2014).
Kalb, N., Reiserer, A., Ritter, S. & Rempe, G. Heralded storage of a photonic quantum bit in a single atom. Phys. Rev. Lett. 114, 220501 (2015).
Shomroni, I. et al. All-optical routing of single photons by a one-atom switch controlled by a single photon. Science 345, 903–906 (2014).
Inomata, K. et al. Microwave down-conversion with an impedance-matched Λ system in driven circuit QED. Phys. Rev. Lett. 113, 63604 (2014).
Rosenblum, S. et al. Extraction of a single photon from an optical pulse. Nat. Photon. 10, 19–22 (2016).
Inomata, K. et al. Single microwave-photon detector using an artificial Λ-type three-level system. Nat. Commun. 7, 12303 (2016).
Rosenblum, S., Borne, A. & Dayan, B. Analysis of deterministic swapping of photonic and atomic states through single-photon Raman interaction. Phys. Rev. A 95, 33814 (2017).
Koshino, K., Ishizaka, S. & Nakamura, Y. Deterministic photon-photon √SWAP gate using a Λ system. Phys. Rev. A 82, 10301 (2010).
Koshino, K. et al. Tunable quantum gate between a superconducting atom and a propagating microwave photon. Phys. Rev. Appl. 7, 064006 (2016).
Bouwmeester, D. et al. Experimental quantum teleportation. Nature 390, 575–579 (1997).
Gao, W. et al. Quantum teleportation from a propagating photon to a solid-state spin qubit. Nat. Commun. 4, 2744 (2013).
Takeda, S., Mizuta, T., Fuwa, M., van Loock, P. & Furusawa, A. Deterministic quantum teleportation of photonic quantum bits by a hybrid technique. Nature 500, 315–318 (2013).
Haroche, S. & Raimond, J.-M. Exploring the Quantum: Atoms, Cavities, and Photons (Oxford Univ. Press, Oxford, 2006).
Thompson, R. J., Rempe, G. & Kimble, H. J. Observation of normal-mode splitting for an atom in an optical cavity. Phys. Rev. Lett. 68, 1132–1135 (1992).
Nickerson, N. H., Li, Y. & Benjamin, S. C. Topological quantum computing with a very noisy network and local error rates approaching one percent. Nat. Commun. 4, 1756 (2013).
Hacker, B., Welte, S., Rempe, G. & Ritter, S. A photon–photon quantum gate based on a single atom in an optical resonator. Nature 536, 193–196 (2016).
Pinotsi, D. & Imamoglu, A. Single photon absorption by a single quantum emitter. Phys. Rev. Lett. 100, 93603 (2008).
Lin, G. W., Zou, X. B., Lin, X. M. & Guo, G. C. Heralded quantum memory for single-photon polarization qubits. EPL 86, 30006 (2009).
Bradford, M. & Shen, J.-T. Single-photon frequency conversion by exploiting quantum interference. Phys. Rev. A 85, 43814 (2012).
Gea-Banacloche, J. & Wilson, W. Photon subtraction and addition by a three-level atom in an optical cavity. Phys. Rev. A 88, 33832 (2013).
Junge, C., O’Shea, D., Volz, J. & Rauschenbeutel, A. Strong coupling between single atoms and nontransversal photons. Phys. Rev. Lett. 110, 213604 (2013).
Lodahl, P. et al. Chiral quantum optics. Nature 541, 473–480 (2017).
Aiello, A., Banzer, P., Neugebauer, M. & Leuchs, G. From transverse angular momentum to photonic wheels. Nat. Photon. 9, 789–795 (2015).
Massar, S. & Popescu, S. Optimal extraction of information from finite quantum ensembles. Phys. Rev. Lett. 74, 1259–1263 (1995).
Tokunaga, Y. & Koshino, K. A photon–photon controlled-phase gate using a Λ system. in European Quantum Electronics Conf. Proc. EB_P_7 (Optical Society of America, 2015).
Acknowledgements
Support from the Israeli Science Foundation, the Minerva Foundation and the Crown Photonics Center is acknowledged. This research was made possible in part by the historic generosity of the Harold Perlman family.
Author information
Authors and Affiliations
Contributions
All authors contributed to the carrying out of the experiment, discussed the results and commented on the manuscript. O.B. and A.B. analysed the data. O.B., A.B., S.R. and B.D. contributed to the design and construction of the experimental set-up. O.B., A.B., S.R. and B.D. wrote the manuscript. O.B., A.B. and S.R. contributed equally to this work.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary figures 1 and 2
Rights and permissions
About this article
Cite this article
Bechler, O., Borne, A., Rosenblum, S. et al. A passive photon–atom qubit swap operation. Nature Phys 14, 996–1000 (2018). https://doi.org/10.1038/s41567-018-0241-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-018-0241-6
This article is cited by
-
Giant optical polarisation rotations induced by a single quantum dot spin
Nature Communications (2024)
-
On-chip spin-photon entanglement based on photon-scattering of a quantum dot
npj Quantum Information (2023)
-
A quantum network node with crossed optical fibre cavities
Nature Physics (2020)
-
A unidirectional on-chip photonic interface for superconducting circuits
npj Quantum Information (2020)
-
Controlling free electrons with optical whispering-gallery modes
Nature (2020)