Abstract
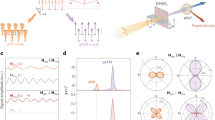
Recent advances in the emerging field of magnon spintronics have stimulated renewed interest in phenomena involving the interaction between spin waves, the collective excitations of spins in magnetic materials that quantize as magnons, and the elastic waves that arise from excitations in the crystal lattice, which quantize as phonons. In magnetic insulators, owing to the magnetostrictive properties of materials, spin waves can become strongly coupled to elastic waves, forming magnetoelastic waves—a hybridized magnon–phonon excitation. While several aspects of this interaction have been subject to recent scrutiny, it remains unclear whether or not phonons can carry spin. Here we report experiments on a film of the ferrimagnetic insulator yttrium iron garnet under a non-uniform magnetic field demonstrating the conversion of coherent magnons generated by a microwave field into phonons that have spin. While it is well established that photons in circularly polarized light carry a spin, the spin of phonons has had little attention in the literature. By means of wavevector-resolved Brillouin light-scattering measurements, we show that the magnon–phonon conversion occurs with constant energy and varying linear momentum, and that the light scattered by the phonons is circularly polarized, thus demonstrating that the phonons have spin.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout





Similar content being viewed by others
References
Chumak, A. V., Vasyuchka, V. I., Serga, A. A. & Hillebrands, B. Magnon spintronics. Nat. Phys. 11, 453–461 (2015).
Serga, A. A., Chumak, A. V. & Hillebrands, B. YIG magnonics. J. Phys. D 43, 264002 (2010).
Uchida, K. et al. Long-range spin Seebeck effect and acoustic spin pumping. Nat. Mater. 10, 737–741 (2011).
Weiler, M. et al. Spin pumping with coherent elastic waves. Phys. Rev. Lett. 108, 176601 (2012).
Kamra, A., Keshtgar, H., Yan, P. & Bauer, G. E. W. Coherent elastic excitation of spin waves. Phys. Rev. B 91, 104409 (2015).
Li, X., Labanowski, D., Salahuddin, S. & Lynch, C. S. Spin wave generation by surface acoustic waves. J. Appl. Phys. 122, 043904 (2017).
Kikkawa, T. et al. Magnon polarons in the spin Seebeck effect. Phys. Rev. Lett. 117, 207203 (2016).
Man, H. et al. Direct observation of magnon–phonon coupling in yttrium iron garnet. Phys. Rev. B 96, 100406(R) (2017).
Flebus, B. et al. Magnon–polaron transport in magnetic insulators. Phys. Rev. B 95, 144420 (2017).
Cornelissen, L. J. et al. Nonlocal magnon–polaron transport in yttrium iron garnet. Phys. Rev. B 96, 104441 (2017).
Bozhko, D. A. et al. Bottleneck accumulation of hybrid magnetoelastic bosons. Phys. Rev. Lett. 118, 237201 (2017).
Kamra, A. & Bauer, G. E. W. Actuation, propagation, and detection of transverse magnetoelastic waves in ferromagnets. Solid State Commun. 198, 35–39 (2014).
Ogawa, N. et al. Photodrive of magnetic bubbles via magnetoelastic waves. Proc. Natl Acad. Sci. USA 112, 8977–8981 (2015).
An, K. et al. Magnons and phonons optically driven out of local equilibrium in a magnetic insulator. Phys. Rev. Lett. 117, 107202 (2016).
Kabos, P. & Stalmachov, V. S. Magnetostatic Waves and Their Applications (Chapman and Hall, London, 1994).
Rezende, S. M. & Zagury, N. Coherent magnon states. Phys. Lett 29A, 47–48 (1969).
Zagury, N. & Rezende, S. M. Theory of macroscopic excitations of magnons. Phys. Rev. B 4, 201–209 (1971).
Damon, R. W. & Eshbach, J. R. Magnetostatic modes of a ferromagnet slab. J. Phys. Chem. Solids 19, 308–320 (1961).
Stancil, D. D. & Prabhakar, A. Spin Waves: Theory and Applications (Springer, New York, 2009).
Rezende, S. M. Theory of coherence in Bose–Einstein condensation phenomena in a microwave driven interacting magnon gas. Phys. Rev. B 79, 174411 (2009).
Kittel, C. Interaction of spin waves and ultrasonic waves in ferromagnetic crystals. Phys. Rev. 110, 836–841 (1958).
Akhiezer, A. I., Bar’yakhtar, V. G. & Peletminskii, S. V. Spin Waves (North-Holland, Amsterdam, 1968).
Gurevich, A. G. & Melkov, G. A. Magnetization Oscillations and Waves (CRC, Boca Raton, 1994).
Schlömann, E. & Joseph, R. I. Generation of spin waves in nonuniform magnetic fields. III. Magnetoelastic interaction. J. Appl. Phys. 35, 2382–2390 (1964).
Rezende, S. M. & Morgenthaler, F. R. Magnetoelastic waves in time‐varying magnetic fields. I. Theory, II. Experiments. J. Appl. Phys. 40, 524–545 (1969).
Guerreiro, S. C. & Rezende, S. M. Magnon–phonon interconversion in a dynamically reconfigurable magnetic material. Phys. Rev. B 92, 214437 (2015).
Rückriegel, A., Kopietz, P., Bozhko, D. A., Serga, A. A. & Hillebrands, B. Magnetoelastic modes and lifetime of magnons in thin yttrium iron garnet films. Phys. Rev. B 89, 184413 (2014).
Kane, E. O. Theory of tunneling. J. Appl. Phys. 32, 83–91 (1961).
Eshbach, J. R. Spin-wave propagation and the magnetoelastic interaction in yttrium iron garnet. Phys. Rev. Lett. 8, 357–359 (1962).
Strauss, W. Magnetoelastic waves in yttrium iron garnet. J. Appl. Phys. 36, 118–123 (1965).
Auld, B. A., Collins, J. H. & Webb, D. C. Excitation of magnetoelastic waves in YIG delay lines. J. Appl. Phys. 39, 1598–1602 (1968).
Smith, K. R., Kabatek, M. J., Krivosik, P. & Wu, M. Spin wave propagation in spatially nonuniform magnetic fields. J. Appl. Phys. 104, 043911 (2008).
Demokritov, S. O. et al. Bose–Einstein condensation of quasi-equilibrium magnons at room temperature under pumping. Nature 443, 430–433 (2006).
Sandweg, C. W. et al. Wide-range wavevector selectivity of magnon gases in Brillouin light scattering spectroscopy. Rev. Sci. Instrum. 81, 073902 (2010).
Heitler, W. The Quantum Theory of Radiation (Oxford Univ. Press, New York, 1944).
Leach, J. et al. Interferometric methods to measure orbital and spin, or the total angular momentum of a single photon. Phys. Rev. Lett. 92, 013601 (2002).
Yao, A. M. & Padgett, M. J. Optical angular momentum: origins, behavior, and applications. Adv. Opt. Photon. 3, 161–204 (2011).
Zhang, L. & Niu, Q. Angular momentum of phonons and the Einstein–de Haas effect. Phys. Rev. Lett. 112, 085503 (2014).
Garanin, D. A. & Chudnovsky, E. M. Angular momentum in spin-phonon processes. Phys. Rev. B 92, 024421 (2015).
Cottam, M. G. & Lockwood, D. J. Light Scattering in Magnetic Solids (Wiley, New York, 1986).
White R. M., Quantum Theory of Magnetism, 3rd edn (Springer-Verlag, Berlin, 2007).
Jaworski, C. M. et al. Spin-Seebeck effect: a phonon driven spin distribution. Phys. Rev. Lett. 106, 186601 (2011).
Acknowledgements
This research was supported by Conselho Nacional de Desenvolvimento Científico e Tecnológico (CNPq), Coordenação de Aperfeiçoamento de Pessoal de Nível Superior (CAPES), Financiadora de Estudos e Projetos (FINEP) and Fundação de Amparo à Ciência e Tecnologia do Estado de Pernambuco (FACEPE).
Author information
Authors and Affiliations
Contributions
All experimental arrangements and measurements were performed by J.H. and D.S.M. with consultation from A.A. and S.M.R. A.A. prepared the YIG films using liquid phase epitaxy. Calculations were performed by S.M.R. and J.H. Supervision of the work was carried out by S.M.R., who wrote the manuscript with contributions from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Supplementary Information
Supplementary notes, Supplementary figures 1–5
Rights and permissions
About this article
Cite this article
Holanda, J., Maior, D.S., Azevedo, A. et al. Detecting the phonon spin in magnon–phonon conversion experiments. Nature Phys 14, 500–506 (2018). https://doi.org/10.1038/s41567-018-0079-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41567-018-0079-y
This article is cited by
-
Quantifying Entanglement by Purity in a Cavity-Magnon System
Brazilian Journal of Physics (2024)
-
Non-equilibrium dynamics of spin-lattice coupling
Nature Communications (2023)
-
Direct observation of topological magnon polarons in a multiferroic material
Nature Communications (2023)
-
Unraveling the spin current hermiticity
Journal of Materials Science (2023)
-
Analyzing the Magnetic Influence on Magneto-optical Interactions
Journal of Superconductivity and Novel Magnetism (2023)