Abstract
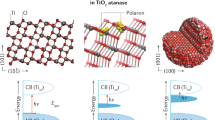
The concepts of oxidation state and atomic charge are entangled in modern materials science. We distinguish between these quantities and consider their fundamental limitations and utility for understanding material properties. We discuss the nature of bonding between atoms and the techniques that have been developed for partitioning electron density. While formal oxidation states help us count electrons (in ions, bonds, lone pairs), variously defined atomic charges are usefully employed in the description of physical processes including dielectric response and electronic spectroscopies. Such partial charges are introduced as quantitative measures in simple mechanistic models of a more complex reality, and therefore may not be comparable or transferable. In contrast, oxidation states are defined to be universal, with deviations constituting exciting challenges as evidenced in mixed-valence compounds, electrides and highly correlated systems. This Perspective covers how these concepts have evolved in recent years, our current understanding and their significance.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$259.00 per year
only $21.58 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout


Similar content being viewed by others
References
Karen, P. Oxidation state, a long-standing issue! Angew. Chem. Int. Ed. 54, 4716–4726 (2015).
IUPAC Compendium of Chemical Terminology 2nd edn (eds McNaught, A. D. & Wilkinson, A.) (Blackwell Scientific, Oxford, 1997); https://doi.org/10.1351/goldbook.O04365
Goodman, C. H. L. Ionic-covalent bonding in crystals. Nature 187, 590–591 (1960).
MooserE.. & PearsonW. B.. The ionic character of chemical bonds. Nature 406–408, 1961 (1920).
Cochran, W. ‘Effective’ ionic charge in crystals. Nature 191, 60–61 (1961).
Catlow, C. R. A. & Stoneham, A. M. Ionicity in solids. J. Phys. C Solid State 16, 4321–4338 (1983).
Raebiger, H., Lany, S. & Zunger, A. Charge self-regulation upon changing the oxidation state of transition metals in insulators. Nature 453, 763–766 (2008).
Jansen, M. & Wedig, U. A piece of the picture - misunderstanding of chemical concepts. Angew. Chemie Int. Ed. 47, 10026–10029 (2008).
Koch, D. & Manzhos, S. On the charge state of titanium in titanium dioxide. J. Phys. Chem. Lett. 8, 1593–1598 (2017).
Walsh, A., Sokol, A. A., Buckeridge, J., Scanlon, D. O. & Catlow, R. A. Electron counting in solids: oxidation states, partial charges, and ionicity. J. Phys. Chem. Lett. 8, 2074–2075 (2017).
Pauling, L. The modern theory of valency. J. Chem. Soc. 0, 1461–1467 (1948).
Massidda, S., Yu, J., Freeman, A. J. & Koelling, D. D. Electronic structure and properties of YBa2Cu3O7-δ, a low dimensional, low density of states superconductor. Phys. Lett. A 122, 198–202 (1987).
Kageyama, H. et al. Expanding frontiers in materials chemistry and physics with multiple anions. Nat. Commun. 9, 772 (2018).
Zhang, J. et al. Designing high-performance layered thermoelectric materials through orbital engineering. Nat. Commun. 7, 10892 (2016).
Zeier, W. G. et al. Engineering half-heusler thermoelectric materials using Zintl chemistry. Nat. Rev. Mater. 1, 16032 (2016).
Gillespie, R. J. The valence-shell electron-pair repulsion (VSEPR) theory of directed valency. J. Chem. Educ. 40, 295–301 (1963).
Griffith, J. & Orgel, L. Ligand-field theory. Q. Rev. 11, 381–393 (1957).
Cockayne, E., Levin, I., Wu, H. & Llobet, A. Magnetic structure of bixbyite α-Mn2O3: a combined DFT+U and neutron diffraction study. Phys. Rev. B 87, 184413 (2013).
Shen, X.-F. et al. A magnetic route to measure the average oxidation state of mixed-valent manganese in manganese oxide octahedral molecular sieves (OMS). J. Am. Chem. Soc. 127, 6166–6167 (2005).
Dirac, P. A. M. Quantum mechanics of many-electron systems. Proc. R. Soc. London A Math. Phys. Eng. Sci. 123, 714–733 (1929).
Szabo, A. & Ostlund, N. S. Modern Quantum Chemistry: Introduction to Advanced Electronic Structure Theory (Dover, Mineola, 1996).
Mcweeny, R. The density matrix in many-electron quantum mechanics. I. Generalized product functions. factorization and physical interpretation of the density matrices. Proc. R. Soc. Lond. A. Math. Phys. Sci. 253, 242–259 (1959).
Kantorovich, L. N. & Zapol, B. P. A diagram technique for nonorthogonal electron group functions. I. Right coset decomposition of symmetric group. J. Chem. Phys. 96, 8420–8426 (1992).
Bader, R. F. W. & Nguyen-Dang, T. T. Quantum theory of atoms in molecules—Dalton revisited. Adv. Quantum Chem. 14, 63–124 (1981).
Kohn, W. Analytic properties of Bloch waves and Wannier functions. Phys. Rev. 115, 809–821 (1959).
Marzari, N. & Vanderbilt, D. Maximally localized generalized Wannier functions for composite energy bands. Phys. Rev. B 56, 12847–12865 (1997).
Mulliken, R. S. Electronic population analysis on LCAO–MO molecular wave functions. I. J. Chem. Phys. 23, 1833–1840 (1955).
Christoffersen, R. E. & Baker, K. A. Electron population analysis. gross atomic charges in molecules. Chem. Phys. Lett. 8, 4–9 (1971).
Hirshfeld, F. L. Bonded-atom fragments for describing molecular charge densities. Theor. Chim. Acta 44, 129–138 (1977).
Becke, A. D. & Edgecombe, K. E. A simple measure of electron localization in atomic and molecular systems. J. Chem. Phys. 92, 5397–5403 (1990).
Savin, A. et al. Electron localization in solid‐state structures of the elements: the diamond structure. Angew. Chem. Int. Ed. English 31, 187–188 (1992).
Dick, B. G. & Overhauser, A. W. Theory of the dielectric constants of alkali halide crystals. Phys. Rev. 112, 90–103 (1958).
Spaldin, N. A. A beginner’s guide to the modern theory of polarization. J. Solid State Chem. 195, 2–10 (2012).
King-Smith, R. D. & Vanderbilt, D. Theory of polarization of crystalline solids. Phys. Rev. B 47, 1651–1654 (1993).
Jiang, L., Levchenko, S. V. & Rappe, A. M. Rigorous definition of oxidation states of ions in solids. Phys. Rev. Lett. 108, 166403 (2012).
Day, P., Hush, N. S. & Clark, R. J. H. Mixed valence: origins and developments. Philos. Trans. A. Math. Phys. Eng. Sci. 366, 5–14 (2008).
Robin, M. B. & Day, P. Mixed valence chemistry—a survey and classification. Adv. Inorg. Chem. Radiochem. 10, 247–422 (1968).
Allen, J. P., Scanlon, D. O. & Watson, G. W. Electronic structure of mixed-valence silver oxide AgO from hybrid density-functional theory. Phys. Rev. B 81, 161103 (2010).
Conejeros, S., Moreira, I., de, P. R., Alemany, P. & Canadell, E. Nature of holes, oxidation states, and hypervalency in covellite (CuS). Inorg. Chem. 53, 12402–12406 (2014).
Senn, M. S., Wright, J. P. & Attfield, J. P. Charge order and three-site distortions in the Verwey structure of magnetite. Nature 481, 173–176 (2012).
Oganov, A. R. et al. Ionic high-pressure form of elemental boron. Nature 457, 863–867 (2009).
Albers, R. C. Condensed-matter physics: an expanding view of plutonium. Nature 410, 759–761 (2001).
Janoschek, M. et al. The valence-fluctuating ground state of plutonium. Sci. Adv. 1, e1500188 (2015).
Watanabe, S. & Miyake, K. Quantum valence criticality as an origin of unconventional critical phenomena. Phys. Rev. Lett. 105, 186403 (2010).
Watanabe, S. & Miyake, K. Roles of critical valence fluctuations in Ce- and Yb-based heavy fermion metals. J. Phys. Condens. Matter 23, 094217 (2011).
Yamaoka, H. et al. Role of valence fluctuations in the superconductivity of Ce122 compounds. Phys. Rev. Lett. 113, 086403 (2014).
Putzke, C. et al. Anomalous critical fields in quantum critical superconductors. Nat. Commun. 5, 5679 (2014).
Mondal, S. et al. Experimental evidence of orbital order in α-B12 and γ-B28 polymorphs of elemental boron. Phys. Rev. B 88, 024118 (2013).
Nilsson Pingel, T., Jørgensen, M., Yankovich, A. B., Grönbeck, H. & Olsson, E. Influence of atomic site-specific strain on catalytic activity of supported nanoparticles. Nat. Commun. 9, 2722 (2018).
Anasori, B., Lukatskaya, M. R. & Gogotsi, Y. 2D metal carbides and nitrides (MXenes) for energy storage. Nat. Rev. Mater. 2, 16098 (2017).
Jiang, B., Zuo, J. M., Jiang, N., O’Keeffe, M. & Spence, J. C. H. Charge density and chemical bonding in rutile, TiO2. Acta Crystallogr. A 59, 341–350 (2003).
Burdett, J. K. Structural–electronic relationships in rutile. Acta Crystallogr. Sect. B 51, 547–558 (1995).
Scanlon, D. O. et al. Band alignment of rutile and anatase TiO2. Nat. Mater. 12, 798–801 (2013).
Morgan, B. J. & Watson, G. W. A density functional theory + U study of oxygen vacancy formation at the (110), (100), (101), and (001) surfaces of rutile TiO2. J. Phys. Chem. C 113, 7322–7328 (2009).
Weller, M., Overton, T., Rourke, J. & Armstrong, F. Inorganic Chemistry 6th edn (Oxford Univ. Press, Oxford, 2014).
Brown, I. D. The Chemical Bond in Inorganic Chemistry: The Bond Valence Model (Oxford Univ. Press, Oxford, 2002).
Kurtz, R. L. & Henrich, V. E. Comparison of Ti 2p core-level peaks from TiO2, Ti2O3, and Ti metal, by XPS. Surf. Sci. Spectra 5, 179–181 (1998).
Acknowledgements
C.R.A.C. is grateful for many discussions with A. M. Stoneham on the topic in this Perspective. A.W. thanks R. G. Egdell and A. Regoutz for discussions on X-ray photomemission. A.A.S. is indebted to L. N. Kantorovich for discussions of the electron groups theory and structural elements. The research was supported by the EPSRC (grant nos EP/K016288/1 and EP/N01572X/1), the Leverhulme Trust, and the Royal Society. D.O.S. acknowledges support from the European Research Council (grant no. 758345). This work was carried out with funding from the Faraday Institution (https://faraday.ac.uk; EP/S003053/1), grant no. FIRG003.
Author information
Authors and Affiliations
Contributions
All authors contributed equally to the design, writing and editing of the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Walsh, A., Sokol, A.A., Buckeridge, J. et al. Oxidation states and ionicity. Nature Mater 17, 958–964 (2018). https://doi.org/10.1038/s41563-018-0165-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/s41563-018-0165-7
This article is cited by
-
CHGNet as a pretrained universal neural network potential for charge-informed atomistic modelling
Nature Machine Intelligence (2023)
-
Water Splitting on a Pt1/C3N4 Single Atom Catalyst: A Modeling Approach
Topics in Catalysis (2023)
-
Electronic structure and optical properties of Br- and Cl-doped rutile TiO2 for application in self-cleaning and photovoltaic panel’s coatings: first-principle calculations
Environmental Science and Pollution Research (2023)
-
Data-driven models for ground and excited states for Single Atoms on Ceria
npj Computational Materials (2022)
-
A single-atom library for guided monometallic and concentration-complex multimetallic designs
Nature Materials (2022)