Introduction

Quantum computers have the potential of simulating physics beyond the capacity of classical computers1,2,3,4. Gate-defined quantum dots are extensively studied for quantum computation5,6, but are also a natural platform for implementing quantum simulations7,8,9,10,11. The control over the electrical charge degree of freedom has facilitated the exploration of novel configurations such as effective attractive electron–electron interactions12, collective Coulomb blockade13 and topological states14. Coherent systems may be simulated when using the spin states of electrons in quantum dots, though experiments thus far have relied on gallium arsenide heterostructures15,16,17, where the hyperfine interaction limits the spin coherence and therefore the complexity of simulations that can be performed. This bottleneck can be tackled by using group IV materials with nuclear spin-free isotopes. A natural candidate would be silicon, but this material comes with additional challenges due to the presence of valley states and a large effective electron mass18.

Hole quantum dots in planar Ge/SiGe heterostructures exhibit many favorable properties found in different quantum dot platforms19. Natural germanium has a high abundance of nuclear spin-free isotopes and can be isotopically purified20. Holes in germanium benefit from a low effective mass21,22, absence of valley degeneracies, ohmic contacts to metals23, and strong spin-orbit coupling for all-electrical control24,25. Recent advances in heterostructure growth have resulted in stable, low-noise germanium devices26. This has sparked rapid progress, with demonstrations of hole quantum dots23, single hole qubits25, singlet-triplet (ST) qubits27, two-qubit logic28 and a four qubit quantum processor29.

Here, we explore the prospects of hole quantum dots in Ge/SiGe for quantum simulation. We focus on the simulation of resonating valence bond (RVB) states, which are of fundamental relevance in chemistry30 and solid state physics31,32,33,34 and have been used in other platforms as a feasibility test for quantum simulation35,36,37,38. In our simulation, we probe RVB states in a square 2 × 2 configuration. First, we realize ST qubits for all nearest-neighbor configurations. We then study the coherent evolution of four-spin states and demonstrate exchange control spanning an order of magnitude. Furthermore, we tune the system to probe valence bond resonances whose observed characteristics comply with predictions derived from the Heisenberg model. We finally demonstrate the preparation of s-wave and d-wave RVB states from spin-singlet states via adiabatic initialization and tailored pulse sequences.

Results

RVB simulation in a quantum dot array with a square geometry

The experiments are based on a quantum dot array defined in a high-quality Ge/SiGe quantum well, as shown in Fig. 1a29,39. The array comprises four quantum dots and we obtain good control over the system, enabling to confine zero, one, or two holes in each quantum dot as required for the quantum simulation. The dynamics of resonating valence bonds is governed by Heisenberg interactions. The spin states in germanium quantum dots, however, also experience Zeeman, spin–orbit and hyperfine interactions (see Supplementary Note 6). We therefore operate in small magnetic fields and acquire a detailed understanding of the system dynamics to apply tailored pulses. In the regime where Heisenberg interactions are dominating, the total spin is conserved. We can therefore study the subspaces of different total spin separately. The relevant subspace for the RVB physics is the zero total spin space spanned by the basis formed by the four-spin states \(\vert {S}_{x}\rangle =\vert {S}_{12}{S}_{34}\rangle\) and \(\frac{1}{\sqrt{3}}(\vert {T}_{12}^{+}{T}_{34}^{-}\rangle +\vert {T}_{12}^{-}{T}_{34}^{+}\rangle -\vert {T}_{12}^{0}{T}_{34}^{0}\rangle )\), where \(\vert {S}_{ij}\rangle =\frac{1}{\sqrt{2}}(\vert {\uparrow }_{i}{\downarrow }_{j}\rangle -\vert {\downarrow }_{i}{\uparrow }_{j}\rangle )\) and \(\vert {T}_{ij}^{0}\rangle =\frac{1}{\sqrt{2}}(\vert {\uparrow }_{i}{\downarrow }_{{{{\rm{j}}}}}\rangle +\vert {\downarrow }_{i}{\uparrow }_{j}\rangle )\), \(\vert {T}_{ij}^{+}\rangle =\vert {\uparrow }_{i}{\uparrow }_{j}\rangle\), \(\vert {T}_{ij}^{-}\rangle =\vert {\downarrow }_{i}{\downarrow }_{j}\rangle\) denote the singlet and triplet states formed by the spins in the quantum dots i and j. In this basis, the Heisenberg Hamiltonian HJ reads:

$${H}_{J}({S}_{{{\mbox{tot}}}}=0)\equiv {H}_{S}=\left(\begin{array}{cc}-{J}_{x}-\frac{{J}_{y}}{4}&\frac{\sqrt{3}}{4}{J}_{y}\\ \frac{\sqrt{3}}{4}{J}_{y}&-\frac{3}{4}{J}_{y}\end{array}\right),$$
(1)

where Jx = J12 + J34 and Jy = J14 + J23. Figure 1b, c shows the eigen energies and eigenstates of HS for different regimes of exchange interaction. When the exchange interaction is turned on in only one direction, Jx ≫ Jy or Jx ≪ Jy, the system is equivalent to two uncoupled double quantum dots. The ground state is then a product of singlet states \(\left\vert {S}_{x}\right\rangle\) or \(\left\vert {S}_{y}\right\rangle =\left\vert {S}_{14}{S}_{23}\right\rangle\). However, when all exchanges are on and in particular when they are equal, Jx = Jy, the eigenstates are coherent superpositions of \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\), which simulate the RVB state. In this regime, the ground state is the s-wave superposition state \(\left\vert s\right\rangle =\frac{1}{\sqrt{3}}(\left\vert {S}_{x}\right\rangle -\left\vert {S}_{y}\right\rangle )\) and the excited state is the d-wave superposition state \(\left\vert d\right\rangle =\left\vert {S}_{x}\right\rangle +\left\vert {S}_{y}\right\rangle\).

Fig. 1: RVB states in a 2 × 2 quantum dot array.
figure 1

a False-colored scanning electron micrograph of the Ge quantum dot array. Plunger and barrier gates are colored in blue and green respectively, and the corresponding gate voltages applied on them are labeled. To achieve independent control of the quantum dot potentials and tunnel couplings, virtual plunger and barrier gate voltages are defined (see Supplementary Note 1). Single hole transistors used as charge sensors are colored in yellow. The scale bar corresponds to 100 nm. b Energy diagram corresponding to the Hamiltonian HS. The stars denote the corresponding eigenstates depicted in (c). When the exchange interaction is dominated by horizontal (vertical) pairs, the ground state is \(\left\vert {S}_{x}\right\rangle\) (\(\left\vert {S}_{y}\right\rangle\)), and in our experiments we use this configuration for initialization. Resonating valence bond states appear when Jy = Jx, the eigenstates are the ground state with s-wave symmetry and excited state with d-wave symmetry.

Full size image

Figure 1b shows that RVB states can be generated from uncoupled spin singlets by adiabatically equalizing the exchange couplings. Alternatively, if the exchange couplings are pulsed diabatically to equal values, valence bond resonances between \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\) states occur.

Singlet-Triplet oscillations in the four double quantum dots

Probing the RVB physics relies on measuring the singlet probabilities in the (1,1) charge state17,36. We thus investigate ST oscillations within all nearest-neighbor pairs.

To generate ST oscillations, we operate in a virtual gate landscape and apply pulses on the virtual plunger gates vPi of each quantum dot pair according to the pulse sequence depicted in Fig. 2a 27,40,41,42,43. The double quantum dot system is initialized in a singlet (0,2) state. Then, the detuning between the quantum dots is varied by changing the virtual plunger gate voltages. The system is diabatically brought to a manipulation point in the (1,1) sector creating a coherent superposition of \(\left\vert S\right\rangle\), \(\left\vert {T}^{-}\right\rangle\) and \(\left\vert {T}^{0}\right\rangle\)27,40,41,42,43. After a dwell time tD, the system is diabatically pulsed back to the (0,2) sector where the ST probabilities are determined via single-shot readout using (latched) Pauli-spin-blockade44,45,46.

Fig. 2: Singlet-triplet qubits on all nearest-neighbor configurations.
figure 2

a Schematics of the pulse sequence used to generate singlet-triplet oscillations in double quantum dots. b Charge stability diagram of a double quantum dot (Q3Q4) in the few-hole regime. c S-T− oscillations as a function of time and detuning δvP34 = 0.5(vP3 − vP4) varied along the dashed line in (b). At larger magnetic fields, here B = 3 mT, and limited tunnel couplings, we observe a minimum oscillation frequency due to the S-T− anticrossing. We tune the system in a regime with smaller magnetic fields (B = 1 mT) and larger tunnel couplings, to operate away from this point. d S-T− oscillations observed in this regime for all possible permutations of nearest-neighbor quantum dot pairs. Black lines are fits of the data (see Supplementary Note 2).

Full size image

Results of such experiments performed at B = 3 mT with Q3Q4 pair are presented in Fig. 2c. Clear oscillations between the \(\left\vert S\right\rangle\) and \(\left\vert {T}^{-}\right\rangle\) state are observed over a large range of gate voltage. Importantly, using this method we find the S-T− anticrossing, which is the position where the frequency has a minimum. The observation of such oscillations, predominating over oscillations between \(\left\vert S\right\rangle\) and \(\left\vert {T}^{0}\right\rangle\) states, agrees with recent investigations suggesting that S-T− oscillations dominate in germanium ST qubits placed in an in-plane B field43.

Figure 2c also suggests that a (1,1)-singlet can be initialized from a (0,2)-singlet, by changing the energy detuning between the quantum dots while avoiding to pass the S-T− anticrossing. We achieve this by shifting the anticrossing towards the center of the (1,1) charge sector by decreasing the magnetic field to B = 1 mT and increasing the tunnel couplings (Supplementary Fig. 1). Figure 2d demonstrates clear S-T− oscillations observed in this regime for all nearest-neighbor configurations (see also Supplementary Fig. 2). Importantly, these oscillations also enable to determine the singlet/triplet states on two parallel quantum dot pairs by using sequential readout47.

Tuning of individual exchanges using coherent oscillations

The overlap of the HS eigenstates with \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\) depends on Jx and Jy (see Supplementary Note 4). A quantitative comparison between experiments and theoretical expectations thus requires fine control over the exchange couplings.

In this purpose, we focus on the evolution of coherent four-spin ST oscillations. These oscillations are induced using the experimental sequence depicted in Fig. 3a (see also Supplementary Fig. 3). We turn off two parallel exchange couplings and initialize a \(\left\vert {S}_{x}\right\rangle\) or a \(\left\vert {S}_{y}\right\rangle\) state in parallel double quantum dots. We then rotate one of the singlet pairs to a triplet \(\left\vert {T}^{-}\right\rangle\) state through coherent time evolution after pulsing to the S-T− anticrossing, creating a four-spin singlet-triplet product state (e.g., \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\) or \(\left\vert {T}_{23}^{-}{S}_{14}\right\rangle\)). All barrier gate voltages are then diabatically pulsed to turn on all the exchange couplings leading to coherent evolution of the four-spin system. After a dwell time tD, two pairs are isolated (not necessarily the initial ones) and their spin-states are readout sequentially, which allows to deduce spin-correlations of opposite pairs, as was realized in linear arrays in GaAs17.

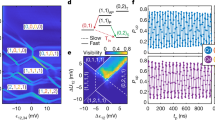
Fig. 3: Four-spin coherent singlet-triplet oscillations and exchange characterization.
figure 3

a Schematics of the pulse sequence used to measure four-spin ST oscillations from an initial \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\) state. b The 2D histograms of the sensor signals formed by sequential 500 single-shot readouts of Q3Q4 and Q1Q2 ST states. The left panel shows the initial state \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\) at tD = 0 ns. The right panel shows the state at tD = 19 ns corresponding to half an oscillation period. (Data corresponds to (c) for δVx = 0). c Oscillations in ST probability \({P}_{{S}_{34}{T}_{12}}\) as a function of gate voltage variation δVx. The amplitude of exchange pulses applied on the virtual barrier gates during the free evolution step are varied between measurements around a predetermined set of barrier gate voltages where Jx < Jy. The amplitude of voltage pulses on vB12,34 are varied anti-symmetrically while the amplitudes of the pulses on vB23,14 are kept constant, as shown in the top illustration. The initial state is \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\). d Similar experiment where oscillations in \({P}_{{S}_{34}{T}_{12}}\) are studied as a function of the gate voltage variation δVy. δVy is the shift in the amplitudes of the exchange pulses applied anti-symmetrically on vB23,14 (see top illustration). The initial state is \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\). e Oscillations in \({P}_{{S}_{23}{T}_{14}}\) (left) and \({P}_{{S}_{34}{T}_{12}}\) (right) as functions of tD and \(\delta {V}_{x}^{{\prime} }\). The amplitude of exchange pulses are varied symmetrically around the operation point (\(\delta {V}_{x}^{{\prime} }=0\) mV where Jx ≃ Jy) according to the top illustration ensuring that J12 ≃ J34 and J14 ≃ J23 along the full voltage range. The initial states are respectively a \(\left\vert {T}_{23}^{-}{S}_{14}\right\rangle\) (left) and a \(\left\vert {T}_{34}^{-}{S}_{12}\right\rangle\) state (right). f Exchange couplings Jx,y extracted by fitting the oscillations in (e) with \(A\cos (2\pi {f}_{ST}{t}_{{{{\rm{D}}}}}+\phi )\exp (-{({t}_{{{{\rm{D}}}}}/{T}_{\varphi })}^{2})+{A}_{0}\) as a function of gate voltage variation \(\delta {V}_{x}^{{\prime} }\). The oscillation frequencies of \({P}_{{S}_{23}{T}_{14}}\) (\({P}_{{S}_{34}{T}_{12}}\)) corresponds to Jx/2h (Jy/2h). The shaded areas correspond to the estimated uncertainty on the exchange couplings derived based on assumptions discussed in Supplementary Note 5.

Full size image

The observation of resonating valence bond requires equal couplings between all four quantum dots. In navigating to this point, we carefully develop a virtual landscape, keep control over all the individual exchange interactions. First, we separately equalize the horizontal (J12 = J34) and vertical (J14 = J23) exchange couplings. Then, we tune the vertical and horizontal exchanges to the same coupling strength. The Chevron patterns displayed in Fig. 3c, d are consistent with a Heisenberg Hamiltonian (see Supplementary Figs. 4–6) and the minima in the oscillation frequency mark the location of equal exchange couplings for horizontal (J12 ≃ J34 ≃ Jx/2 for Fig. 3c) or vertical pairs (J14 ≃ J23 ≃ Jy/2 for Fig. 3d). Through an iterative process, we can find ranges of virtual gate voltages where J12 ≃ J34 and J23 ≃ J14.

We can now control the spin pairs simultaneously, while maintaining the exchange couplings in both the horizontal and vertical directions equal (see Supplementary Note 5), with a priori Jx ≠ Jy. Through the readout of both pairs, we can obtain the frequency of four-spin ST oscillations observed in this regime (Fig. 3e), which is given by fST = Jy/2h or Jx/2h depending on the initial state, and with that determine the exchange interaction. As highlighted in Fig. 3f, the virtual control enables to tune Jx from 15 MHz to 109 MHz with Jy remaining between 46 and 56 MHz. Clearly, the exchange interaction can be controlled and measured over a significant range and tuned to a regime where all couplings are equal (we obtain a precision of ≈ 3 MHz, as discussed in Supplementary Note 5, mostly determined by drifts between experiments).

Valence bond resonances

Valence band resonances can occur when all Jij are equal. To experimentally assess this, we prepare \(\left\vert {S}_{x}\right\rangle\) or \(\left\vert {S}_{y}\right\rangle\), which are superposition states of HS. We then pulse the exchanges such that Jx ≈ Jy. Figure 4a shows the result of the time evolution in this regime of equal exchange couplings. Since we start from a superposition state of HS, the time evolution leads to coherent oscillations between \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\), which results in periodic swaps between the singlet states as depicted in Fig. 4b. In addition, we readout both in the horizontal and vertical configuration, and observe an anti-correlated signal, consistent with signatures of valence bond resonances32,36. The observation of more than ten oscillations shows the relatively high level of coherence achieved during these experiments further confirmed by the characteristic dephasing time Tφ ≈ 150 ns.

Fig. 4: Valence bond resonances.
figure 4

a Probabilities of having horizontal singlet pairs \({P}_{{S}_{12}{S}_{34}}\) and vertical singlet pairs \({P}_{{S}_{23}{S}_{14}}\) as a function of dwell time tD. All the exchange couplings are tuned toward an identical value of Jij/h ≃ 25 MHz. Lines are fits to the data with \({P}_{SS}=1/2\,{{{\mathcal{V}}}}\cos (2\pi {f}_{SS}{t}_{{{{\rm{D}}}}}+\phi )\exp (-{({t}_{{{{\rm{D}}}}}/{T}_{\varphi })}^{2})+{A}_{0}\) giving respectively Tφ = 167 ns and 143 ns for data corresponding to \({P}_{{S}_{12}{S}_{34}}\) and \({P}_{{S}_{23}{S}_{14}}\). The state is initialized as \(\left\vert {S}_{x}\right\rangle\). b Illustration showing valence bond resonances characterized by oscillations between the singlet product states \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\). c \({P}_{{S}_{12}{S}_{34}}\) and, d \({P}_{{S}_{23}{S}_{14}}\) as a function of tD and virtual barrier gate voltage variation \(\delta {V}_{x}^{{\prime} }\). The state is initialized as \(\left\vert {S}_{x}\right\rangle\). e The oscillation frequency as a function of \(\delta {V}_{x}^{{\prime} }\). The blue (red) points are extracted from (c, d). The black points are the theoretical predictions \({f}_{SS}=\sqrt{{J}_{x}^{2}+{J}_{y}^{2}-{J}_{x}{J}_{y}}/h\) computed using the exchanges Jx and Jy measured in Fig. 3e. f Visibility \({{{{\mathcal{V}}}}}_{x,y}\) as a function of gate voltage variation \(\delta {V}_{x}^{{\prime} }\). The triangles in blue (red) are extracted from (c, d). The expected values are derived from equations (5) and (7) of Supplementary Note 4 using the measured exchanges. The shaded areas correspond to one standard deviation from the best fit for the experimental data, and for the theoretical data they correspond to the uncertainties on the amplitude and the frequency computed using the uncertainties on the exchange couplings values. g Ratio of the visibilities \({{{{\mathcal{V}}}}}_{y}/({{{{\mathcal{V}}}}}_{x}+{{{{\mathcal{V}}}}}_{y})\) as a function of the gate voltage variation \(\delta {V}_{x}^{{\prime} }\).

Full size image

Figure 4c, d shows a more detailed measurement, which we can fit using \(\frac{{{{{\mathcal{V}}}}}_{x,y}}{2}\cos (2\pi {f}_{SS}{t}_{{{{\rm{D}}}}}+\phi )\exp (-{({t}_{{{{\rm{D}}}}}/{T}_{\varphi })}^{2})+{A}_{0}\) to extract the evolution of the frequencies fSS and of the visibilities \({{{{\mathcal{V}}}}}_{x,y}\), plotted on Fig. 4e and Fig. 4f. We find a quantitative agreement between the measured frequencies and the theoretical expectation \({f}_{SS}=\sqrt{{J}_{x}^{2}+{J}_{y}^{2}-{J}_{x}{J}_{y}}/h\) despite deviations for the lowest values of \(\delta {V}_{x}^{{\prime} }\) that could result from the uncertainties in the exchange couplings. We also find a qualitative agreement for the visibilities though the measured \({{{{\mathcal{V}}}}}_{x,y}\) remain lower, in particular when the exchange is larger. Fermi-Hubbard simulations and further analysis (see Supplementary Notes 7 and 8) reveal that part of the visibility loss can be attributed to leakage and to the insufficient diabaticity of the voltages pulses. We speculate that the rest of the visibility loss is mainly due to the decoherence induced by the voltage pulses at the manipulation stage, or by pulse distortion arising from the non-ideal electrical response of the wiring. The underlying mechanism affects similarly the results of the measurements in the both readout directions over most of the voltage range spanned (see Supplementary Note 8). Consequently, a more quantitative agreement is reached when comparing the ratio \({{{{\mathcal{V}}}}}_{y}/({{{{\mathcal{V}}}}}_{x}+{{{{\mathcal{V}}}}}_{y})\) (Fig. 4g) of the visibilities measured over the visibilities predicted, similarly as is done in ref. 36. Overall, the good agreement observed confirms that the dynamics is governed by HS.

Preparation of resonating valence bond eigenstates

Having observed valence bond resonances, we now focus on the preparation of eigenstates of HS which are the \(\left\vert s\right\rangle\) and \(\left\vert d\right\rangle\) RVB states. \(\left\vert s\right\rangle\) is the ground state of HS when Jx = Jy, whereas \(\left\vert {S}_{x}\right\rangle\) and \(\left\vert {S}_{y}\right\rangle\) are the ground states when Jx ≫ Jy and Jx ≪ Jy. Experimentally we therefore prepare \(\left\vert s\right\rangle\) from \(\left\vert {S}_{x}\right\rangle\) or \(\left\vert {S}_{y}\right\rangle\) by adiabatically tuning the exchange interactions to equal values. Figure 5a shows experiments where we control the ramp time tramp to tune to this regime and we observe a progressive vanishing of phase oscillations. For large tramp ≳ 140 ns, the oscillations nearly disappear and the measured probability saturates to \({P}_{{S}_{12}{S}_{34}}\simeq 0.78\). Performing similar experiments starting from a \(\left\vert {S}_{y}\right\rangle\) state or measuring \({P}_{{S}_{23}{S}_{14}}\) leads to identical features with singlet-singlet probabilities saturating between 0.66 and 0.72 (see Supplementary Fig. 22). These values are close to the probabilities \({| \langle {S}_{x,y}| s \rangle |}^{2}=3/4\) expected when the s-wave state is prepared.

Fig. 5: Initialization of RVB eigenstates.
figure 5

a Evolution of RVB oscillations as a function of the time to set all exchanges equal (Jij ≃ 25 MHz) (see Fig. 3a). For tramp ≳ 140 ns, the ground state with s-wave symmetry is adiabatically prepared. b Evolution of the singlet-singlet probability \({P}_{{S}_{12}{S}_{34}}\) with \(\delta {V}_{x}^{{\prime} }\) after adiabatic initialization of the ground state. c Evolution of the mean singlet-singlet probability measured after adiabatic initialization of the ground state with \(\delta {V}_{x}^{{\prime} }\) for both readout directions. The experiments are compared to theoretical expectations using exchange coupling values extracted from four-spin singlet-triplet oscillations (Supplementary Notes 4 and 5). The shaded areas correspond to one standard deviation for the experimental data, and for the theoretical data they correspond to the uncertainties on the expected probabilities computed using the uncertainties on the exchange couplings values. Rescaled data (dark red and blue triangles) are obtained by multiplying each raw dataset (red and blue triangles) by a constant factor corresponding to the mean ratio of the predicted probabilities over the measured probabilities. d Experimental sequence used to investigate the formation of the d-wave state. Before the free evolution step, one exchange pulse on vB23 is applied for a time tJ. e, f Evolution of singlet-singlet oscillations measured for different exchange pulse durations tJ. The vanishing of oscillations at tJ ≃ 25 ns marks the formation of a d-wave state. g, h Linecuts of (e) and (f) for tJ = 25 ns.

Full size image

We can now also prepare the ground state HS for arbitrary exchange values, by carefully tuning the ramp time (tramp = 160 ns in our experiments). Figure 5b shows the evolution of \({P}_{{S}_{12}{S}_{34}}\) for different \(\delta {V}_{x}^{{\prime} }\). Since we prepare the ground state, coherent phase evolution results in a \({P}_{{S}_{12}{S}_{34}}\) that is virtually constant for any \(\delta {V}_{x}^{{\prime} }\) and only faint oscillations are observed. \({P}_{{S}_{12}{S}_{34}}\), however, is strongly dependent on \(\delta {V}_{x}^{{\prime} }\), as increasing Jx changes the ground state to \(\left\vert {S}_{x}\right\rangle\).

The measured \({P}_{{S}_{12}{S}_{34}}\) values can be compared with predictions using Jx,y values extracted from four-spin singlet-triplet oscillations (see Supplementary Note 4). Figure 5c shows that a good agreement exists between the theory and the experiments. The raw experimental probabilities \({P}_{{S}_{12}{S}_{34}}\) remains smaller than the theoretical predictions due to systematic errors during the experiments, which are most likely state initialization and readout errors (see Supplementary Note 8). Measuring \({P}_{{S}_{23}{S}_{14}}\) leads to a similar agreement, although the imperfections have a larger impact in this experiment. Rescaling the data by constant factors, that compensate for systematic errors, allows to reach a quantitative agreement, as shown in Fig. 5c. From this we conclude that the ground state of HS is adiabatically initialized in these experiments.

We prepare the d-wave state by including an additional operation where we exchange two neighboring spins36. This results in a transformation of neighboring spin-spin correlations to diagonal correlations. We experimentally implement this step by adding, before the free evolution step, an exchange pulse of duration tJ during which only one exchange coupling is turned on (see Fig. 5d).

Figure 5e, f shows \({P}_{{S}_{12}{S}_{34}}\) and \({P}_{{S}_{23}{S}_{14}}\) measured as functions of tD and tJ in experiments where the system is initialized in \(\left\vert {S}_{x}\right\rangle\) and the exchange J23 is pulsed. As a function of the exchange pulse duration, we observe a periodic vanishing of RVB oscillations (linecuts provided in Fig. 5g, h, imperfections in exchange control cause residual oscillations). Due to the exchange pulse, a periodic swapping of neighboring spins occurs, and thus a periodic evolution between neighboring spin-spin correlations and diagonal correlations. Thus the regime where the d-wave eigenstate is prepared is marked by the vanishing of RVB states. The mean of the probabilities, \({P}_{{S}_{23}{S}_{14}}\simeq 0.21\) and \({P}_{{S}_{12}{S}_{34}}\simeq 0.13\), measured for tJ = 25ns are in the direction of theoretical expectations \({| \langle {S}_{x,y}| d \rangle }|^{2}=1/4\).

Discussion

In this work we demonstrated a coherent quantum simulation using germanium quantum dots. Clear evolution of resonating valence bond states appeared after tuning to a regime where all nearest neigbours have equal exchange coupling. We furthermore established the preparation of the s-wave and d-wave eigenstates. In addition, we have shown that we can control the exchange interaction over a significant range in a multi-spin setting.

The low-disorder and quantum coherence make germanium a compelling candidate for more advanced quantum simulations. Improving the initialization and readout fidelities will enable to observe a stronger correspondence between ideal predictions and experimental results. Additionally, advanced voltage pulsing may facilitate to reduce errors occurring when controlling the spin states. Furthermore, a significant improvement in the quantum coherence may be obtained by exploring sweet spots48 and by using purified germanium.

Controlling multi-spin states is also highly relevant in the context of quantum computation. The realization of exchange-coupled singlet-triplet qubits enables to implement fast two-qubit gates49,50,51,52. Leakage may then be reduced by exploiting the large out-of-plane g-factor for holes in germanium 27,43. Also, operation with four-spin manifolds provides means for decoherence-free subspaces53.

Extensions of this work leveraging the full tunability of germanium quantum dots could provide new insights for extensive studies of strongly-correlated magnetic phases and associated quantum phase transitions. In particular, the implementation of similar simulations in triangular lattices offer new possibilities to investigate the emergence of non-trivial phases arising from frustration33,34. Likewise, the preparation of RVB states and the investigation of their dynamics in larger devices may help to probe their properties experimentally and explore how they relate to superconductivity in doped cuprates31.

Methods

Materials and device fabrication

The device is fabricated on a strained Ge/SiGe heterostructure grown by chemical vapor deposition. Starting from a natural Si wafer, a 1.6 μm thick relaxed Ge layer is grown, followed by a 1 μm reverse graded Si1−xGex (x going from 1 to 0.8) layer, a 500 nm relaxed Si0.2Ge0.8 layer, a 16 nm Ge quantum well under compressive stress, a 55 nm Si0.2Ge0.8 spacer layer and a < 1 nm thick Si cap. The quantum well is contacted by aluminum ohmic contacts after a buffered oxide etch of the oxidized Si cap. The ohmics are isolated from the gates by a 10 nm ALD grown alumina layer. Two sets of Ti/Pd gates, separated by 7 nm of alumina, are deposited on top of the heterostructure to define the quantum dots. The potential of the quantum dots is tuned using the plunger gates (blue in Fig. 1a) while barrier gates are used to tune the tunnel couplings between the quantum dots (green).

Experimental set-up

Experiments are performed in the 2 × 2 array of quantum dots showed in Fig. 1a and the changes of the charge states in the array are measured using two single hole transistors (yellow in Fig. 1a) via rf-reflectometry. Further information regarding the experimental set-up used are provided in ref. 29.

Measurement techniques

Virtual barrier and plunger gate voltages, defined as linear combinations of real gate voltages, are used to tune independently the potentials/couplings and compensate effects of cross-capacitances (see Supplementary Note 1). For four-spin coherent oscillations, the spin–spin probabilities (or equivalently the spin-spin correlations) are determined by reading out sequentially the states of two parallel quantum dot pairs, either first Q3Q4 and then Q1Q2 or first Q2Q3 and then Q1Q4. While reading one pair, the second is stored deep in the (1,1) charge sector to prevent cross-talk between the measurements17,47. The state of each pairs is determined for each single shot-measurements by comparing the sensor signal to a predetermined threshold.