Abstract
Inorganic semiconductor α-Ag2S exhibits a metal-like ductile behavior at room temperature, but the origin of this high ductility has not been fully explored yet. Based on density function theory simulations on the intrinsic mechanical properties of α-Ag2S, its underlying ductile mechanism is attributed to the following three factors: (i) the low ideal shear strength and multiple slip pathways under pressure, (ii) easy movement of Ag–S octagon framework without breaking Ag−S bonds, and (iii) a metallic Ag−Ag bond forms which suppresses the Ag–S frameworks from slipping and holds them together. The easy slip pathways (or easy rearrangement of atoms without breaking bonds) in α-Ag2S provide insight into the understanding of the plastic deformation mechanism of ductile semiconductor materials, which is beneficial for devising and developing flexible semiconductor materials and electronic devices.
Similar content being viewed by others
Introduction
Since 20th century, the semiconductor industry has been recognized one of the most important industrial fields in the world.1 During the last two decades, various novel high-performance semiconductor materials have been explored to facilitate the industrial development process.2,3,4,5 For example, in solid-state thermoelectric (TE) technology, such excellent TE semiconductors as SnSe,6 filled CoSb3,7 PbTe,8 and half-Heusler9 are found to be promising high-performance candidates with high energy conversion efficiency. However, the typical brittleness of semiconductors easily leads to the crack initiation, giving rise to the mechanical failure of semiconductor devices.10 Consequently, highly-reliable semiconductor devices require good ductility of semiconductor materials.
Ductility is a material’s ability to undergo extensive plastic deformation before failure.11 Ductility often occurs in metals and alloys due to metallic bonds, in which the delocalized valence electrons allow metal atoms to slide past each other.12 In contrast semiconductors are generally brittle, because they are mainly comprised of directional covalent bonds or ionic bonds that lead to repulsive interactions when atoms slide.13 Nevertheless, ductility in semiconductors can be realized by heating them to the high temperature or lowering their dimensionality to nanofilm or nanowire form.14,15,16 For example, the brittle-to-ductile transition in single crystal Si was found to occur within the temperature range of 700–950 °C. This is because the thermally-activated dislocation glide becomes the dominate deformation mechanism.14 Si nanowires were found to exhibit a large-strain plasticity at room temperature, which originates from a dislocation-initiated amorphization.15 Recently, α-Ag2S, which has been widely applied in optoelectronic and electronic devices, was found to be a ductile inorganic semiconductor at room temperature with a large plastic deformation strain, such as >50% for compression, 4.2% elongation for tension.17 This offers promise for the application of ductile materials in flexible electronic devices. The ductility mechanism was deeply discussed before.17 In particular, chemical bonding analysis showed that irregularly distributed Ag–Ag and Ag–S bonds suppress α-Ag2S from cleavage, resulting in the ductility of α-Ag2S.17 However, the deformation mechanism of α-Ag2S that leads to ductility is not fully explored yet.
To understand the mechanism for ductile behavior of semiconducting α-Ag2S, we applied ab-initio based density function theory (DFT) to investigate the plastic structural response under pure shear, uniaxial tension, and biaxial shear deformations, respectively. We found that
-
α-Ag2S has a low ideal shear strength of 1.02 GPa along (001)[010] and (100)[010] slip systems, which is much lower than the ideal tensile strength of 2.22 GPa. This is expected to create pathways easy slip.
-
The Ag-S octagon frameworks are highly distorted accommodating the (001)[010] shear deformation with Ag−S bond lengths slightly changed. This retains the structural integrity, leading to the plasticity of α-Ag2S along (001)[010].
-
A newly formed Ag−Ag metallic bond couples the stacked Ag–S frameworks, suppressing their sliding and maintaining the structural integrity under the (100)[010] shear load. This strain-stiffening is the origin of plastic behavior of α-Ag2S along (100)[010].
-
The deformation strain under nano-indentation is expected to be smaller than that under pressure.
Results and discussion
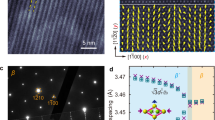
Semiconductor α-Ag2S crystalizes in monoclinic structure (space group P21/c (14)) at room temperature,18 as shown in Fig. 1. The unit cell contains 8 × Ag and 4 × S atoms with mirror symmetry along a–b, b–c, or a–c plane, as highlighted by the red dashed line in Fig. 1a–c. The large Pauling electronegative difference (Δχ = 0.65) between Ag (χAg = 1.93) and S (χS = 2.58) suggests an ionic bonding character between Ag and S. This agrees well with the electron localization function (ELF) analysis which shows localized isosurfaces around the S atom (Fig. 1a–c). α-Ag2S is a layered structure with the “zigzag” Ag–S framework stacked along the [100] direction (a axis) and connected with Ag−S bonds of 2.67 Å (Fig. 1a). The Ag-S framework is comprised of a distorted 4 × Ag − 4 × S octagon with Ag−S bond length of 2.52 Å along the [001] direction (c axis) and 2.41 Å along the [010] direction (b axis), respectively (Fig. 1b). In particular, each Ag is two-fold coordinated with S atoms, with a distorted S−Ag−S angle of 133° along the [001] direction (c axis). While each S atom is 4-fold coordinated with Ag atoms, each with Ag−S−Ag angle of ~77° (Fig. 1d).
Crystal structure of α-Ag2S. a Structure with ELF (at the value of 0.65) along a–b plane highlighting the Ag–S sub-layers. b Structure along b–c plane. c Structure along a–c plane. d Ag–S framework highlighting the distorted octagon consisted of 4 × Ag and 4 × S atoms. In Fig. 1a–c, the black quadrangle line shows the unit cell of α-Ag2S, and the dashed red line highlights the mirror symmetry plane which can act as a twin boundary
To understand the intrinsic mechanical properties of α-Ag2S, we used DFT to compute the stress responses under pure shear and biaxial tensile strain, respectively (Fig. 2). The ideal shear strength, which is defined as the first maximum stress point, is found to be 1.02 GPa both along (100)[010] and (001)[010] slip systems. This value is lower than that of the shear strength (1.72 GPa) along the (100)[00-1] system and much lower than the ideal tensile strength of 2.22 GPa along [100], suggesting both (100)[010] and (001)[010] are the most plausible slip systems activated under pressure. In the (001)[010] shear deformation the structure undergoes an extremely large plastic deformation, with the shear strain increasing from 0.16 to 1.20 before failure. Whereas shearing along the (100)[010] slip system the structure first undergoes a slight strain-softening from 0.42 to 0.66 shear strain, and then a strong strain-stiffening process from 0.67 to 1.09 shear strain before failure (Fig. 2a). Thus, α-Ag2S clearly exhibits strong ductile character against external deformation. In addition, the [100] tension shows an obvious structural softening and yielding process from 0.12 to 0.28 tensile strain as well (Fig. 2b). However, Fig. 2 clearly shows that the plastic deformation of α-Ag2S for [100] tension is far inferior to the shearing along the (001)[010] and (100)[010]. This clearly explains why α-Ag2S shows an over 50% deformation strain under compression but a much smaller elongation of 4.2% under tension experimentally.17
To understand the underlying ductile mechanism of α-Ag2S, we examine the atomic patterns and bond changes along its least stress slip systems: (001)[010] and (100)[010]. Figure 3 shows the pure shear deformation mechanism along the (001)[010] system. Due to a twin-like rearrangement at the mirror plane indicated in Fig. 1, the Ag–S octagon framework is distorted enabling shear strain without breaking Ag–S bonds (Fig. 3b). As the shear strain increases to 0.417 corresponding to the ideal shear strength, the Ag1−S1−Ag3 angle is remarkably bent from 76.5° to 110.4° (44.3%), while the Ag2−S1−Ag3 angle is slightly shrunk from 76.5° to 71.4° (6.7%) as shown in Fig. 3f. With the shear strain continuously increasing to 1.149 before failure, the Ag1−S1−Ag3 angle is further bent to 148.6°. However, the system retains its structural integrity (Fig. 3c) because all the bonds are held together, as shown in Fig. 3e. At the failure strain of 1.196, the Ag4−S1 length sharply increases to 4.35 Å. The Ag4−S1 bond breakage deconstructs the Ag–S octagon framework, leading to the failure of α-Ag2S (Fig. 3d). After structural rearrangement (Fig. 3d), S1 atom is 3-coordinated with Ag1, Ag2, and Ag3 atoms, with Ag–S bond lengths of 2.44–2.57 Å. While the rest each S atom is still 4-coordinated with Ag atoms. However, the Ag4−S1 bond breakage leads to significant changes of Ag−S−Ag bond angles (Fig. 3d), i.e., Ag1−S1−Ag3 angle reducing from 146.7° to 117.0° (Fig. 3f). This structural change releases the internal shear stress, resulting in the structural failure. The shear deformation in Fig. 3 clearly shows that the bending of the Ag1−S1−Ag3 angle dominates the plastic mechanism of α-Ag2S for shearing along (001)[010].
Deformation mechanism of α-Ag2S under pure shear load along the (001)[010] slip system. a The initial structure at 0.0 shear strain. b Atomic structure at 0.417 shear strain, which corresponds to the maximum shear stress. c Atomic structure at 1.149 shear strain, before the structural failure. d Atomic structure at the failure strain of 1.196. e The typical bond lengths (Ag1−S1, Ag2−S1, Ag3−S1, and Ag4−S1) as a function of shear strain. f The Ag1−S1−Ag3 and Ag2−S1−Ag3 bond angles as a function of shear strain
Figure 4 displays the atomic configuration and bond-responding process along the (100)[010] slip system. As the system is sheared along the (100)[010], the stacked Ag-S frameworks slide past each other resisting the shear load until 0.671 shear strain (Fig. 4b). The bond-responding response shows that The Ag2−S1 bond is stretched from 2.41 to 2.57 Å (6.6%) as shown in Fig. 4e. While the Ag1−S1 bond is slightly shrunk from 2.41 to 2.37 Å, and the Ag5−S1 and Ag3−S1 bond lengths remain unchanged. This indicates that the stretching and softening Ag2−S1 bond is responsible for the structural softening from 0.417 to 0.671 shear strain shown in Fig. 2a. In addition, a slight stress drop at 0.671 shear strain is observed (Fig. 2a), suggesting a structural rearrangement. It is noted that the Ag1 and Ag5 rapidly move towards each other with the increasing shear strain. At 0.671 shear strain, the Ag1−Ag5 length is 3.15 Å, which is only 8.9% larger than the Ag−Ag bond length (2.89 Å) in metal Ag.19 This suggests that a new Ag1−Ag5 metallic bond forms at 0.671 strain, which starts to strengthen the interaction between these stacked Ag–S frameworks. With the shear strain increasing to 1.087, the Ag5−S1 bond is rapidly stretched and softened (Fig. 4e), but the Ag1−Ag5 length continuously reduces to 2.87 Å which is even shorter than the Ag−Ag length (2.89 Å) in metal Ag,19 completely coupling the stacked Ag–S frameworks with metallic bonds. This explains the strain-stiffening in Fig. 2a, which was also observed in Fe3C and Al3BC2.20 The newly formed Ag1−Ag5 bond suppresses the further slip between stacked Ag–S frameworks, hence maintaining the structural integrity (Fig. 4c) under shear deformation. This is the origin of the ductile behavior of α-Ag2S for shearing along (100)[010]. At the failure strain of 1.102, the Ag2−S1 bond breakage leads to the failure of α-Ag2S (Fig. 4d). While the Ag5−S1 bond recovers from 2.98 to 2.53 Å, further coupling the Ag-S framework along the [100] direction. However, each S atom is still 4-coordinated with Ag atoms, with Ag−S bond lengths of 2.40–2.76 Å. This bond-breakage and bond-recovery process releases the internal shear stress, leading to the failure of Ag2S along (100)[010] slip system (Fig. 4d).
Deformation mechanism of α-Ag2S under pure shear load along the (100)[010] slip system. a The initial structure at 0.0 shear strain. b Atomic structure at 0.671 shear strain, at which the newly formed Ag1−Ag5 bond starts to strengthen the system. c Atomic structure at 1.087 shear strain, before the structural failure. d Atomic structure at the failure strain of 1.102. e The typical bond lengths (Ag5−S1, Ag1−Ag5, Ag2−S1, Ag1−S1, and Ag3−S1) as a function of shear strain
We also extracted the atomic configuration of α-Ag2S along the (100)[00-1] slip system, and found that the breakage of the Ag3−S2 bond decouples the interaction between stacked Ag-S frameworks, leading to their sliding past each other (Fig. S1), and hence causing the failure of α-Ag2S for shearing along (100)[00-1].
In addition, the uniaxial tensile deformation of α-Ag2S along the [100] direction (Fig. S2) suggests that the softening and breakage of the Ag5−S1 bond leads to the structural softening from 0.104 to 0.149 tensile strain (Fig. 2a). While recovery of the Ag6−S3 bond couples the stacked Ag-S frameworks (Fig. S2b), maintaining the structural integrity. This leads to the plastic deformation of α-Ag2S with the further increasing tensile strain from 0.149 to 0.27 (Fig. 2a). At the failure strain of 0.27, the breakage of the Ag3−S2 bond leads to the failure of α-Ag2S along the [100] tension (Fig. S2c).
The major plastic deformation mechanism in metals involves the slip induced dislocations or/and twinning.12,21 This indicates that a ductile material requires multiple slip pathways (or easy movement of atoms) along certain crystallographic planes with good structural integrity. Figure 5 displays the ideal shear strength of various materials. The ideal shear strength of ceramics is much higher than those of metals, suggesting slipping is hard to occur in ceramics. Thus, ceramics are brittle. In 2D layered semiconductor Bi2Te3 and SnSe, their ideal shear strengths are very low and the slipping easily occurs,22,23 but they behave brittle as well.24 This is because the system cannot maintain the structural integrity during the slipping process.22,23 However, α-Ag2S is found to be ductile,17 where we attribute the plastic deformation to the following three mechanisms.
-
i.
The low ideal strength and multiple slip pathways. The ideal shear strength of α-Ag2S is only 1.02 GPa, which is comparable with those of metals (Fig. 5). In addition, we found that both (001)[010] and (100)[010] slip systems are most likely activated under pressure (Fig. 2a), creating easy pathways for slip.
-
ii.
Easy movement of Ag-S octagon framework without breaking Ag−S bonds. As shown in Fig. 3, the Ag1−S1−Ag3 angle is highly bent accommodating the shear deformation while Ag−S bond lengths are only slightly changed, maintaining the structural integrity. This leads to the plastic deformation of α-Ag2S along (001)[010].
-
iii.
Ag−Ag bond formation holds stacked Ag–S frameworks together. The Ag1−Ag5 bonding couples the stacked Ag-S frameworks, suppressing their further sliding and maintaining the structural integrity. This strain-stiffening is the origin of plastic behavior of α-Ag2S along (100)[010].
To further understand the deformation of α-Ag2S under nano-indentation conditions, we examined the stress response of (100)[010] oriented α-Ag2S against biaxial shear (shear and compression) deformation, as shown in Fig. 6. We found that compression has little influence on the ductile character but lowers the strength of α-Ag2S. α-Ag2S exhibits the similar stress response under biaxial shear and pure shear load. The same strain-stiffening is also observed under biaxial shear load. This is attributed to the similar bond response (Fig. S3): The Ag1−Ag5 bond formation strengthens the material, holding the stacked Ag–S frameworks together and maintaining the structural integrity. Our results show that α-Ag2S is ductile as well under nano-indentation. However, the deformation strain under nano-indentation is expected to be smaller than that under pressure, as shown in Fig. 6.
The mechanical properties of polycrystalline α-Ag2S heavily associate with defects such as dislocations and grain boundaries. This is why the ideal shear strength (1.02 GPa) of flawless α-Ag2S is much higher than the experimental shear strength (~150 MPa) of polycrystalline α-Ag2S.17 The investigation on the deformation mechanism of polycrystalline α-Ag2S requires a large-scale molecular dynamics (MD) simulation that can model these defects. It is worth to examine the deformation mechanism in much larger systems using MD simulations in the future, and compare with our current DFT results.
In conclusion, we applied ab-initio based DFT calculations to determine the ductile deformation mechanism of the inorganic semiconductor α-Ag2S. We attribute its ductile deformation to three aspects:
-
i.
The ideal shear stress of α-Ag2S (1.02 GPa) is comparable with metals, leading to multiple slip systems ((001)[010] and (100)[010]) under pressure.
-
ii.
The Ag–S octagon framework is easily distorted without breaking Ag−S bonds.
-
iii.
A newly formed Ag−Ag metallic bond can couple the stacked Ag–S frameworks along the [100] direction (a axis), suppressing their sliding and maintaining the structural integrity.
This work reveals that the easy slip pathways (or easy movement of atoms) with good structural integrity is the origin of ductility in α-Ag2S. This offers a new insight into understanding the plastic deformation mechanism of ductile semiconductor materials, which is helpful for the design and the development of flexible semiconductor devices.
Methodology
All ab-initio based DFT calculations were carried out with the Vienna ab-initio Simulation Package (VASP) code,25,26,27 using the projector augmented wave (PAW) method and the Perdew-Burke-Ernzerhof (PBE) based generalized gradient approximation.28 A energy cutoff of 500 eV was adopted for convergence on structure, stress, and force, with an electronic energy and force convergence criteria of 1 × 10-6 eV and 1 × 10-2 eV/Å. The k-points were sampled on Г-centered symmetry reduced Monkhorst-Pack meshes with a fine resolution of 2π × 1/40 Å−1 for all supercell (96 atoms) deformation simulations. The 4d105s1 and 3s23p4 electrons define the valence shell for Ag and S, respectively. The electron localization function (ELF) was calculated to understand the chemical bonding in α-Ag2S.29 The pure shear, uniaxial tensile, and biaxial shear deformation simulations are similarly with our previous studies,22,23,30,31 which is explained in the Supplementary Information.
Data availability
The data that support the findings of this study are available from the corresponding author (G.L., Q. Z., or G.J.S.) upon reasonable request.32
References
Sreejith, P. S., Udupa, G., Noor, Y. B. M. & Ngoi, B. K. A. Recent advances in machining of silicon wafers for semiconductor applications. Int. J. Adv. Manuf. Technol. 17, 157–162 (2001).
Duan, X. F. et al. High-performance thin-film transistors using semiconductor nanowires and nanoribbons. Nature 425, 274–278 (2003).
Ko, H. et al. Ultrathin compound semiconductor on insulator layers for high-performance nanoscale transistors. Nature 468, 286–289 (2010).
Schmidt, R. et al. High-performance air-stable n-channel organic thin film transistors based on halogenated perylene bisimide semiconductors. J. Am. Chem. Soc. 131, 6215–6228 (2009).
Li, L. Q. et al. An Ultra closely pi-stacked organic semiconductor for high performance field-effect transistors. Adv. Mater. 19, 2613–2617 (2007).
Zhao, L. D. et al. Ultrahigh power factor and thermoelectric performance in hole-doped single-crystal SnSe. Science 351, 141–144 (2016).
Tang, Y. L. et al. Convergence of multi-valley bands as the electronic origin of high thermoelectric performance in CoSb3 skutterudites. Nat. Mater. 14, 1223–1228 (2015).
Pei, Y. Z. et al. Convergence of electronic bands for high performance bulk thermoelectrics. Nature 473, 66–69 (2011).
Zeier, W. G. et al. Engineering half-Heusler thermoelectric materials using Zintl chemistry. Nat. Rev. Mater. 1, 16032 (2016).
Barako, M. T., Park, W., Marconnet, A. M., Asheghi, M. & Goodson, K. E. Thermal cycling, mechanical degradation, and the effective figure of merit of a thermoelectric module. J. Electron. Mater. 42, 372–381 (2013).
Taylor, G. I. The mechanism of plastic deformation of crystals. Part I. Theoretical. Proc. R. Soc. Lond. A 145, 362–387 (1934).
Barret, C. S., & Massalski, T. B. Structure of Metals. (Pergamon: Oxford, 1992).
Yu, P. Y., & Cardona, M. Fundamentals of Semiconductors: Physics and Materials Properties. (Springer, NewYork, 2010)..
John, C. S. The brittle-to-ductile transition in pre-cleaved silicon single crystals. Philos. Mag. 32, 1193–1212 (1975).
Han, X. D. et al. Low-temperature in situ large-strain plasticity of silicon nanowires. Adv. Mater. 19, 2112–2118 (2007).
Zheng, K. et al. Atomic mechanisms governing the elastic limit and the incipient plasticity of bending Si nanowires. Nano Lett. 9, 2471–2476 (2009).
Shi, X. et al. Room-temperature ductile inorganic semiconductor. Nat. Mater. 17, 421–426 (2018).
Blanton, T., Misture, S., Dontula, N. & Zdzieszynski, S. In situ high-temperature X-ray diffraction characterization of silver sulfide, Ag2S. Powder Diffr. 26, 114–118 (2011).
Spreadborough, J. & Christian, J. W. High-temperature X-ray diffractometer. J. Sci. Instrum. 36, 116–118 (1959).
Jiang, C. & Srinivasan, S. G. Unexpected strain-stiffening in crystalline solids. Nature 496, 339–342 (2013).
Alexandrov, I. V. & Chembarisova, R. G. Development and application of the dislocation model for analysis of the microstructure evolution and deformation behavior of metals subjected to severe plastic deformation. Rev. Adv. Mater. Sci. 16, 51–72 (2007).
Li, G. et al. Superstrengthening Bi2Te3 through nanotwinning. Phys. Rev. Lett. 119, 085501 (2017).
Li, G. et al. Ideal strength and deformation mechanism in high-efficiency thermoelectric SnSe. Chem. Mater. 29, 2382–2389 (2017).
Zhao, L. D., Zhang, B. P., Li, J. F., Zhang, H. L. & Liu, W. S. Enhanced thermoelectric and mechanical properties in textured n-type Bi2Te3 prepared by spark plasma sintering. Solid State Sci. 10, 651–658 (2008).
Kresse, G. & Furthmuller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comp. Mater. Sci. 6, 15–50 (1996).
Kresse, G. & Furthmuller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186 (1996).
Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758–1775 (1999).
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996).
Silvi, B. & Savin, A. Classification of chemical-bonds based on topological analysis of electron localization functions. Nature 371, 683–686 (1994).
Li, G. et al. Enhanced strength through nanotwinning in the thermoelectric semiconductor InSb. Phys. Rev. Lett. 119, 215503 (2017).
Li, G. et al. Brittle failure mechanism in thermoelectric skutterudite CoSb3. Chem. Mater. 27, 6329–6336 (2015).
Kostenetskiy, P. S. & Safonov, A. Y. SUSU Supercomputer Resources. Proceedings of the 10th Annual International Scientific Conference on Parallel Computing Technologies. (Arkhangelsk, Russia, 2016).
Ogata, S., Li, J., Hirosaki, N., Shibutani, Y. & Yip, S. Ideal shear strain of metals and ceramics. Phys. Rev. B 70, 104104 (2004).
Ogata, S., Li, J. & Yip, S. Ideal pure shear strength of aluminum and copper. Science 298, 807–811 (2002).
An, Q., Goddard, W. A. & Cheng, T. Atomistic explanation of shear-induced amorphous band formation in boron carbide. Phys. Rev. Lett. 113, 095501 (2014).
Acknowledgements
This work is partially supported by NSF of China under No. 51772231, the 111 Project of China under Project no. B07040. Q.A. was supported by the National Science Foundation CMMI program under grant no. 1727428. S.M. was thankful for the support by Act 211 Government of the Russian Federation, under No. 02.A03.21.0011 and by the Supercomputer Simulation Laboratory of South Ural State University.
Author information
Authors and Affiliations
Contributions
G.L., P.Z., Q.Z., and G.J.S. designed the idea. G.L., Q.A., Q.Z., and G.J.S. wrote the manuscript. G.L., S.M., and W.A.G. performed the DFT calculations. All authors discussed the results and commented on the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Li, G., An, Q., Morozov, S.I. et al. Ductile deformation mechanism in semiconductor α-Ag2S. npj Comput Mater 4, 44 (2018). https://doi.org/10.1038/s41524-018-0100-0
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41524-018-0100-0
This article is cited by
-
Plasticity in single-crystalline Mg3Bi2 thermoelectric material
Nature (2024)
-
Defect-free and crystallinity-preserving ductile deformation in semiconducting Ag2S
Scientific Reports (2022)
-
Theoretical insights into the Peierls plasticity in SrTiO3 ceramics via dislocation remodelling
Nature Communications (2022)
-
Shear induced deformation twinning evolution in thermoelectric InSb
npj Computational Materials (2021)