Abstract
Polar skyrmions are predicted to emerge from the interplay of elastic, electrostatic and gradient energies, in contrast to the key role of the anti-symmetric Dzyalozhinskii-Moriya interaction in magnetic skyrmions. Here, we explore the reversible transition from a skyrmion state (topological charge of −1) to a two-dimensional, tetratic lattice of merons (with topological charge of −1/2) upon varying the temperature and elastic boundary conditions in [(PbTiO3)16/(SrTiO3)16]8 membranes. This topological phase transition is accompanied by a change in chirality, from zero-net chirality (in meronic phase) to net-handedness (in skyrmionic phase). We show how scanning electron diffraction provides a robust measure of the local polarization simultaneously with the strain state at sub-nm resolution, while also directly mapping the chirality of each skyrmion. Using this, we demonstrate strain as a crucial order parameter to drive isotropic-to-anisotropic structural transitions of chiral polar skyrmions to non-chiral merons, validated with X-ray reciprocal space mapping and phase-field simulations.
Similar content being viewed by others
Introduction
A structural transition in materials involves the rearrangement of a periodic array of motifs in response to external stimuli, which governs the materials’ functional properties. Such a transition is not limited to atoms, but also anticipated in lattices consisting of unconventional quasi-particles such as skyrmions or merons1,2,3,4. The recent discovery of non-trivial topological textures, including flux-closure5,6,7,8, vortices9,10,11,12,13,14,15, skyrmion16,17,18,19,20,21, merons22,23,24, or hopfions25 in ferroelectric-oxide nanostructures provides a framework for exploring topology and exotic physical phenomena in condensed matter physics with a focus on polar order26,27,28. Polar skyrmion bubbles consist of three-dimensional (3D) electric dipole textures which, plane by plane, are characterized by an integer topological charge, called the skyrmion number, and defined as
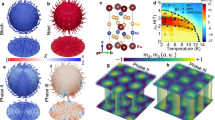
where \(\vec{n}\) is the normalized local dipole moment. Merons are another kind of topological solitons. To understand the differences in their topology, Fig. 1 sketches the dipolar textures for a Bloch-like skyrmion versus a meron. As shown in Fig. 1A, B, at the central xy-plane, a polar skyrmion exhibits an out-of-plane polarization (Pop) at the core and an antiparallel Pop outside the boundary (magenta arrows, Fig. 1A, B). The polarization continuously rotates in such a way that the in-plane component shows a curling Bloch-like pattern29 (blue/yellow arrows, Fig.1A, B) that provides chirality to the skyrmion20. In contrast, merons (Fig. 1C–E) exhibit Pop at the core and gradually evolve to in-plane Pip at the periphery, without being fully surrounded by an antiparallel Pop component30. As an example, a meron and a skyrmion can have the same Pip texture but vary only by their Pop components. Therefore, simultaneous three-dimensional experimental characterization is necessary to correctly determine the topology of the polar textures, and we show how both in-plane and out-plane polar order can be mapped simultaneously.
Schematic of Bloch-like skyrmions with (A) right-handed and (B) left-handed chiralities, both possessing a topological charge of \({N}_{{Sk}}=-1\). A common feature of both the Bloch (\(\nabla \times {{{{{\boldsymbol{P}}}}}} \,\ne\, 0,\,{N}_{{Sk}}=-1\)) and Néel (\(\nabla \cdot {{{{{\boldsymbol{P}}}}}}\ne 0,\,{N}_{{Sk}}=-1\)) skyrmions is the out-of-plane polarization rotates from maximal at the core, to maximal in the opposite direction at the periphery. Instead, the meronic phases can be characterized by a vanishing out-of-plane polarization at the periphery, and a non-zero value at the core. The in-plane component of the polarization presents a non-vanishing vorticity. In the case of a vortex, both right-handed (C), and left-handed (D) chiralities are possible, with a topological charge of \({N}_{{Sk}}=-1/2\). In the case of an antivortex, and although the total topological charge might remain invariant if we reverse the direction of the out-of-plane polarization (E), the final meron configuration is achiral.
A fundamental question pertaining to the spatial arrangement of the skyrmions is the degree of long-range order, if any, amongst the skyrmions, in terms of both the orientational order as well as translational order4,31. The emergence of long range order, or conversely the disappearance of a possible long range order in the polar skyrmion lattice driven by temperature can provide a heretofore unexplored possibility of a melting phase transition in such a topologically protected two-dimensional (2D) array of polar skyrmions (akin to the well-known Kosterlitz-Thouless-Halperin-Nelson-Young (KTHNY) transition)4,32. Although phase field models had predicted the possibility of forming a long-range ordered skyrmion lattice for certain values of mismatch strain with the substrate33, experimentally this is yet to be demonstrated. Given that the magnitude and sign (compressive vs. tensile) of the strain in the superlattice is a critical component of such long-range order, we sought to manipulate this by lifting off the superlattice from the substrate34,35,36. In doing so, we are able to study the ground state of the skyrmions without any interference from substrate constraint. On such a free-standing membrane, we then imposed different elastic boundary conditions by varying the temperature, due to differences in thermal expansion of the two materials, to manipulate the degree of long-range order in the skyrmion lattice.
Here, we report the direct observation of sequential structural transformations for the polar skyrmions: from stripe-shaped to circular-shaped disordered skyrmions bubbles, to a tetratic-ordered meron lattice in lifted-off [(PbTiO3)16/(SrTiO3)16]8 superlattice membranes through integrated experimental measurements and theoretical phase-field calculations. Electron imaging of the polar distortions can be challenging due to local sample mis-tilt artifacts which can affect or dominate the contrast. To disentangle tilt and polarization, we developed an approach for the analysis of Kikuchi bands by using the intensity asymmetry in diffuse scattering, recorded by four-dimensional scanning transmission electron microscopy (4D-STEM) with an electron microscopy pixel array detector (EMPAD)37. Using the high-dynamic-range EMPAD, we were able to collect the full scattering distribution, including Kikuchi bands and Bragg reflections (>104 difference in diffracted intensities), which also contains lattice information for determining the strain state of the specimen. Using 4D-STEM and dynamical diffraction analysis we also demonstrate, for the first time, a direct experimental determination of chirality of individual polar skyrmions. Our observations reveal the emergence of a square meron lattice with Nsk = −1/2 from the disordered skyrmion phase with a Nsk = −1, in which the chirality changed from left-handed to zero-net chirality, respectively.
Results
Experimental setup of 4D-STEM
To examine the local polarization distribution, we performed 4D-STEM experiments on plan-view, lifted-off samples (Fig. S1) to image the in-plane Bloch components (details in Methods). Briefly, 4D-STEM works by using an EMPAD detector which records the 2D electron diffraction pattern over a 2D grid of probe positions, resulting in 4D datasets (Fig. 2A, details in Methods)38,39,40. As a result of dynamical diffraction effects, the charge redistribution associated with ferroelectric polarization leads to the breakdown of Friedel’s law41,42. Thus, due to channeling effects (Fig. S2), the polarization field within the top PbTiO3 layer can be measured quantitatively from intensity differences of polarity-sensitive Kikuchi bands43,44 (Fig. 2B, D) or Bragg reflections45,46 (Fig. S3). We note that due to different channeling conditions, for plan-view imaging, atomic-resolution STEM is more sensitive to the top of the skyrmion, where the Néel component dominates, while the 4D-STEM method is more sensitive to the middle of the skyrmion where Bloch component dominates. To illustrate these channeling effects, we have performed multislice simulations of HAADF-STEM (semi-convergence angle of 21.4 mrad) and 4D-STEM datasets (semi-convergence angle of 2.45 mrad) on a model polar skyrmion obtained from 2nd-principles calculations (Fig. S4).We employed these Kikuchi bands for polarity mapping as they are less sensitive to artifacts such as disinclination strain or crystal mis-tilts, which are inevitable in ferroic oxides47,48 (Figs. S5 and S6). These Kikuchi bands are generally weak features require detectors of high dynamic range which is only available until very recently37. The fast detectors also allow for the mapping of polar textures which outruns the charging effects caused by the focused electron beam. For example, Fig. 2C shows a high-angle annular dark-field (HAADF) image of the skyrmions from plan-view reconstructed from the 4D-STEM dataset, where the out-of-plane polarization (Pop) is separated by domain walls with circular or elongated features. At the same region (same 4D-STEM dataset), Fig. 2E shows the in-plane polarization (Pip) map of Bloch-like rotation can be reconstructed by using the polarity-sensitive Kikuchi bands. The white arrows and colors in Fig. 2E denote the direction of Pip, whereas the saturation represents the vector magnitude. The dark color indicates the Pop regions.
A Schematic of the plan-view 4D-STEM imaging technique on the [(PbTiO3)16/(SrTiO3)16]8 superlattice which uses a scanning electron probe and pixelated array detector, where a diffraction pattern was recorded at each probe position. The local polarization direction can be determined by observing the difference of diffracted intensities of Friedel pairs, \({I}_{\mathop{G}\limits^{ \rightharpoonup }}\) and \({I}_{-\mathop{G}\limits^{ \rightharpoonup }}\). Representative diffraction patterns taken from (B) top and (D) bottom of a skyrmion, where the polarity-sensitive Kikuchi bands in the thermal diffuse scattering are selected for determining polarization, as marked by pink and yellow boxes. For clarity of display, the Kikuchi bands intensity were weighted by k2, where \(\mathop{{{{{{\mathrm{k}}}}}}}\limits^{ \rightharpoonup }\) denotes the scattering vector from the transmitted spot. C Plan-view dark field STEM image of a (SrTiO3)16/(PbTiO3)16/(SrTiO3)16 trilayer reconstructed from the 4D-STEM dataset showing nanometer-size round and elongated features. E Polarization map reconstructed using Kikuchi bands from the same region as C showing the in-plane Bloch components of polar-skyrmions. The color map represents the in-plane polarization direction at each point.
Observation of topological phase transition in polar textures
Upon heating from 223 K to 373 K, we observed successive structural transitions in the skyrmion ensemble, from striped to circular-shaped polar skyrmions to a tetratic-ordered lattice. Figure 3A shows the polarization configuration at 223 K, which consists of elongated stripes of ~100 nm in length. In analogy with in-plane cuts (\({Q}_{x}-{Q}_{y}\)) from X-ray reciprocal space maps (RSM), the fast Fourier transform (FFT) patterns of plan-view HAADF images indicate the in-plane ordering of polar textures (Fig. S7). For example, two peaks were found in the FFT pattern along the pseudocubic ±(100)pc directions (inset, Fig. 3A) consistent with the stripe features.
Plan-view dark field STEM imaging of [(SrTiO3)16/(PbTiO3)16]8 superlattice acquired with temperatures at (A) 223 K, (B) 298 K, and (C) 373 K. Insets, fast Fourier transform (FFT) of the images in A–C showing different types of ordering. Polarization maps reconstructed from the 4D-STEM dataset of superlattice at (D) 223 K, (E) 298 K, and (F) 373 K, showing the in-plane Bloch components of polar-skyrmions. D–F are acquired from nearby regions of A–C. The corresponding phase-field simulations with temperatures and in-plane lattice parameters (a,b) of (G) 223 K, a = 3.875 Å, b = 3.885 Å; H 298 K, a = b = 3.905 Å; and (I) 373 K, a = b = 3.899 Å. The color wheel hue (saturation) corresponds to the direction (magnitude) of the in-plane component of the ferroelectric polarization. We note that the phase field simulations capture the qualitative changes in morphology of particle-like objects as a function of temperature/strain conditions. However, while the general symmetry of the phase is predicted, the microscopic details of polar vectors at the boundaries require first-principles based calculations.
When heated to 298 K, the stripes deformed into circular shapes (Fig. 3B, E) of ~10 nm in diameter. The packing of circular skyrmions appears to be random and isotropic, i.e., no preferred orientational order, as indicated by the halo in the FFT pattern (inset, Fig. 3B). With further increase of temperature to 373 K, the random skyrmion arrangements were replaced by an ordered tetratic arrangement (Fig. 3C), confirmed by the four peaks in ±(100)pc and ±(010)pc directions in the corresponding FFT. Figure 3D-F show the corresponding polarization maps at different temperatures obtained from 4D-STEM datasets. For the stripe- (223 K) and disordered, circular-shaped (298 K) skyrmions, the polarization maps (Fig. 3D, E) indicate that the maximum Pip is observed at the periphery of the skyrmions, whereas the minima (almost zero) are at the core and outside the boundaries. Figure 3G–I show the corresponding phase-field simulations of polar textures at different temperatures and under various strain conditions, in which the average effective in-plane lattice constants are obtained from experimental measurements of >80,000 convergent beam electron diffraction (CBED) patterns (Fig. S8). The phase field simulations demonstrate a systematic change from the labyrinthine skyrmions at low temperature (223 K) to an ordered tetratic structure at higher temperatures (373 K), driven not by a pure thermal effect but by the changes in the in-plane strain state originating from lattice thermal expansion.
To compare the difference of polar textures, regions in Fig. 4A, B (yellow box) were selected to show the Pip polarization maps (Fig. 4C, D). The white arrows and colors in Fig. 4C, D denote the magnitude and the direction of Pip, whereas the saturation represents the vector magnitude. The dark color indicates the Pop regions at skyrmion cores and outside the boundary, which are separated by Bloch domain walls (Pip) consistent with cross-section data (Fig. S9). The Pop at the skyrmion cores points positively towards the growth direction ([001], or +z) and are antiparallel to Pop outside of the boundary, labeled as green dots (+z) and red crosses (−z), respectively (Fig. 4E). The Bloch components (Pip) exhibit a continuous rotation of the local polarization vector forming a closed loop, as illustrated in a map of the curl of the polarization vector field \({\left(\nabla \times {{{{{\bf{P}}}}}}\right)}_{\left[001\right]}\) (Fig. 4A). Both elongated and circular skyrmions exhibit Pip having clockwise (CW) rotation at the periphery yielding a vorticity of +1. Combining Pip and Pop information, we confirm that both elongated and circular skyrmions manifest with a skyrmion number of Nsk = −1.
The curl of in-plane polarization (\(\mathop{\nabla }\limits^{ \rightharpoonup }\times \mathop{P}\limits^{ \rightharpoonup }\))[001] showing the rotation directions of the structures at temperatures of (A) 298 K and (B) 373 K. C, D Enlarged in-plane polarization mapping from the yellow box region of A and B, respectively exhibiting the skyrmion texture at 298 K and local ordered meron textures at 373 K. E, F Details of (C, D), where vortices (clockwise: green, counterclockwise: blue) and antivortices (red) are labeled. The dots in circles represent polarization pointing out of the page, while the cross points into the page.
The most striking observation is the appearance of an ordered structure at 373 K (Fig. 4D, F), which represents a square meron lattice with Nsk = −1/2. Figure 4F clearly demonstrates these periodic arrays, which also indicates that the maximum Pip polarization is observed at the periphery, whereas the minimum (almost zero) is at the core. Three types of core regions were observed, which we labeled according to the cores of Pip having CW rotation (green), counterclockwise rotation (CCW; blue), and antivortices (red). The dot in the circle indicates the Pop pointed out of the page (along the growth direction), while the cross indicates Pop pointing into the page. From a cross-section polarization map (Fig. S9), the Pop at the cores of vortices (vorticity of +1) and antivortices (vorticity of −1) are plausibly antiparallel. On the other hand, Pop appears at vanishing points of Pip, implying the Pop at vortex cores are not fully surrounded by Pop of the opposite direction (Fig. S10). From this, we can compute the skyrmion number3:
where \(v\) represents the vorticity, \({P}_{{op}}^{c}\) the value of the out of plane polarization at the core and \({P}_{{op}}^{p}\) the value of the out of plane polarization at the periphery. We deduce the vortices (blue and green dots) to be merons with a topological number of \({N}_{{Sk}}=\frac{1}{2}1\cdot \left(0-1\right)=-\frac{1}{2}\), and the antivortices as merons25 with \({N}_{{Sk}}=\frac{1}{2}\left(-1\right)\cdot \left(0-\left(-1\right)\right)=-\frac{1}{2}\) (Fig. 1).
Imaging chirality of individual polar texture
While 4D-STEM works well for mapping Pip polarizations, we have so far only speculated about the corresponding Pop at skyrmion cores based on the cross-section data (Fig. S9). To test our hypothesis, it is necessary to experimentally determine the chirality of the 3D polar-vector field for each skyrmion, which poses challenges for projection techniques such as TEM. Fortunately, we can overcome this problem by utilizing the dynamical diffraction effects in higher-order Laue zone (HOLZ) reflections, which was established to retrieve 3D structural information such as handedness of chiral crystals49,50. In this study, we specifically examine the intensity differences of chirality sensitive Bijvoet pairs51, such as (671)/(\(\bar{6}71\)) and (771)/ (\(\bar{7}71\)) (yellow box, Fig. 5A).
A A representative experimental diffraction pattern acquired at 373 K (from the meron square lattice phase), oriented ~6.2° away from [001] zone axis, and tilted along one of the mirror planes. B Map of normalized intensity difference between (771) and (\(\bar{7}71\)) reflections reconstructed from the 4D-STEM dataset—i.e. one diffraction pattern is recorded at each spatial pixel in B. The positive (negative) regions indicate the polar textures having left-handed (right-handed) chirality. C Intensity line profiles of HOLZ reflections from diffraction patterns taken in from the selected regions #1, #2 labeled in B, displaying the intensity difference between two pairs of reflections: (671) and (\(\bar{6}71\)), (771) and (\(\bar{7}71\)). The colored labels in B indicates the region from which the diffraction patterns of C were extracted. The colored diffraction intensity profiles with markers show experimental data, whereas the simulation of a right-handed meron is shown in dashed gray line at the bottom. The dashed yellow box in A shows the region of diffraction space integrated to produce the HOLZ line profiles in C.
First, we use dynamical diffraction simulation of a right-handed skyrmion and meron as a reference. For a right-handed skyrmion or meron, the diffracted intensity for (\(\bar{6}71\)) is stronger than that of (671) at this exact incident beam direction, while other pairs of reflections remain approximately symmetrical (Fig. 5C, black curve). With this in mind, we can determine the chirality of an individual skyrmion by comparing intensity variations of Bijvoet pairs in a 4D-STEM dataset. At 298 K, we carefully selected regions with minimal crystal mis-tilts and determined that both elongated and circular skyrmions are left-handed (Fig. S11). An ordered tetratic lattice appears upon heating to 373 K, as shown in the (771)/(\(\bar{7}71\)) intensity ratio map (Fig. 5B). In this region, two types of chiral structures are identified, labeled as #1 (red) and #2 (blue). HOLZ intensity profiles show that (\(\bar{6}71\)) is weaker than (671) from the diffraction pattern averaged over meron type #1, and the opposite case for meron type #2 (Fig. 5C, red and blue curves, respectively). Using dynamical diffraction simulations as reference (Fig. 5C, dashed gray curve), we can thus determine the chirality for meron type #1 as left-handed, and type #2 as right-handed. The alternating chirality of types #1 & #2 shown in Fig. 5B is consistent with our previous hypothesis of out-of-plane polarizations shown in Fig. 4F, labeled as green and blue vortices, respectively. More interestingly, the diffraction pattern averaged over a larger field of view (Fig. 5B, black box) shows symmetrical intensity, indicating the loss of chirality (Fig. 5C, black curve). Note that our observation does not violate the Poincaré-Hopf theorem16,52, where a periodic array of vortices and antivortices yields zero net vorticity, yet imposing no constraints on the chiralities in such topological textures.
Discussion
The transition from skyrmions to merons has been reported in chiral magnets with increasing in-plane magnetic anisotropy2,30,53. In 2D ferroelectrics, from the mesoscopic symmetry-breaking perspective, the observed isotropic-to-anisotropic structural transition upon heating was recently reported as an “inverse transition” of labyrinthine domains22. Such a transition was driven by the increase of thermal fluctuations of dipoles, which led to the domain reorientation or coalescence associated with the annihilation of merons and antimerons. In case of polar vortices, thermal fluctuations can lead to the melting of chiral crystals and even the loss of chirality54. On the other hand, in the case of polar skyrmions, the origin of the observed transition from skyrmions to a meron lattice can be attributed to changes in the elastic boundary conditions. The strain imposed on the [(PbTiO3)16/(SrTiO3)16]8 lifted-off membranes is caused by the thermal stress upon heating/cooling. In our experiment, the oxide membranes are ~4\(\times\) higher in thermal expansion coefficients than the SiNx TEM grid to which they are attached55,56. The oxide membranes are suspended over a 2 µm hole, the strain is applied from the edge of the hole due to thermal stress, and therefore small locally varying anisotropy within which gives small local bias to the texture orientation. As a consequence, the lifted-off membrane is under compressive strain at 373 K and tensile strain at 223 K, with additional local bending. In case of 4D-STEM experiments, we deliberately searched for flat regions to avoid artifacts in measuring Pip. A detailed 4D-STEM analysis of averaged in-plane lattice parameters (>80,000 diffraction patterns) shows that the lifted-off membrane is locally more rectangular at 223 K than at 373 K (Fig. S8). Experimentally, the lattice constant is strongly temperature dependent. To decouple the influence of strain versus purely thermal effects on the phase transition, phase-field simulations were carried out at controlled strain boundary conditions for the range of measured temperatures (Fig. S12). For a fixed in-plane lattice constant, simulation results for temperatures from 223–373 K showed a similar disordered skyrmion phase, indicating that here strain plays an important role in the skyrmion ordering. Furthermore, the change in anisotropy of in-plane lattice parameters, as seen in the 4D-STEM strain analysis (inset, Fig. S8D), is also consistent with FFT patterns for stripes and square lattice (Fig. 3A and C). Thus, we anticipate the occurrence of a long-range ordered meron lattice at room temperature in (PbTiO3)n/(SrTiO3)n superlattices grown on a compressive substrate such as LaAlO3.
In summary, we report the observation of a topological phase transition sequence in polar skyrmions, whereby tuning the temperature and strain boundary conditions, the anisotropic stripe phase deforms into an isotropic disordered circular phase, and finally transforms into an anisotropic ordered phase. This is the first observation of such transitions in a dipolar topological structure, in which the skyrmions deform in shape and a square lattice of merons appears, thereby preserving the topological charge of the system. The chiralities for each phase were also determined experimentally at nm-scale, for the first time. We hope that the microscopic observation of such a topological phase transition will stimulate further work to explore the macroscopic manifestation of the changes in topology with strain, which clearly is the critical external stimulus (in contrast to magnetic systems, where the Dzyalozhinskii-Moriya coupling plays a key role). Finally, our findings imply that such dipolar textures are a fertile ground for exploring new phases and topology, and with possible applications in nanoscale ferroelectric logic and storage devices.
Methods
Deposition of thin film superlattices and membrane lift-off
The epitaxial lift off sacrificial layer of 16 nm Sr2CaAl2O6 with a 2.4 nm SrTiO3 capping layer was synthesized on single-crystalline SrTiO3 (001) substrates via reflection high-energy electron diffraction (RHEED) – assisted pulsed laser deposition. Subsequent to this, n-SrTiO3/n-PbTiO3/n-SrTiO3 trilayers (n- is the number of monolayers; n = 16) and [(PbTiO3)16/(SrTiO3)16]8 superlattices were synthesized ex-situ on this template via RHEED-assisted pulsed-laser deposition (KrF laser). RHEED was used during the deposition to ensure the maintenance of a layer-by-layer growth mode for both the PbTiO3 and SrTiO3. (Sample Preparation using RHEED-assisted Pulsed-laser Deposition). The specular RHEED spot was used to monitor the RHEED oscillations. The heterostructure was first spin-coated with a polymer support of 500 nm thick polymethyl methacrylate (PMMA) film and placed in deionized water at room temperature until the sacrificial Sr2CaAl2O6 layer was fully dissolved. The PMMA coated film was then released from the substrate and transferred onto the TEM grid. Finally, the PMMA layer was dissolved and removed from the membrane in acetone. Additional details can be found in Membrane Lift-off and Transfer.
4D-STEM for Polarization and Chirality determination
We performed 4D-STEM experiments using an electron microscopy pixel array detector (EMPAD), where the 2D electron diffraction pattern was recorded over a 2D grid of real space probe positions, resulting in 4D datasets. Due to dynamical diffraction effects, the charge redistribution associated with polarization leads to the breakdown of Friedel’s law (4D-STEM for Polarization Mapping). From the collected CBED patterns, the polarization direction in the plan-view samples is reconstructed by calculating the center-of-mass in polarity sensitive Kikuchi bands along the cubic directions. We employ the Kikuchi bands as a more robust means to extract polarity information against internal crystal mis-tilts, which often occurs in ferroic oxides due to disinclination strain. Since chirality is a 3D property and TEM is a projection technique, we can retrieve this 3D information by utilizing the intensity asymmetries of chirality sensitive Bijvoet pairs in higher order Laue zone (HOLZ) reflections. Additional details for chirality determination are discussed in 4D-STEM for Chirality Determination.
Phase-field simulations
Detailed expressions of the energy terms, materials parameters as well as the numerical simulation procedure is described in ref(10). A periodic boundary condition is used along the in-plane dimensions, while a superposition method is applied in the out-of-plane direction. To determine the local strain state, the reference pseudocubic lattice constants for STO (PTO) at 223 K, 300 K and 373 K are set as 3.901 Å (3.953 Å), 3.905 Å (3.957 Å) and 3.909 Å (3.961 Å), respectively. The average effective substrate lattice constants are taken from experimental measurements, which are set as a = 3.875 Å, b = 3.885 Å for 223 K, a = b = 3.905 Å for 300 K and a = b = 3.899 Å for 373 K. The large compressive strains are due to local bending when the lift-off membrane is heated/cooled down. We believe that the dominant effect is the global thermal induced large compressive strain under heating, thus, for the simplicity of the model, we ignore the local strain inhomogeneity. A background dielectric constant of 40 is used. Random noise with a magnitude of 0.0001 C/m2 is added to the system as the initial polarization distribution of the system. Additional details of the simulation parameters and the resulting structures are discussed in Phase-Field Calculations.
Data availability
Experimental and simulation data (4D-STEM dataset and python script for analysis) are provided in the figures and Supplementary Information, and are publicly available at https://doi.org/10.5281/zenodo.7500030. Additional data are available from the corresponding authors upon request.
References
Yu, X. Z. et al. Real-space observation of a two-dimensional skyrmion crystal. Nature 465, 901–904 (2010).
Yu, X. Z. et al. Transformation between meron and skyrmion topological spin textures in a chiral magnet. Nature 564, 95–98 (2018).
Nagaosa, N. & Tokura, Y. Topological properties and dynamics of magnetic skyrmions. Nat. Nanotechnol. 8, 899–911 (2013).
Kosterlitz, J. M. & Thouless, D. J. Ordering, metastability and phase transitions in two-dimensional systems. J. Phys. C: Solid State Phys. 6, 1181 (1973).
Nelson, C. T. et al. Spontaneous vortex nanodomain arrays at ferroelectric heterointerfaces. Nano Lett. 11, 828–834 (2011).
Jia, C. L., Urban, K. W., Alexe, M., Hesse, D. & Vrejoiu, I. Direct observation of continuous electric dipole rotation in flux-closure domains in ferroelectric Pb(Zr,Ti)O-3. Science 331, 1420–1423 (2011).
Tang, Y. L. et al. Observation of a periodic array of flux-closure quadrants in strained ferroelectric PbTiO3 films. Science (80-.). 348, 547–551 (2015).
Hadjimichael, M. et al. Metal–ferroelectric supercrystals with periodically curved metallic layers. Nat. Mater. 2021 204 20, 495–502 (2021).
Naumov, I. I., Bellaiche, L. & Fu, H. Unusual phase transitions in ferroelectric nanodisks and nanorods. Nature 432, 737–740 (2004).
Yadav, A. K. et al. Observation of polar vortices in oxide superlattices. Nature 530, 198–201 (2016).
Shafer, P. et al. Emergent chirality in the electric polarization texture of titanate superlattices. Proc. Natl. Acad. Sci. USA 115, 915–920 (2018).
Tan, C. et al. Engineering polar vortex from topologically trivial domain architecture. Nat. Commun. 12, 1–8 (2021).
Du, K. et al. Manipulating topological transformations of polar structures through real-time observation of the dynamic polarization evolution. Nat. Commun. 2019 101 10, 1–8 (2019).
Susarla, S. et al. Atomic scale crystal field mapping of polar vortices in oxide superlattices. Nat. Commun. 12, 1–7 (2021).
Sun, Y. et al. Subunit cell–level measurement of polarization in an individual polar vortex. Sci. Adv. https://doi.org/10.1126/sciadv.aav4355 (2019).
Nahas, Y. et al. Discovery of stable skyrmionic state in ferroelectric nanocomposites. Nat. Commun. 6, 1–6 (2015).
Zhang, Q. et al. Nanoscale bubble domains and topological transitions in ultrathin ferroelectric films. Adv. Mater. 29, 1702375 (2017).
Hong, Z. & Chen, L. Q. Blowing polar skyrmion bubbles in oxide superlattices. Acta Mater. 152, 155–161 (2018).
Das, S. et al. Observation of room-temperature polar skyrmions. Nature 568, 368–372 (2019).
Gonçalves, M. A. P., Escorihuela-Sayalero, C., Garca-Fernández, P., Junquera, J. & Íñiguez, J. Theoretical guidelines to create and tune electric skyrmion bubbles. Sci. Adv. 5, eaau7023 (2019).
Das, S. et al. Local negative permittivity and topological phase transition in polar skyrmions. Nat. Mater. 20, 194–201 (2021).
Nahas, Y. et al. Inverse transition of labyrinthine domain patterns in ferroelectric thin films. Nature 577, 47–51 (2020).
Nahas, Y. et al. Topology and control of self-assembled domain patterns in low-dimensional ferroelectrics. Nat. Commun. 2020 111 11, 1–8 (2020).
Wang, Y. J. et al. Polar meron lattice in strained oxide ferroelectrics. Nat. Mater. 2020 198 19, 881–886 (2020).
Luk’yanchuk, I., Tikhonov, Y., Razumnaya, A. & Vinokur, V. M. Hopfions emerge in ferroelectrics. Nat. Commun. 2020 111 11, 1–7 (2020).
Zhao, H. J., Chen, P., Prosandeev, S., Artyukhin, S. & Bellaiche, L. Dzyaloshinskii–Moriya-like interaction in ferroelectrics and antiferroelectrics. Nat. Mater. https://doi.org/10.1038/s41563-020-00821-3 (2020).
Das, S. et al. A new era in ferroelectrics. APL Mater. 8, 120902 (2020).
Rusu, D. et al. Ferroelectric incommensurate spin crystals. Nature 602, 240–244 (2022).
Wojdeł, J. C. & Íñiguez, J. Ferroelectric transitions at ferroelectric domain walls found from first principles. Phys. Rev. Lett. 112, 247603 (2014).
Gao, N. et al. Creation and annihilation of topological meron pairs in in-plane magnetized films. Nat. Commun. 10, 5603 (2019).
Nelson, D. R. & Halperin, B. I. Dislocation-mediated melting in two dimensions. Phys. Rev. B 19, 2457–2484 (1979).
Halperin, B. I. & Nelson, D. R. Theory of Two-Dimensional melting. Phys. Rev. Lett. 41, 121–124 (1978).
Zijian Hong. Phase-Field Simulations of Topological Structures and Topological Phase Transitions in Ferroelectric Oxide Heterostructures (The Pennsylvania State University, 2017).
Lu, D. et al. Synthesis of freestanding single-crystal perovskite films and heterostructures by etching of sacrificial water-soluble layers. Nat. Mater. 15, 1255–1260 (2016).
Hong, S. S. et al. Extreme tensile strain states in La0.7Ca0.3MnO3 membranes. Science (80-.). 368, 71–76 (2020).
Pesquera, D. et al. Beyond substrates: strain engineering of ferroelectric membranes. Adv. Mater. 32, 2003780 (2020).
Tate, M. W. et al. High dynamic range pixel array detector for scanning transmission electron microscopy. Microsc. Microanal 22, 237–249 (2016).
Zuo, J.-M. & Shao, Y.-T. Scanning Convergent Beam Electron Diffraction (CBED), the essential questions of why, what and how? Microsc. Microanal. 24, 172–173 (2018).
Zuo, J. M. & Spence, J. C. H. Advanced Transmission Electron Microscopy. Advanced Transmission Electron Microscopy: Imaging and Diffraction in Nanoscience (Springer New York, 2017).
Ophus, C. Four-Dimensional Scanning Transmission Electron Microscopy (4D-STEM): from scanning nanodiffraction to ptychography and beyond. Microsc. Microanal. 25, 563–582 (2019).
Tsuda, K., Sano, R. & Tanaka, M. Nanoscale local structures of rhombohedral symmetry in the orthorhombic and tetragonal phases of BaTiO3 studied by convergent-beam electron diffraction. Phys. Rev. B 86, 214106 (2012).
Shao, Y.-T. & Zuo, J.-M. Nanoscale symmetry fluctuations in ferroelectric barium titanate, BaTiO3. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 73, 708–714 (2017).
Marthinsen, K. & Høier, R. On the breakdown of Friedel’s law in electron backscattering channelling patterns. Acta Crystallogr. Sect. A 44, 700–707 (1988).
Bird, D. M. & Wright, A. G. Phase dependence of Kikuchi patterns. I. Theory. Acta Crystallogr. Sect. A 45, 104–109 (1989).
Spence, J. C. H. On the accurate measurement of structure‐factor amplitudes and phases by electron diffraction. Acta Crystallogr. Sect. A 49, 231–260 (1993).
Deb, P. et al. Imaging polarity in two dimensional materials by breaking Friedel’s law. Ultramicroscopy 215, 113019 (2020).
Shao, Y.-T. & Zuo, J.-M. Lattice-rotation vortex at the charged monoclinic domain boundary in a relaxor ferroelectric crystal. Phys. Rev. Lett. 118, 157601 (2017).
MacLaren, I. et al. On the origin of differential phase contrast at a locally charged and globally charge-compensated domain boundary in a polar-ordered material. Ultramicroscopy 154, 57–63 (2015).
Goodman, P. & Johnson, A. W. S. Identification of enantiomorphically related space groups by electron diffraction—a second method. Acta Crystallogr. Sect. A 33, 997–1001 (1977).
Johnson, A. W. S. Chiral determination: Direct interpretation of convergent-beam electron diffraction patterns using the series expansion of Cowley and Moodie. Acta Crystallogr. Sect. B Struct. Sci. 63, 511–520 (2007).
Guo, Y., Nakashima, P. N. H. & Etheridge, J. Three-beam convergent-beam electron diffraction for measuring crystallographic phases. IUCrJ 5, 753–764 (2018).
Milnor, J. W. & John W. Topology From the Differentiable Viewpoint (Princeton University Press, 1997).
Lin, S.-Z., Saxena, A. & Batista, C. D. Skyrmion fractionalization and merons in chiral magnets with easy-plane anisotropy. Phys. Rev. B 91, 224407 (2015).
Gómez-Ortiz, F., García-Fernández, P., López, J. M. & Junquera, J. Melting of crystals of polarization vortices and chiral phase transitions in oxide superlattices. Phys. Rev. B 105, L220103 (2022).
Tien, C.-L. & Lin, T.-W. Thermal expansion coefficient and thermomechanical properties of SiNx thin films prepared by plasma-enhanced chemical vapor deposition. Appl. Opt. 51, 7229 (2012).
de Ligny, D. & Richet, P. High-temperature heat capacity and thermal expansion of SrTiO3 and SrZrO3 perovskites. Phys. Rev. B 53, 3013–3022 (1996).
Acknowledgements
The authors acknowledge fruitful discussions with Prof. Jian-Min Zuo, Prof. Kin Fai Mak, Dr. Shengwei Jiang, and Zui Tao. Funding was primarily provided by the Department of Defense, U.S. Army Research Office under the MURI ETHOS, via cooperative agreement W911NF-21-2-0162 (R.R., D.M., Y.-T.S.); and the U.S. Air Force Office of Scientific Research Hybrid Materials MURI, under award no. FA9550-18-1-0480 (H.Y.H.). The electron microscopy studies were performed at the Cornell Center for Materials Research, a National Science Foundation (NSF) Materials Research Science and Engineering Center (DMR-1719875). The Cornell FEI Titan Themis 300 was acquired through NSF-MRI-1429155, with additional support from Cornell University, the Weill Institute and the Kavli Institute at Cornell. The authors thank M. Thomas, J. G. Grazul, M. Silvestry Ramos, K. Spoth for technical support and careful maintenance of the instruments. The materials synthesis work is supported by the Quantum Materials program from the Office of Basic Energy Sciences, US Department of Energy (DE-AC02-05CH11231). The membrane lift-off techniques were developed with support from US Department of Energy, Office of Basic Energy Sciences, Division of Materials Sciences and Engineering, under contract number DE-AC02-76SF00515. The phase-field simulation work is supported as part of the Computational Materials Sciences Program funded by the U.S. Department of Energy, Office of Science, Basic Energy Sciences, under Award No. DE-SC0020145. F.G.-O., P.G.-F., and J.J. acknowledge financial support from Grant No. PGC2018-096955-B-C41 funded by MCIN/AEI/10.13039/501100011033 and by ERDF “A way of making Europe,” by the European Union. F.G.-O. acknowledges financial support from Grant No. FPU18/04661 funded by MCIN/AEI/10.13039/501100011033. S.D. acknowledges Science and Engineering Research Board (SRG/2022/000058) and Indian Institute of Science start up grant for financial support. L.W.M. acknowledges support from the U.S. Department of Energy, Office of Science, Office of Basic Energy Sciences, under Award Number DE-SC-0012375 for the development and study of ferroic heterostructures.
Author information
Authors and Affiliations
Contributions
Y.-T.S., S.D., R.X., H.Y.H., R.R., and D.M. designed research; Y.-T.S., S.D., Z.H., R.X., S.C., F.G.-O., and P.G.-F., and J.J. performed research; Y.-T.S., S.D., and R.X. contributed new reagents/analytic tools; Y.-T.S. supervised by D.M., S.D., Z.H., F.G.-O., P.G.-F., L.-Q.C., H.Y.H., J.J., L.W.M., and R.R. analyzed data; and Y.-T.S. and D.M. wrote the paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Peer review
Peer review information
Nature Communications thanks Haifeng Du and the other anonymous reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Additional information
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Shao, YT., Das, S., Hong, Z. et al. Emergent chirality in a polar meron to skyrmion phase transition. Nat Commun 14, 1355 (2023). https://doi.org/10.1038/s41467-023-36950-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-023-36950-x
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.