On-chip generation and dynamic piezo-optomechanical rotation of single photons

Abstract

Integrated photonic circuits are key components for photonic quantum technologies and for the implementation of chip-based quantum devices. Future applications demand flexible architectures to overcome common limitations of many current devices, for instance the lack of tuneabilty or built-in quantum light sources. Here, we report on a dynamically reconfigurable integrated photonic circuit comprising integrated quantum dots (QDs), a Mach-Zehnder interferometer (MZI) and surface acoustic wave (SAW) transducers directly fabricated on a monolithic semiconductor platform. We demonstrate on-chip single photon generation by the QD and its sub-nanosecond dynamic on-chip control. Two independently applied SAWs piezo-optomechanically rotate the single photon in the MZI or spectrally modulate the QD emission wavelength. In the MZI, SAWs imprint a time-dependent optical phase and modulate the qubit rotation to the output superposition state. This enables dynamic single photon routing with frequencies exceeding one gigahertz. Finally, the combination of the dynamic single photon control and spectral tuning of the QD realizes wavelength multiplexing of the input photon state and demultiplexing it at the output. Our approach is scalable to multi-component integrated quantum photonic circuits and is compatible with hybrid photonic architectures and other key components for instance photonic resonators or on-chip detectors.

Introduction

Photonic quantum technologies^{1,2,3,4,5,6,7} have seen rapid progress and hallmark quantum protocols have been implemented using integrated quantum photonic circuits (IQPCs)^{8,9,10,11,12,13}. For most applications, the on-chip generation of single photons is highly desirable to avoid the inevitable coupling losses when using an off-chip source. For this purpose, quantum emitters¹⁴, for instance semiconductor quantum dots (QDs)^15,16 or defect centers^{17,18,19,20,21,22}, are excellent candidate systems. Among these systems, QDs made from (In,Ga,Al)As semiconductor compounds offer several advantages^{23,24,25,26,27}: they are extremely bright sources of single photons^28,29 and entangled photon pairs^30,31 which can be elegantly included in the photonic structure during epitaxial growth of a heterostructure³². These heterostructures are then ready for monolithic fabrication of IQPCs using advanced cleanroom technology^{33,34,35,36,37}. In contrast to monolithic approaches, heterogeneous integration of these QDs and other types of quantum emitters on IQPCs made on material platforms with complementary strengths promise superior performance¹². Such hybrid devices have been reported on silicon (Si)^38,39, silicon nitride (SiN)^{40,41,42,43,44}, aluminum nitride (AlN)⁴⁵ or lithium niobate (LiNbO₃)⁴⁶ IQPCs. However, their fabrication is natively connected to a significant increase of the complexity compared to monolithic routes. Furthermore, the on-chip control of light propagation in photonic elements is crucial. To this end, for instance thermo-optic^47,48,49, electro-optic^50,51,52 or acousto-optic^53,54,55,56 effects or nanomechanical actuation^57,58 have proven to be viable routes. Among these mechanisms, acoustic phonons are an attractive choice because they couple to literally any system⁵⁹ enabling strong optomechanical modulation and dynamic reconfiguration of quantum emitters^60,61,62. In the form of radio frequency Rayleigh surface acoustic waves (SAWs)⁶³ or Lamb waves⁶⁴, phonons can be routed on-chip^65,66,67 and interfaced with integrated photonic elements^{53,54,55,56,68,69}, quantum emitters^{70,71,72,73,74,75} or even superconducting quantum devices^76,77. The electrical generation of SAWs and their ultralow dissipation offers distinct advantages over local tuning schemes. Thermo-optic, electro-optic or Stark-effect tuning require local electrodes to generate heat or electric fields for each element. SAWs, in contrast however can be piezo-electrically generated by applying a rf voltage to an interdigital transducer (IDT) and the propagating SAW beam modulates any IQPC element or QD in its propagation path. The underlying mechanical tuning mechanism does not induce inherent losses, in contrast to the well-known Franz-Keldysh electroabsorption in electro-optic devices⁷⁸. These unique properties together with the ability to synchronize SAWs and optical pulses^79,80 pave the way towards parallelized control in large scale IQPC networks.

In this work, we report on a piezo-optomechanically reconfigurable quantum photonic device with integrated tuneable QDs, schematically shown in Fig. 1. The validation of fully fledged dynamic single photon routing, single qubit logic and single photon wavelength (de)multiplexing is illustrated in Fig. 1b-d. First, we excite a QD, which emits a single photon into an integrated and dynamically tuneable MZI. Second, we employ a SAW with a frequency of f_SAW ≈ 525 MHz to dynamically route the single photons between the outputs by tuning the time-dependent phase gate which creates a superposition state with a visibility of >0.75 at the operation frequency. Third, dynamic spectral modulation of the QD phase-locked to the tuneable phase gate enables freely programmable spectral multiplexing of single photons. Our demonstrated operation bandwidth of >1 GHz for single photon routing exceeds that reported for state-of-the-art monolithic devices employing electro-optic⁵² and nanomechanical⁵⁸ tuning by more than a factor 100. Also, our achieved spectral tuning range of the integrated QD of ≥ 0.8 nm is competitive with Stark-effect tuning on this platform⁸¹. The achieved >1 GHz operation nests our system well in the resolved sideband regime enabling on-chip parametric quantum phase modulation of the QD^74,82,83 and the routed single photons⁸⁴.

Fig. 1: Device and qubit rotation.

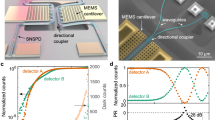
a Schematic representation of the dynamically tuneable ridge waveguide integrated quantum photonic circuit (IQPC). The concept, based on a Mach Zehnder Interferometer (MZI) comprises two input waveguides (Input WG-A and Input WG-B) connected to a 2 × 2 multimode interference device (MMI1) whose outputs are connected by two WGs to MMI2 with two output WGs (Output WG-A and Output WG-B). Two interdigital transducers (IDTs) generate SAWs for spectral modulation of single QDs (IDT_SM) in the Input WGs and optomechanical phase modulation of the MZI (IDT_PM). b Selective single photon generation by a single QD in the input WGs. c Single photon rotations shown on Bloch spheres. The evolution of the states is indicated by the green arrows (Beamsplitter gates by MMI1 & MMI2, respectively) and a red arrow (SAW-driven dynamic phase gate). d projection measurement of the rotated superposition state by collection and detection from the Output WGs (Output WG-A / Output WG-B) using a lensed optical fiber.

Results

Device layout

Our device is based on a waveguide IQPC monolithically fabricated on a GaAs semiconductor platform^24,26,34. The piezoelectricity of this class of materials enables the direct all-electrical excitation of SAWs using IDTs⁸⁵. The SAW tunes the integrated MZI via a time-modulated photoelastic effect and switches photons between the two outputs. Importantly, the heterostructure contains (In,Ga)As QDs, one of the most mature semiconductor quantum emitter system²⁵. These QDs are established as high quality sources of single photons with high indistinguishability^28,29 and their potential has been unambiguously proven by the implementation of fundamental quantum protocols^86,87,88,89.

Our device, schematically shown in Fig. 1a, comprises key functionalities required of an IQPC: it contains integrated, tuneable quantum emitters for in-situ wavelength multiplexed single photon generation. A combination of two static and one programmable elementary single qubit gates rotates the qubit and controls its output state which is detected in a projection measurement. The IQPC itself is based on etched GaAs ridge waveguides (WGs) on an (Al,Ga)As cladding layer. During crystal growth of the semiconductor heterostructure, a single layer of (In,Ga)As QDs is embedded in the active region to provide high-quality built-in, anti-bunched quantum emitters. The photonic circuit consists of two symmetric WGs, referred to as WG-A and WG-B, and two 4-port multimode interference (2 × 2 MMI) beamsplitters. To achieve fully fledged tuneability, our device is equipped with IDTs to generate two SAW beams with the same frequency of f_SAW ≈ 525 MHz.

As explained above, the QDs emit single photons in the input waveguides WG-A and WG-B. Since the QDs are in different WGs, our single photon generation scheme does not strictly correspond to a fully fledged initialization of a photonic qubit. Nevertheless, we apply the corresponding terminology in the following because the state control scheme is equivalent to a qubit rotation. A common representation of a photonic qubit is the so-called rail encoding which can be applied for our IQPC. Here, the qubit states are defined as $|{1}_{A},{0}_{B}\rangle=\left(\begin{array}{c}1\\ 0\end{array}\right)\equiv|0\rangle$ and $|{0}_{A},{1}_{B}\rangle=\left(\begin{array}{c}0\\ 1\end{array}\right)\equiv|1\rangle$, with indices A,B denoting WG-A and WG-B, respectively²⁶. Moreover, we point out that the embedded QDs are dynamically tuneable. They can be strained by a SAW generated by IDT_SM and the time-dependent local phase of the SAW φ_SAW,SM(t) = 2πf_SAW,SMt programs the emission wavelength of the QD emitted single photons λ(t) = λ₀ + Δλ · sin 2πf_SAW,SMt used to encode the input⁶¹.

Employing the terminology of rail-encoded photonic qubits, its state vector is controllably rotated on the Bloch sphere in the IQPC as follows. The two input WGs are connected to the first MMI beamsplitter, labeled MMI1, which executes an Hadamard $H=\frac{1}{\sqrt{2}}\left(\begin{array}{cc}1 & 1\\ 1 & -1\end{array}\right)$ and a Pauli-Z, $Z=\left(\begin{array}{cc}1 & 0\\ 0 & -1\end{array}\right)$, gate operation on the input state. The full beamsplitter gate operation caused by a single MMI can be expressed as

$${{{{{\rm{MMI}}}}}}=Z\cdot H=\frac{1}{\sqrt{2}}\left(\begin{array}{cc}1 & 1\\ -1 & 1\end{array}\right)$$

(1)

Thus, MMI1 creates a superposition state from the input photonic qubit, $|0\rangle \to \frac{1}{\sqrt{2}}(|0\rangle -|1\rangle )\equiv|-\rangle$ and $|1\rangle \to \frac{1}{\sqrt{2}}(|0\rangle+|1\rangle )\equiv |+\rangle$, which propagates to the second MMI beamsplitter, MMI2. MMI2 rotates these states |−〉 and |+〉 to output states |1〉 and |0〉, respectively. Using Eq. (1), the full rotation of the static IQPC can be described by ${{{{{{\rm{IQPC}}}}}}}_{{{{{{\rm{static}}}}}}}={{{{{\rm{MMI}}}}}}\cdot {{{{{\rm{MMI}}}}}}=\left(\begin{array}{cc}0 & 1\\ -1 & 0\end{array}\right)=Z\cdot X$, which corresponds to the combination of a Pauli-X gate (NOT-gate) and a Pauli-Z gate. Since the Pauli-Z gate only affects the phase of the qubit, but not the projection of the qubit on the base states |0〉 and |1〉, and the qubit is measured after exiting the IQPC, the Z-gate does not influence the outcome of the experiments presented in this work.

The SAW generated by IDT_PM optomechanically modulates the optical phase difference between the WGs connecting the two MMIs^54,90. For this operation, the two arms (length 140 μm) of the MZI connecting the MMIs are separated by 1.5 Λ_SAW,PM, with Λ_SAW,PM = 5.6 μm, being the acoustic wavelength of the applied SAW. This geometric separation ensures that the optical phase modulations in the two arms are antiphased, which can be expressed as ϕ_A,B(t) = ∓∆ϕ sin(φ_SAW,PM(t)), with – for WG-A and + for WG-B. Here, φ_SAW,PM(t) = 2πf_SAW,PMt is the dynamic phase of the SAW. Δϕ is the amplitude of the optical phase modulation, which is given by the strength of the underlying acousto-optic interaction⁵⁴. Most importantly, Δϕ can be tuned by the electrical radio frequency (rf) power of the electrical signal P_rf,PM, applied to the IDT. Thus, the total optical phase shift amounts to

$$\phi \left(t\right)=2\cdot \triangle \phi {{\sin }}\,\left(2\pi {f}_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}}t\right)+{\phi }_{0}$$

(2)

In this expression, ϕ₀ is a finite optical phase offset introduced by imperfections during nanofabrication. We obtain as the full SAW-driven dynamic phase gate operation

$${R}_{\phi }\left(t\right)=\left(\begin{array}{cc}1 & 0\\ 0 & {{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi \left(t\right)}\end{array}\right)$$

(3)

After this phase gate operation, MMI2 executes the second beamsplitter gate operation whose output now depends on R_ϕ(t).

Most notably, this gate operation allows us to program the output state of the qubit

$$|\varPsi \rangle=\alpha ({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}})|0\rangle+\beta ({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}})|1\rangle$$

(4)

α(φ_SAW,PM) and β(φ_SAW,PM) are SAW-programmable complex amplitudes obeying |α|² + |β|² = 1. Figure 1c exemplifies the respective rotations on the Bloch sphere starting from the initial |0〉 qubit state propagating through the modulated device. First, MMI1 rotates the input state into the xy-plane of the Bloch sphere to the |–〉 state. Second, the SAW-programmable optical phase shift ϕ(φ_SAW,PM), rotates the Bloch vector in the equatorial plane (xy-plane) and third, MMI2 rotates the Bloch vector from the xy- to the yz-plane.

Combining Eq. (1) and Eq. (3), we obtain for the full operation executed by our dynamic IQPC

$${{{{{\rm{IQPC}}}}}}\left(\phi \right)={{{{{\rm{MMI}}}}}}\cdot {R}_{\phi }\left(t\right)\cdot {{{{{\rm{MMI}}}}}}=\frac{1}{2}\left(\begin{array}{cc}1-{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi } & 1+{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\\ -1-{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi } & -1+{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\end{array}\right)$$

(5)

This full gate operations yield the following states for a single photon created at the input WG-A and WG-B:

$$|{1}_{A},{0}_{B}\rangle \equiv|0\rangle \to \frac{1}{2}\left(\begin{array}{c}1-{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\\ -1-{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\end{array}\right)$$

(6)

$$|{0}_{A},{1}_{B}\rangle \equiv|1\rangle \to \frac{1}{2}\left(\begin{array}{c}1+{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\\ -1+{{{{{{\rm{e}}}}}}}^{{{{{{\rm{i}}}}}}\phi }\end{array}\right)$$

(7)

at the Output WG-A and Output WG-B, respectively.

The corresponding probability amplitudes |α|² and |β|² are obtained via a projection measurement by collecting the output signals of the two waveguides with a lensed fiber.

Note, that truly arbitrary output states can be created simply by adding a second SAW-modulator after MMI2 which can be actively phase-locked to the first SAW-modulator. This straightforward extension creates a dynamic and fully programmable single qubit rotator, an elemental building block of photonic quantum processors^2,91.

Devices were fabricated monolithically on a semiconductor heterostructure grown by molecular beam epitaxy. Full details on the semiconductor heterostructure, the experimental setup and device fabrication are included in the Methods Section and Supplementary Notes 1 and 2. The optical and SAW-properties of the as-fabricated devices were as follows: the optical waveguide losses of (10.8 ± 2.50)dB⋅cm⁻¹ of the fabricated IQPCs are competitive with the state of the art in this material system^34,35. The insertion loss of the delay line is S₂₁ = 28 dB at low temperatures. The full electromechanical conversion efficiency including losses at the cryostat wiring is 4%, proving efficient generation of the SAW on the comparably weak piezoelectric GaAs. Full details are included in Supplementary Notes 3.1 and 3.2. In the experiments presented in the remainder of the paper, SAWs are generated by a continuous wave (cw) rf signal(s) applied to one or two IDTs. We then assess the φ_SAW-dependence using a cw laser to photoexcite the QD and initialize the input qubit by the subsequentially emitted single photon. The output signals collected by the lensed fiber are analyzed in the time domain to obtain the φ_SAW-dependence. This scheme allows us to elegantly detect the dynamic modulation by the SAW. In our proof-of-principle study, the cw applied rf power leads to unwanted sample heating. The latter can be significantly suppressed by using a pulsed SAW excitation scheme⁶¹ or in hybrid devices comprising a strong piezoelectrics e.g. LiNbO₃ with heterointegrated semiconductor QDs⁹². Full details on the experimental setup are presented in the “Methods” section and in Supplementary Note 2.

Tuneable single photon routing and state rotation

We continue with the characterization of the dynamic modulation of the quantum interference in the MZI with a SAW generated by IDT_PM. The operation principle relies on the local modulation of the refractive index in the two MZI WGs and depends on the local phase of the SAW, φ_SAW,PM. The change in refractive index causes an optical phase shift ϕ(φ_SAW,PM) between WG-A and WG-B and leads to a dynamic modulation of the interference at MMI2 and, thus, the superposition state of the output photonic qubit, given by Eq. (4)–Eq. (7). Figure 2a shows the schematic of the measurement configuration. A single QD is optically excited in Input WG-A to prepare a |0〉-like state of the photonic qubit at Input WG-A and WG-B.

Fig. 2: Dynamic routing of quantum dot emission.

a Schematic representation of the experimental configuration: although the optical transition of a single QD in Input WG-A is not modulated (IDT_SM is inactive), the response of the MZI is modulated (IDT_PM is active) as the photons pass through. Emission is collected by a lensed fiber from either Output WG-A (red) or Output WG-B (black). b Phase-integrated emission spectra of the same single QD measured without a SAW (top panels, IDT_PM off) and an applied SAW (P_rf,PM = 23 dBm, lower panels). c Measured SAW phase-dependent intensity of the main QD emission line (λ₀ in b) detected via Output WG-A (red symbols) and Output WG-B (black symbols) for different P_rf,PM as defined by Eq. (6) and Eq. (7). The simulation results (solid lines) for phase modulation amplitudes Δϕ = 0.3, 0.5, 0.8 and 1.3 rad are also shown for both outputs. The upper panel shows the time-resolved SAW-induced optical phase shift ϕ_A/B in the upper (WG-B, solid line) and lower arms (WG-A, dashed line) of the MZI. The right-hand side panel shows the 〈S_Z〉-projection of the qubit for the measurement and the corresponding simulation given by Eq. (8) The scale bar in the upper right panel shows the 300 ps timing jitter of the used single photon detector. d–f Rotations of the qubit states in the equatorial plane of the Bloch sphere at three distinct phases during the acoustic cycle given by Eq. (2) and Eq. (3). Symbols mark these phases in the projection data in (c).

First, we compare the phase-integrated photon output characteristics for the as-fabricated passive and active device in Fig. 2b. As confirmed in the upper panel, the device cross-couples photons of the excited QD from Input WG-A to Output WG-B (|β(φ_SAW,PM)|², right, black) and almost no emission is detected from Output WG-A (|α(φ_SAW,PM)|², left, red), as expected from Eq. (6) and Eq. (7) using ϕ = 0. This result corresponds precisely to the inversion of the input photonic qubit |0〉 → |1〉 with a high static fidelity or switching contrast of 0.94 ± 0.01. The small deviation arises from the fabrication-related imperfections’ static phase ϕ₀ = 0.51 rad. Supplementary Note 3.3 contains a full set of simulation data.

When an acoustic power of P_rf,PM = 23 dBm and a frequency of f_SAW,PM = 525 MHz is applied to IDT_PM (lower panels), the signal is routed dynamically between both outputs. Thus, the phase-integrated spectra detected from Output WG-A (lower left panel, red) and Output WG-B (lower right panel, black) are almost perfectly identical. Note the spectra in the lower panels are shifted to longer wavelengths accompanied by a small reduction of the total emission intensity due to an increase of the temperature when the radio frequency signal is applied⁹³. This is described in detail in Supplementary Note 3.6. For clarity, corresponding emission lines in the spectra are marked by λ₀. Importantly, no additional broadening is observed. Thus, no unwanted spectral modulation occurs when the quantum interference of the photonic qubit is dynamically controlled by R_ϕ(φ_SAW,PM).

Second, we study the modulation of the qubit rotation induced by the R_ϕ(φ_SAW,PM)-gate as a function of φ_SAW,PM. The upper panel in Fig. 2c shows the time and phase dependence of the antiphased optical phase modulations, of ϕ_A,B, in WG-A (dashed line) and WG-B (solid lines) of the MZI, respectively. In the left panels below, we analyze the intensity of the dominant QD emission line λ₀ = 874 nm collected from Output WG-A (red, |α(φ_SAW,PM)|²) and Output WG-B (black, |β(φ_SAW,PM)|²) as a function of the acoustic phase, φ_SAW,PM, with the applied rf power increasing from top to bottom. At the lowest drive power P_rf,PM = 9 dBm the modulation of the refractive index and thus Δϕ is weak. Even at this low power level however, the signals detected from the two outputs already exhibit the expected antiphased modulation. This indicates that the pure |1〉-output state of the unmodulated MZI is dynamically adopting |0〉-character due to the oscillation of ϕ(φ_SAW,PM). When P_rf,PM increases, the modulation amplitude of the optical phase, Δϕ, increases further and the resulting interference in the modulated MZI develops a pronounced oscillation. To better visualize the dynamic nature of the qubit rotation by the SAW, we extract the Z-projection of the qubit,

$$\left\langle {S}_{Z}\right\rangle=\frac{{\left|\alpha \left({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}}\right)\right|}^{2}-{\left|\beta \left({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}}\right)\right|}^{2}}{{\left|\alpha \left({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}}\right)\right|}^{2}+{\left|\beta \left({\varphi }_{{{{{{\rm{SAW}}}}}},{{{{{\rm{PM}}}}}}}\right)\right|}^{2}}$$

(8)

This figure of merit is plotted in the right panels. For low P_rf,PM, the qubit is predominantly in the |1〉 state, i.e 〈S_Z〉 = −1. For the highest P_rf,PM = 23 dBm, the Bloch vector rotates between 〈S_Z〉 = −0.71 and 〈S_Z〉 = +0.82. From these experimental values we obtain the switching contrast of 0.75, which is competitive with electro-optic modulation in monolithic GaAs IQPCs with embedded QDs⁵². This value is a lower bound since the timing jitter of our detector of 300 ps (scale bar in Fig. 2c) limits the time resolution at the high modulation frequency 2f_SAW,PM = 1.05 GHz of the data. A realistic value may range between this resolution limited value of 0.75 and the near-unity predicted by our simulation. Importantly, it is rotated into the equatorial plane (〈S_Z〉 = 0) at well-defined phases during the acoustic cycle. The non-zero static phase, ϕ₀, gives rise to the observed device-characteristic beating of the dynamic qubit rotation while for a perfectly symmetric MZI (ϕ₀ = 0) the frequency of this oscillation is given by 2f_SAW,PM = 1.05 GHz. Note, that the non-zero ϕ₀ does not represent a limitation of our concept because it can be adjusted after calibration if deemed necessary. A rigorous analytical model of this beating is detailed in Supplementary Note 3.4 together with the respective theoretical optical field intensity propagations.

Third, we validate our experimental findings by performing beam propagation method simulations considering ϕ(φ_SAW,PM) in the MZI. Phase-dependent simulation results are indicated by the solid lines in Fig. 2c. They show that the experimental data can be nicely reproduced for ∆ϕ ranging between Δϕ = 0.3 rad and 1.3 rad and near-unity |〈S_Z〉| can be predicted from the projection of the strongest modulation. Figure 2d–f then shows the rotation of the qubit states in the equatorial plane of the Bloch sphere at three distinct acoustic phases, φ_SAW,PM = −0.06π, 0.14π and 0.5π, which are accordingly marked in the projection measurement data in Fig. 2c. Clearly, the data presented in Fig. 2 validate that the applied SAW induces a dynamic R_ϕ(φ_SAW,PM)-gate and such driven qubit rotations enable the faithful generation of a superposition state in the equatorial plane of the Bloch sphere. Since these rotations are gated with f_SAW,PM = 525 MHz, the data prove dynamic routing of the on-chip generated single photons on sub-nanosecond timescales, which are challenging to reach in alternative electro-optic or nanoelectromechanical approaches^57,58.

Conservation of single photon character

As the next step of our proof-of-principle study, we now prove that the single photon nature of the qubit is conserved. We measure the second-order correlation function g⁽²⁾(τ) using a standard Hanbury-Brown and Twiss setup and Supplementary Note 4 shows data of a reference QD with no modulation applied. These anti-bunching data in Supplementary Fig. 13 yield g⁽²⁾(0) = 0.48 ± 0.03 < 0.5. The observed values of g⁽²⁾ (0) in our experiments are limited by the finite time resolution of our detectors and the non-resonant, above bandgap optical pumping and not by the SAW modulation and there exists no fundamental limitations^74,93,94 to reach the g⁽²⁾ (0) record low levels reported for this platform^34,35. Importantly, in the context of this paper, g⁽²⁾ (0) > 0.5 enables us to conduct proof-of-principle experiments and assess the impact of SAW modulation of our IQPC on the stream of single photons. In Fig. 3, we compare g⁽²⁾ (τ) of two different QDs with a frequency f_SAW,PM = 525 MHz SAW applied to IDT_PM at a power level of P_rf,PM = 17 dBm. As shown by the schematics in the upper panels, the two QDs located in Input WG-A (Fig. 3a) and Input WG-B (Fig. 3b), initialize |0〉 and |1〉 input photonic qubit states, respectively. The time-dependent qubit rotations of both QDs are presented in Supplementary Note 4.2. Correlations are measured from Output WG-B, i.e. the time-dependent |β(φ_SAW,PM)|² projection of the qubit on its |1〉 component. Data are fitted using a procedure provided in Supplementary Note 4.3. For the QD in Fig. 3a, the emitted single photon is routed to Output WG-B, i.e. |0〉 → |1〉 in the static case. At the applied power level, the modulation of the output intensities is weak [c.f. Fig. 2c]. Thus, g² (τ) of this QD is expected to be like that of the unmodulated case with a weak f_SAW,PM⁻¹ = 1.9 ns-periodic modulation superimposed. The measured g⁽²⁾ (τ) (blue line) shows precisely the expected anti-bunching behavior and f_SAW,PM is clearly resolved in the fast Fourier Transform (FFT) of the data shown as an inset. From a best fit (red line) to the data considering the timing resolution of our detectors, we obtain g⁽²⁾ (0) = 0.38 ± 0.06. For the QD in Fig. 3b, the emitted single photon is routed to Output WG-A, i.e |1〉 → |0〉 in the static case. At the selected SAW amplitude [c.f. Figure 2c], the time-dependent |β(φ_SAW,PM)|² projection measured from Output WG-B is non-zero for short time intervals. Thus, the measured g² (τ) exhibits a clear f_SAW,PM⁻¹ = 1.9 ns-periodic modulation confirmed by the FFT of the data shown as an inset. Most importantly, the data (blue line) exhibit a clear suppression of coincidences at τ = 0, with g⁽²⁾ (0) = 0.42 ± 0.1 obtained from a best fit (red line). Both g⁽²⁾ (0) values agree well with that of the unmodulated QD shown in Supplementary Note 4.1. Our proof-of-principle experiments unambiguously show that the antibunched single photon nature of the transmitted light and thus photonic qubit is preserved with the dynamic R_ϕ (φ_SAW,PM)-gate applied, which is a key requirement for practical applications.

Fig. 3: Photon antibunching of on-chip routed quantum dot emission.

Upper panels show a schematic representation of the experimental configuration: the optical transition of a single QD in Input WG-A (a, red) or Input WG-B (b, black) is not modulated (IDT_SM is inactive) while the response of the MZI is modulated (IDT_PM is active) as the photons passes. Emission is collected from Output WG-B in both cases. Main panels show the measured second order correlation function g⁽²⁾ (τ) (blue) for the QD in Input WG-A (a) and Input WG-B (b) for P_SAW,PM = 17 dBm and f_SAW,PM = 525 MHz. The red lines are best fits to the data yielding: g⁽²⁾ (0) = 0.38 ± 0.06 and g⁽²⁾ (0) = 0.42 ± 0.09 in (a) and (b), respectively. Insets show the fast Fourier transform (FFT) of the data with a clear signal at f_SAW,PM.

Dynamic wavelength-selective single photon multiplexing

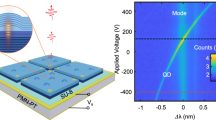
Finally, we apply spectral modulation to the QD. This enables us to implement a proof-of-principle multiplexing and demultiplexing of single photons. To this end, we simultaneously modulate the spectral emission characteristics of the QD using IDT_SM and dynamically control the qubit in the MZI using IDT_PM. A schematic of the experimental configuration is shown in Fig. 4a. We study a QD located in Input WG-B initializing a |1〉-input qubit and read-out is performed at Output WG-B (|β|²). Both SAWs are active as we set f_SAW = f_SAW,SM = f_SAW,PM = 524.12 MHz and P_rf,SM = P_rf,PM = 19 dBm. Importantly, we can program the relative phase Δφ_SAW = φ_SAW,SM − φ_SAW,PM between the SAWs driving the spectral and phase modulations, simply by setting the phases of the driving electrical signals.

Fig. 4: Single photon (de)multiplexing.

a Schematic representation of the experimental configuration: Both IDTs are active. The optical transition of a single QD in Input WG-B is modulated by IDT_SM while the response of the MZI is modulated by IDT_PM as photons pass through. b Upper panel: Acoustic phase-dependent spectral modulation of the QD. Lower panel: intensity modulations at Output WG-B at four distinct relative phases Δφ_SAW. c–f Acoustic phase-dependent emission spectra of the QD measured from Output WG-B for the four distinct relative phases marked in (b). g Programmable spectral demultiplexing of the maximum (λ_max, red symbols), center (λ₀, black symbols) and minimum (λ_min, blue symbols) of the spectral modulation as a function of Δφ_SAW. Colored lines are best fits to the data.

To start, we confirm the spectral modulation of the quantum emitter. The measured phase-dependent emission spectra of the QD are shown in false-color representation as a function acoustic phase, φ_SAW,SM, and optical wavelength in the upper panel of Fig. 4b. The upper axis is in units of time during the SAW-cycle. The emission exhibits the expected sinusoidal modulation λ(t) = λ₀ + Δλ⋅sin 2πf_SAWt due to the SAW-induced modulation through the deformation potential coupling⁹⁵. For our devices we find a total tuning bandwidth of 2Δλ = 0.8 nm (cf. Supplementary Fig. 10) comparable to recent reports of static Stark tuning on this platform⁸¹. A detailed analysis is included in Supplementary Note 3.5. In the experiments presented in the following, we set Δλ = 0.05 nm. The data clearly proves that a single photon is emitted at a well-defined wavelength at a given phase during the acoustic cycle. Since the QD is optically pumped by a continuous wave laser, all wavelengths are injected into the MZI and are thus, multiplexed at the respective phases. This spectral oscillation sets the reference for the MZI modulation driven by the SAW generated by IDT_PM. Next, the lower panel of Fig. 4b shows the dynamic single photon routing (QD in Input WG-B, i.e. |1〉 initialization of the qubit) as a function of phase from Output WG-B (|β(φ_SAW,SM)|²) for four different Δφ_SAW = 0, π/2, π and 3π/2. The vertical lines connect to the emission wavelengths of the QD when the dynamically tuned quantum interference projects the input |1〉-state to the output |1〉-state. Clearly, Δφ_SAW programs the projected wavelength: the center wavelength (λ₀ = 876.35 nm) is detected from Output WG-B for Δφ_SAW = 0 (purple) and Δφ_SAW = π(orange), while for Δφ_SAW = 0.5π (green), and for Δφ_SAW = 1.5π (blue), the maximum (λ_max = 876.4 nm) and minimum wavelength (λ_min = 876.3 nm) leave via this output, respectively. This dynamic, single photon wavelength de-multiplexing is demonstrated in phase resolved experiments. In Fig. 4c-f, we show the phase-dependent emission of the QD. The symbols mark the above selected Δφ_SAW. First, we observe that emission is detected only at distinct phases of the acoustic cycle. This confirms that the SAW-modulated MZI is operated as a tuneable, dynamic signal router. Second, as Δφ_SAW is tuned, the wavelength of the photons exiting via Output WG-B changes. At Δφ_SAW = 0 (Fig. 4c) and Δφ_SAW = π (Fig. 4e) the QD emission is coupled out during the rising and falling edge of the spectral modulation, respectively. At Δφ_SAW = 0.5π (Fig. 4d), λ_max is filtered, perfectly antiphased to the detection of λ_min at Δφ_SAW = 1.5π (Fig. 4f). Lastly, we further corroborate the faithful demultiplexing in Fig. 4g. We plot the intensities at λ_max (red), λ_min (blue) and λ₀ (black) over two complete cycles of Δφ_SAW. These data unambiguously confirm that λ_max and λ_min are filtered at well-defined Δφ_SAW. Furthermore, the center wavelength (λ₀) oscillates at 2f_SAW because the applied demultiplexing filters this wavelength at both the rising and falling edges of the spectral modulation.

Discussion

In conclusion, we designed, monolithically fabricated, and demonstrated proof-of-principle operation of key functionalities important for hybrid photonic and phononic quantum technologies. We used integrated quantum emitters to generate single photons in an IQPC with acoustically tuneable wavelength. We executed dynamic and tuneable rotations in a compact SAW-modulated MZI which faithfully preserve the single photon nature. We show dynamic 2f_SAW ≈ 1.05 GHz routing of the QD-emitted single photons between the two outputs exceeding that of electro-optic and nanoelectromechanical tuning on this platform by more than a factor of 100. Moreover, our scheme enables the generation of output states perfectly located in the equatorial plane of the Bloch sphere providing a dynamically tuneable on-chip beamsplitter. Finally, we implemented spectral multiplexing of the emitted single photons by two SAWs, one dynamically straining the emitter which are demultiplexed by a variable acoustic phase-lock to the second SAW driving the single qubit rotation.

The reported proof-of-principle results open several exciting perspectives. First, full arbitrary unitary beamsplitter operation and thus single qubit rotations⁹¹ are straightforward and can be realized simply by adding another IDT at the Output WGs to execute a second phase gate. Second, the purity of the single photon emission can be enhanced by embedding the QDs in SAW-tuneable photonic cavities^68,96. When establishing stable phase lock between a train of excitation laser pulses and the SAW, the latter modulates the cavity-emitter coupling and precisely triggers the Purcell-enhanced emission of single photons⁹³. Third, our approach is scalable because the low propagation loss of SAWs allows to modulate multiple photonic systems and QDs by a single SAW beam⁹⁷ for parallelized control schemes. Fourth, power levels required for switching can be significantly reduced further by embedding the photonic components in phononic resonators and waveguides to enhance the interactions^{65,66,83,98,99,100,101}. Fifth, all demonstrated functionalities can be transferred to hybrid architectures. Important examples include the heterointegration on LiNbO₃ SAW and IQPC devices^46,92, with 100-fold enhanced electromechanical coupling compared to monolithic GaAs devices or CMOS compatible Silicon IPCs with AlN piezoelectric coupling layers⁵⁶ and heterogeneously integrated III-V QDs³⁹

Additionally, we note that the presented concept can be directly applied to other types of quantum emitters^{19,45,73,75,102,103} and spin degrees of freedom^{104,105,106,107}, even in the resolved sideband regime^71,74. Moreover, they can be combined with static electric field^81,108 or stressors¹⁰⁹ tuning to dissimilar QDs could be tuned into resonance to create multi-qubit systems. The integration density can be increased for instance by implementing a phase gate in a single MMI¹¹⁰ and multi-port MMIs enable phased-array wavelength-division multiplexing¹¹¹.

Methods

Sample design and fabrication

The heterostructure was grown by molecular beam epitaxy on a semi-insulating (001) GaAs substrate. It consists of a 1500 nm Al_0.2Ga_0.8As cladding layer followed by a 300 nm thick GaAs waveguide layer with a single layer of optically active self-assembled (In,Ga)As QDs in its center. Devices were fabricated monolithically on these substrates using optical lithography. The IQPCs were etched using inductively coupled plasma reactive ion etching ICP-RIE. IDTs (10 nm Ti/30 nm Al/10 nm Ti) were fabricated by a standard lift-off process.

Device simulation

MMI dimensions were calculated using established numerical simulation methodologies¹¹². The full IQPC comprising individual MMI dimensions, tapered waveguides, S-bends and the respective waveguide interfaces was optimized by finite difference 3D beam propagation method simulations using commercial software packages. For the simulation of the active device a sinusoidal modulation of the refractive index in the optomechanical interaction region of the MZI arms was applied. Device parameters and full details on the model used for the modulated transmission behavior can be found in Supplementary Note 1 and 3.

Experimental setup

Experiments were conducted in a cryogenic photonic probe station which is equipped with custom-made radio frequency lines. A schematic of the full setup and a detailed description are included in Supplementary Note 2. In essence, QDs were optically excited by a non-resonant continuous wave laser (λ_laser = 660 nm) under normal incidence. The QD emission is collected from the cleaved end facets using a lensed fiber and spectrally filtered by a 0.75 m grating monochromator. A cooled CCD detector or up to two silicon single photon detectors (timing resolution 300 ps) are used for time-integrated and time-resolved detection, respectively. The outputs of up to two locked radio frequency signal generators are applied to the IDTs for SAW generation. Additionally, time-resolved optical detection was referenced to these electrical signals^93,113,114.