Abstract
Ferroelectricity, the electrostatic counterpart to ferromagnetism, has long been thought to be incompatible with metallicity due to screening of electric dipoles and external electric fields by itinerant charges. Recent measurements, however, demonstrated signatures of ferroelectric switching in the electrical conductance of bilayers and trilayers of WTe2, a semimetallic transition metal dichalcogenide with broken inversion symmetry. An especially promising aspect of this system is that the density of electrons and holes can be continuously tuned by an external gate voltage. This degree of freedom enables measurement of the spontaneous polarization as free carriers are added to the system. Here we employ capacitive sensing in dual-gated mesoscopic devices of bilayer WTe2 to directly measure the spontaneous polarization in the metallic state and quantify the effect of free carriers on the polarization in the conduction and valence bands, separately. We compare our results to a low-energy model for the electronic bands and identify the layer-polarized states that contribute to transport and polarization simultaneously. Bilayer WTe2 is thus shown to be a fully tunable ferroelectric metal and an ideal platform for exploring polar ordering, ferroelectric transitions, and applications in the presence of free carriers.
Similar content being viewed by others
Introduction
Polar materials exhibit charge separation in the absence of an applied electric field, an effect of broken inversion symmetry and a unique polar axis in the crystal1,2. In certain polar systems, the charge polarization can be switched by an external electric field, an effect known as ferroelectricity. In principle, the presence or absence of ferroelectric effects depends only on the crystal class and not on the details of the electronic structure. Despite this, nearly all known conventional ferroelectrics are electrically insulating. Since the first theoretical proposals for ferroelectric metals in 19651, only a handful of experimental claims of ferroelectric-like phases in metallic systems have been reported3,4,5,6,7,8,9. Many such claims fail to demonstrate two key signatures of ferroelectric behavior—direct evidence of the polarization and ferroelectric switching—due to bulk screening effects. With reports of polar switching in the metallic state of WTe210,11,12, a strong case for a ferroelectric metal has emerged.
Here we focus on the polar, semimetallic van der Waals (vdW) crystal, Td-WTe2, in the limit of two atomic layers, thin enough to admit an external electric field (Fig. 1d, e). Few-layer crystals of WTe2 have drawn recent interest for exhibiting a wide variety of low-temperature phases13,14,15,16,17. Recent transport measurements showed that bilayer and trilayer WTe2 exhibit intrinsic, switchable electrical polarization in the conducting state by detecting the out-of-plane polarization with a monolayer graphene sensor10. Separately, surfaces of bulk WTe2 crystals were shown to display hysteresis in piezoresponse force microscopy11. Subsequent first-principles calculations indicated that the net polarization points only in the out-of-plane direction and that the underlying mechanism results from a subtle interlayer sliding between the layers in two stable configurations18,19, an effect later demonstrated by second harmonic generation12. The in-plane rigidity of the lattice adds an unfavorable energy cost to the formation of neighboring dipoles with opposite polarization, facilitating long-range order and leading to macroscopic polarization. Combined with low carrier density and a thickness less than the out-of-plane screening length11,20, these factors conspire to stabilize a ferroelectric metal state in bilayer WTe2. These findings are exciting given the semimetallic and tunable nature of bilayer WTe2, which enables reaching both electron and hole bands by electrostatic gating in the ferroelectric state. While the detection of polarization and hysteresis in previous experiments10,11 is promising, a measurement of the metallic polarization as a function of charge density for electrons and holes in WTe2 is still missing. In particular, using a remote graphene layer previously enabled direct detection of the out-of-plane polarization10 but prevented independent control of the charge density and electric field. Crucially, this limitation precluded observing the effect of free carriers on the polarization and its dependence on carrier type and density, a fundamental open question for ferroelectric metals.
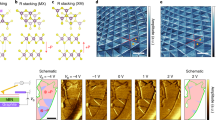
a Schematic of our lithography-free encapsulation and contact method, using a boron nitride (BN) crystal previously prepared with through-hole Au contacts to pick up and transfer WTe2 on to another BN dielectric layer with a graphite bottom gate below. Top gate and leads to the through-hole contacts and bottom gate are patterned after fully encapsulating the WTe2. b Measurement schematic showing the measurable capacitances: Ct, between the top gate and WTe2, and Cb, between the bottom gate and WTe2. Ci is the interlayer capacitance across the bilayer. c Measured top capacitance, Ct, as a function of carrier density, n0, at zero and finite electric field, E⊥. d Side-view structure of bilayer WTe2 in two stable configurations, each showing the net polarization state along c-axis (P). The two states are related equivalently by a mirror operation along the c-axis (\({{{{{{{{\mathcal{M}}}}}}}}}_{c}\)) or by lateral translation between the layers along the b-axis of 0.72 Å18,19, or ~11% of the unit cell. The ⋆ symbol labels a Te atom before and after the mirror operation (black) and translation (red) as a visual guide. The mirror/translation equivalence allows a subtle shift between the layers to switch the structure between polarization states. e Top-view structure showing the only invariant symmetry of the crystal, mirror reflection along the a-axis (\({{{{{{{{\mathcal{M}}}}}}}}}_{a}\)).
In this work, we directly measure the charge polarization and electronic compressibility as a function of density for electrons and holes with independent control of the electric field. We study the simplest polar WTe2 system, a bilayer, via capacitive sensing in a dual-gated, planar capacitance device (Fig. 1b). Capacitance measures the electronic compressibility (and thus metallicity) of a two-dimensional (2D) system. In a bilayer 2D system, the top- and bottom-gate capacitances provide a direct measurement of the layer-specific charge distribution21, and thus the out-of-plane polarization, as previously demonstrated in bilayer graphene22. Furthermore, the parallel-plate geometry enables this charge sensing with simultaneous and independent control of the vertical electric field and the carrier density in the bilayer by electrostatic gating.
Results
Our devices each consist of a bilayer WTe2 crystal encapsulated by two hexagonal boron nitride (hBN) dielectric layers, with metallic top and bottom gates, and contacts integrated into the top hBN layer23 (Fig. 1a).
We measure the capacitance between the top gate and the bilayer, Ct, while applying DC voltages to the top and bottom gates to tune the (nominal) total carrier density, \({n}_{0}\propto {C}_{t}^{0}{V}_{t}+{C}_{b}^{0}{V}_{b}\), and out-of-plane electric field, \({E}_{\perp }\propto {C}_{t}^{0}{V}_{t}-{C}_{b}^{0}{V}_{b}\) (Fig. 1b). While the geometric contributions to the capacitance (\({C}_{t}^{0}\) and \({C}_{b}^{0}\)) are constant, there are additional contributions to the measured capacitance Ct from the electronic compressibility. In a bilayer system with partial electric field penetration, the layer-specific densities ni can differ between the two layers (i = 1, 2) for a given total density n = n1 + n2, particularly in the presence of an electric field. The electrostatic potentials of each layer ϕi depend on the top- and bottom-gate voltages and, crucially, any built-in electric field in the bilayer21,22. As such, ϕi in each layer can also differ, even in thermodynamic equilibrium. The electronic compressibility of a 2D bilayer is generally described by a 2 × 2 matrix, νij = − ∂ni/∂ϕj; however, in a weakly coupled bilayer it is possible to characterize the system with only the diagonal elements, νii, the layer-specific compressibilities. Due to the vdW nature of the interlayer coupling, in bilayer WTe2 this is indeed the case (Supplementary Fig. 9). Subsequently, the top capacitance may be written,
(see “Methods” for details). The second term in Eq. (1) is a quantum correction to the geometric capacitance \({C}_{{{{{{\rm{t}}}}}}}^{0}\), inversely proportional to the layer-specific compressibility of the top layer, ν11. The layer-specific densities n1 and n2 can be obtained by integrating the capacitance, and thus the polarization, proportional to p = n1 − n2, can be measured.
For fixed, external field E⊥ = 0, the measured Ct as a function of the electron density exhibits a minimum near charge neutrality, n0 ≈ 0 (Fig. 1c). The minimum in Ct indicates the presence of a small band gap (incompressible state) in the WTe2 bilayer, a feature consistent with previous observations of a sharp drop in the conductance of bilayer WTe2 in transport10,24. At fixed, negative electric field, the capacitance minimum becomes more prominent, suggesting that the gap is electric field tunable (see Supplementary Fig. 4), similar to bilayer graphene22,25. However, in contrast to bilayer graphene the electric field response is robustly asymmetric around E⊥ = 0, as shown in the full n0 and E⊥ dependence in Fig. 2a. This effect results from the absence of an inversion center or mirror plane between the layers of bilayer WTe2, implying the crystal is polar along the c-axis (Fig. 1e).
a Forward and b backward scans of the top capacitance Ct as a function of electric field, E⊥, for a range of carrier densities, n0. c Capacitance traces measured along the dashed lines in a, b (beginning at the ⋆ symbol in each case) displaying a smooth background as well as sudden jumps at electric field values that depend on the sweep direction. d Difference between the traces in c. e Compilation of differences between forward and backward scans in a, b for an extended range of carrier densities, showing the change in sign of the switching from electrons to holes and the gradual density dependence of the switching behavior.
To probe the switching behavior of the polar direction, we sweep the electric field back and forth at fixed density, as shown in Fig. 2a, b. Sudden changes are observed in Ct at all densities: Ct jumps at a positive critical electric field value \({E}_{{{{{{\rm{c}}}}}}}^{+}\) when sweeping toward positive E⊥ (Fig. 2a), whereas the critical field \({E}_{{{{{{\rm{c}}}}}}}^{-}\) is negative when sweeping in the negative direction (Fig. 2b), forming a hysteresis loop (Fig. 2c) at each density. Taking the difference of two representative sweeps in opposite directions \({{\Delta }}{C}_{{{{{{\rm{t}}}}}}}\equiv {C}_{{{{{{\rm{t}}}}}}}^{\leftarrow }-{C}_{{{{{{\rm{t}}}}}}}^{\to }\) (Fig. 2d), we see that Ct overlaps nearly everywhere excluding the hysteretic region between the critical fields, \({E}_{{{{{{\rm{c}}}}}}}^{\pm }\), where Ct is multi-valued. The critical fields generally fall within ∣E⊥∣ ≲ 0.1 V/nm and appear to be weakly dependent on charge density (Fig. 2e). Interestingly, the sign of the hysteretic difference switches for holes (ΔCt < 0) compared to electrons (ΔCt > 0), and the magnitude of the difference decreases at large densities of either sign.
Switching behavior is clearly present in the capacitance, but to understand how this relates to the polarization we must first recognize that the ground state structure of bilayer WTe2 possesses two stable configurations with opposite polarization12,18,19 (Fig. 1d). Similarly, from Fig. 2a–c, we see that there are two stable values for Ct in the central region between the two critical fields, \({E}_{{{{{{\rm{c}}}}}}}^{\pm }\). To reveal how the Ct bistability relates to the two polarization states, we employ a self-consistent calculation of the capacitance using a k ⋅ p model for the bilayer WTe2 bands26 together with an exact form of Eq. (1) (see Eq. (11a) in “Methods”). The k ⋅ p Hamiltonian (Eq. (4)) provides the low-energy electronic structure, shown schematically in Fig. 3a, and layer-projected wavefunctions in each polarization state. The layer-specific densities ni (Fig. 3b) and electric potentials ϕi are obtained from the bands by performing a self-consistent electrostatics calculation for the parallel-plate system (see “Methods”). The compressibilities (Fig. 3c) are obtained by numerical differentiation and inserted into the general form of Eq. (1) to compute the capacitance (given by Eq. (11a)). Since there are two stable configurations for the bilayer, these quantities all depend on the polarization state, yielding different values \({n}_{i}^{\pm }\), \({\phi }_{i}^{\pm }\), and \({\nu }_{ii}^{\pm }\) in each case. Using these calculated quantities, the polarization at fixed n0 can be determined from the difference \({P}^{\pm }=e{d}_{i}({n}_{1}^{\pm }-{n}_{2}^{\pm })\) for interlayer separation di. Evaluating this directly, we can construct a schematic polarization loop that illustrates both the dielectric polarization response as well as the spontaneous polarization from the computed layer densities (shown for n0 ≈ 0 in Fig. 3d, with switching behavior added manually for illustration). The difference between the layer imbalance in the two polarization states yields the change in the spontaneous polarization, ΔPs ≡ P+ − P−, as indicated for E⊥ = 0 in Fig. 3d.
a Schematic low-energy bands showing layer-polarized valleys (orange and purple) in the P+ state, for small electron density and E⊥ = 0. In the P− state, the colors and layer polarization would be interchanged. Each pair of valleys is centered around a point along Γ–X, labeled Q. Band parameters are exaggerated to emphasize separation of valleys. b Representative calculated layer densities, c compressibilities, and d polarization \(\propto {n}_{1}^{\pm }-{n}_{2}^{\pm }\) in each polarization state, ±, from n0 ≈ 0. e Computed top capacitances, \({C}_{{{{{{\rm{t}}}}}}}^{+}\) and \({C}_{{{{{{\rm{t}}}}}}}^{-}\) in the P+ and P− states, respectively, for the listed densities versus electric field, with colored symbols indicating the hysteretic path observed in experiment, while gray symbols denote portions of each capacitance branch that are inaccessible in experiment due to switching behavior (switching fields are not computed in the model; solid vertical lines are shown extending from experimental switching fields in f to reflect the loop observed in the experiment). Capacitance is calculated using computed layer densities, potentials, and compressibilities to evaluate Eq. (11a). f Measured top capacitance hysteresis loops for matching electron and hole densities in e.
Polarization switching reflects a reversal of the density imbalance between the layers (Fig. 3a, b) and manifests as switching in the top-gate capacitance due to the inequivalence of the layer-specific compressibility in the two polarization states (Fig. 3c). For instance, in Fig. 3e, we show calculated curves for Ct as a function of E⊥ at a few selected electron and hole densities using the calculated compressibilities. The result of the calculation is two capacitance curves, \({C}_{{{{{{\rm{t}}}}}}}^{+}\) and \({C}_{{{{{{\rm{t}}}}}}}^{-}\), that correspond to the polarization states P+ and P−, respectively. Comparing to the experiment (Fig. 3f), for sufficiently large positive (negative) E⊥, we only measure the \({C}_{{{{{{\rm{t}}}}}}}^{+}\) (\({C}_{{{{{{\rm{t}}}}}}}^{-}\)) branch. In the hysteretic region, the polarization state P+ or P− depends on the direction of the electric field sweep, and thus the measured capacitance jumps between the \({C}_{{{{{{\rm{t}}}}}}}^{+}\) and \({C}_{{{{{{\rm{t}}}}}}}^{-}\) branches at the coercive fields, \({E}_{{{{{{\rm{c}}}}}}}^{\pm }\). While our model does not include all the details of the electronic structure (Supplementary Fig. 6), and thus we do not expect a perfect match with experiment, the general trends of the capacitance with E⊥ and n0 are captured nicely, for instance, the observation that \({C}_{{{{{{\rm{t}}}}}}}^{+} \, > \, {C}_{{{{{{\rm{t}}}}}}}^{-}\) for electrons and \({C}_{{{{{{\rm{t}}}}}}}^{+} \, < \, {C}_{{{{{{\rm{t}}}}}}}^{-}\) for holes. Next we will see that some of the details of the electronic structure are less relevant for difference quantities such as ΔCt and ΔPs, which leads to improved agreement between the model and experiment, as shown in Fig. 4.
a Measured density dependence of \({{\Delta }}{C}_{{{{{{\rm{t}}}}}}}\equiv {C}_{{{{{{\rm{t}}}}}}}^{+}-{C}_{{{{{{\rm{t}}}}}}}^{-}\) at E⊥ = 0. b Change in measured spontaneous polarization calculated by integration, ΔPs ∝ ∫ΔCtdn0. Left axis provides 2D polarization units while the right scale is given in terms of charge separation between the layers, Δps = ΔPs/di for WTe2 interlayer separation, di = 0.7 nm. Background shading follows the magnitude of ΔPs, illustrating distinct regions of ferroelectric (FE) behavior. c Computed ΔCt and d ΔPs based on model Hamiltonian for bilayer WTe2, with inset in d showing computed ΔPs for an extended density range (with identical units). ΔPs is computed by two different methods: “Exact” using Eq. (18), and “Approx.” using Eq. (33).
Finally, we address the density dependence of the polarization to ascertain the effect of adding free charge to a polarized semimetal. Previously, we defined ΔCt as the difference between the right-sweeping and left-sweeping Ct curves. Having identified the \({C}_{{{{{{\rm{t}}}}}}}^{\pm }\) curves in the central region of each hysteresis loop, we may now equate \({{\Delta }}{C}_{t}={C}_{t}^{+}-{C}_{t}^{-}\) for \({E}_{c}^{-} \, > \,{E}_{\perp } \, > \, {E}_{c}^{+}\). Figure 4a shows the density dependence of ΔCt for E⊥ = 0, equivalent to a vertical line cut from Fig. 2e. The non-monotonic curve follows the size of the Ct hysteresis loop, showing a maximum and minimum on either side of charge neutrality and trending toward zero at large densities. The difference ΔCt evaluated at E⊥ = 0 is related to a dipole compressibility, ∂p/∂n0 (see “Methods”), a measure of spontaneous dipole formation as carriers are added to the system. This quantity yields the change in layer polarization density p = n1 − n2 as the chemical potential is varied (via the combination of gate voltages, n0) and thus is not relevant in ferroelectric insulators. By integrating ΔCt with respect to carrier density, we obtain the change in spontaneous polarization as a function of density along E⊥ = 0,
The result of this integration along E⊥ = 0 is shown in Fig. 4b (including geometrical prefactors given in Eq. (33)). Intuitively, the spontaneous polarization is strongest at charge neutrality, where there are few free carriers available to screen the built-in polarization. Adding electrons or holes to the system reduces the net polarization. However, a pronounced asymmetry emerges in the spontaneous polarization depending on the carrier type, with the polarization decreasing faster from its value at charge neutrality for holes compared to electrons. Thus, holes appear to screen the polarization much more effectively than electrons for equivalent charge densities. This effect can be understood by considering the layer character of the available states in the conduction and valence bands. First-principles calculations indicate that the low-energy states are intrinsically layer-polarized (see Supplementary Fig. 6), even in the absence of an external electric field. Though there are states from both layers in the conduction and valence bands, the cumulative effect of filled states up to the Fermi level results in a net polarization due to an imbalance of layer character, particularly near the band edges (see Supplementary Fig. 7). The magnitude of this imbalance is larger for valence band states compared to the conduction band. Consequently, adding holes to the valence band quickly suppresses the net spontaneous polarization, whereas adding electrons to the conduction band weakly screens it, allowing a net polarization to persist to relatively large densities (Fig. 4b). Alternatively, in an all-electron picture, adding electrons to a partially filled valence band acts to increase the net polarization due to the cumulative layer polarization of the filled states. Conduction band electrons possess an opposite layer polarization relative to the valence band and thus partially screen the cumulative polarization of the valence band as carriers are added (see Supplementary Fig. 7). Notably, the free carriers at the Fermi level are layer-polarized and thus contribute to the polarization, contrary to the simple electrostatic picture of screening in bulk metals.
Discussion
Broken inversion and mirror-z symmetries together with the ultrathin, layered structure conspire to allow layer-polarized states at the Fermi level. These broken symmetries and large spin–orbit coupling further contribute to the pronounced e–h asymmetry (see Supplementary Fig. 8). This asymmetry manifests as several distinct ferroelectric regimes with both bound and free charges contributing to the net polarization. At large hole densities, the measured polarization quickly trends toward zero, entering a screened polar metal regime. In this phase, there remains underlying polarized bound charge made up of remote states; however, there are enough filled states with opposing layer character to screen this charge, resulting in a suppressed net polarization. On the other hand, at large electron densities the polarization decreases more slowly, exhibiting a persistent, n-type metallic ferroelectric state. Near charge neutrality, we observe a crossover between these p- and n-type metallic ferroelectric states. The crossover regime is further complicated by the electric field dependence of the conduction and valence bands, as shown in Fig. 2a, b. From E⊥ = 0, the charge neutral behavior appears to transition from a ferroelectric semimetal with finite band overlap to a ferroelectric insulator with a maximal band gap of ~3 meV from 0.3 V/nm > ∣E⊥∣ > 0.2 V/nm (Supplementary Fig. 4).
These interpretations are supported by our model calculations, with both the density dependence of calculated ΔCt and the integrated ΔPs matching well with experiment (Fig. 4c, d). The model also allows us to investigate the expected behavior over an extended density range, inaccessible in experiment due to dielectric breakdown of hBN. As shown in the inset of Fig. 4d, the calculated ΔPs continues to exhibit sustained ferroelectric behavior up to electron densities of at least n0 ≈ 4 × 1013 cm−2, whereas the p-type ferroelectric behavior is substantially suppressed below n0 ≈ −2 × 1013 cm−2.
In conclusion, we see that bilayer WTe2 may be tuned from a sustained ferroelectric n-type metal for n0 > 1013 cm−2 to a variable-polarization ferroelectric p-type metal at intermediate hole densities, followed by a screened polar metal at large hole densities. In the crossover regime between the n- and p-type ferroelectric metal states, a ferroelectric insulator or semimetallic phase can be obtained depending on the electric field. The out-of-plane polarization is largest in the neighborhood of these latter phases, reaching a charge separation of ~3.7 × 1011 electrons/cm2 over 0.7 nm between the layers, equivalent to a volume polarization density of ~0.6 mC/m2. This maximum and the range of measured polarization magnitudes shown in Fig. 4b are comparable to the experimental value of 2 × 1011 e/cm2 measured in bilayers without independent control of the carrier density10 and theoretical estimates of 3.2 × 1011 e/cm2 for bilayers18 and 1.2 × 1012 e/cm2 for bulk crystals11. While these magnitudes are small compared to state-of-the-art ferroelectric insulators, the ability to tune the magnitude of the spontaneous polarization in situ and the ease of integration into vdW heterostructures is especially promising for future investigations. The ferroelectric behavior in each metallic phase is supported by the strong layer-polarized character of the states, a result of broken inversion and mirror symmetries combined with weak interlayer coupling. Together, these observations and the ingredients in WTe2 that lead to them provide a recipe for engineering and measuring new ferroelectric metal systems. Looking forward, we anticipate that metallic ferroelectricity will manifest in additional transition metal dichalcogenide and vdW structures27,28,29,30,31,32,33, enabling a host of new experiments in this under-explored regime.
Methods
Fabrication of capacitance devices
WTe2 is an especially air-sensitive material, quickly degrading in ambient conditions. To avoid air exposure during the fabrication process, we first integrate metal contacts into the top BN layer and then transfer this template onto the WTe2 in a N2-filled glovebox. The metal contacts are prepared ahead of time by etching holes through the BN crystal and subsequently evaporating pure Au contacts to fill these holes. We pick up the top BN along with the integrated Au contacts and use this layer to pick up the WTe2 crystal using standard dry transfer techniques23. We then pick up a bottom layer of BN to fully encapsulate the WTe2 and place the stack on a graphite or PdAu bottom gate, with the WTe2 partially overlapping the gate but with the through-hole contacts positioned as near as possible to the gated region (Fig. 1a). At this stage, the WTe2 is sealed from the environment on all sides, allowing us to pattern leads and a top gate using standard electron-beam lithography and metal evaporation. The top gate is designed to overlap the WTe2 crystal up to the edge of the bottom gate, aligning carefully to this edge. This ensures that the entire gated region of the crystal is dual-gated, preventing single-gate features in our capacitance measurements.
Capacitance measurement
Capacitance measurements were performed by connecting the bilayer WTe2 in each device to a capacitance bridge circuit (Supplementary Fig. 1) with a standard, known capacitor, Cstd. We apply an 11 kHz alternating current (ac) excitation δVt with an root-mean-square amplitude from 64 to 140 mV on the top gate of the device and a nearly out-of-phase signal δVstd to Cstd in order to null the total ac signal at the bridge balance point. Both the amplitude and relative phase of δVstd are tuned to produce a null signal. As the total capacitance of the device changes, deviations from the null voltage are amplified by a high-electron-mobility transistor mounted within a few millimeters of the sample in the cryostat. We determine the measured top capacitance Ct from the amplified deviation from the null voltage, Ct = Cstd(ΔVnull/δVstd). All measurements were performed in a dilution refrigerator with the sample between 100 mK (as in Figs. 1, 2a–d, and 3) and 30 K (Fig. 2e) though little change was observed in the capacitance features in this range.
k ⋅ p model for bilayer WTe2
To compute the layer compressibilities for bilayer WTe2, we adopt a k ⋅ p model for the Hamiltonian describing massive Dirac fermions in two layers with spin–orbit and interlayer coupling. Beginning with a tilted massive Dirac Hamiltonian26, we introduce a layer index, i = 1, 2, and electrostatic potential on each layer, ϕi, to include the effect of a vertical electric field as well as the polarization,
along with a shifted kx-coordinate, \({\tilde{k}}_{x,i}\equiv {k}_{x}+{q}_{i}\) that accounts for the position of each layer-polarized valley (from the Q-point shown in Fig. 3c, for \({{{{{{{\bf{Q}}}}}}}}=Q{\hat{{{{{{{{\bf{k}}}}}}}}}}_{x}\)). The Pauli matrices, σx,y,z, act in the orbital pseudospin space, s = ↑, ↓ is the spin degree of freedom, η = ±1 is a chiral index, t tilts the Dirac cones along kx, and m gives rise to the gap at charge neutrality (αk2 ensures convergence of \({\hat{{{{{{{{\mathcal{H}}}}}}}}}}_{i}\) as k → ∞). For E⊥ = 0, the electrostatic potentials take fixed values \({\phi }_{i}({E}_{\perp }=0)={\phi }_{i}^{0}\) (see Supplementary Table 2) to account for the spontaneous polarization. The spin and layer degrees of freedom are coupled in the \(\left|1,\uparrow \right\rangle\), \(\left|1,\downarrow \right\rangle\), \(\left|2,\uparrow \right\rangle\), \(\left|2,\downarrow \right\rangle\) basis to obtain the effective Hamiltonian,
by interlayer coupling, γ, and spin–orbit coupling,
where λx, λy govern the spin–orbit coupling strength in the x and y directions. Such a model has previously been applied to describe the Berry curvature dipole observed in bilayer WTe2 in vertical electric fields17 and captures the spin and shifted-valley character of WTe2 bands determined by first-principles methods26,34. Here we explicitly include a polarization state label, ±, in the Hamiltonian, \({\hat{{{{{{{{\mathcal{H}}}}}}}}}}^{\pm }\), to denote the two possible configurations for the WTe2 bilayer. The Hamiltonian for the opposite polarization state, \({\hat{{{{{{{{\mathcal{H}}}}}}}}}}^{-}\), is obtained by interchanging layers, 1 ↔ 2, and spin. Note that both the electrostatic potential, ϕi, and shifted valley coordinate, \({\tilde{k}}_{x,i}\), depend on the layer index and thus are interchanged upon a simultaneous mirror operation (\({{{{{{{{\mathcal{M}}}}}}}}}_{c}\)) and inversion of the electric field. As a result, the Hamiltonian possesses an identity,
relevant for extracting the polarization from the measured capacitance, to be discussed in a subsequent section.
Though this model is single particle in nature, we note that we should view the model as describing effective single-particle orbits after electron–electron interactions are included to a certain degree. That is, screening effects due to electron–electron interactions are included in first-principles calculations at the mean field level, and our model is tuned to be consistent with the first-principles results. Furthermore, we find that the result of the model, our computed capacitance curves, match quite well with experiment, particularly when comparing to the capacitance difference in the two polarization states. As such, we conclude that additional effects of electron–electron interactions are not necessary to describe the observed polarization phenomena.
Capacitance calculation
To understand the various features of the measured capacitance, we apply this effective model to calculate the band structure and eigenstates and numerically evaluate the capacitance for a similar geometry. Starting with Eq. (4), we determine the probability densities in each layer and sum over occupied states to obtain the layer-specific densities, n1 and n2 for the top and bottom layers, respectively. The layer-specific compressibility elements νij are determined by taking partial derivatives of the densities ni with respect to the electric potentials on each layer. The resulting compressibilities in conjunction with the geometric capacitances then determine the calculated capacitances.
The eigenstates of Eq. (4) span an 8 × 8 Hilbert space of layer, spin, and orbitals. We obtain layer densities by first calculating the probability density on each layer from the eigenstates,
for spin s = ↑, ↓ and orbital pseudospin m. Note that here, and for the remainder of this section, we have dropped explicit labels making reference to the polarization state for simplicity. Layer densities are then determined by integration,
where f(p) is the Fermi-Dirac distribution. However, Eq. (8) only includes contributions from valence electrons. The contribution from ions can be determined as follows. With the absence of an external electric field, E⊥ = 0 and \({\phi }_{i}={\phi }_{i}^{0}\), and the bilayer is at charge neutrality. We then have a vanishing total charge, \({n}_{1}^{\prime}+{n}_{2}^{\prime}+{n}_{1}^{{{{{{\rm{ion}}}}}}}+{n}_{2}^{{{{{{\rm{ion}}}}}}}=0\). On the other hand, the two layers have same ionic composition. As a result,
From this definition, we obtain the relevant layer densities,
To perform a self-consistent calculation, we treat the electrostatic potentials, ϕi, as parameters and compute the layer densities, ni = ni(ϕ1, ϕ2) for an appropriate grid of values. The compressibilities are then obtained by taking the partial derivatives νij = ∂ni/∂μj = −∂ni/∂ϕj over the same parameter space (where ϕi = −μi for layer-specific chemical potential μi and with the sample connected to ground). Finally, the capacitances are defined in terms of small signal variations, Ct ≡ (δn1 + δn2)/δVt, Cb ≡ (δn1 + δn2)/δVb, and Cp ≡ δnt/δVb, where Cp is the penetration field capacitance, measured from the top gate to the bottom gate, for charge on the top gate nt. These expressions may be evaluated in terms of the compressibility elements (following ref. 21),
with geometric capacitances \({C}_{{{{{{\rm{t}}}}}}}^{0}\), \({C}_{{{{{{\rm{b}}}}}}}^{0}\), and interlayer capacitance Ci, as shown in Fig. 1b, each given by Cj = ϵϵ0A/dj using ϵ = 3.2 for hBN and ϵ = 1 within the bilayer (modeling the bilayer in the out-of-plane direction as two compressible metal sheets separated by vacuum, an assumption that does not significantly affect the outcome). The compressibility matrix, \(\hat{\nu }\), is a symmetric matrix, νij = νji, and takes the form
while \(\hat{C}\) is a matrix of geometric capacitances,
To obtain results in terms of E⊥ and n0, we first apply a transformation derived from the charge balance equations,
to determine Vb and Vt,
and then calculate E⊥ = (Vt/dt − Vb/db)/2 for top and bottom hBN thicknesses, dt and db, respectively, and \({n}_{0}=({C}_{{{{{{\rm{t}}}}}}}^{0}{V}_{{{{{{\rm{t}}}}}}}+{C}_{{{{{{\rm{b}}}}}}}^{0}{V}_{{{{{{\rm{b}}}}}}})/e\). Self-consistent solution of Eqs. (14a), (14b), and (15) includes the charge density and electric potential on each layer for a given set of applied gate voltages and thus fully incorporates the effect of screening the externally applied electric fields. Finally, to include the effect of the two polarization states, as shown in the main text for \({C}_{{{{{{\rm{t}}}}}}}^{\pm }\), we compute the capacitances separately for each case, ±, using the compressibilities, \({\nu }_{ij}^{\pm }\), calculated from the eigenstates of \({\hat{{{{{{{{\mathcal{H}}}}}}}}}}^{\pm }\), derived from the appropriate form of Eq. (4).
Simplifications and approximations
In practice, quantum capacitances of 2D electron gases tend to dominate over geometric capacitances from external electrodes and gates due to the small energy spacing between levels. For instance, the density of states (and thus the non-interacting electronic compressibility) in monolayer graphene is of the order ρMLG(0) ~ 1013 eV/cm2, yielding a quantum capacitance of order Cq ~ 20 fF/μm2. In comparison, a metallic gate separated by 10 nm of hBN from a parallel plate generates a geometric capacitance one order of magnitude smaller, Cg ~ 2 fF/μm2. Typical thicknesses for hBN gate dielectrics are even larger, such as the device shown in the main text with dt ≈ 15 nm and db ≈ 19 nm, resulting in even smaller geometric capacitances. Additionally, in each layer of bilayer WTe2, screening from carriers in the nearby layer and weak interlayer coupling significantly reduces the off-diagonal compressibilities relative to the diagonal terms in Eq. (12). Together, these considerations imply a hierarchy, \(| {\nu }_{11}| ,| {\nu }_{22}| \gg {C}_{{{{{{\rm{t}}}}}}}^{0},{C}_{{{{{{\rm{b}}}}}}}^{0},{C}_{i}\gg | {\nu }_{12}| ,| {\nu }_{21}|\) (ignoring factors of e2 preceding the νij terms). As a result, the diagonal compressibilities νii dominate the behavior of Ct and Cb, enabling an approximate form for Eqs. (11a–11c),
as shown in Eq. (1) in the main text. While the numerical computations shown in the paper make use of the full Eq. (11a), deviations of the approximate formulas from the exact quantities are negligible and thus the simpler form is given in main text Eq. (1) to facilitate understanding.
Capacitance relation to polarization
To extract the spontaneous polarization from the measured capacitance, we seek the change in the polarization,
obtained by integrating the difference of dipole compressibilities, ∂p/∂n0 in each polarization state,
defined up to a constant of integration, ΔP0, which we take to be zero at large hole densities, where the density-dependent spontaneous polarization appears to saturate. The dipole compressibility in each state, ±, is defined by
Equation (18) together with Eq. (19) is employed to compute the “Exact” spontaneous polarization in the model calculations shown in Fig. 4d. In the experiment, we do not have direct access to the partial derivatives in Eq. (19); however, the symmetry of our device allows an approximate form of Eq. (18) to be related to ΔPs.
Following ref. 22, we express the dipole compressibility in Eq. (19) as,
where we have dropped the label indexing the polarization state. We then employ
to obtain the partials ∂ni/∂Vt,b and finally rewrite the dipole compressibility in terms of the average geometric capacitance, \(\langle C\rangle =({C}_{{{{{{\rm{b}}}}}}}^{0}+{C}_{{{{{{\rm{t}}}}}}}^{0})/2\), and the asymmetry, \(\delta =({C}_{{{{{{\rm{b}}}}}}}^{0}-{C}_{{{{{{\rm{t}}}}}}}^{0})/({C}_{{{{{{\rm{b}}}}}}}^{0}+{C}_{{{{{{\rm{t}}}}}}}^{0})\),
For the device shown in the main text (Device A in Supplementary Table 1), δ ≈ 0.11 and 〈C〉/2Ci ≈ 0.067. Keeping only the leading order terms in these two small parameters, we obtain
Therefore, we have
where \({{\Delta }}{C}_{{{{{{\rm{t(b)}}}}}}}\equiv {C}_{{{{{{\rm{t(b)}}}}}}}^{+}-{C}_{{{{{{\rm{t(b)}}}}}}}^{-}\). To evaluate this further, we first rearrange Eq. (1) and explicitly show the E⊥ dependence (ignoring factors of e2),
which implies
Similarly, we have
Using the fact that switching the polarization state is equivalent to a mirror operation between the layers, we find
a symmetry that is evident in the identity relating the Hamiltonian in the two polarization states, Eq. (6), and the subsequent compressibilities derived from each. Physically, this implies that measuring the capacitance from one side of the device in the two polarization states is related to a measurement of the capacitance from both sides of the device by geometrical factors. Thus, we invoke Eq. (28) to relate Eqs. (26) and (27),
or equivalently
for hBN dielectrics on both sides of the device. Finally, we insert this identity into Eq. (24),
solely in terms of measured ΔCt(E⊥). Integrating this expression from the largest hole density measured, nl, up to n0, we obtain
Evaluating at E⊥ = 0, we arrive at the approximate integral used to determine the measured polarization in Fig. 4b,
The same expression is employed in Fig. 4d to calculate the “Approx.” curve based on computed capacitance data, illustrating the small deviation from the exact polarization introduced by making the approximations outlined in this section. A small constant of integration is included in the total measured spontaneous polarization shown in Fig. 4b, P0 = 0.42 e/cm, inferred by matching the magnitude of the measured ΔPs(n0) with the calculated curve. The latter curve exhibits saturation of ΔPs at large hole densities (taken to be zero) and thus offers a lower bound on the constant of integration and ultimately the maximum value of the polarization near charge neutrality.
First-principles calculations
As the essential ingredient to interpret the capacitance in experiments, the low-energy model in Eq. (4) is well supported by first-principles calculations. The most essential element for the capacitance is the polarization or the occupation difference between the top and bottom layer. In Supplementary Fig. 6, we show such layer character in the band structure near one group of valleys from the first-principles calculations compared with those from the low-energy model. By tracing the valence band, we find that bands from the low-energy model and first-principles calculation show similar tilting, together with similar layer-occupancy variation. This striking similarity demonstrates that the low-energy model is indeed capable of capturing the essential physics for the capacitance calculation.
The supporting density functional theory (DFT) calculations shown in Supplementary Fig. 6b are performed with the Vienna Ab initio Simulation Package using the PBEsol functional. The plane wave basis cutoff is 300 eV. The atomic structures are relaxed until forces on every atom are <0.01 eV/Å and the vacuum layer is >15 Å. The vdW functional used here is the zero damping DFT-D3 method with small modifications: the vdW correction is only applied to atomic pairs from different layers, since monolayers can be calculated well without vdW correction. This modification ensures that the structure of the sub-layer of the bilayer will not be affected by an inappropriate vdW correction, leading to the same lattice constant for the monolayer and bilayer system.
Data availability
All relevant data in this study are available from the corresponding authors upon reasonable request.
Code availability
Custom computer codes used to support the conclusions in this study are available from the corresponding authors upon reasonable request.
References
Anderson, P. W. & Blount, E. I. Symmetry considerations on martensitic transformations: “ferroelectric” metals? Phys. Rev. Lett. 14, 217 (1965).
Rabe, K. M., Dawber, M., Lichtensteiger, C., Ahn, C. H., & Triscone, J. M. In Physics of Ferroelectrics, (eds Rabe, K. M., Ahn, C. H. & Triscone, J.-M.) Vol. 105, 1–30 (Springer, 2007).
Shi, Y. et al. A ferroelectric-like structural transition in a metal. Nat. Mater. 12, 1024 (2013).
Liebmann, M. et al. Giant Rashba-type spin splitting in ferroelectric GeTe(111). Adv. Mater. 28, 560 (2016).
Kim, T. H. et al. Polar metals by geometric design. Nature 533, 68 (2016).
Filippetti, A., Fiorentini, V., Ricci, F., Delugas, P. & Íñiguez, J. Prediction of a native ferroelectric metal. Nat. Commun. 7, 11211 (2016).
Benedek, N. A. & Birol, T. ‘Ferroelectric’ metals reexamined: fundamental mechanisms and design considerations for new materials. J. Mater. Chem. C 4, 4000 (2016).
Rischau, C. W. et al. A ferroelectric quantum phase transition inside the superconducting dome of Sr1−xCaxTiO3−δ. Nat. Phys. 13, 643 (2017).
Yuan, S. et al. Room-temperature ferroelectricity in MoTe2 down to the atomic monolayer limit. Nat. Commun. 10, 1 (2019).
Fei, Z. et al. Ferroelectric switching of a two-dimensional metal. Nature 560, 336 (2018).
Sharma, P. et al. A room-temperature ferroelectric semimetal. Sci. Adv. 5, eaax5080 (2019).
Xiao, J. et al. Berry curvature memory through electrically driven stacking transitions. Nat. Phys. 16, 1028 (2020).
Wu, S. et al. Observation of the quantum spin Hall effect up to 100 kelvin in a monolayer crystal. Science 359, 76 (2018).
Fatemi, V. et al. Electrically tunable low-density superconductivity in a monolayer topological insulator. Science 362, 926 (2018).
Sajadi, E. et al. Gate-induced superconductivity in a monolayer topological insulator. Science 362, 922 (2018).
Xu, S. Y. et al. Electrically switchable Berry curvature dipole in the monolayer topological insulator WTe2. Nat. Phys. 14, 900 (2018).
Ma, Q. et al. Observation of the nonlinear Hall effect under time-reversal-symmetric conditions. Nature 565, 337 (2019).
Yang, Q., Wu, M. & Li, J. Origin of two-dimensional vertical ferroelectricity in WTe2 bilayer and multilayer. J. Phys. Chem. Lett. 9, 7160 (2018).
Liu, X. et al. Vertical ferroelectric switching by in-plane sliding of two-dimensional bilayer WTe2. Nanoscale 11, 18575 (2019).
Wang, L. et al. Direct observation of a long-range field effect from gate tuning of nonlocal conductivity. Phys. Rev. Lett. 117, 176601 (2016).
Young, A. F. & Levitov, L. S. Capacitance of graphene bilayer as a probe of layer-specific properties. Phys. Rev. B 84, 085441 (2011).
Hunt, B. M. et al. Direct measurement of discrete valley and orbital quantum numbers in bilayer graphene. Nat. Commun. 8, 948 (2017).
Telford, E. J. et al. Via method for lithography free contact and preservation of 2D materials. Nano Lett. 18, 1416 (2018).
Fei, Z. et al. Edge conduction in monolayer WTe2. Nat. Phys. 13, 677 (2017).
Young, A. F. et al. Electronic compressibility of layer-polarized bilayer graphene. Phys. Rev. B 85, 235458 (2012).
Du, Z. Z., Wang, C. M., Lu, H.-Z. & Xie, X. C. Band signatures for strong nonlinear Hall effect in bilayer wte2. Phys. Rev. Lett. 121, 266601 (2018).
Li, L. & Wu, M. Binary compound bilayer and multilayer with vertical polarizations: two-dimensional ferroelectrics, multiferroics, and nanogenerators. ACS Nano 11, 6382 (2017).
Zheng, Z. et al. Unconventional ferroelectricity in Moiré heterostructures. Nature 588, 71 (2020).
Yasuda, K., Wang, X., Watanabe, K., Taniguchi, T. & Jarillo-Herrero, P. Stacking-engineered ferroelectricity in bilayer boron nitride. Science 372, 1458 (2021).
Vizner Stern, M. et al. Interfacial ferroelectricity by van der Waals sliding. Science 372, 1462 (2021).
Woods, C. R. et al. Charge-polarized interfacial superlattices in marginally twisted hexagonal boron nitride. Nat. Commun. 12, 347 (2021).
Zhang, Y., Liu, T. & Fu, L. Electronic structures, charge transfer, and charge order in twisted transition metal dichalcogenide bilayers. Phys. Rev. B 103, 155142 (2021).
Wang, Y. et al. Tunable ferroelectricity in hBN intercalated twisted double-layer graphene. Preprint at https://arxiv.org/abs/2102.12398 (2021).
Muechler, L., Alexandradinata, A., Neupert, T. & Car, R. Topological nonsymmorphic metals from band inversion. Phys. Rev. X 6, 041069 (2016).
Acknowledgements
We thank Qiong Ma, Kenji Yasuda, Felix Lüpke, Dacen Waters, and Evan Telford for fruitful discussions. B.M.H., S.C.d.l.B., and Q.C. were supported by the Department of Energy under the Early Career award program (DE-SC0018115) for all aspects of this project. Yang Gao and D.X. are supported by the Department of Energy, Basic Energy Sciences, Grant No. DE-SC0012509. J.Y. and D.G.M. acknowledge support from the U.S. Department of Energy (U.S.-DOE), Office of Science - Basic Energy Sciences (BES), Materials Sciences and Engineering Division. W.Z. acknowledges support from the National Key Research and Development Program of China (2019YFA0210004).
Author information
Authors and Affiliations
Contributions
S.C.d.l.B. and B.M.H. conceived the project. S.C.B. and Q.C. fabricated the devices and performed the measurements, with fabrication support from V.S.B. Yang Gao and Yuan Gao performed the theoretical calculations under the supervision of W.Z. and D.X. J.Y. and D.G.M. grew the bulk WTe2 crystals. S.C.d.l.B., Q.C., and B.M.H. analyzed and interpreted the data and wrote the manuscript with contributions from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
de la Barrera, S.C., Cao, Q., Gao, Y. et al. Direct measurement of ferroelectric polarization in a tunable semimetal. Nat Commun 12, 5298 (2021). https://doi.org/10.1038/s41467-021-25587-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-021-25587-3
This article is cited by
-
Coupled ferroelectricity and superconductivity in bilayer Td-MoTe2
Nature (2023)
-
Orbital multiferroicity in pentalayer rhombohedral graphene
Nature (2023)
-
Interfacial ferroelectricity in rhombohedral-stacked bilayer transition metal dichalcogenides
Nature Nanotechnology (2022)
-
Non-synchronous bulk photovoltaic effect in two-dimensional interlayer-sliding ferroelectrics
npj Computational Materials (2022)
-
Giant ferroelectric polarization in a bilayer graphene heterostructure
Nature Communications (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.