Abstract
Electrical manipulation of magnetization could be an essential function for energy-efficient spintronics technology. A magnetic topological insulator, possessing a magnetically gapped surface state with spin-polarized electrons, not only exhibits exotic topological phases relevant to the quantum anomalous Hall state but also enables the electrical control of its magnetic state at the surface. Here, we demonstrate efficient current-induced switching of the surface ferromagnetism in hetero-bilayers consisting of the topological insulator (Bi1-xSbx)2Te3 and the ferromagnetic insulator Cr2Ge2Te6, where the proximity-induced ferromagnetic surface states play two roles: efficient charge-to-spin current conversion and emergence of large anomalous Hall effect. The sign reversal of the surface ferromagnetic states with current injection is clearly observed, accompanying the nearly full magnetization reversal in the adjacent insulating Cr2Ge2Te6 layer of an optimal thickness range. The present results may facilitate an electrical control of dissipationless topological-current circuits.
Similar content being viewed by others
Introduction
Spin-polarized surface electronic states of three-dimensional topological insulators (TIs) offer novel physical properties, being potentially applicable to future low-power-consumption electronics/spintronics and topological quantum computation1. One of the representative features is the emergence of anomalous Hall conductance in the gapped surface state when magnetization perpendicular to the surface is induced by the incorporation of magnetic elements or proximity coupling with a ferromagnetic (FM) layer on the TI2,3,4,5,6,7,8. This magnetic TI exhibits exotic magnetic insulating phases, such as a quantum anomalous Hall (QAH) insulator and an axion insulator. In particular, the QAH state provides a research arena based on the non-dissipative chiral edge conduction, whose direction is determined by the magnetization direction. By controlling the magnetization direction, a topological invariant of the Chern number C in the QAH conditions can correspondingly be controlled, as manifested by the switching of the conduction direction (C = 1 or −1) and on/off switching (|C| = 1 or 0) of the chiral edge channel4,5,6.
Besides external magnetic fields, an electric current injection can also control the magnetization directions. Owing to the spin-momentum locking of the electrons at the TI surface state9,10, the flow of electrons produces nonequilibrium spin accumulation, which exerts spin torques on a FM layer adjacent to the TI surface11,12. The current-induced switching of the perpendicular magnetization in the FM layer in conjunction with a TI has been demonstrated in a highly efficient manner with lower critical current densities than those in FM-metal/heavy-metal heterostructures13,14,15,16,17,18,19. Moreover, the spin accumulation may also enable the electrical manipulation of the TI surface ferromagnetism that originates from the FM proximity coupling at the FM-layer/TI interface. However, in the intensively studied FM-metal/TI systems, the current at the TI surface state is mostly shunted by the FM-metal layer. Hence, the replacement of the FM metal with a FM insulator (FMI) would be effective to control the magnetization with lower electric current20,21,22, which is also required to observe the QAH states with nontrivial Chern numbers8. It has been demonstrated that the magnetization in the FMI layer can be partly switched by current excitation in magnetically doped TI13,14 and FMI/TI heterostructures20, but the switching ratio of the magnetization has been limited to <50%. Such a low ratio, which likely originates from the inhomogeneous nature of doping-induced ferromagnetism and weak magnetic coupling at the interface of FMI/TI, respectively, results in the formation of multi-domain states after each switching operation and causes difficulty in the control of the topological invariant. Therefore, it is expected that the choice of a suitable FMI with high crystallinity and strong interfacial coupling can achieve a high switching ratio.
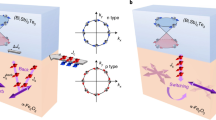
Here, we demonstrate the nearly full magnetization switching in an all-telluride-based intrinsic FMI (Cr2Ge2Te6; CGT)/TI heterostructure (Fig. 1a). The layered FMI compound of CGT23,24 has recently been found to provide strong proximity coupling with the TI surface state, as exemplified by the observation of large anomalous Hall effect (AHE) originating from a prominent Berry curvature near the exchange gap formed in the proximity-coupled surface state25 (Fig. 1b). Since the efficient spin transfer to the FMI layer requires strong couplings with well-ordered interfaces between the TI surface state and the magnetic moments in the FMI layer26,27, the CGT/TI heterostructures may be of great advantage for highly efficient switching. Through the optimization of the CGT thickness for the switching ratio and efficiency, the magnetization of the CGT layer can be almost fully switched by the in-plane current injection on the TI layer as low as 2–4 A cm−1 in the CGT thickness range of 3–5 nm (corresponding to four to seven CGT monolayers). Furthermore, high switching current efficiencies irrespective of the Fermi level (EF) indicate that the spin torques are dominantly generated from the TI surface state rather than its bulk state. Such a FMI/TI heterostructure is highly suitable for the current-induced control of the surface ferromagnetism of TI.
a Schematic illustration of magnetization switching in Cr2Ge2Te6/(Bi1-xSbx)2Te3 (CGT/BST) bilayer. The magnetization M in CGT is reversed by current injection along x-direction (Jx) under an in-plane magnetic field (Hx) parallel to Jx via the spin–orbit torque from the spin (σ)-polarized surface state conduction. The perpendicular component of the magnetization is probed by AHE. b Schematic illustration of the electronic structures of surface states gapped by the magnetic proximity coupling. The magnetization switching in the CGT layer corresponds to the switching of the sign of the magnetic gap at the proximity-coupled surface states. c Out-of-plane magnetic field μ0Hz dependence of the magnetization Mz in the CGT (12 nm)/BST (x = 0.5, 6 nm) bilayer at 2 K. d Optical microscope image of a Hall bar device with an illustration of the measurement setup. e Longitudinal resistance Rxx as a function of temperature T for the CGT single-layer (12 nm; green), BST(x = 0.5) single-layer (6 nm; purple), and CGT (12 nm)/BST (x = 0.5, 6 nm) bilayer (red) films.
Results
Heterostructure and device characterization
We grew CGT/(Bi1-xSbx)2Te3 (CGT/BST) bilayer films (Fig. 1a) on InP(111) substrates by molecular beam epitaxy (MBE; see “Methods” section). To examine the EF position dependence, the Bi/Sb ratio (x) was systematically controlled in the BST layer. The previous study25 on the CGT/BST heterostructure, which was prepared in the same way as in the present study, has proven the high crystal quality and well-ordered, sharp interfaces due to van der Waals bonding, as well as negligible atomic interdiffusion by cooperatively using x-ray diffraction, depth-sensitive x-ray/neutron reflectometry, and cross-sectional scanning transmission electron microscopy/energy-dispersive x-ray spectroscopy (Supplementary Note 1). The magnetization of a MBE-grown CGT (12 nm)/BST (6 nm) structure (the Curie temperature: TC ~ 80 K) at a temperature of T = 2 K (Fig. 1c) shows the hysteresis loop with the out-of-plane easy axis, which is nearly identical with the property of the MBE-grown CGT single layer itself24. For the electrical transport measurements and magnetization switching characterizations, we fabricated Hall bars with 10 μm in width (Fig. 1d). Figure 1e shows the T dependence of sheet resistance Rxx for the CGT single-layer (12 nm), BST (x = 0.5, 6 nm) single-layer, and CGT (12 nm)/BST (x = 0.5, 6 nm) bilayer films. Whereas the CGT single-layer film exhibits a highly insulating behavior (>1 MΩ <50 K), the resistance of the CGT/BST bilayer film is comparable to that of the BST single-layer film. The electrical conductivity in the TI layer depends on the EF, yet the dominant conduction channel is still in the TI layer for all the heterostructures (Supplementary Fig. 2).
Proximity-induced anomalous Hall effect
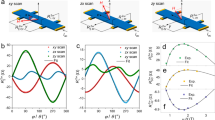
We first discuss the CGT layer thickness (tCGT) dependence of the AHE for six samples of the CGT/BST(x = 0.5, 6 nm) bilayers. This composition x = 0.5 is the optimum value in the bilayer system for the observation of the largest AHE as discussed later. We note that the optimum x value is different from the charge-neutrality point value (x ~ 0.8) in BST single-layer films3,4,5,8 to suppress a possible charge transfer at the CGT/BST interface. Figure 2a shows the Hall resistance Ryx of typical four samples, exhibiting comparable AHE responses. In addition, the coercive field of the hysteresis loops is consistent with the magnetization hysteresis curve of the CGT layer (tCGT = 12 nm; Fig. 1c). The value of remnant anomalous Hall resistance RyxAH at zero magnetic field (~250 Ω) is nearly constant irrespective of tCGT (2.9 ≤ tCGT ≤ 12 nm), reflecting that the Berry curvature generated at the magnetically gapped TI surface state.
a μ0Hz dependence of the Hall resistance Ryx in the CGT/BST (x = 0.5, 6 nm) bilayer devices with various CGT thickness (tCGT = 2.9, 4.6, 5.8, and 12 nm) at 2 K. RyxAH is the remnant value of Ryx at μ0Hz = 0 T. b Magnetization switching with current pulses Jx, as tracked by the variation of Hall resistance Ryx (left axis) of the CGT (tCGT = 2.9 nm)/BST (x = 0.5, 6 nm) device under in-plane magnetic fields μ0Hx = +0.1 T (blue) and −0.1 T (red) at 2 K. Right axis is the switching ratio defined as Ryx/RyxAH. The broken lines represent Ryx/RyxAH = 1 for the full switching of magnetization. c Magnetization switching in the CGT/BST devices with various tCGT (=2.9, 4.6, 5.8, 8.1, and 12 nm) under μ0Hx = −0.1 T. d, e The tCGT dependence of the switching current Jxsw (left axis) and the switching ratio of Ryxsw/RyxAH (right axis) (d), and the coefficient ξ [=2eμ0MsHctCGT/(ħjxsw)] representing the efficiency of current-induced magnetization reversal (e). Note that ξ for tCGT = 8 and 12 nm increases up to ~1.4 nm−1, where the Joule heating seemingly improves the efficiency. The inset to e shows the tCGT dependence of the product of the coercive field μ0Hc and the spontaneous magnetization Ms. The horizontal error bars represent the film roughness determined by x-ray reflectivity measurements. The vertical ones for d and e represent the measurement uncertainties. The red broken (d) and solid (e) lines are the guides to the eyes.
Current-induced magnetization switching
In Fig. 2b, we present the current-induced magnetization switching of the CGT (2.9 nm)/BST (x = 0.5, 6 nm) bilayer. To perform the perpendicular magnetization switching28,29,30, 100-μs duration current pulses (Jx) were injected under in-plane magnetic fields (μ0Hx = ±0.1 T) much smaller than the anisotropy fields (HK ~ 0.9 T; see Supplementary Note 2). After every current pulse injection, Ryx was measured with a much smaller probe current of Jx = 10 μA to elucidate the magnetization direction of the CGT layer and the proximity-induced surface ferromagnetism via Hall measurement. As shown in Fig. 2b, when the amplitude of the injection current pulse exceeds the switching threshold current Jxsw ~ 2.0 mA, the sign of the Hall resistance is reversed. As expected from the antidamping-like spin–orbit torque switching28,29,30, the switching polarity is reversed when we reverse the direction of the in-plane magnetic field (Hx (blue curve) → −Hx (red curve)). The change of Hall resistance Ryxsw = |Ryx(Jx → +0, Hx < 0) − Ryx(Jx → −0, Hx < 0)|/2 = 200 Ω is comparable to the remnant Hall resistance RyxAH = 228 Ω, in which the ratio Ryxsw/RyxAH ~ 0.88 corresponds to the reversed magnetization ratio in the CGT. This large value means the realization of the nearly full magnetization switching, resulting in the switching of the FM surface states and the topological spin structures in the surface state (Fig. 1b).
Cr2Ge2Te6 thickness dependence of the magnetization switching
Having established the current-induced magnetization switching, we next examine the tCGT dependence of Jxsw to evaluate the switching ratio and efficiency. As shown in Fig. 2c, the Jxsw increases with increasing tCGT, while the switching volume fraction measured by Ryxsw/RyxAH decreases. A linear increase of Jxsw in Fig. 2d saturates at 6 < tCGT < 12 nm. Furthermore, Ryxsw/RyxAH drastically decreases at 6 < tCGT < 12 nm. The tCGT-linear relation for tCGT < 6 nm is attributed to that the spin torques required for the switching linearly increase with the spontaneous magnetization per sample area, MstCGT. On the other hand, for tCGT = 8 and 12 nm, the large MstCGT requires a large Jxsw, resulting in a thermal instability of magnetization due to the Joule heating which perhaps not only assists the switching, but also reduces Ryxsw/RyxAH due to the multi-domain formation (see Supplementary Note 4 for the estimation of heating effect).
To quantitatively compare the tCGT-dependent switching behavior, we define a coefficient parameter ξ = 2eμ0MsHctCGT/(ħjxsw) (refs. 12,30). Here e is the elementary charge, ħ is the reduced Planck’s constant, μ0Hc is the coercive field of the CGT layer, and jxsw = Jxsw/W (W = 10 μm: the width of the Hall bars) is the sheet switching current density. This coefficient describes how efficiently the surface spin accumulation is absorbed in the FMI layer via the charge-to-spin current conversion (Js = ξ jx), where the resulting antidamping-like spin–orbit torque magnitude (in a unit moment) is described by τAD = ħJs/(2eMstCGT). Since the switching is likely driven by current-induced domain nucleation and subsequent domain wall motion processes30 due to the large-scale Hall bar (Fig. 1d) compared with the magnetic domain size (see Supplementary Fig. 6 for the observed magnetic domain structure), the spin–orbit torque acts as the domain wall depinning field, which is assigned to the coercive field of the CGT layer (μ0Hc) at the switching threshold current injection30. As shown in Fig. 2e, we observe ξ for tCGT < 6 nm takes a roughly constant value of ξ ~ 1.0 nm−1, being consistent with the linear Jxsw–tCGT relation shown in Fig. 2d. Furthermore, this value obtained without any correction of current distribution in the TI layer owing to the insulating FM layer is comparable to that derived in the previous spin–torque FM resonance experiment on the FM-metal layer in proximity to the TI BST12. Incidentally, to more quantitatively estimate the spin-charge conversion efficiency, we tried to utilize the prevailing method to use the second harmonic Hall measurement31,32. The analysis based on it, however, gave an unphysically large efficiency value, i.e., ~1000 nm−1. Such a large nonlinear Hall signal is perhaps due to a magnon scattering of the spin-momentum-locked surface Dirac electrons of TI14 (see Supplementary Note 6 for details).
Fermi-level position dependence of the switching efficiency
We turn to EF position dependence of magnetization switching for the evaluation of the surface state contribution. Figure 3a shows the AHE in CGT (3.5 nm)/BST (6 nm) having different x with the fixed thicknesses of each layer. Judging from the sign of the ordinary Hall term at high magnetic fields, the dominant carrier type is systematically controlled with increasing x from electron type for x = 0, 0.3, and 0.5 to hole type for x = 0.7 and 1. At the x = 0.5, RyxAH is maximized, indicating that EF is closest to the magnetic gap where the Berry curvature contribution is the largest2,3. In addition, the sign of AHE is reversed, while reducing its magnitude, from positive to negative for 0.5 < x < 0.7, reflecting the sign change of the Berry curvature. Such a sign change has been observed also in magnetically doped TI heterostructures33, where an additional anomalous Hall conductivity with the opposite sign is generated from Rashba-split bulk valence bands due to the broken inversion symmetry by the heterostructure. Hence, both the surface and bulk states are likely to contribute to the electrical conduction in p-type BST (x = 0.7 and 1). We thus observed several characteristic behaviors in the current-induced magnetization switching in Fig. 3b. First, its switching polarity change (Ryx versus Jx) between x = 0.5 and 0.7 coincides with that of AHE (Ryx versus Hz), indicating the same spin–torque directions irrespective of the carrier types. Second, Ryxsw/RyxAH is nearly constant against the variation of x. Third, Jxsw, on the other hand, varies with x, where the minimum 2 mA is observed for x = 0.5, while both x = 0 and 1 require the larger Jxsw of 5 mA, implying the switching efficiency depends on the EF.
a AHE showing Ryx versus μ0Hz for CGT (tCGT = 3.5 nm)/(Bi1-xSbx)2Te3 (6 nm) with various x (=0, 0.3, 0.5, 0.7, and 1). The samples are categorized to be n-type (x = 0, 0.3, and 0.5) or p-type (x = 0.7 and 1). b Magnetization switching under in-plane magnetic fields μ0Hx = −0.1 T. c Sb content (x) dependence of ξ (solid red circle) and RyxAH (solid blue circle). Simplified schematics of the band structures with the EF positions are depicted above the panel. The vertical error bars represent the measurement uncertainties. d Illustration of the energy dispersion of the TI surface state. e, f The spin accumulation driven by a shift of the Fermi surface (top) and the difference in the Fermi distribution for electrons δf under an electric field (Ex; bottom) for the n-type (e) and p-type (f) TI.
To clarify the relationship between the switching efficiency and the EF position, we compare the x dependence of ξ and RyxAH in Fig. 3c. Both are enhanced at x = 0.5 in which the bulk conduction is mostly suppressed with EF being close to the magnetic gap of the TI surface state. The large RyxAH (solid blue circles) comes from the prominent Berry curvature generated around the gap. Moreover, the broad peak of ξ x = 0.5 implies that the spin-polarized surface state plays a dominant role in the magnetization switching because the bulk contribution present at x = 0 and 1 does not increase ξ. Thus, EF tuning is advantageous for improving the switching efficiency, as well as for maximizing the AHE while the almost full magnetization switching is accomplished for all the samples (Fig. 3b). Note that despite the surface state is magnetically gapped by the proximity coupling with the FMI layer, suppression of the charge-to-spin current conversion efficiency in the nearly charge-neutral samples is not observed, possibly due to still unprecise tuning of the Fermi level into the middle of the exchange gap or to the spatially inhomogeneous gap opening.
Discussions
Finally, we argue that these magnetization switching features are consistent with current-induced dynamics of the spin-momentum-locked TI surface state as the dominant source of the spin torques11,12,13,14,15,16,17,18,19. First, possibilities for the source of the spin torques other than the topological surface states may include the spin Hall effect from the bulk bands34 and the inversion symmetry breaking of the hetero-interface, where a vertical electric field at the interface can induce Rashba spin splitting in the bulk states35. However, these scenarios cannot account for the present observation that the efficiency is increased when the EF is within the TI bulk gap rather than in the bulk states. Second, the accumulated spin direction is irrespective of the carrier types. The Fermi circle with the opposite spin helicities for n-type and p-type (Fig. 3c) suggests that the direction of spins would be opposite for the carrier types. However, in consideration of the Fermi surface response to the electric current or field, the accumulated spin directions are the same as elucidated in the following. When an electric field (+Ex) is applied, the shift of the Fermi circle has the same direction irrespective of the carrier type: \(k_x \to k_x - \frac{{eE_x\tau }}{\hbar } = k_x - \delta k\). Then, as shown in Fig. 3e, if EF > EDP = 0, the Fermi circle shift increases the population of electrons for the −kx branch, while reducing that for the +kx branch. On the other hand, if EF < EDP, the Fermi circle shift reduces the population of electrons for the −kx branch, while increasing that for the +kx branch (Fig. 3f). Hence, the increased spin populations for the n-type and p-type have the same direction \({\hat{\mathbf{\sigma }}} = + {\hat{\mathbf{y}}}\) under +Ex. Since the antidamping effective field, which is given by \({\hat{\mathbf{\sigma }}} \times {\hat{\mathbf{m}}}\), does not depend on the momentum but the spin direction, the spin–orbit torque direction is not changed by the carrier types. Third, the magnetization switching direction itself is consistent with the spin-momentum-locked surface state of BST by using a macrospin model29: the effective field originating from antidamping torques \({\mathbf{\tau }}_{{\mathrm{AD}}} = \tau _{{\mathrm{AD}}}\left( {{\hat{\mathbf{m}}} \times \left( {{\hat{\mathbf{\sigma }}} \times {\hat{\mathbf{m}}}} \right)} \right)\), where τAD > 0, is described by \({\mathbf{H}}_{{\mathrm{AD}}} = (\tau _{{\mathrm{AD}}}/\mu _0){\hat{\mathbf{\sigma }}} \times {\hat{\mathbf{m}}}\). Suppose the magnetic moment is \({\hat{\mathbf{m}}} = + {\hat{\mathbf{z}}}\), the effective field \({\mathbf{H}}_{{\mathrm{AD}}}|| + {\hat{\mathbf{x}}}\) under the current pulse of jx > 0 (i.e., \({\hat{\mathbf{\sigma }}} = + {\hat{\mathbf{y}}}\) due to the spin-momentum locking nature of BST), which rotates the magnetization via magnetic damping as shown in Fig. 1a. When the magnetic moment is slightly tilted to \(+ {\hat{\mathbf{x}}}\) (\(- {\hat{\mathbf{x}}}\)) by an in-plane magnetic field, the \({\hat{\mathbf{m}}} = - {\hat{\mathbf{z}}}\) (\(+ {\hat{\mathbf{z}}}\)) state is favored under the current pulse injection, which well describes the observed behaviors shown in Fig. 2b. Note that once the reversed magnetic domains are nucleated by the above macrospin model mechanism, the domains may be expanded toward the single domain state more efficiently than the macrospin rotation29,30.
In conclusion, by the current excitation at the TI surface state, we have successfully demonstrated the nearly full switching of the FM surface states in the TI layer proximity-coupled to the insulating CGT. The systematic CGT thickness tCGT dependence of the switching current reveals that the tCGT should be <6 nm to realize the full switching. Our results indicate the compatibility of the large proximity-induced AHE and the efficient magnetization switching, paving a way to electrically manipulate topological quantum states. For instance, thick FMI/TI/thin FMI sandwich structures8,25 with different switching currents (Fig. 2d) for the top and bottom TI surfaces would allow selective controls of the magnetic layers, namely electrical switching between the QAH insulator (parallel magnetization) and axion insulator (antiparallel magnetization) states5,6. Whereas such topological states are surface insulating and would not directly contribute to the spin–torque generation, an additional electrostatic gating capability to control the Fermi level36 or a current-driven breakdown of the QAH state during a current pulse injection37,38,39 could retrieve the spin-polarized surface transport. This allows linking the spintronic functionalities to the topological quantum states, opening a new avenue to unprecedented control of dissipationless topological current devices.
Methods
Film growth and characterization
The CGT (top)/BST (bottom) films were grown by MBE on semi-insulating InP substrates using standard Knudsen cells in a MBE chamber under a vacuum condition (~1 × 10−7 Pa). The growth temperatures for the CGT layers and the BST layers were 180 °C and 200 °C, respectively24,25. Taking out the films from the MBE chamber, the AlOx capping layer (~5 nm) was immediately deposited by atomic layer deposition at room temperature. The crystal structures and thicknesses of the respective layers were confirmed by x-ray diffraction and reflectivity measurements, respectively25.
Device fabrication
The films were patterned into Hall bars with 10 μm in width and 30 μm in length by using photolithography and chemical etching, with H2O2–H3PO4–H2O and HCl–H2O mixtures. The electrodes were made of Ti (5 nm)/Au (45 nm) deposited by electron beam evaporation.
Electrical transport measurements
The electrical transport measurements of the Hall bars were performed in a Quantum Design PPMS (2 K, 9 T). The current value for the resistivity and Hall effect measurements was 10 μA.
Magnetization switching measurements
The pulse current with varying pulse amplitudes between ±8 mA was injected into the Hall bar by using a current source (Keithley Model 6221). After the injection, the Hall resistance was subsequently measured with a voltmeter (Keithley Model 2182A) under a low probe current of 10 μA.
Data availability
All relevant data within this paper are available from the authors upon reasonable request. Source data are provided with this paper.
References
Hasan, M. Z. & Kane, C. L. Colloquium: topological insulators. Rev. Mod. Phys. 82, 3045 (2010).
Tokura, Y., Yasuda, K. & Tsukazaki, A. Magnetic topological insulators. Nat. Rev. Phys. 1, 126–143 (2019).
Chang, C.-Z. et al. Experimental observation of the quantum anomalous Hall effect in a magnetic topological insulator. Science 340, 167–170 (2013).
Kou, X. et al. Metal-to-insulator switching in quantum anomalous Hall states. Nat. Commun. 6, 8474 (2015).
Mogi, M. et al. A magnetic heterostructure of topological insulators as a candidate for an axion insulator. Nat. Mater. 16, 516–521 (2017).
Liu, C. et al. Robust axion insulator and Chern insulator phases in a two-dimensional antiferromagnetic topological insulator. Nat. Mater. 19, 522–527 (2020).
Deng, Y. et al. Quantum anomalous Hall effect in intrinsic magnetic topological insulator MnBi2Te4. Science 367, 895–900 (2020).
Watanabe, R. et al. Quantum anomalous Hall effect driven by magnetic proximity coupling in all-telluride based heterostructure. Appl. Phys. Lett. 115, 182401 (2019).
Burkov, A. A. & Hawthorn D. G. Spin and charge transport on the surface of a topological insulator. Phys. Rev. Lett. 105, 066802 (2010).
Pesin, D. & MacDonald, A. H. Spintronics and pseudospintronics in graphene and topological insulators. Nat. Mater. 11, 409–416 (2012).
Mellnik, A. R. et al. Spin-transfer torque generated by a topological insulator. Nature 511, 449–451 (2014).
Kondou, K. et al. Fermi-level-dependent charge-to-spin current conversion by Dirac surface states of topological insulators. Nat. Phys. 12, 1027–1031 (2016).
Fan, Y. et al. Magnetization switching through giant spin-orbit torque in a magnetically doped topological insulator heterostructure. Nat. Mater. 13, 699–704 (2014).
Yasuda, K. et al. Current-nonlinear Hall effect and spin-orbit torque magnetization switching in a magnetic topological insulator. Phys. Rev. Lett. 119, 137204 (2017).
Han, J. et al. Room-temperature spin-orbit torque switching induced by a topological insulator. Phys. Rev. Lett. 119, 077702 (2017).
Wang, Y. et al. Room temperature magnetization switching in topological insulator-ferromagnet heterostructures by spin-orbit torques. Nat. Commun. 8, 1364 (2017).
Dc, M. et al. Room-temperature high spin–orbit torque due to quantum confinement in sputtered BixSe(1–x) films. Nat. Mater. 17, 800–807 (2018).
Khang, N. H., Khang, D., Ueda, Y. & Hai, P. M. A conductive topological insulator with large spin Hall effect for ultralow power spin-orbit torque switching. Nat. Mater. 17, 808–813 (2018).
Wu, H. et al. Room-temperature spin-orbit torque from topological surface states. Phys. Rev. Lett. 123, 207205 (2019).
Li, P. et al. Magnetization switching using topological surface states. Sci. Adv. 5, eaaw3415 (2019).
Avci, C. O. et al. Current-induced switching in a magnetic insulator. Nat. Mater. 16, 309–314 (2017).
Shao, Q. et al. Role of dimensional crossover on spin-orbit torque efficiency in magnetic insulator thin films. Nat. Commun. 9, 3612 (2018).
Gong, C. et al. Discovery of intrinsic ferromagnetism in two-dimensional van der Waals crystals. Nature 546, 265–269 (2017).
Mogi, M. et al. Ferromagnetic insulator Cr2Ge2Te6 thin films with perpendicular remanence. APL Mater. 6, 091104 (2018).
Mogi, M. et al. Large anomalous Hall effect in topological insulators with proximitized ferromagnetic insulators. Phys. Rev. Lett. 123, 016804 (2019).
Zhang, W., Han, W., Jiang, X., Yang, S.-H. & Parkin, S. S. P. Role of transparency of platinum-ferromagnet interfaces in determining the intrinsic magnitude of the spin Hall effect. Nat. Phys. 11, 496–502 (2015).
Chen, W., Sigrist, M., Sinova, J. & Manske, D. Minimal model of spin-transfer torque and spin pumping caused by the spin Hall effect. Phys. Rev. Lett. 115, 217203 (2015).
Miron, I. M. et al. Perpendicular switching of a single ferromagnetic layer induced by in-plane current injection. Nature 476, 189–193 (2011).
Liu, L., Lee, O. J., Gudmundsen, T. J., Ralph, D. C. & Buhrman, R. A. Current-induced switching of perpendicularly magnetized magnetic layers using spin torque from the spin Hall effect. Phys. Rev. Lett. 109, 096602 (2012).
Lee, Q. J. et al. Central role of domain wall depinning for perpendicular magnetization switching driven by spin torque from the spin Hall effect. Phys. Rev. B 89, 024418 (2014).
Kim, J. et al. Layer thickness dependence of the current-induced effective field vector in Ta|CoFeB|MgO. Nat. Mater. 12, 240–245 (2013).
Garello, K. et al. Symmetry and magnitude of spin-orbit torques in ferromagnetic heterostructures. Nat. Nanotech. 8, 587–593 (2013).
Yasuda, K. et al. Geometric Hall effects in topological insulator heterostructures. Nat. Phys. 12, 555–559 (2016).
Liu, Y. et al. Direct visualization of current-induced spin accumulation in topological insulators. Nat. Commun. 9, 2492 (2018).
Yang, F. et al. Switching of charge-current-induced spin polarization in the topological insulator BiSbTeSe2. Phys. Rev. B 94, 075304 (2016).
Chiba, T. & Komine, T. Voltage-driven magnetization switching via Dirac magnetic anisotropy and spin-orbit torque in topological-insulator-based magnetic heterostructures. Phys. Rev. Appl. 14, 034031 (2020).
Fox, E. J. et al. Part-per-million quantization and current-induced breakdown of the quantum anomalous Hall effect. Phys. Rev. B 98, 075145 (2018).
Kawamura, M. et al. Current-driven instability of the quantum anomalous Hall effect in ferromagnetic topological insulators. Phys. Rev. Lett. 119, 016803 (2017).
Kawamura, M. et al. Current scaling of the topological quantum phase transition between a quantum anomalous Hall insulator and a trivial insulator. Phys. Rev. B 102, 041301(R) (2020).
Acknowledgements
This research project was partly supported by JSPS/MEXT Grant-in-Aid for Scientific Research (Nos. 15H05853, 15H05867, 17J03179, 18H04229, and 18H01155) and JST CREST (No. JPMJCR16F1).
Author information
Authors and Affiliations
Contributions
M.M. and K.Y. planned the experiments. M.M. and R.F. fabricated the samples with the help of R.Y., A.T., M. Kawamura, K.S.T., and M. Kawasaki. M.M. and R.F. performed the transport and magnetization switching measurements, and analysed the data with the help of K.Y. and R.Y. K.Y. performed the magnetic force microscopy measurements. M.M., K.Y., R.F., N.O., A.T., and Y.T. discussed the results. M.M., A.T., and Y.T. wrote the manuscript with inputs from all the other authors. Y.T. and M. Kawasaki conceived and supervised the project.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks Can Onur Avci, Yabin Fan and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Source data
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Mogi, M., Yasuda, K., Fujimura, R. et al. Current-induced switching of proximity-induced ferromagnetic surface states in a topological insulator. Nat Commun 12, 1404 (2021). https://doi.org/10.1038/s41467-021-21672-9
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-021-21672-9
This article is cited by
-
Unraveling the dynamics of magnetization in topological insulator-ferromagnet heterostructures via spin-orbit torque
npj Spintronics (2024)
-
Electrical switching of the edge current chirality in quantum anomalous Hall insulators
Nature Materials (2024)
-
Visualizing thickness-dependent magnetic textures in few-layer Cr2Ge2Te6
Communications Materials (2024)
-
Investigation of the mechanism of the anomalous Hall effects in Cr2Te3/(BiSb)2(TeSe)3 heterostructure
Nano Convergence (2023)
-
Magnetotransport and ARPES studies of the topological insulators Sb2Te3 and Bi2Te3 grown by MOCVD on large-area Si substrates
Scientific Reports (2022)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.