Abstract
Cross-relaxation among neighboring emitters normally causes self-quenching and limits the brightness of luminescence. However, in nanomaterials, cross-relaxation could be well-controlled and employed for increasing the luminescence efficiency at specific wavelengths. Here we report that cross-relaxation can modulate both the brightness of single upconversion nanoparticles and the threshold to reach population inversion, and both are critical factors in producing the ultra-low threshold lasing emissions in a micro cavity laser. By homogenously coating a 5-μm cavity with a single layer of nanoparticles, we demonstrate that doping Tm3+ ions at 2% can facilitate the electron accumulation at the intermediate state of 3H4 level and efficiently decrease the lasing threshold by more than one order of magnitude. As a result, we demonstrate up-converted lasing emissions with an ultralow threshold of continuous-wave excitation of ~150 W/cm2 achieved at room temperature. A single nanoparticle can lase with a full width at half-maximum as narrow as ~0.45 nm.
Similar content being viewed by others
Introduction
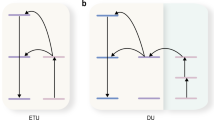
Cross-relaxation (CR) is an energy transfer process between a pair of nearby emitters, where one at a higher excited energy state transfers its photon energy to the other one at a lower excited state or ground state, so that both can simultaneously reach their intermediate excited states1. This process can be often observed in lanthanides ions those featured with a series of sophisticated intermediate energy levels and each with long lifetimes2,3. CR accumulates energy in the intermediate excited states, as a conducive process to the formation of population inversion that is essential for the generation of lasing emissions. This explains why lanthanide ions doped laser crystals and glass are ascendant gain medium4,5,6. Though the concentration of lanthanide ions determines the extent of CR and thereby the threshold for lasing emission generation, in the bulk materials the doping uniformity and dynamic range of lanthanide ions are hard to control, e.g., typically 0.25–1% for Tm3+ in YVO4, MgWO4 or YAG laser crystal (<8 × 1020 ions/cm3)7,8,9. High doping in bulk crystal and glass often leads to the non-uniform distribution of the ions and localized excessive CR, which results in luminescence quenching, self-heating, and high laser threshold.
At the nanoscale, lanthanides doped upconversion nanoparticles (UCNPs) and wet chemistry synthesis strategies have been well developed, so that their size, shape and doping concentrations can be precisely controlled with high accuracies in both morphological and optical uniformities10,11,12,13,14,15. In a typical NaYF4 host, the doping percentage of a lanthanide ion can be arbitrarily tuned between zero and its unity to form the “alloyed” nanocrystals16,17. This allows several recent studies on the role of doping concentrations and the degree of CR between ions in increasing the efficiency of optical depletion in super-resolution microscopy and facilitating the generation of near-infrared and single band emissions2,18,19,20,21,22. Encouragingly, singly Tm3+ doped UCNPs with energy looping effect have been recently reported as an efficient gain medium to generate room-temperature continuous-wave (CW) pumped laser with a threshold of ~14 kW/cm2 23.
Here we study the role of CR (doping concentration), excitation power density and the size of a single nanoparticle, and to identify the optimum conditions and key characteristics to set a single nanoparticle to lase. We find that the brightness of UCNPs and its efficiency in establishing the population inversion as the gain medium as well as the quality factors of a typical whispering-gallery-mode (WGM) cavity can be strongly affected by the doping concentration, excitation power density and the size of UCNPs when coating a typical polystyrene microsphere by a single layer of self-assembled UCNPs or only a single UCNP. In the classical Yb3+-Tm3+ co-doped energy transfer system, we find that the higher doping concentration of Tm3+ facilities higher probability for CR, but requires higher laser excitation intensity to produce sufficiently bright upconversion emissions. Moreover, though the larger size of UCNPs typically produces stronger emissions, it generates a stronger scattering loss by the increased roughness of the cavity surface. Since the scattering scales with the 6th power of the particles’ diameter, reducing the number of particles will significantly reduce the loses of the cavities24,25. This effect can significantly reduce the efficiency for micro cavity lasers coated by a single layer of self-assembled UCNPs, but has negligible influence on the lasing emissions from a single UCNP.
Results and discussion
Population inversion property of CR nanoparticles
TEM images displayed the Fig. 1a of a series of morphology-uniform UCNPs at different Tm3+ concentrations show the monodispersity of our nanocrystals. Within the volume of ~7240 nm3, in a 24 nm UCNP (Supplementary Fig. 1), we tune the amount of the Tm3+ ions from ~200 to ~8000, 0.2 mol.% to 8 mol.% correspondingly. As shown in Fig. 1b, when the concentration of Tm3+ is fixed at low level, the population inversion purely depends on the high power density of the pumping laser, as the activation of the emitters is mainly through the sensitization of Yb3+ (Supplementary Fig. 2) and there is negligible CR due to the long distance between the lanthanide ions, e.g., the averaged large distance can be calculated as ~3.3 nm at the doping concentration of 0.2 mol.%. The dependence on the high power excitation can be alleviated by CRs, (1G4, 3H6 → 3H5, 3H4), (1G4, 3H6 → 3F2,3, 3F4), (3F2,3, 3F4 → 3H4, 3H5)18,26, which facilitates the establishment of population inversion at intermediate levels, such as 3H4. Besides, highly Tm3+ doped UCNPs leads to the decrease in the Yb3+-Tm3+ distance and increase in the Yb3+-Tm3+ energy transfer efficiency. But this strategy, as illustrated in Fig. 1d, could lead to a quenching of the overall upconversion emissions, when doping too many emitters. As shown in Supplementary Fig. 4, the decrease in the Yb3+ lifetime values and the trend of energy transfer efficiency saturation appeared at the very large Tm3+ concentrations (e.g., >8 mol.%), indicate the possible Tm3+-Yb3+ energy back transfer27. To alleviate the concentration quenching and energy back transfer effects in the highly doped UCNPs, high excitation power density is required to pump the significant amount of the ground level Tm3+ emitters and Yb3+ sensitizers. These suggest the existence of a sweet spot of optimum Tm3+ doping concentration and excitation pumping power for the low threshold establishment of population inversion.
We first characterize the upconversion emission spectra of the series of 24 nm UCNPs (Fig. 1a). Diversified spectral distributions are observed when varying the Tm3+ concentrations (Fig. 2a), suggesting the concentration-dependent CR effect. The proportions of the emissions from 3H4 to 3H6 transition show an upward tendency with the increase of Tm3+ concentration, which indicates the CR induced efficient energy accumulation (Supplementary Fig. 6). At the low concentration range, the energy, sensitized by and transferred from Yb3+, is distributed onto all the excited states, including 1D2, 1G4, and 3H4 (Supplementary Fig. 7). When the Tm3+ concentration increases from 1 mol.% to 2 mol.%, the peak intensities associated with the 1G4 level decrease, while the 3H4 initiated transition intensity increases. Figure 2b displays the quantitative intensity evolution and ratios as the function of Tm3+ concentration. The intensity at 802 nm (3H4 → 3H6) transition, the intensity ratios of 802 nm/473 nm and 802 nm/645 nm reach their peak values at 2 mol.% Tm3+ due to the CR induced population enrichment at the excitation power density of 100 W/cm2. Further increase in the Tm3+ concentration, e.g. 4 mol% and 8 mol.%, depopulates the 3H4 level with decreased intensity at 802 nm due to the excessive CR induced energy loss and the possible back energy transfer from Tm3+ to Yb3+. As CR involves different energy levels between a pair of nearby emitters, the population distribution of each energy level is strongly dependent on the excitation power density, so is the CR dynamics2,28. And Fig. 2c shows the power-dependent population of 3H4 level by analyzing the peak intensity at 802 nm. The 2 mol.% Tm3+ doped sample shows the highest intensities of 802 nm emissions within the excitation power density range of 0.6–150 W/cm2 due to the CR effect. Above the power density of 10 W/cm2, the low doping samples are saturated while the highly doped samples exhibit an accelerated growth trend of the emission intensities, indicating the non-linear transition dynamics caused by the power-dependent and concentration-dependent CR effect.
a Comparison emission intensities and spectra of UCNPs doped with 20 mol.% Yb3+ and x mol.% Tm3+ (x = 0.2, 0.5, 1, 2, 4, 8). b Comparison emission intensities at the characteristic wavelengths and the intensity ratios of 802 nm/473 nm and 802 nm/645 nm. Both results displayed in (a, b) were acquired at the excitation power density of 100 W/cm2. c Power dependent emission intensity evaluation at 802 nm. d SEM image of a 5-μm polystyrene micro cavity coated with a single layer of self-assembled UCNPs. e Cross-sectional TEM image of micro cavity showing the distribution of UCNPs on the surface. f Excitation and detection scheme (see Supplementary Fig. 5 for the details of a purpose-built imaging system).
Characterization of microlasers coated with UCNPs
Figure 2d, e and Supplementary Fig. 8 present the typical images from SEM and cross-sectional TEM measurements of a 5-μm polystyrene microsphere, showing the single layer of 2 mol.% Tm3+ doped UCNPs on the surface of the cavity through the electrostatic force assembly (see zeta potential data in Supplementary Fig. 9). Microsphere confines the light through total internal reflection and supports both transverse electric (TE) and transverse magnetic (TM) propagating modes with high quality (Q) factor, e.g., the Q factor for a 750 μm fused-silica microsphere could even reach ~109 29,30,31,32. When nanoparticles are coated onto the surface, the increased roughness induces scattering losses and reduces Q factors33, therefore homogeneous coatings avoid aggregations and minimize scattering.
Figure 3a shows the multi-mode lasing emissions under the pumping power of 10 kW/cm2 and simulated optical modes of the cavity at 800 nm (see the details of Finite difference time domain simulations in Supplementary information and Supplementary Figs. 10 and 11). The 5-μm microsphere with a refractive index of ~1.59 leads to an effective coupling of upconversion emissions within the cavity modes. The full width at half-maximum (FWHM) of the sharp lasing peaks is calculated to be 0.54 nm using the Lorentz function fitting. According to Q ≈ λ0/Δλ, where λ0 and Δλ are the centre wavelength and FWHM of the peak profile, the quality factor Q is estimated as ~1500. Both the narrow FWHM and high Q benefit from the small size of ca. 24 nm of the UCNPs and the homogenous coating of a single layer of UCNPs (as the contrast, see the results of uneven coating in Supplementary Fig. 12). The inset in Fig. 3a and Supplementary Fig. 10 indicate that the jacinth-shaded region with high field intensity overlaps the coating layer of UCNPs.
a Numerical simulations of resonance spectrum and experimental emission spectrum. The inset shows a numerical simulation of the electrical-field distributions at 800 nm within a major plane. b Emission polarization angle-dependent intensities of the lasing peaks. c Power dependent upconversion emission spectra showing the gradual appearance of lasing peaks. d The pumping power-dependent plots of emission intensities and spectral linewidth narrowing, showing the onset of upconversion lasing emissions. e Tm3+concentration-dependent thresholds for the onsets of upconversion lasing emissions.
The characterized polarization properties of both the TE and TM mode emissions exhibiting linear polarization with orthogonal periodicity as observed by the different polarization angles collection in Fig. 3b. The free spectral range (FSR) between two adjacent TE modes or TM modes fit well with the theoretical value (\({\Delta}\lambda _{{\mathrm{FSR}}} = \frac{{\lambda ^2}}{{2\pi {\mathrm{R}}}}\), R is the radius of microcavity). Noting that the slight difference of experimental lasing peaks is attributed to the imperceptible slight variations of each cavity, as the mode position will move more than 8 nm when the size of this cavity changes for only 1% (Supplementary Fig. 11).
The transition from below, near, and at the threshold lasing emissions are seen in Fig. 3c when increasing the pumping powers to the onset of lasing emissions with the characteristic sharp and regularly spaced emission peaks. The narrow peaks that indicative of increased coherence and laser emissions are emerging above the threshold at pump intensities of 120 W/cm2 for the laser mode at 805 nm. Both the slopes for the intensity and linewidth curves (Fig. 3d) display a non-linear change characteristic at the ultra-low pumping threshold of ~150 W/cm2 achieved for the homogeneously coated 2 mol.% Tm3+ doped UCNPs sample. Figure 3e shows 2 mol.% Tm3+ doped UCNPs as the most effective gain medium as a result of the CR induced efficient population inversion at low pumping power (for the comparison characterizations of other UCNPs samples see Supplementary Fig. 13). Note that the accurate laser threshold should be ideally determined by using the absorbed pump power instead of incident pump power. We reason that the actual laser thresholds should be much lower than the current values once the absorbed power could be measured, though it’s inaccessible in our experimental conditions.
Although the larger nanoparticles often produce stronger brightness, the size-induced scattering losses cannot be neglected. As shown in Fig. 4a–d, with the increase of nanoparticle size, from 24 nm, to 43 nm and 51 nm the FWHM in the lasing spectra increases from ~0.5 nm to 0.8 nm and ~1.2 nm, with the increased spontaneous emission background (Fig. 4e). Though larger sized UCNPs are brighter due to the reduced degree of surface-quenching, by testing more than 20 micro cavity lasers, the Q factor reduces from ~1900 to ~700 with the increased size of UCNPs (Fig. 4f). The reduction in the Q factor indicates the increased size-induced scattering loss. When the size of UCNPs becomes too large, the catchment area by the evanescent field of micro cavity modes also reduces (Supplementary Figs. 10 and 14).
Characterization of single nanocrystal lasing
Figure 5a, b further show that lanthanide ions doped single UCNPs are optically uniform and sufficiently bright, as there are averaged 23640 ± 832 photon counts per 50 milliseconds from single nanoparticles under a confocal microscoy, which are suitable for single particle lasing in Fig. 5c. We demonstrate that CR assisted population inversion in single UCNPs as a highly efficient gain medium, which can be widely used in any photonics cavities for single nanoparticle lasers. Single 43 nm NaYF4: 20 mol.% Yb3+-2 mol.% Tm3+ UCNPs can lase at 808 nm with the sharp peaks and narrow FWHM of ~0.45 nm once in a cavity (Fig. 5c–f), which preserves the Q-factors of the cavity since scattering losses are reduced to the minimum. Due to the reduced scattering from single UCNP, it allows to use of bigger particles and increase the total gain, compared to the uniform 24 nm single layer coating.
a TEM image of 43 nm monodispersed NaYF4:20%Yb3+,2%Tm3+ nanocrystals. b Point-scanning confocal microscopic image of single UCNPs (in pseudocolour) and their brightness distribution at an excitation power density of 100 kW/cm2. c Power dependent upconversion emission spectra showing the gradual appearance of lasing emission peaks at 808 nm. d The pumping power-dependent plots of emission intensities and spectral linewidth narrowing (peak@808 nm), showing the onset of single UCNP’s lasing emissions. e, f Wide field image (d) and SEM image (e) of a single UCNP in a micro cavity.
Conclusion
We have achieved ultra-low threshold lasing by controlling CR in an upconversion energy transfer system and thereby the easy establishment of population inversion in single UCNPs. By using the doping-concentration-optimized UCNPs as the gain medium and single layer coating of monodispersed UCNPs on the cavity with minimized scattering loss, strong absorption and high efficiencies for both upconversion and population inversion establishment have been achieved. Employing a 5-μm cavity and homogenous coating architecture, we have achieved lasing emissions with a threshold of ~150 W/cm2, nearly two orders of magnitude lower than the recently reported benchmark value of 14 kW/cm2. We have further verified that the size of single nanoparticle does not affect the Q factor of micro cavity lasing, with an FWHM of ~0.45 nm achieved for upconversion lasing emissions from a single 43 nm nanoparticle. This study suggests great potential to using the concentration tunable UCNPs as an efficient gain medium for room temperature CW microscale and nanoscale lasers. The single upconversion nanocrystal lasing offers prospects to achieve NIR pumped anti-Stokes nanolaser platform for a variety of practical applications, such as intracellular tagging and imaging34. A further reduction of the threshold values and mode volumes is achievable by optimizing the combination strategy between the gain medium and cavity, and selecting higher-Q cavities, e.g., plasmonic nanocavity, Spaser, hyperbolic metacavity, photonic crystals or photonic topological insulator array cavities35,36. The CR mediated lanthanides energy transfer system might be embedded in semiconducting matrixes to achieve electrical pumped emission and further expand low threshold CW lasers via electrical pumping, which are more compatible with current standard technologies37.
Methods
Upconversion nanoparticles synthesis
The uniform oleic-acid-capped nanoparticles (β-NaYF4:20%Yb, x%Tm3+) were synthesized by the coprecipitation method. A typical procedure is as follows: YCl3·6H2O (0.8-x mmol), YbCl3·6H2O (0.2 mmol) and TmCl3·6H2O (x mmol) were added into a 50 mL three-necked flask containing 6 mL oleic acid (OA) and 15 mL 1-octadecene (ODE). The mixture was first heated to 160 °C under argon for 30 min to form a transparent solution and remove residual water. The solution was cooled down to room temperature, and 10 mL of a methanol solution containing NaOH (2.5 mmol) and NH4F (4 mmol) was slowly dropped into the flask and stirred for 30 min. Then, the solution was heated to 70–80 °C and maintained for 30 min to evaporate methanol. Subsequently, the solution was heated to 300 °C and maintained for 1 h under argon atmosphere. After cooling down to room temperature, the resulting products were precipitated by ethanol and collected by centrifugation at 6000 rpm for 5 min. The precipitate was then purified with ethanol three times, and finally dispersed in cyclohexane for further use.
Surface modification for water-soluble upconversion nanoparticles
In a typical procedure, the nanoparticles were first precipitated by adding ethanol (2.0 mL) to a cyclohexane colloidal solution of the OA coated hydrophobic β-NaYF4:20%Yb, x%Tm3+ nanoparticles (1 mL, 10 mg/mL) and then collected by centrifugation at 6000 rpm for 5 min. The obtained nanoparticles were re-dispersed in a mixed solution of ethanol (1 mL) and HCl (1 mL, 2 M) upon sonication for 5 min. The ligand free nanoparticles were collected by centrifugation at 14680 rpm for 10 min and re-dispersed in deionized water (1 mL).
Fabrication of upconverting microlasers
Upconverting microlasers were produced through a solution process. In a typical procedure, 10 μL PS microbeads (10% solids) were first dispersed in 1000 μL DI water upon sonication for 5 min, then 7 μL ligand free water-soluble UCNPs (8 mg/mL) were mixed together with PS microbeads upon sonication. Then, this mixed solution was kept for a gentle shake (750 rpm) for 2 h before centrifugation. These UCNPs coated microbeads were further washed with ethanol and water for three times, and finally were re-dispersed in water. Noting that, the coated surface and thickness can be controlled precisely by tuning the concentration ratio of microbeads and nanoparticles.
Characterization and simulation
TEM
Transmission electron microscope (TEM) measurements were performed using a FEI Tecnai T20 instrument with an operating voltage of 120 kV. The samples for TEM analysis were prepared by placing a drop of a dilute suspension of nanoparticles onto carbon-coated copper grids. For the cross-sectional TEM images, we first embedded the single layer UCNPs coated microcavity in epoxy (353ND), and then cut the microspheres using Leica microtome to get ultrathin slices.
SEM
The morphology of microcavity coated with UCNPs was characterized via scanning electron microscope (SEM) imaging (Supra 55VP, Zeiss) operated at 3.00 kV.
Zeta potential
The measurement of zeta potential (ζ-potential) was carried out by Zetasizer (Malvern Panalytical).
Confocal microscopy and spectroscopy
The inverted confocal optical system was built on a sample scanning configuration employing a 3D piezo stage. A single-mode fiber-coupled 980 nm diode laser was used as the excitation source and was focused onto the sample through an oil-immersion objective lens (UPlanAPO, Olympus; ×100, NA = 1.4). The emission from sample was collected by the same objective lens then refocused into an optical fiber that has a core size matching with system Airy disk. A single photon counting avalanche diode (SPAD) detector was connected to the collection optical fiber to detect the emission intensity. The spectra were measured with a fiber-coupled spectrometer (Andor) with a grating of 1200 grooves/mm (resolution: 0.21 nm, data collection step: ~0.04 nm). And the polarization performance of this upconverting microlaser was characterized by rotating a λ/2 plate @808 nm while cooperated with a polarizer (620–1000 nm).
Finite difference time domain simulations
Numerical simulations of our microcavity were performed using Lumerical FDTD solutions. For the simulation of the electrical-field distributions and resonance spectrum, the perfectly matched layer (PML) was set as the boundary condition with a simulation region of 6 μm × 6 μm × 6 μm; the monitored wavelength was 400–1000 nm; the meshes order was 10 nm; the light type was a dipole source. The simulated resonance spectrum and electric field distribution of microcavity were calculated separately, as shown in Fig. 3a. The cavity modes in Supplementary Fig. 11 were achieved while changing the sizes of the microcavity. The electrical-field distributions at ~800 nm mode was plotted along the diameter.
Data availability
All the relevant data are available from the corresponding authors upon reasonable request.
References
Auzel, F. Upconversion and anti-stokes processes with f and d ions in solids. Chem. Rev. 104, 139–173 (2004).
Liu, Y. et al. Amplified stimulated emission in upconversion nanoparticles for super-resolution nanoscopy. Nature 543, 229–233 (2017).
Zhao, J. et al. Single-nanocrystal sensitivity achieved by enhanced upconversion luminescence. Nat. Nanotechnol. 8, 729 (2013).
Jackson, S. D. Cross relaxation and energy transfer upconversion processes relevant to the functioning of 2 μm Tm3+-doped silica fibre lasers. Opt. Commun. 230, 197–203 (2004).
Hebert, T., Wannemacher, R., Macfarlane, R. & Lenth, W. Blue continuously pumped upconversion lasing in Tm: YLiF4. Appl. Phys. Lett. 60, 2592–2594 (1992).
Chen, X., Sun, T. & Wang, F. Lanthanide-based luminescent materials for waveguide and lasing. Chem.- Asian J. 15, 21–33 (2020).
Ermeneux, F. et al. Growth and fluorescence properties of Tm3+ doped YVO4 and Y2O3 single crystals. Optical Mater. 8, 83–90 (1997).
Zhang, L. et al. Crystal growth, optical spectroscopy and laser action of Tm3+-doped monoclinic magnesium tungstate. Opt. Express 25, 3682–3693 (2017).
Wen, S. et al. Advances in highly doped upconversion nanoparticles. Nat. Commun. 9, 2415 (2018).
Lu, Y. Q. et al. Tunable lifetime multiplexing using luminescent nanocrystals. Nat. Photonics 8, 33–37 (2014).
Wang, F. et al. Simultaneous phase and size control of upconversion nanocrystals through lanthanide doping. Nature 463, 1061 (2010).
Wang, F. et al. Tuning upconversion through energy migration in core-shell nanoparticles. Nat. Mater. 10, 968 (2011).
Liu, D. et al. Three-dimensional controlled growth of monodisperse sub-50 nm heterogeneous nanocrystals. Nat. Commun. 7, 10254 (2016).
Gargas, D. J. et al. Engineering bright sub-10-nm upconverting nanocrystals for single-molecule imaging. Nat. Nanotechnol. 9, 300–305 (2014).
Liu, Q. et al. Single upconversion nanoparticle imaging at sub-10 W cm−2 irradiance. Nat. Photonics 12, 548–553 (2018).
Tian, B. et al. Low irradiance multiphoton imaging with alloyed lanthanide nanocrystals. Nat. Commun. 9, 3082 (2018).
Gu, Y. et al. High-sensitivity imaging of time-domain near-infrared light transducer. Nat. Photonics 13, 525–531 (2019).
Zhan, Q. et al. Achieving high-efficiency emission depletion nanoscopy by employing cross relaxation in upconversion nanoparticles. Nat. Commun. 8, 1058 (2017).
Wei, W. et al. Cross relaxation induced pure red upconversion in activator-and sensitizer-rich lanthanide nanoparticles. Chem. Mater. 26, 5183–5186 (2014).
Chen, Q. S. et al. Confining excitation energy in Er3+-sensitized upconversion nanocrystals through Tm3+-mediated transient energy trapping. Angew. Chem. Int. Ed. 56, 7605–7609 (2017).
Zhong, Y. et al. Boosting the down-shifting luminescence of rare-earth nanocrystals for biological imaging beyond 1500 nm. Nat. Commun. 8, 737 (2017).
Wang, J., Wang, F., Wang, C., Liu, Z. & Liu, X. Single-band upconversion emission in lanthanide-doped KMnF3 nanocrystals. Angew. Chem. Int. Ed. 50, 10369–10372 (2011).
Fernandez-Bravo, A. et al. Continuous-wave upconverting nanoparticle microlasers. Nat. Nanotechnol. 13, 572 (2018).
Hulst, H. C. & van de Hulst, H. C. Light scattering by small particles (Courier Corporation, 1981).
Bohren, C. F. & Huffman, D. R. Absorption and scattering of light by small particles (1983).
Misiak, M., Prorok, K., Cichy, B., Bednarkiewicz, A. & Stręk, W. Thulium concentration quenching in the up-converting α-Tm3+/Yb3+ NaYF4 colloidal nanocrystals. Optical Mater. 35, 1124–1128 (2013).
Yu, D. et al. Understanding and tuning blue-to-near-infrared photon cutting by the Tm3+/Yb3+ couple. Light.: Sci. Appl. 9, 107 (2020).
Chen, C. et al. Multi-photon near-infrared emission saturation nanoscopy using upconversion nanoparticles. Nat. Commun. 9, 3290 (2018).
Vahala, K. J. Optical microcavities. Nature 424, 839 (2003).
He, L., Özdemir, Ş. K. & Yang, L. Whispering gallery microcavity lasers. Laser Photonics Rev. 7, 60–82 (2013).
Jiang, X. F., Qavi, A. J., Huang, S. H. & Yang, L. Whispering-gallery sensors. Matter 3, 371–392 (2020).
Gorodetsky, M. L., Savchenkov, A. A. & Ilchenko, V. S. Ultimate Q of optical microsphere resonators. Opt. Lett. 21, 453–455 (1996).
Boriskina, S. V., Benson, T. M., Sewell, P. & Nosich, A. I. Spectral shift and Q change of circular and square-shaped optical microcavity modes due to periodic sidewall surface roughness. J. Optical Soc. Am. B 21, 1792–1796 (2004).
Martino, N. et al. Wavelength-encoded laser particles for massively multiplexed cell tagging. Nat. Photonics 13, 720–727 (2019).
Jeong, K. Y. et al. Recent progress in nanolaser technology. Adv Mater, 202001996 (2020).
Garcia-Vidal, F. J. & Moreno, E. Lasers go nano. Nature 461, 604–605 (2009).
Ma, R.-M. & Oulton, R. F. Applications of nanolasers. Nat. Nanotechnol. 14, 12–22 (2019).
Acknowledgements
This project is primarily supported by ARC Discovery Early Career Researcher Award Scheme (J.Z., DE180100669), China Scholarship Council Scholarships (Y.S.: No. 201706120322), National Natural Science Foundation of China (NSFC, 61729501), Major International (Regional) Joint Research Project of NSFC (51720105015), Science and Technology Innovation Commission of Shenzhen (KQTD20170810110913065), and Australia China Science and Research Fund Joint Research Centre for Point-of-Care Testing (ACSRF658277, SQ2017YFGH001190).
Author information
Authors and Affiliations
Contributions
Y.S., J.Z., and D.J. conceived the project and designed experiments. J.Z., C.Y., L.J., and D.J. supervised the project. Y.S. and Y.C. synthesized the upconversion nanoparticles. Y.S., F.W., and J.Z. conducted the optical setup. Y.S. fabricated the microlasers and performed the characterizations. Y.S., J.Z., A.F., and D.J. analyzed the results, prepared the figures and wrote the paper. All authors contributed to discussion, interpreting the data and the writing.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks the anonymous reviewers for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Shang, Y., Zhou, J., Cai, Y. et al. Low threshold lasing emissions from a single upconversion nanocrystal. Nat Commun 11, 6156 (2020). https://doi.org/10.1038/s41467-020-19797-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-020-19797-4
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.