Abstract
The photoionization of xenon atoms in the 70–100 eV range reveals several fascinating physical phenomena such as a giant resonance induced by the dynamic rearrangement of the electron cloud after photon absorption, an anomalous branching ratio between intermediate Xe+ states separated by the spin-orbit interaction and multiple Auger decay processes. These phenomena have been studied in the past, using in particular synchrotron radiation, but without access to real-time dynamics. Here, we study the dynamics of Xe 4d photoionization on its natural time scale combining attosecond interferometry and coincidence spectroscopy. A time-frequency analysis of the involved transitions allows us to identify two interfering ionization mechanisms: the broad giant dipole resonance with a fast decay time less than 50 as, and a narrow resonance at threshold induced by spin-flip transitions, with much longer decay times of several hundred as. Our results provide insight into the complex electron-spin dynamics of photo-induced phenomena.
Similar content being viewed by others
Introduction
The absorption of X-rays by matter has been used since more than a century ago to understand the structure of its fundamental constituents1. An X-ray photon absorbed by an atom triggers multiple electron dynamics. The emission of an electron from an inner shell is accompanied by ultrafast rearrangement of the electronic cloud, which simultaneously modifies the potential seen by the electron, sometimes leading to resonances in the emission spectrum. An outer-shell electron may fill an inner hole, while another electron is emitted, a process called Auger decay2. Finally, the electron spin may be affected by the magnetic field induced by the ultrafast orbital motion, giving rise to spin flip, which is forbidden for purely electric dipole transitions. All of this complex hole or electron motion occurs on a rapid time scale, in the attosecond (1 as = 10−18 s) range.
The interaction of xenon atoms with photons in the 70–100 eV range illustrates many aspects of the electron dynamics sketched above. Collective many-electron effects in the 4d shell3,4,5,6 lead to a broad “giant dipole” resonance in the photoionization cross-section, which is maximum at 100 eV7,8. Photoionization is accompanied by Auger decay, involving the 5s and 5p shells, leading to the formation of Xe2+ ions9 (see Fig. 1). Relativistic (spin-orbit) effects can be observed at threshold (in the 70–75 eV region), with, in particular, an anomalous branching ratio between the 2D5/2 and 2D3/2 final states of the ion, separated by a spin-orbit splitting of 2 eV10,11,12.
Attosecond pulses produced by high-order harmonic generation in gases13,14 enable measuring ultrafast electron dynamics, as shown in a series of seminal experiments15,16,17,18,19,20,21,22. Temporal information is obtained by pump/probe techniques combining attosecond pulses and a synchronized laser field. The reconstruction of attosecond beating by interference of two-photon transition (RABBIT) technique23, based on interferometry, allows the determination of the photoionization spectral amplitude in the complex plane. The temporal dynamics is then obtained by Fourier transform or more generally by time-frequency analysis16,24. This technique has been successfully used to measure photoionization time delays, due to electron propagation in the potential following the absorption of an extreme ultraviolet (XUV) photon. Most of these studies25,26,27,28, however, have concentrated on relatively simple systems, ionized from the valence shells.
In this work, we present measurements of photoionization time delays in the Xe 4d shell for different ionic states 4d−1(2D5/2) and 4d−1(2D3/2), denoted 4d5/2 and 4d3/2 in the following (see Fig. 1b). Auger-photoelectron coincidence spectroscopy is used to disentangle electrons from different photoionization and decay channels29. The RABBIT interferometric technique allows the extraction of a phase, or a time (or group) delay, from the photoelectron spectra. At high photon energy (between 80 and 100 eV), both 4d5/2 and 4d3/2 photoelectrons are emitted with the same positive time delay. Close to the 4d-ionization threshold (75–80 eV), the measured time delays differ by more than 100 as. Supported by relativistic random phase approximation (RRPA) theoretical calculations30, we show that this difference is due to the interference of the broad giant dipole resonance with a narrow threshold resonance due to relativistic spin-orbit effects.
Results
The experiments were performed with attosecond pulse trains generated in neon by a femtosecond Ti:sapphire laser system, covering a spectral range from the 4d ionization threshold to the maximum of the giant dipole resonance (see Methods for details). A small fraction of the infrared (IR) laser beam was used as a probe with a variable time delay. The XUV and IR pulses were focused into Xe gas and the created electrons were detected by an electron spectrometer.
Photoionization to different ionic states followed by Auger decay produces a complex electron spectrum, with two sets of photoelectrons separated by 2 eV (see Fig. 1). Single Auger decay from Xe+ (e.g., 4d5/2) to Xe2+ (e.g., 5s−15p−1) leads to electrons at kinetic energies equal to the difference between intermediate and final state energies, spanning from 8.3 eV to 36.4 eV31 and thus overlapping with the photoelectrons ionized by 75–100 eV photons32. Figure 2 shows XUV-only (a) and XUV+IR (b) two-dimensional coincidence maps. For a given final state of Xe2+, Auger electrons detected in coincidence with photoelectrons contribute to a stripe with discrete spots related to absorption of different harmonics (with odd orders 53 to 63 in the figure), or absorption of harmonics and absorption or emission of an IR photon (sidebands 54 to 62). In addition, weak signals due to absorption or emission of an IR photon by the Auger electron, are observed (see, e.g., the difference between the blue and red curves in Fig. 2e at 9.8 eV). This coincidence technique requires long acquisition times, but allows us to disentangle unambiguously the 4d5/2 and 4d3/2 photoelectrons by the energy of the Auger electron.
The coincidence map using a XUV field only and b XUV+IR fields. The number of measured two-electron pairs is indicated by the color code. The spots with constant slow-electron energy and variable fast-electron energy correspond to photoelectrons created by absorption of consecutive harmonics (labeled as H57-H61 in (a) as an example) and a given Auger electron (e.g., 4d−1(2D3/2) → 5s−2(1S0)). Photoelectrons corresponding to absorption or emission of an additional IR photon (sidebands, labeled as S58 and S60 as an example) appear in b. The projection on the fast electron energy axis c and d is the sum of the signal with slow electron energy from 10 eV to 10.4 eV, i.e., the photoelectrons in coincidence with 4d−1(2D3/2) → 5s−2(1S0) Auger electrons. The projection on the slow electron energy axis e shows the sum of the signal for the different Auger processes indicated on the right, with (red) and without (blue) IR field. A RABBIT energy scheme is indicated at the top of d.
Each sideband arises from the interference between two quantum paths as illustrated at the top of Fig. 2(d). The sideband signal oscillates as a function of the delay τ between the attosecond pulse train and the probe IR field, according to,
where A and B are constants, ω is the IR frequency and ϕ is a phase offset, which can be extracted by fitting with a cosine function. The phase offset ϕ divided by the oscillation frequency (2ω) can be written as the sum of two delays, τXUV + τA. The first one is the group delay of the attosecond pulses, while the second, called atomic time delay, arises from the two-photon ionization process. As shown in previous work33,34 and as discussed in more details in the Supplementary Fig. 1, the variation of the atomic time delay τA, as a function of XUV photon energy or between two spin-orbit split final states, reflects, to a large extent, one-photon ionization dynamics. To remove the influence of τXUV in our time delay measurements, we alternate experiments in Xe and Ne, and measure the time delay difference. Atomic time delays in Ne 2p can be measured and calculated with good accuracy. They are very small in the energy range considered33, so that the time delay differences between Xe and Ne are, to a very good approximation, absolute time delays in Xe (see Supplementary Fig. 2). The sidebands corresponding to the same photoelectron but different Auger final state are found to oscillate in phase within our error bar, which allows us to average the time delays over the different Auger decay channels.
Figure 3 presents the absolute atomic delays for 4d5/2 (a) and 4d3/2 (b) as a function of photon energy. We also present theoretical calculations obtained by solving the Dirac equation in RRPA (see Methods). At high photon energy (>80 eV), both 4d5/2 and 4d3/2 exhibit very similar positive time delay, about 40 as at 80 eV, slightly decreasing with energy. At low photon energy (<80 eV), the delays show a rapid variation with energy, opposite for the two final states. Theory and experiment are in good agreement at high photon energy. Although the agreement in the threshold region is not as good, the main features of the experiment are reproduced. Our theoretical calculations are in excellent agreement with the predictions of Mandal and coworkers35,36.
Absolute atomic time delays for a 4d3/2 and b 4d5/2; c Difference between these delays. The experimental data are given in red dots. The black lines indicate the results of two-photon RRPA calculations. The estimation of the error bars is the standard error of the weighted mean, detailed in Methods section. In d, the calculated branching ratio of the 4d5/2 over 4d3/2 cross sections is shown in black. The experimental data in blue dots is from ref. 37.
Figure 3c shows the difference between 4d3/2 and 4d5/2 time delays. This difference is close to zero at high energy but jumps to more than 100 as at 75 eV. Unfortunately, we could not reliably extract time delays below 75 eV, due to the low cross section and the overlap with double Auger electrons below 5 eV kinetic energy. The RRPA calculation predicts a strong decrease of the time delay difference towards the threshold. It also shows a strong deviation of the branching ratio between 4d5/2 and 4d3/2 cross sections from the statistical prediction in the same energy range, reproducing well experimental results37,38 (see Fig. 3d).
Discussion
To understand the underlying physics behind the variation of the time delays, we examine the behavior of the RRPA transition matrix elements involved in Xe 4d single photon ionization. In the energy range considered in this work, photoionization is dominated by the transitions from the 4d shell to continuum f states, which we denote 4d → ϵf in the following. The contribution from 4d → ϵp transitions is one order of magnitude smaller in this energy region, as shown in the Supplementary Fig. 3. The asymptotic phase for a given channel is the sum of the Coulomb phase and a phase due to the short-range potential. The Coulomb phase is removed in the phases displayed in Fig. 4, as well as in the calculation of the time delays, in order to focus on the short range effects (see Supplementary Fig. 1). Figure 4a shows that photoionization is dominated by 4d3/2 → ϵf5/2 and 4d5/2 → ϵf7/2, especially at high photon energy, in the region of the giant dipole resonance. In the threshold region, the 4d5/2 → ϵf5/2 channel contributes significantly. This transition is accompanied by a spin flip, which points out the role of the spin-orbit interaction. The phases and time delays for the three channels (Fig. 4b,c) coincide above 80 eV photon energy, showing the first half of a π phase variation across the giant dipole resonance with a time delay of ~40 as. Below 80 eV, the three quantities plotted in Fig. 4a–c show a strong, oscillating, channel dependence, indicating a quantum interference phenomenon.
a, d Modulus, b, e phase and c, f time delay of the transition matrix element Di(E) as a function of photon energy for the coupled channels (a–c) 4d3/2 → ϵf5/2 (blue), 4d5/2 → ϵf5/2 (red), 4d5/2 → ϵf7/2 (brown) and eigenchannels (d–f) 1P (black), 3P (magenta), 3D (orange), extracted from ref. 12. We exclude the Coulomb phase-shift in b, e and c, f. A π phase shift has been added to the phase of 4d5/2 → ϵf5/2 for better comparison. The time delay is the energy derivative of the phase.
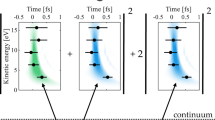
The dynamics behind this effect can be unraveled by calculating the Wigner representation24,39, defined as the Fourier transform of the auto-correlation function of the transition matrix elements, Di(E), i denoting the channel, and E the electron kinetic energy.
where ℏ is the reduced Planck constant. The results are shown in Fig. 5a for the 4d5/2 → ϵf5/2 channel (see Supplementary Fig. 4, for the other two channels). All three channels show similar features. (i) A broad resonance with a maximum around 100 eV and a short decay of a few tens of attosecond, which can be interpreted as the giant dipole resonance; (ii) A sharp resonance at low energy, around 75 eV, with a long decay of a few hundreds of attoseconds; (iii) Interferences between these resonances, leading to rapid oscillations of the Wigner distribution.
To interpret the sharp spectral feature at 75 eV, we utilize the theoretical analysis performed in the seminal work of Cheng and Johnson12. Using a multichannel quantum defect theory approach40, results obtained within RRPA, similar to those of the present work, were analyzed and eigenchannel solutions were extracted. These eigenchannel solutions are completely decoupled from each other and can be used as a basis to describe coupled-channel transitions. They are labeled using the closest corresponding LS-coupled channel [(d9f) and (d9p)1P, 3P, 3D], where in all these cases it is only the J = 1 states that can be populated from the xenon ground state by one-photon absorption. Neglecting the weak contribution of the 4d → ϵp transitions, the 4d → ϵf transitions are superpositions of (d9f) 1P, 3P and 3D eigenchannels (In the following, we drop the d9f label).
Figure 4d–f present the modulus, phase and time delay of these three eigenchannels. The behavior of the three curves is much simpler than those in Fig. 4a–c. For each eigenchannel, a single resonance feature can be identified, with a peak for the modulus and time delay and a π phase variation across the resonance. While the broad feature, maximum at 100 eV, obviously represents the giant dipole resonance (1S → 1P), the narrow peaks at 75 and 76 eV exist because of the spin-orbit interaction that enables singlet to triplet mixing. The maximum of the time delay varies from a few tens (1P) to a few hundreds (3D and 3P) of attoseconds, in agreement with the results in Fig. 5a.
The difference in time delays can be further interpreted by examining the effective potential experienced by the escaping f photoelectron. We represent in Fig. 5b a mean-field average potential (red), as well as the potential modified by 1S → 1P dipole polarization (screening) effects (black), which are included in the random phase approximation with exchange (RPAE) approach. These effects lead to an effective high and narrow potential barrier and therefore to a broad resonance, with a maximum at high energy, and a short decay time (see black dashed line). In contrast, an electron emitted in the triplet channels does not feel these dipole polarization effects and sees essentially the potential indicated in red, with a relatively low barrier only due to angular momentum and a long decay time (red dashed line). The time delay is directly related to the resonance lifetime, being equal to it at the maximum of the resonance41. Figure 5b even suggests that the increase of the temporal width of the broad resonance in Fig. 5a towards low energy might be due to the influence of the long tail of the screened potential (black).
The rapid variation of the amplitude, phase and delays of the three 4d → ϵf channels (Fig. 4a–c) at threshold can therefore be interpreted as a quantum interference effect between the “direct” dipole-allowed 1S → 1P transition and the spin-orbit-induced 1S → 3P, 3D transitions, which have similar amplitudes in this region. This interference explains the difference in time delays for 4d3/2 and 4d5/2, as well as the anomalous branching ratio (Fig. 3c, d).
In conclusion, we have measured photoionization time delays in Xe using attosecond interferometry, giving us high temporal resolution, and coincidence spectroscopy, which allows us to avoid spectral congestion and to obtain a high spectral resolution. These time delays are positive and similar for the two spin-orbit split Xe+ states over a large energy range (up to 100 eV photon energy), except at threshold (75 eV) where they differ by 100 as. With the help of RRPA calculations for one- and two-photon ionization, we attribute this difference to the interference of several channels coupled by the spin-orbit interaction. A time-frequency analysis of the dominant transition matrix elements, allows us to unravel two main ionization processes, with very different time and energy scales: the broad giant dipole resonance, dominated by the 1S to 1P transition and the narrow resonances due to the 1S to 3P and 3D transitions, which are enabled by the spin-orbit interaction. While the former takes place over a few tens of attoseconds, the latter, involving spin flip, occurs over several hundreds of attoseconds. Our experimental approach, which adds temporal information to traditional spectral studies, provides increased understanding of the complex electron dynamics taking place in Xe 4d photoionization. Finally, shape resonances are ubiquitous in nature and the methods developed in this work should be useful to investigate the electronic properties of a variety of molecular systems (see recent work in N242 and CH3I43).
Methods
Experimental method
The experiments were performed with 40-fs long pulses, centered at 800 nm with 1-kHz repetition rate from a Ti:sapphire femtosecond laser system. The laser was focused into a 6-mm long gas cell filled with Ne to generate high-order harmonics. A 200-nm thick Zr filter was used to filter out the infrared (IR) and most of the harmonics below the Xe 4d threshold (67.5 eV for 4d5/2). The filtered harmonics thus span the 4d ionization region from the threshold to the maximum of the giant dipole resonance (100 eV). A small fraction (30%) of the IR beam, split-off before generation, is used as a probe with a variable time delay. The XUV and IR pulses were focused in an effusive Xe gas jet. The electrons were detected by a magnetic bottle electron spectrometer, which combines high collection efficiency and high spectral resolution up to E/ΔE ~ 80.
Data analysis
Each data point in Fig. 3 is the arithmetic mean weighted with the uncertainty estimated from the cosine fitting to Eq. (1). In each measurement, we average the time delays of electron pairs corresponding to the same photoelectron but different Auger decay. For N measurements yielding N data points: τ1, τ2, …, τN with corresponding uncertainties: σ1, σ2, …, σN, the weighted average can be calculated as:
where \({w}_{i}=1/{\sigma }_{i}^{2}\) is the weight. The uncertainty for each measurement is estimated from the fit of the RABBIT oscillation to a cosine function. The uncertainty on the time delay difference, τA − τB, can be expressed as:
The error bars of the experimental results indicate the standard error of the weighted mean and can be calculated as:
where ωs = ∑ωi.
Theoretical method
Theoretical calculations consisted in calculating one-photon and two-photon matrix elements within lowest-order perturbation theory for the radiation fields, using wavefunctions obtained by solving the Dirac equation, and including electron correlation effects within the RPAE for one-photon XUV photoionizaton.
The complex-valued two-photon matrix elements44,45 are calculated following the procedure described in46,47 for the non-relativistic case. Briefly, the absorption of one ionizing photon is treated within the RPAE approximation and a perturbed wave function is calculated. Exterior complex scaling is used in order to be able to use a finite numerical box. The two-photon matrix element is dominated by a one-photon dipole matrix element between the intermediate perturbed wave function and the final continuum state48. The integration is performed numerically out to a distance far outside the atomic core, but within the unscaled region, while the last part of the integral is carried out using analytical Coulomb waves along the imaginary radial axis. The amplitude and phase shift of these Coulomb waves are determined from the numerical solutions for the perturbed wave function and for the final state describing a free electron within the potential of the remaining ion. The numerical stability is monitored by comparison of different “break points” between the numerical and analytical descriptions.
A few adjustments have to be made in the relativistic case: Relativistic RPAE is used to obtain the perturbed wave function after absorption of one photon and the Dirac Hamiltonian to determine the phase shift of the final state. The Coulomb solution for the large component approaches the non-relativistic solution in the asymptotic region, albeit with relativistically adjusted parameters. For a given energy, the small component is easily obtained from the large one30.
Data availability
The data that support this study are available online https://doi.org/10.5878/rhak-nd96 at Swedish National Data Service. Source data are provided with this paper.
Code availability
The codes used in this study are available from the corresponding author upon reasonable request.
References
Schmidt, V. Photoionization of atoms using synchrotron radiation. Rep. Prog. Phys. 55, 1483–1659 (1992).
Howell, R. W. Auger processes in the 21st century. Int. J. Radiat. Bio. 84, 959–975 (2008).
Amusia, M. Y., Cherepkov, N. A. & Chernysheva, L. V. Cross section for the photoionization of noble-gas atoms with allowance for multielectron correlations. Zh. Eksp. Teor. Fiz. 60, 160–174 (1971).
Wendin, G. Collective resonance in the 4d10 shell in atomic Xe. Phys. Lett. A 37, 445–446 (1971).
Wendin, G. Collective effects in atomic photoabsorption spectra. III. Collective resonance in the 4d10 shell in Xe.J. Phys. B At. Mol. Phys. 6, 42–61 (1973).
Crljen, Z. & Wendin, G. Many-body theory of effective local potentials for electronic excitations. III. Phys. Rev. A 35, 1571–1581 (1987).
Lukirskii, A. P., Brytov, I. A. & Zimkina, T. M. Photoionization absorption of He, Kr, Xe, CH4, and methylal in the 23.6–250 Å region. Opt. Spectrosc. 17, 234–237 (1964).
Ederer, D. L. Photoionization of the 4d electrons in xenon. Phys. Rev. Lett. 13, 760–762 (1964).
Penent, F. et al. Multielectron spectroscopy: the xenon 4d hole double Auger decay. Phys. Rev. Lett. 95, 083002 (2005).
Amusia, M. Y., Chernysheva, L. V., Manson, S. T., Msezane, A. M. & Radojevic, V. Strong electron correlation in photoionization of spin-orbit doublets. Phys. Rev. Lett. 88, 093002 (2002).
SunilKumar, S., Banerjee, T., Deshmukh, P. C. & Manson, S. T. Spin-orbit-interaction activated interchannel coupling in dipole and quadrupole photoionization. Phys. Rev. A 79, 043401 (2009).
Cheng, K. T. & Johnson, W. R. Orbital collapse and the photoionization of the inner 4d shells for Xe-like ions. Phys. Rev. A 28, 2820–2828 (1983).
Paul, P. M. et al. Observation of a train of attosecond pulses from high harmonic generation. Science 292, 1689–1692 (2001).
Hentschel, M. et al. Attosecond metrology. Nature 414, 509–513 (2001).
Drescher, M. et al. Time-resolved atomic inner-shell spectroscopy. Nature 419, 803–807 (2002).
Gruson, V. et al. Attosecond dynamics through a Fano resonance: monitoring the birth of a photoelectron. Science 354, 734–738 (2016).
Itatani, J. et al. Tomographic imaging of molecular orbitals. Nature 432, 867–871 (2004).
Sansone, G. et al. Electron localization following attosecond molecular photoionization. Nature 465, 763–766 (2010).
Beaulieu, S. et al. Attosecond-resolved photoionization of chiral molecules. Science 358, 1288–1294 (2017).
Vos, J. et al. Orientation-dependent stereo Wigner time delay and electron localization in a small molecule. Science 360, 1326–1330 (2018).
Schultze, M. et al. Controlling dielectrics with the electric field of light. Nature 493, 75–78 (2013).
Tao, Z. et al. Direct time-domain observation of attosecond final-state lifetimes in photoemission from solids. Science 353, 62–67 (2016).
Muller, H. G. Reconstruction of attosecond harmonic beating by interference of two-photon transitions. Appl. Phys. B 74, S17–S21 (2002).
Busto, D. et al. Time-frequency representation of autoionization dynamics in helium. J. Phys. B Mol. Phys. 51, 044002 (2018).
Schultze, M. et al. Delay in photoemission. Science 328, 1658–1662 (2010).
Klünder, K. et al. Probing single-photon ionization on the attosecond time scale. Phys. Rev. Lett. 106, 143002 (2011).
Heuser, S. et al. Angular dependence of photoemission time delay in helium. Phys. Rev. A 94, 063409 (2016).
Cirelli, C. et al. Anisotropic photoemission time delays close to a Fano resonance. Nat. Commun. 9, 955 (2018).
Månsson, E. P. et al. Double ionization probed on the attosecond timescale. Nat. Phys. 10, 207–211 (2014).
Vinbladh, J. Two photon above threshold ionization in a relativistic framework: attosecond delays in high Z elements (Stockholm University, Stockholm, 2019).
Kivimäki, A., Pfeiffer, L., Aksela, H., Nõmmiste, E. & Aksela, S. Intensities of the xenon N4,5 OO Auger electron spectrum revisited. J. Electron Spectrosc. Relat. Phenom. 43, 101–103 (1999).
Jain, A., Gaumnitz, T., Bray, A., Kheifets, A. & Wörner, H. J. Photoionization delays in xenon using single-shot referencing in the collinear back-focusing geometry. Opt. Lett. 43, 4510–4513 (2018).
Isinger, M. et al. Photoionization in the time and frequency domain. Science 358, 893–896 (2017).
Dahlström, J. M. et al. Theory of attosecond delays in laser-assisted photoionization. Chem. Phys. 414, 53–64 (2013).
Mandal, A., Deshmukh, P. C., Kheifets, A. S., Dolmatov, V. K. & Manson, S. T. Angle-resolved Wigner time delay in atomic photoionization: the 4d subshell of free and confined Xe. Phys. Rev. A 96, 053407 (2017).
Mandal, A., Saha, S. & Deshmukh, P. C. In Quantum Collisions and Confinement of Atomic and Molecular Species, and Photons (Springer, Singapore, 2019).
Adam, M. Y. et al. Recent progress in the study of photoionization processes of atomic species by electron spectroscopy using synchrotron radiation. J. Electron Spectrosc. Relat. Phenom. 15, 211–224 (1979).
Shannon, S. P., Codling, K. & West, J. B. The absolute photoionization cross sections of the spin-orbit components of the xenon 4d electron from 70–130 eV. J. Phys. B Mol. Phys. 10, 825–830 (1977).
Wigner, E. P. On the quantum correction for thermodynamic equilibrium. Phys. Rev. 40, 749–759 (1932).
Lu, K. T. Spectroscopy and collision theory. The Xe absorption spectrum. Phys. Rev. A 4, 579–596 (1971).
Friedrich, H. Theoretical atomic physics (Springer-Verlag, Berlin, Heidelberg, 1998).
Nandi, S. et al. Attosecond timing of electron emission from a molecular shape resonance. Sci. Adv. 6, eaba7762 (2020).
Biswas, S. et al. Probing molecular environment through photoemission delays. Nat. Phys. 16, 778–783 (2020).
Kawai, S. & Bandrauk, A. D. Phase determination of harmonics by three-color photoionization of aligned molecules: The H\({}_{2}^{+}\) system. Phys. Rev. A 75, 063402 (2007).
Dahlström, J. M., L’Huillier, A. & Maquet, A. Introduction to attosecond delays in photoionization. J. Phys. B Mol. Phys. 45, 183001 (2012).
Dahlström, J. M., Carette, T. & Lindroth, E. Diagrammatic approach to attosecond delays in photoionization. Phys. Rev. A 86, 061402(R) (2012).
Dahlström, J. M. & Lindroth, E. Study of attosecond delays using perturbation diagrams and exterior complex scaling. J. Phys. B Mol. Phys. 47, 124012 (2014).
Vinbladh, J., Dahlström, J. M. & Lindroth, E. Many-body calculations of two-photon, two-color matrix elements for attosecond delays. Phys. Rev. A 100, 043424 (2019).
Acknowledgements
The authors acknowledge support from the Swedish Research Council, the European Research Council (advanced grant PALP-339253), the Knut and Alice Wallenberg Foundation and Olle Engkvist’s Foundation.
Funding
Open Access funding provided by Lund University.
Author information
Authors and Affiliations
Contributions
S.Z., D.B., R.J.S., M.I., L.N., H.L., R.W. and C.L.A. performed the experiment. R.J.S. and R.F. provided part of the experimental setup. J.V., J.M.D. and E.L. performed RRPA calculations. S.Z., D.B., J.M.D., G.W., M.G., E.L. and A.L. worked on the analysis and the theoretical interpretation. S.Z. and A.L. wrote the main part of the manuscript. All authors gave feedback on the manuscript.
Corresponding author
Ethics declarations
Competing Interests
The authors declare no competing interests.
Additional information
Peer review information Nature Communications thanks André Bandrauk, Niranjan Shivaram and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary information
Source data
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Zhong, S., Vinbladh, J., Busto, D. et al. Attosecond electron–spin dynamics in Xe 4d photoionization. Nat Commun 11, 5042 (2020). https://doi.org/10.1038/s41467-020-18847-1
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-020-18847-1
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.