Introduction

The transfer of thermal energy to mechanical, electrical, or magnetic excitations is of great interest and is widely considered for energy harvesting when waste heat is transferred to more usable types of energy1. The rational utilization of heat is a critical task for nanoscale electronics, where operations are accompanied by extensive production of parasitic heat. Nanoscale devices for communication and computing operate with digital signals, which are generated by ultrafast current or optical pulses with high repetition rate. The heat generated in this case is also modulated at the same frequency and could be transferred to nonthermal types of excitation, which become the elements of the energy-harvesting process. This process would be most efficient in the case of resonance, when the modulated heat is transferred to a subsystem with the same intrinsic frequency. Resonant heat transfer is widely used in photoacoustics and was proposed by Bell in 18812. There the modulated optical signal is absorbed in a system with acoustic resonance at the frequency of modulation and as a result the modulated heat resonantly excites the acoustic wave. Modulation frequencies in photoacoustics do not exceed the MHz frequency range3, while thermomodulation with frequencies up to 400 GHz4 is used in picosecond ultrasonics for studying sub-THz coherent phonon dynamics.

Here we propose to convert high-frequency (GHz) modulated heat to magnons, which are collective spin excitations in magnetically ordered materials. The manipulation of coherent high-frequency magnons on the nanoscale is one of the most prospective concepts for information technologies5, also in the quantum regime6. While the most common way to excite coherent magnons is nonthermal and based on microwave techniques7, thermal methods have proven successful in ferromagnetic metals. These methods are based on ultrafast modulation of the magnetic anisotropy induced by rapid lattice heating, for example, due to the absorption of optical pulses8,9. In ferromagnetic metals, the intrinsic magnon frequency depends on the external magnetic field and may be varied between ~1 and ~100 GHz10. This range covers the clocking frequency for most electronic devices and, thus, magnons are suitable to receive dissipated heat modulated at the resonant frequency.

In the present paper, we introduce the concept of transferring heat dissipated in a semiconductor from the relaxation of hot electrons excited by a 10 GHz laser to coherent magnons. In our experiments, the energy harvester is a metallic ferromagnetic film of 5 nm thickness grown on a semiconductor substrate. We demonstrate an efficient heat–magnon transfer by measuring a 12-fold increase of the fundamental magnon mode amplitude at the resonance conditions. The experiments and related theoretical analysis show that GHz modulation of the temperature on the scale of ~0.1 K is sufficient for the excitation of magnons with amplitude reliable for the operation of spintronic devices.

Results

Samples and experimental technique

The ferromagnetic energy harvester is a 5 nm layer of Galfenol (Fe0.81Ga0.19) grown by magnetron sputtering on a (001) semi-insulating GaAs substrate and covered by a 2 nm Cr cap layer to prevent oxidation. The chosen composition of Fe and Ga is characterized by a high Curie temperature (Tc ≈ 900 K) and large saturation magnetization M011. Experiments were carried out at ambient conditions at room temperature with an external magnetic field, B, applied in the layer plane at an angle −π/8 from the [100] crystallographic direction, which corresponds to the maximal sensitivity of the magnetization, M, to the temperature-induced changes of the magnetic anisotropy12. In the studied layer, the lowest, fundamental mode of the quantized magnon spectrum is well separated from the higher-order modes due to the large exchange mode splitting12. As a result, the magnon spectrum consists of a narrow single spectral line of Lorentzian shape with a width of ≈500 MHz. Examples of the magnon spectrum for several values of B measured by monitoring the magnetization precession excited by a single optical pulse are shown in Fig. 1a (for details see “Methods” section). The experimentally measured dependence of magnon frequency f on B is shown by the symbols in the right panel of Fig. 1a.

Fig. 1: Magnon resonances and temperature modulation.
figure 1

a Fast Fourier transform spectra showing the fundamental magnon mode for several values of magnetic field, B (left panel) and the field dependence of its frequency, f (right panel) for the 5-nm-thick Fe0.81Ga0.19 energy-harvesting layer. Symbols show f(B) obtained by fast Fourier transformation of Kerr rotation signals measured in a single pulse pump–probe experiment. Solid lines are calculated dependences for ΔT = 0 K (upper curve) and ΔT = 200 K (lower curve). The dashed horizontal lines show the frequencies of the harmonics in the temperature modulation spectrum induced by the 10 GHz optical excitation. The vertical arrows indicate the expected resonances for the fundamental magnon mode at these harmonics. b Scheme of the experiment. c Calculated temporal evolution of the Fe0.81Ga0.19 lattice temperature induced by the 10 GHz optical excitation of the Fe0.81Ga0.19/GaAs heterostructure at the pump excitation power W = 95 mW (upper panel) and its Fourier spectrum (lower panel).

Full size image

Figure 1b illustrates a schematic of the pump–probe experiment for magnon energy harvesting from high-frequency modulated heat. A femtosecond pump laser with repetition rate f0 = 10 GHz and average power up to W = 150 mW is used for modulation of the lattice temperature. A second laser with repetition rate of 1 GHz and average power of 18 mW is used to probe the response of the magnons to the high-frequency heat modulation by means of the transient polar Kerr rotation (KR) effect. The pump and probe beams are focused on the Fe81Ga19 layer into overlapping spots of 17 and 14 μm diameter, respectively, using different sectors of the same reflective microscope objective (for details see the “Methods” section).

Temperature evolution

Figure 1c (upper panel) shows the calculated temporal evolution of the lattice temperature T(t) in the Fe0.81Ga0.19 layer under 10 GHz pump optical excitation. The calculations were performed by solving the heat equations taking into account the thermal resistance at the Fe0.81Ga0.19/GaAs interface (see “Methods” section and Supplementary Notes 1 and 2). The temperature modulation amplitude \(\delta T\sim W/(C_{\mathrm{f}}r^2)\), where Cf and r are the heat capacity and radius of the excitation spot. It is seen that the lattice temperature oscillates with amplitude δT on a stationary background that exceeds the room temperature T0 by ΔT. Both ΔT and δT increase linearly with W: δT = pW and ΔT = PW, where p and P are constants, which for our structure are equal to 4.9 and 510 K W−1, respectively. The temperature modulation is not harmonic and its spectrum, which is shown in the lower panel of Fig. 1c, consists of discreet harmonics at the frequencies fn = nf0, where n is an integer. The harmonic amplitude decreases with the increase of n.

The idea of the experiments illustrated in Fig. 1a is to exploit the periodic thermal modulation for exciting coherent magnons and to monitor the magnetization precession by transient KR measurements of the out-of-plane magnetization projection, δMz, for various values of B. We expect a resonant increase of the precession amplitude at the resonances when B = Bn, which correspond to f = fn. The expected values of Bn for the first three harmonics are shown in the right panel of Fig. 1a by the vertical arrows. The idea is analogous to the excitation of a harmonic oscillator with tunable eigenfrequency f by a periodic force, which acts on the oscillator with repetition rate f0. For magnetization precession, this force is generated by the modulated temperature T(t), and the magnon eigenfrequency f is controlled by the external magnetic field.

Resonant magnon signals and their spectra

Figure 2 shows the transient KR signals measured for W = 95 mW and various values of B. The signals measured in the vicinity of the first resonance (n = 1) have a harmonic shape. At these conditions, we fit the signals by a sine function \(\delta M_z\left( t \right) = A_{B}\,{\mathrm{sin}}\left( {\omega _1t + \varphi _B} \right)\), where ω1 = 2πf1, and AB and φB are the magnetic field-dependent amplitude and phase of the harmonic oscillations. Times t = 0 and 100 ps correspond to excitation of the sample by the pump pulses. It is seen that the amplitude of the signal is maximal when B = B1 = 44 mT, which corresponds to the first resonance f = f1 (see Fig. 1a). In the vicinity of the resonance, the phase changes from φB = −π/2 for B < B1 to φB = +π/2 for B > B1. At the resonance φB ≈ 0. Similar results are observed for the signals measured in the vicinity of the second resonance (n = 2). The amplitude of the oscillations at B = B2 = 250 mT are 1.4 times smaller than for the first resonance and the same conclusions as for the first resonance can be made concerning the field dependences of the amplitude and phase of the signal around B2. Away from the resonances the signals are periodic but not harmonic. An example of a nonresonant signal measured at B = 190 mT is presented in the right panel of Fig. 2. The amplitude of the signal is much smaller than in the case of resonance and the temporal shape is impossible to fit with a single sine function.

Fig. 2: Magnon signals in time domain.
figure 2

Transient Kerr rotation signals measured in the vicinity of the first (left panel) and second (central panel) resonances and at the intermediate magnetic field of 190 mT, corresponding to off-resonance (right panel). The amplification of the precession amplitude observed at B = B1 = 44 mT and B = B2 = 250 mT is due to thermally induced resonant driving of the precession M by the oscillating effective field Beff. The torque Q acting on the magnetization (see sketch at the top right) is maximal at the resonance conditions: f = nf0.

Full size image

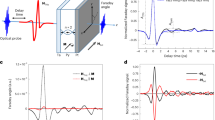
To plot the dependence of the measured signal amplitude as a function of B, we present the root-mean-square (RMS) amplitude \(\tilde A_B\) of the measured KR signal. The symbols in Fig. 3a show the field dependences \(\tilde A_B(B)\) for three pump excitation powers W. It is clearly seen that the dependences have peaks at the resonant values of Bn corresponding to n = 1, 2, and 3. Figure 3b shows zoomed fragments of the field dependences of the amplitude and phase, respectively, obtained for the first resonance at W = 95 mW. The 12-fold increase of \(\tilde A_B\) at the resonance condition is clearly seen by comparison with the out-of-resonance RMS amplitude shown by the horizontal dashed line in the upper panel of Fig. 3b. No peaks in the dependence of the precession amplitude on B are detected in the case of single pulse excitation, where \(\tilde A_B\) gradually decreases with the increase of B.

Fig. 3: Magnon spectra.
figure 3

a Measured magnetic field dependences of the root-mean-square (RMS) amplitude of the Kerr rotation signal, \({\tilde A}_{B}\), for three values of pump excitation power, W. The vertical arrows indicate the magnetic fields Bn at which the resonance condition f = fn is fulfilled; the horizontal bars indicate zero signal levels. b Measured (symbols) and calculated (solid lines) zoomed fragments of the field dependences of \({\tilde A}_{B}\) (upper panel) and the phase (lower panel) in the vicinity of the first resonance (n = 1) for W = 95 mW; the dashed horizontal line shows \({\tilde A}_{B}\) at the intermediate off-resonance field. c Calculated field dependences of the RMS precession amplitude for the background temperatures ΔT obtained from the experimental dependences in a. d Power dependence of the relative precession amplitude (left axis) and the corresponding precession angle (right axis) at the first resonance (n = 1) when f = f0.

Full size image

The values of the measured resonance fields Bn shift to slightly higher fields when W increases. We explain this shift by the heat-induced decrease of the magnon frequencies13 and respective increase of the magnetic field value required for achieving the resonance conditions. We use the values of this shift to obtain the background temperature ΔT of the Galfenol film by comparison with the known dependence of f(B) on temperature. Two dependences of f(B) calculated using the known dependence of the Galfenol magnetic parameters on temperature13,14 are demonstrated in the right panel of Fig. 1a by the solid lines (for details see Supplementary Note 2). The corresponding values of the background temperature obtained from the experimentally measured shifts of the resonances are ΔT = 28, 44, and 77 K for W = 35, 55, and 95 mW, respectively. They are 40% higher than the values calculated theoretically from the heat equations. We attribute this difference to the additional background heating by the probe beam that is not considered in the theoretical modeling.

Discussion

In the analysis, we consider thermal modulation of the magnetic anisotropy as the main mechanism for magnon excitation in our experiment and do not take into account other mechanisms (e.g., thermal strain). This approach is based on previous experiments and theoretical analysis where various mechanisms of laser-pulse-induced excitation of magnons in Galfenol were considered14. We also exclude the effect of thermal gradients inside a ferromagnetic film (we estimate 4% temperature difference across the film). This gradient in special cases can induce alternating current (a.c.) spin transfer15,16 modulated at the laser repetition rate, f0. In our experiment, due to the uniform in-plane magnetization in a single thin ferromagnetic layer, this transfer does not produce a torque on the magnetization17. The dominating role of the thermal modulation of the magnetocrystalline anisotropy is also confirmed by the dependence of the precession amplitude excited by a single laser pulse on the direction of the external magnetic field. This dependence demonstrates a four-fold in-plane symmetry with a slight uniaxial distortion, which corresponds to the magnetocrystalline anisotropy of the studied layer12.

We describe the effect of thermal modulation on the magnetization, M, by considering the magnetization precession motion in a time-dependent effective field Beff as schematically shown in Fig. 214. The magnetization precession is described by the Landau–Lifshitz–Gilbert (LLG) equation7:

$$\frac{{{\mathrm{d}}{\mathbf{m}}}}{{{\mathrm{d}}t}} = - \gamma _0{\mathbf{m}} \, \times \, {\mathbf{B}}_{{\mathrm{eff}}}(t) + \alpha _0{\mathbf{m}} \, \times \frac{{{\mathrm{d}}{\mathbf{m}}}}{{{\mathrm{d}}t}},$$
(1)

where m = M/M0 is the normalized magnetization and α0 is the Gilbert damping parameter. The effective magnetic field is determined as Beff = −∇mFM(m, t), where FM is the normalized free energy density7:

$$F_{\mathbf{M}}\left( m \right) = - \left( {{\mathbf{m}} \cdot {\mathbf{B}}} \right) + B_{\mathrm{d}}m_z^2 + K_1\left( {m_x^2m_y^2 + m_z^2m_y^2 + m_x^2m_z^2} \right) - K_{\mathrm{u}}\left( {{\mathbf{m}} \cdot {\mathbf{s}}} \right)^2,$$
(2)

where the first term is the Zeeman energy, the second term is the demagnetization energy \(\left( {B_{\mathrm{d}} = \frac{{\mu _0M_0}}{2}} \right)\), and the following two terms describe the cubic and uniaxial magnetocrystalline anisotropy with respective coefficients K1 and Ku, with the unit vector s ∥ [110] along the uniaxial anisotropy axis. In the chosen coordinate system, the x, y, and z axes correspond to the main crystallographic directions [100], [010], and [001] (normal to the layer plane), respectively. At equilibrium, that is, without temperature modulation, the value of Beff determines the fundamental magnon frequency, while its direction sets the equilibrium orientation of the magnetization m. The change of temperature alters Beff through the temperature-dependent parameters M0, K1, and Ku. Within the studied temperature range their dependence on T is linear13: \(X = X^{{\mathrm{RT}}} + \beta _X\left( {T - T_0} \right)\), where X = M0, K1, or Ku, the index RT indicates the room-temperature values and βX is the corresponding thermal coefficient. The room-temperature values \(M_0^{{\mathrm{RT}}}\) = 1.96 T, \(K_1^{{\mathrm{RT}}}\) = 20 mT, and \(K_{\mathrm{u}}^{{\mathrm{RT}}}\) = 9 mT for our sample are found from fitting the experimental dependence f(B) (shown in the right panel of Fig. 1a)12,14. The thermal coefficients, \(\beta _{M_0} = - 0.97\) mT K−1, \(\beta _{K_1} = - 0.046\) mT K−1, and \(\beta _{K_{\mathrm{u}}} = - 0.025\) mT K−1 for Galfenol are taken from previous studies13,14. The Gilbert damping coefficient α0 = 0.006 is obtained from the precession kinetics excited by a single laser pulse.

The modulation amplitude δT << ΔT and for solving Eq. (1), we assume that the magnitude of Beff is constant and the temperature modulation affects only its direction. We also consider only the ground magnon mode with zero wave vector, that is, we assume a uniform precession, due to the large diameter (17 μm) of the excitation spot, which limits the in-plane wave vector of magnons to q|| ≤ 4700 cm−1. In the 5-nm-thick Galfenol layer, the frequency range for such q|| does not exceed 270 MHz18,19, which is clearly below the 500 MHz spectral width of the fundamental magnon mode. For details of the magnon dispersion and contribution of magnons with finite wave vectors in a 100-nm-thick layer, see Supplementary Note 4.

With these assumptions, the LLG equation can be reduced to a system of equations (see the “Methods” section for details) for the magnetization components, where the thermal modulation of the anisotropy coefficients alters the direction of Beff and generates a tangential driving torque, Q, acting on the magnetization14 as schematically shown in the inset of Fig. 2. The vector Beff follows the temporal temperature evolution T(t) shown in Fig. 1c, which can be written as sum of background heating and a Fourier series expansion for the small periodic temperature modulation:

$$T\left( t \right) = T_0 + {\Delta}T + \delta T \sum\limits_{n = 1}^\infty u_n\,{{\sin}} \left( {\omega _nt + \psi _n} \right).$$
(3)

Here the amplitude and phase of the nth harmonic are \(u_n = \frac{2}{\pi }\frac{{\omega _1\tau _0}}{{\sqrt {1 + \omega _n^2\tau _0^2} }}\) and \(\psi _n = {\mathrm{arctan}}( {\frac{1}{{\omega _n\tau _0}}} )\), respectively, \(\omega _n = 2\pi f_n\), τ0 is the characteristic cooling time of the Galfenol layer, which is determined by the thermal boundary resistance, R, at the interface with GaAs20. The solution of the linearized Eq. (1) for the δmz component expanded as a Fourier series has the form:

$$\delta m_z\left( t \right) = \sum\limits_{n = 1}^\infty A_n\left( B \right){\mathrm{sin}}\left( {\omega _nt + \varphi _n\left( B \right)} \right),$$
(4)

where An(B) and φn(B) are the time-independent amplitude and phase for the nth harmonic. Like in the case of a simple harmonic oscillator, at the resonant conditions f(B) = fn the precession amplitude reaches maximum with zero phase-shift relative to the driving force \(\varphi _n\left( B \right) \approx 0\). The analytical expressions for An(B) and φn(B) are presented in the “Methods” section.

Figure 3c shows the field dependences of the RMS amplitude of the calculated δmz(t) for three values of the background temperature ΔT, which correspond to the W indicated near the experimentally measured data in Fig. 3a. The agreement of the measured and calculated spectral shapes is explicitly demonstrated in the zoomed dependences of the RMS amplitude and phase in Fig. 3b, where the calculated dependences (solid lines) for n = 1 are presented together with the experimental values. Good agreement is observed also for higher n and W.

The measured power dependence of the resonant magnon amplitude for n = 1 in the studied sample is shown in Fig. 3d. The precession amplitudes are obtained from the absolute values of the KR angle in the transient KR signals12. The field-independent small amplitude background, which is due to the response of the electron system and not related to the magnetization21, has been subtracted from the experimental values. At W = 100 mW the precession amplitude reaches 0.1% of the saturation magnetization M0. To get this value, the parameters of the magnetic anisotropy have to be modulated by the oscillating temperature with the amplitude δT = 0.54 K. This is in perfect agreement with the values obtained by solving the thermal equations, which for the same power give δT = 0.49 K. The achieved precession amplitude, which corresponds to the precession angle Θ ≈ 0.25° (with ellipticity of 0.2) and the generated a.c. induction ΔMz ~ 1 mT, is large enough for prospective spintronics applications: detection of spin currents by spin pumping22,23; manipulating single spin states of NV centers in nanodiamonds by microwave magnetic fields24; excitation of propagating spin waves in magnonic devices25.

For the practical use of resonant energy harvesting in a device excited optically or electrically, it is important to achieve high efficiency of the transformation of the modulated part of the thermal energy into the oscillations of magnetization. This efficiency is defined by the ratio of the magnetization angle Θ of precession and the power W injected into a device. In our experiment, we demonstrate Θ/W = 2.5 °W−1, which is only one order of magnitude less than the efficiency of conventional mesoscopic microwave devices26 and of the same order as devices utilizing surface acoustic waves for spin pumping23. At resonance, the magnetization precession remains harmonic up to the maximum used power, and, thus, nonlinear (anharmonic) effects do not affect the transformation efficiency. However, the shift of the resonance frequency with the increasing ΔT at higher W affects the linear power dependence of the precession amplitude at fixed magnetic field. This temperature-induced “nonlinearity” is known from the conventional microwave experiments on ferromagnetic resonance27. The effect of this nonlinearity is very weak in the studied structure. For instance, for the second resonance (n = 2) the 20% change of the background temperature without changing thermal modulation amplitude results in a ~3% decrease of the precession amplitude. Thus, stability of the background temperature is not critical for the suggested concept.

The dependences of δT on the parameters of the used structure and materials define the efficiency of the heat transfer to the magnons. For optical excitation, shorter penetration depths and smaller heat capacities of the ferromagnetic layer increase δT and correspondingly Θ/W. It is convenient to define the dimensionless resonant harvesting efficiency, ζ = δT/ΔT, which governs the ratio of the useful temperature modulation δT relative to the parasitic heating, ΔT. Obviously, it is favorable to have ζ as large as possible. For instance, faster cooling to the bulk of the substrate leads to an increase of δT with a simultaneous decrease of ΔT and correspondingly to an increase of ζ. In our particular experiment, where both the Fe0.81Ga0.19 nanolayer and the GaAs substrate are excited optically, we get ζ ~ 10−2. The value of ζ increases with the decrease of the thickness of the ferromagnetic layer and the increase of the substrate thermoconductivity (see Supplementary Note 3). For instance, for the same ferromagnetic layer on a silicon substrate ζ increases by a factor of 1.5. An efficient way to increase ζ and the efficiency of the heat–magnon transfer would be to use a multilayer structure where ΔT is governed by the rapid escape of heat to the substrate while the high-frequency thermal waves, which define δT, are localized inside or in the vicinity of the harvester layer. To demonstrate that the concept is applicable for electrical nanodevices, we have considered the case of a thin conducting layer (e.g., graphene) where Joule heat can be generated as a result of passing GHz current pulses and transferred to the ferromagnetic harvester through a thin spacer layer. For high efficiency, the spacer layer should have high thermal conductivity, while the ferromagnetic layer should have low specific heat capacity. In the example presented in the Supplementary Note 3, we get ζ = 4 × 10−3.

In our experiments, we excite resonances up to 30 GHz. To reach higher, sub-THz frequencies, the corresponding bandwidth should be available for both temperature and magnon modulation. For temperature modulation, we refer to the picosecond acoustic experiment4 where the temperature in a 12-nm Al film was modulated at a frequency up to 400 GHz. The thermal properties of ferromagnetic metals do not differ much from normal metals and thus similar modulation in thin films is achievable. For optical excitation of metals, it is essential that optically excited hot electrons pass their energy to the lattice in a time <1 ps. Theoretically, for the parameters fixed as above, the amplitude of the temperature oscillations is proportional to the laser power and inversely proportional to the frequency up to sub-THz frequencies. For the magnons in thin (Fe,Ga) films, narrow-band precession with frequencies up to 100 GHz was demonstrated at strong magnetic fields12. Moreover, metallic ferrimagnetic materials (e.g., Mn-Te compounds) possess narrow magnon resonances at frequencies up to hundreds of GHz at low magnetic fields28. At the lower frequency end, there is no limit for temperature modulation, while for magnons the lower frequency varies from hundreds of MHz (e.g., for garnets) up to several GHz in metallic ferromagnets. The available choice of a proper magnetic material is not limited to the Galfenol used in our study. This ferromagnet is actively studied nowadays29 and presents a good example for realization of the demonstrated concept due to its pronounced magnetocrystalline anisotropy and narrow magnon resonances (long lifetime of precession). However, other ferro- and ferrimagnetic materials possessing similar properties will also work as resonant harvesters for transferring parasitic heat modulated at high frequencies to magnons.

To conclude, we have demonstrated how the heat generated during 10 GHz pulsed optical excitation is used for the excitation of resonant magnons. The amplitude of the magnetization precession at the fundamental magnon frequency increases enormously when the repetition rate of the optical pulses is equal to the magnon frequency. The resonances also occur at the higher harmonic frequencies, that is, 20 and 30 GHz. An amplitude of the temperature modulation of order ~0.1 K is sufficient to generate a.c. magnetization with an amplitude of 1 mT30, which is sufficient to be exploited in various applications including information and quantum technologies. The concept of resonant heat transfer can be considered as a prospective method to generate magnons in ferromagnetic layers deposited on processors and other microchips operating at GHz and sub-THz clock frequencies.

Methods

Pump–probe measurements with 10 GHz repetition rate

We used two synchronized Titanium sapphire lasers (Taccor x10 and Gigajet 20c from Laser Quantum) generating 50 fs pulses with repetition rates of 10 GHz (pump) and 1 GHz (probe) at center wavelengths of 810 and 780 nm, respectively. The beams were focused onto the sample using a reflective microscope objective with a magnification factor of 15 comprising 4 sectors through which light could enter and exit the objective31. The incidence angles of the laser beams are 17°. The spot diameters for the pump and probe beams were set to 17 and 14 μm, respectively. The diameters are defined at the 1/e2 level of the Gaussian spatial energy distributions. The coherent response of the magnetization was measured by monitoring the polar KR of the reflected probe pulses using a differential scheme based on a Wollaston prism and a balanced optical receiver with 10 MHz bandwidth. Temporal resolution was achieved by means of an asynchronous optical sampling (ASOPS) technique32, where we used an offset frequency of 20 kHz between the 10 GHz laser and the tenth harmonics of the 1 GHz laser in order to resolve the transient signals in 100 ps time window between pump pulses with the time resolution of 50 fs. The sample was mounted between the poles of a dipole electromagnet.

Measurements of the magnon spectrum with single pulse excitation

The magnon spectrum of the studied sample was obtained by a conventional magneto-optical pump–probe scheme based on two mode-locked Erbium-doped ring fiber lasers (FemtoFiber Ultra 780 and FemtoFiber Ultra 1050 from Toptica). The lasers generate pulses of 150 fs duration with a repetition rate of 80 MHz at wavelengths of 1046 nm (pump pulses) and 780 nm (probe pulses). The magnetization precession was excited by the pump pulses with an energy of 3 nJ per pulse focused on the Galfenol film surface to a spot of 17 μm diameter. The linearly polarized probe pulses with energy of 30 pJ per pulse hitting at normal incidence to the sample surface were focused to a spot of 1 μm diameter in the center of the pump spot. The coherent response was measured by transient polar KR in the same way as for the 10 GHz measurements. Temporal resolution was achieved also by the ASOPS technique with a frequency offset of 800 Hz, which in combination with the 80 MHz repetition rate allowed measurement of the time-resolved signals in the time window of 12.5 ns with 150 fs time resolution. The magnon spectra and the magnetic field dependence of the central frequency were obtained by fast Fourier transforming the corresponding transient KR signals.

Modeling of temperature evolution

In the case of periodic laser-pulse excitation, the temperature oscillates around the background temperature T0 + ΔT with amplitude δT. The amplitude δT is estimated by solving the standard heat equations for the lattice temperatures of Fe0.81Ga0.19 and GaAs for periodic excitation by laser pulses using Comsol Multiphysics®33 (see Supplementary Note 1). We estimate the background heating ΔT from the solution of the 3D stationary heat equation for continuous wave laser excitation (see Supplementary Note 2). We use the following parameters for GaAs34: refractive index 3.7 + i0.09, which results in an absorption length of 740 nm; heat capacity 1.76 × 106 J m−3 K−1 and thermal conductivity 55 W m−1 K−1 35,36. We assume that the optical and thermal parameters of Fe0.81Ga0.19 are close to the parameters of Fe: refractive index 2.9 + i3.437; heat capacity 3.8 × 106 J m−3 K−1 and thermal conductivity 80 W m−1 K−1 38. As a result the reflection coefficient of the (Fe,Ga)/GaAs heterostructure is 0.4 and the absorption coefficients for the Fe0.81Ga0.19 layer and GaAs substrate are 0.1 and 0.49, respectively. The calculated values of ΔT and δT at the excitation power W = 95 mW are 48 and 0.47 K, respectively.

In our calculations, we consider the thermal boundary resistance, R, which determines the flux through the Fe0.81Ga0.19/GaAs interface20. In order to estimate R, we measured the reflectivity signal from the studied structure in the case of low-frequency (80 MHz) laser excitation. Using the experimentally observed decay time of the transient reflectivity τ0 = 200 ps, we found the thermal boundary resistance R = 10−8 m2 K W−1.

Modeling the periodic laser-pulse-induced magnetization precession

To calculate the magnetization precession amplitudes An(B) and phases φn(B), we apply the approach developed in ref. 14 where the detailed theory of magnetization precession due to ultrafast heating of a Galfenol film was considered. We analyze the precession by rewriting the LLG equation in a spherical coordinate system with in-plane azimuthal angle, ϕ, and polar angle, θ (θ = 0 corresponds to the layer normal, z), which describes the direction of the normalized magnetization, m. Assuming that the changes of δϕ and δθ from the equilibrium angles ϕ0 and θ0 are small and induced by the modulation of the cubic and uniaxial anisotropy constants, the LLG equation in linear approximation has the form:

$$\begin{array}{l}\frac{{\partial \delta \phi }}{{\partial t}} = \gamma _0F_{\theta \theta }\delta \theta + \alpha _0\frac{{\partial \delta \theta }}{{\partial t}},\\ \frac{{\partial \delta \theta }}{{\partial t}} = \gamma _0F_{\phi \phi }\delta \phi - \gamma _0F_{\phi K_1}\delta K_1(t) - \gamma _0F_{\phi K_{\mathrm{u}}}\delta K_{\mathrm{u}}\left( t \right) - \alpha _0\frac{{\partial \delta \phi }}{{\partial t}},\end{array}$$
(5)

where the \(F_{i,j} = \frac{{\partial ^2}}{{\partial i\partial j}}F_{\mathbf{M}}(i,j = \theta ,\phi ,K_1,K_{\mathrm{u}})\) are calculated for the equilibrium (in-plane) orientation of m, ϕ0(B, T), θ0 = π/2, and α0 is the Gilbert damping parameter. At the in-plane equilibrium orientation of m, \(F_{\theta \phi } = F_{\theta K_1} = F_{\theta K_{\mathrm{u}}} = 0\) and there is no torque due to the thermal change of M0.

In Eq. (4) the precession amplitude and phase are determined as \(A_n(B) = \sqrt {a_n^2\left( B \right) + b_n^2(B)}\) and \(\varphi _n\left( B \right) = {\mathrm{arctan}}\left( {a_n(B)/b_n(B)} \right)\), where \(a_n\left( B \right) = \left( { - \omega _n\xi Q_n^b + 2\tau _M^{ - 1}\omega _n^2Q_n^a} \right)/G\) and \(b_n\left( B \right) = \left( {\omega _n\xi Q_n^a + 2\tau _M^{ - 1}\omega _n^2Q_n^b} \right)/G\), with \(G = \xi ^2 + 4\omega _n^2\tau _M^{ - 2}\), and \(\xi = \omega _n^2 - \omega ^2\). Here \(\omega=2{\pi}f(B)\) and \(\tau _M^{ - 1} = \alpha _0\gamma _0(F_{\theta \theta } + F_{\phi \phi })/2\) are the field-dependent frequency and decay time of the fundamental magnon mode12. The Fourier series expansion coefficients of the effective driving force \(Q_n^a\) and \(Q_n^b\) are determined by the temporal modulation of the temperature given by Eq. (2) and are equal to \(Q_n^a = \frac{2}{\pi } \frac{{\omega _1\tau _0}}{{1 + \omega _n^2\tau _0^2}}Q\) and \(Q_n^b = \frac{2}{\pi } \frac{{\omega _1\omega _n\tau _0^2}}{{1 + \omega _n^2\tau _0^2}}Q\), where \(Q = \gamma _0(\sin \left( {4\phi _0} \right)\beta _{K_1}/2 - {\mathrm{cos}}(2\phi _0)\beta _{K_{\mathrm{u}}})\delta T\).