Abstract
Speed of sound waves in gases and liquids are governed by the compressibility of the medium. There exists another type of non-dispersive wave where the wave speed depends on stress instead of elasticity of the medium. A well-known example is the Alfven wave, which propagates through plasma permeated by a magnetic field with the speed determined by magnetic tension. An elastic analogue of Alfven waves has been predicted in a flow of dilute polymer solution where the elastic stress of the stretching polymers determines the elastic wave speed. Here we present quantitative evidence of elastic Alfven waves in elastic turbulence of a viscoelastic creeping flow between two obstacles in channel flow. The key finding in the experimental proof is a nonlinear dependence of the elastic wave speed cel on the Weissenberg number Wi, which deviates from predictions based on a model of linear polymer elasticity.
Similar content being viewed by others
Introduction
A small addition of long-chain, flexible, polymer molecules strongly affects both laminar and turbulent flows of Newtonian fluid. In the former case, elastic instabilities and elastic turbulence (ET)1,2,3,4,5 are observed at Reynolds number \({\mathrm{Re}} \ll 1\) and Weissenberg number \({\mathrm{Wi}} \gg 1\), whereas in the latter, turbulent drag reduction (TDR) at \({\mathrm{Re}} \gg 1\) and \({\mathrm{Wi}} \gg 1\) has been found about 70 years ago but its mechanism is still under active investigation6. Here both Re = ρUD/η and Wi = λU/D are defined via the mean fluid speed U and the vessel size D, and ρ, η are the density and the dynamic viscosity of the fluid, respectively, and λ is the longest polymer relaxation time. ET is a chaotic, inertialess flow driven solely by nonlinear elastic stress generated by polymers stretched by the flow, which is strongly modified by a feedback reaction of elastic stresses7. The only theory of ET based on a model of polymers with linear elasticity predicts elastic waves that are strongly attenuated in ET, but elastic waves may play a key role in modifying velocity power spectra in TDR7,8. Using the Navier-Stokes equation and the equation for the elastic stresses in uniaxial form of the stress tensor approximation, one can write the polymer hydrodynamic equations in the form of the magneto-hydrodynamic (MHD) equations8. Then, by analogy with the Alfven waves in MHD9,10, one gets the elastic wave linear dispersion relation as \(\omega = ({\bf{k}} \cdot \hat n)\left[ {tr\left( {\sigma _{ij}} \right){\mathrm{/}}\rho } \right]^{1/2}\) with the elastic wave speed7,8 cel = [tr(σij)/ρ]1/2, where ω and k are frequency and wavevector, respectively, σij is the elastic stress tensor, and \(\hat n\) is the major stretching direction, similar to the director in nematics. Such an evident difference between the elastic stress tensor characterized by the director and the magnetic field that is the vector, however, does not alter the similarity between the elastic and Alfven waves, since only uniaxial stretching independent of a certain direction is a necessary condition for the wave propagation determined by the stress value7.
A simple physical explanation of both the Alfven and elastic waves can be drawn from an analogy of the response of either magnetic or elastic tension on transverse perturbations and an elastic string when plucked. As in the case of elastic string, the director is sufficient to define the alignment of the stress. Thus, to excite either Alfven or elastic waves the perturbations should be transverse to the propagation direction, unlike longitudinal sound waves in plasma, gas, and fluid media11. The detection of the elastic waves is of great importance for a further understanding of ET mechanism and TDR, where turbulent velocity power spectra get modified according to ref. 7. Moreover, cel provides unique information about the elastic stresses, whereas the wave amplitude is proportional to the transversal perturbations, both of which are experimentally unavailable otherwise8.
Numerical simulations of a two-dimensional Kolmogorov flow of a viscoelastic fluid with periodic boundary conditions reveal filamented patterns in both velocity and stress fields of ET12. These patterns propagate along the mean flow direction in a wavy manner with a speed cel ≃ U/2, nearly independent of Wi. In subsequent studies, extensive three-dimensional Lagrangian simulations of a viscoelastic flow in a wall-bounded channel with a closely spaced array of obstacles show transition to a time-dependent flow, which resembles the elastic waves13. Further, the elastic stress field around the obstacles demonstrates similar traveling filamental structures12,13 in ET, interpreted as elastic waves7,8. However, in both studies neither the linear dispersion relation nor the dependence of wave speed cel on elastic stress–primary signatures of the elastic waves–were examined. Moreover, cel was found to be close to the flow velocity, contradicting the theory7,8. Strikingly, an indication of the elastic waves, in numerical studies, originates from observed frequency peaks in the velocity power spectra above the elastic instability12,13. Analogous frequency peaks in the power spectra of velocity and absolute pressure fluctuations above the instability were also reported in experiments of a wall-bounded channel flow in a creeping viscoelastic fluid, obstructed by either a periodic array of obstacles14 or two widely-spaced cylinders15,16. These observations were in agreement with numerical simulations17 and were associated with noisy cross-stream oscillations of a pair of vortices engendered due to breaking of time-reversal symmetry.
Our early attempts to excite the elastic waves both in a curvilinear flow and in an elongation flow of polymer solutions at \({\mathrm{Re}} \ll 1\) were unsuccessful18. In the ET regime of the curvilinear channel flow, either an excitation amplitude was insufficient and/or an excitation frequency was too high. The reason we chose the elongation flow, realized in a cross-slot micro-fluidic device, is a strong polymer stretching in a well-defined direction along the flow. However, the elongation flow generated in the cross-slot geometry has the highest elastic stresses in a central vertical plane parallel to the flow in the outlet channels–analogous to a stretched vertical elastic membrane. The transverse periodic perturbations in the experiment were applied in a cross-stream direction from the top wall18, however a more effective method would be to perturb it in a span-wise direction that was difficult to realize in a micro-channel. A higher frequency range of perturbations, compared to that found in the current experiment, was used that lead to the wave excitation with wave numbers in the range of high dissipation.
Here we report evidence of elastic waves observed in elastic turbulence of a dilute polymer solution flow in a wake between two widely-spaced obstacles, hindering a channel flow. The central finding in the experimental proof of the elastic wave observation is a power-law dependence of cel on Wi, which deviates from the prediction based on a model of linear polymer elasticity7. The distinctive feature of the current flow geometry is a two-dimensional nature of the ET flow, in the mid-plane of the device, in contrast to other flow geometries studied earlier.
Results
Flow structure and elastic turbulence
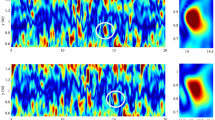
The schematic of the experimental setup is shown in Fig. 1, where two-widely spaced obstacles hinder the channel flow of a dilute polymer solution (see Methods section for the experimental setup, solution preparation and its characterization). The main feature of the flow geometry used is the occurrence of a pair of quasi-two-dimensional counter-rotating elongated vortices, in the region between the obstacles, as a result of the elastic instability15 at \({\mathrm{Re}} \ll 1\) and Wi > 1; \({\mathrm{Re}} = 2R\bar u\rho {\mathrm{/}}\eta\) and \({\mathrm{Wi}} = \lambda \bar u{\mathrm{/}}2R\), where obstacles’ diameter 2R and average flow speed \(\bar u_{}^{}\) are defined in Methods section. The frequency power spectra of cross-stream velocity v fluctuations show oscillatory peaks at low frequencies15,16 below λ−1. Above the elastic instability, the main peak frequency fp grows linearly with Wi, characteristic to the Hopf bifurcation15. The two vortices form two mixing layers with a non-uniform shear velocity profile and with further increase of Wi their dynamics become chaotic, exhibiting ET properties, with vigorous perturbations that intermittently destroy vortices16 and seemingly excite the elastic waves. The ET flow in the region between the obstacles is shown through long-exposure particle streaks imaging in Supplementary Movies 1–3 for three different Wi.
Schematic of experimental setup. It consists of a linear channel of dimension L × w × h = 45 × 2.5 × 1 mm3 with two cylindrical obstacles (shown as two black dots), diameter 2R = 0.3 mm and separated by a distance between the obstacles centers e = 1 mm, embedded at the center line of the channel. The polymer solution is driven by Nitrogen gas and injected through the inlet into the channel. The fluid exiting the channel outlet is weighed instantaneously as a function of time. An absolute pressure sensor, marked as P, after the downstream cylinder is employed to detect pressure fluctuations
Characterization of low frequency oscillations
To investigate the nature of these oscillations we present time series of the streamwise u(t) and cross-stream v(t) velocity components and their temporal auto-correlation functions A(u) = 〈u(t)u(t + τ)〉t/〈|u(t)|2〉t and A(v) = 〈v(t)v(t + τ)〉t/〈|v(t)|2〉t in Fig. 2a–d. Distinct oscillations in v(t) contrary to weak noisy oscillations in u(t) indicate flow anisotropy. Further, the cross-stream velocity power spectra Sf (v) as a function of normalized frequency λf for five Wi values in the ET regime are shown in log-lin and log-log coordinates in Fig. 3a, b, respectively. The power spectra Sf (v) exhibit the oscillation peaks at low frequencies up to λf ≤ 40 with an exponential decay of the peak values (Fig. 3a). These low frequency oscillations look much more pronounced on a linear scale (Supplementary Fig. 1a). Further, these oscillations are also observed in the power spectra of pressure fluctuations S(P) versus λf, though not so regular (Supplementary Fig. 1b). The exponential decay of Sf (v) at λf ≤ 40 implies that only a single frequency (or time) scale is identified for each Wi (Fig. 3a). This frequency fd, for each Wi, is obtained by an exponential fit to the data, i.e., Sf (v) ~ exp(−f/fd). The variation of fd with Wi is shown in the inset in Fig. 3b; it varies from 0.7 to 2.5 Hz in the range of Wi from 75 to 200, which is comparable to oscillation peak frequency fp (Fig. 4) and larger than λ−1. Strikingly, on normalization of f with fd for each Wi, Sf (v) for all Wi collapse on to each other (Fig. 3b). At higher frequencies up to λf ≤ 100, Sf (v) decay as the power-law with the exponent αf = −3.4 ± 0.1 typical for ET5 (Fig. 3c). Contrary to a general case, where the power-law decay of Sf (v) corresponding to ET3,4,5 commences at λf ≈ 1, the low frequency oscillations cause the power-law spectra start to decay at higher frequencies 10 < λf < 40, perhaps due to an additional mechanism of energy pumping into ET associated with the low frequency oscillations. In addition, S(P) exhibit the power spectra decay in the high frequency range 10 < λf < 100 with the exponent close to −3 (see the bottom inset in Fig. 2 in ref. 16), characteristic to the ET regime19. It should be emphasized that the power spectra of the streamwise velocity Sf (u) do not show the low frequency oscillations and decays with a power-law exponent α ≤ 2.
Streamwise and cross-stream components of velocity and corresponding autocorrelation functions. Time series of a streamwise velocity u and b cross-stream velocity v, obtained at (x/R, y/R) = (2.3, 0.03), corresponding to the location near the line connecting the centers’ of obstacles and close to the center region between the obstacles, for three values of Wi. c, d Their respective temporal autocorrelation functions A(u) and A(v)
Cross-stream velocity power spectra versus normalized frequency in elastic turbulence. a Cross-stream velocity power spectra Sf (v) in log-lin coordinates to emphasize an exponential decay of the oscillation peak values at low frequencies λf ≤ 40. An exponential decay is shown by the dashed line, e.g., for the case of Wi = 197.5. b Sf (v) for different Wi collapse on to each other upon normalization of f with fd. Inset: variation of fd with Wi. The error bars on fd are estimated based on standard deviation (s.d.) of exponential fit of Sf (v) versus f, and for Wi they are calculated based on the s.d. from the mean value of fluid discharge rate Q (see Methods section). c Sf (v) in log-log coordinates, for different Wi, to demonstrate the power-law decay at high frequencies ~10 < λf ≤ 100. The spectra are obtained at (x/R, y/R) = (5.2, 0.56), which is close to the downstream obstacle and to the center of the upper large vortex. The dashed line in c is a fit to the data at high frequencies with the power-law exponent αf ≃ −3.4 ± 0.1, typical to the ET regime. Sf (v) of steady flow is shown by gray lines in a, c
Dependence of oscillation peak frequency on Wi. Dashed line is a linear fit to the data in the regime above the elastic instability. Inset: fp as a function of Wiint. The error bars on fp are estimated from the spectral width of the oscillatory peaks of Sf (v), and for Wiint they are calculated based on the s.d. from the mean value of (∂u/∂y)
Figure 4 shows the dependence of fp in a wide range of Wi. The first elastic instability, characterized as the Hopf bifurcation, occurs at low Wi, where fp grows linearly with Wi–in accord with our early results15. At higher Wi in the ET regime, fp(Wi) dependence becomes nonlinear at Wi ≥ 60. In the inset in Fig. 4, we present the same data for fp as a function of Wiint. Here, the Weissenberg number of the inter-obstacle velocity field is defined as \({\mathrm{Wi}}_{{\mathrm{int}}} = \lambda \dot \gamma\) and \(\dot \gamma \left( { = \left\langle {\partial u{\mathrm{/}}\partial y} \right\rangle _t} \right)\) is the time-averaged shear-rate in the cross-stream direction in the inter-obstacle flow region. The parameter Wiint is relevant to the description of elastic waves in ET flow between the obstacles’ region. The inset in Fig. 5b shows a linear dependence of Wiint on Wi.
Elastic wave speed versus Wiint. a Cross-correlation functions of the cross-stream velocity Cv(Δx, τ) versus lag time τ for different values of Δx, obtained at y/R = 0.18 and for Wi = 148.4. Inset: Δx versus τp for Wi = 148.4, and a slope of linear fit to it (shown by dashed line) provides cel. The error bars on Δx are determined by the spatial resolution of measurements, and for τp they are estimated based on the s.d. of gaussian fit of Cv(Δx, τ). b Dependence of cel on Wiint, where the dashed line is a fit of the form \(c_{{\mathrm{el}}} = A\left( {{\mathrm{Wi}}_{{\mathrm{int}}} - {\mathrm{Wi}}_{{\mathrm{int}}}^{\mathrm{c}}} \right)^\beta\), where A = 8.9 ± 1.2 mm s−1, β = 0.73 ± 0.12, and onset value \({\mathrm{Wi}}_{{\mathrm{int}}}^{\mathrm{c}} = 1.75 \pm 0.2\). Inset: Wiint versus Wi. The error on cel is estimated based on the s.d. of the linear fit of Δx versus τp
Dependence of elastic wave speed on Wiint
Figure 5a shows a family of temporal cross-correlation functions Cv(Δx, τ) = 〈v(x, t)v(x + Δx, t + τ)〉t/〈v(x, t)v(x + Δx, t)〉t of v between two spatially separated points, with their distance being Δx, located on a horizontal line at y/R = 0.18 for Wi = 148.4. A gaussian fit to Cv(Δx, τ) in the vicinity of τ = 0 yields the peak value τp at a given Δx. A linear dependence of Δx on τp (e.g., Fig. 5a inset for Wi = 148.4) provides the perturbation propagation velocity as cel = Δx/τp. The variation of cel as a function of Wiint is presented in Fig. 5b together with nonlinear fit of the form \(c_{{\mathrm{el}}} = A\left( {{\mathrm{Wi}}_{{\mathrm{int}}} - {\mathrm{Wi}}_{{\mathrm{int}}}^{\mathrm{c}}} \right)^\beta\), where A = 8.9 ± 1.2 mm s−1, β = 0.73 ± 0.12, and onset value \({\mathrm{Wi}}_{{\mathrm{int}}}^{\mathrm{c}} = 1.75 \pm 0.2\). The same data of cel is plotted against Wi (see Supplementary Fig. 3) and fitted as cel ~ (Wi − Wic)β that yields the onset value Wic = 59.7 ± 1.8.
Discussion
In the light of the predictions7, it is surprising to observe the elastic waves in the ET regime due to their anticipated strong attenuation. An estimate of the wave number k = ω/cel = 2πfp/cel from cel (Fig. 5b) and fp (Fig. 4) provides k in the range between 0.63 and 1.3 mm−1 (Supplementary Fig. 2). The corresponding wavelengths (~2π/k) are significantly larger than the inter-obstacle spacing e − 2R = 0.7 mm. The spatial velocity power spectra Sk is limited by a size of the observation window of about 0.7 mm that gives kx ≈ 9 mm−1, much larger than the wave numbers calculated above. Thus, the low kx part of Sk(v), where the elastic wave peaks can be anticipated, is not resolved by the spatial velocity spectra (Supplementary Fig. 4b). The power-law decay with αk ≈ −3.3 is found at low kx followed by a bottleneck part and a consequent gradual power-law decay with an exponent ~−0.5 at higher kx (Supplementary Fig. 4b), unlike Sf (v), where the peaks appear at low f and the steep power-law decay with the exponent αf = −3.4 at higher f (see Fig. 3b). The spatial streamwise velocity power spectra Sk(u), obtained at the same Wi and near the center line y/R = 0.01, are similar to Sk(v) at low kx and decays gradually with exponent ~−0.3 at higher kx (Supplementary Fig. 4a).
The observed nonlinear dependence of cel on Wiint differs from the theoretical prediction based on the Oldroyd-B model7,8. The expression for the elastic wave speed in the model20 gives cel = [tr(σij)/ρ]1/2 ≈ (N1/ρ)1/2, where \(N_1 = 2{\mathrm{Wi}}_{{\mathrm{int}}}^2\eta {\mathrm{/}}\lambda\) is the first normal stress difference. Then one obtains cel = (2η/ρλ)1/2Wiint. First, cel is proportional to Wiint and second, the coefficient in the expression for the parameters used in the experiment is estimated to be (2η/ρλ)1/2 = 4.5 mm s−1. Taking into account that the model7,8 and the estimate of elastic stress are based on linear polymer elasticity20, whereas in experiments polymers in ET flow are stretched far beyond the linear limit21, thus it is not surprising to find the quantitative discrepancies between them. Indeed, the value of the coefficient found from the fit (8.9 mm s−1) and estimated theoretical value (4.5 mm s−1) differ almost by a factor of two (see Fig. 5b). Moreover, for the maximal value of cel = 17 mm s−1 (at Wiint ≈ 4) obtained in the experiment, an estimate of elastic stress gives \(\left\langle \sigma \right\rangle = c_{{\mathrm{el}}}^2\rho = 0.37\,{\mathrm{Pa}}\) that is lower but comparable with 〈σ〉 ≈ 1 Pa obtained from the experiment on stretching of a single polymer T4DNA molecule at similar concentrations21. Thus, both the cel dependence on Wiint and the coefficient value indicate that the Oldroyd-B model based on linear polymer elasticity cannot quantitatively describe the elastic wave speed and so the elastic stresses. Another aspect of this result is the Mach number \({\mathrm{Ma}} \equiv \bar u{\mathrm{/}}c_{{\mathrm{el}}}\); the maximum value achieved in the experiment is \({\mathrm{Ma}}_{{\mathrm{max}}} = \bar u_{{\mathrm{max}}}{\mathrm{/}}c_{{\mathrm{el}}} \approx 0.3\), contrast to what is claimed in refs.22,23 due to a wrong definition based on the elasticity El = Wi/Re instead of elastic stress σ used for the estimation of cel and Ma.
We discuss two possible reasons related to the detection of the elastic waves. As indicated in the introduction, the key feature of the current geometry is a two-dimensional nature of the chaotic flow, at least in the mid-plane of the device (see Fig. 4SM in Supplemental Material of ref. 16), that makes it analogous to a stretched elastic membrane. This flow structure is different from three-dimensional elastic turbulence in other studied flow geometries and thus may explain the failure in the earlier attempts to observe the elastic waves. Another qualitative discrepancy with the theory7,8 is the predicted strong attenuation of the elastic waves in ET. Below we estimate the range of the wave numbers with low attenuation for the elastic waves and compare with the observed values.
There are two mechanisms of the elastic wave attenuation, namely polymer (or elastic stress) relaxation and viscous dissipation7,8. The former has scale-independent attenuation λ−1, which at the weak attenuation satisfies the relation ωλ > 1, and the latter provides low attenuation24 at ηk2/ρω < 1. The first condition leads to ks > 1, where s = Wiint(2ηλ/ρ)1/2 that provides a minimum wave number in the ET regime as kmin > s−1 = 6.3 × 10−3 mm−1 for Wiint = 4. The maximum value kmax follows from the second condition that gives kΛ < 1 at Λ = (Wiint)−1(ηλ/2ρ)1/2. Thus, one obtains in the ET regime kmax < Λ−1 = 0.2 mm−1 for Wiint = 4 and therefore, the range of the wave numbers with the low attenuation is rather broad 6.3 × 10−3 < k < 0.2 mm−1 and lies far outside of the k-range of Sk(u) and Sk(v) presented in Supplementary Fig. 4, where the range of the wave numbers of the elastic waves is not resolved. However, the range of the observed wave number 0.63 ≤ k ≤ 1.3 mm−1 of the elastic waves, shown in Supplementary Fig. 2, is sufficiently close to the estimated upper bound of k.
Methods
Experimental setup
The experiments are conducted in a linear channel of L × w × h = 45 × 2.5 × 1 mm3, shown schematically in Fig. 1. The channel is prepared from transparent acrylic glass (PMMA). The fluid flow is hindered by two cylindrical obstacles of 2R = 0.30 mm made of stainless steel separated by a distance of e = 1 mm and embedded at the center of the channel. Thus the geometrical parameters of the device are 2R/w = 0.12, h/w = 0.4 and e/2R = 3.3 (see Fig. 1). The longitudinal and transverse coordinates of the channel are x and y, respectively, with (x, y) = (0, 0) lies at the center of the upstream cylinder. The fluid is driven by N2 gas at a pressure up to ~10 psi and is injected via an inlet into the channel.
Preparation and characterization of polymer solution
As a working fluid, a dilute polymer solution of high molecular weight polyacrylamide (PAAm, Mw = 18 MDa; Polysciences) at concentration c = 80 ppm (c/c* ≃ 0.4, where c* = 200 ppm is the overlap concentration for the polymer used25) is prepared using a water-sucrose solvent with sucrose weight fraction of 60%. The solvent viscosity, ηs, at 20 °C is measured to be 100 mPa · s in a commercial rheometer (AR-1000; TA Instruments). An addition of the polymer to the solvent increases the solution viscosity, η, of about 30%. The stress-relaxation method25 is employed to obtain longest relaxation time (λ) of the solution and it yields λ = 10 ± 0.5 s.
Flow discharge measurement
The fluid exiting the channel outlet is weighed instantaneously W(t) as a function of time t by a PC-interfaced balance (BA210S, Sartorius) with a sampling rate of 5 Hz and a resolution of 0.1 mg. The time-averaged fluid discharge rate \(\bar Q\) is estimated as \(\overline {{\mathrm{\Delta }}W{\mathrm{/\Delta }}t}\). Thus, Weissenberg and Reynolds numbers are defined as \({\mathrm{Wi}} = \lambda \bar u{\mathrm{/}}2R\) and \({\mathrm{Re}} = 2R\bar u\rho {\mathrm{/}}\eta\), respectively; here \(\bar u = \bar Q{\mathrm{/}}\rho wh\) and fluid density ρ = 1286 Kg m−3.
Imaging system
For flow visualization, the solution is seeded with fluorescent particles of diameter 1 μm (Fluoresbrite YG, Polysciences). The region between the obstacles is imaged in the mid-plane via a microscope (Olympus IX70), illuminated uniformly with LED (Luxeon Rebel) at 447.5 nm wavelength, and two CCD cameras attached to the microscope: (i) GX1920 Prosilica with a spatial resolution 1000 × 500 pixel at a rate of 65 fps and (ii) a high resolution CCD camera XIMEA MC124CG with a spatial resolution 4000 × 2200 pixel at a rate of 35 fps, are used to acquire images with high temporal and spatial resolutions, respectively. We perform micro particle image velocimetry26 (μPIV) to obtain the spatially-resolved velocity field U = (u, v) in the region between the cylinders. Interrogation windows of 16 × 16 pixel2 (26 × 26 μm2) for high temporal resolution images and 64 × 64 pixel2 (10 × 10 μm2) for high spatial resolution images, with 50% overlap are chosen to procure U.
Data availability
The data that support the findings of this study are available from the corresponding authors upon reasonable request.
References
Larson, R. G. Instabilities in viscoelastic flows. Rheol. Acta 31, 213–263 (1992).
Shaqfeh, E. S. G. Purely elastic instabilities in viscometric flows. Annu. Rev. Fluid. Mech. 28, 129–185 (1996).
Groisman, A. & Steinberg, V. Elastic turbulence in a polymer solution flow. Nature 405, 53–55 (2000).
Groisman, A. & Steinberg, V. Efficient mixing at low Reynolds numbers using polymer additives. Nature 410, 905–908 (2001).
Groisman, A. & Steinberg, V. Elastic turbulence in curvilinear flows of polymer solutions. New J. Phys. 6, 29 (2004).
Toms, B. A. Some observation on the flow of linear polymer solutions through straight tubes at large reynolds numbers. Proc. Cong. Rheol. 2, 135–141 (1948).
Balkovsky, E., Fouxon, A. & Lebedev, V. Turbulence of polymer solutions. Phys. Rev. E 64, 056301 (2001).
Fouxon, A. & Lebedev, V. Spectra of turbulence in dilute polymer solutions. Phys. Fluids 15, 2060–2072 (2003).
Alfvén, H. Existence of Electromagnetic-Hydrodynamic Waves. Nature 150, 405–406 (1942).
Landau, L. D., Lifshitz, E. M. & Pitaevskii, L. P. Electrodynamics of Continuous Media, 2nd edn. (Elsevier, Oxford, 1984).
Landau, L. D. & Lifshitz, E. M. Fluid Mechanics, 2nd edn. (Elsevier, Oxford, 1987).
Berti, S. & Boffetta, G. Elastic waves and transition to elastic turbulence in a two-dimensional viscoelastic Kolmogorov flow. Phys. Rev. E 82, 036314 (2010).
Grilli, M., Vázquez-Quesada, A. & Ellero, M. Transition to turbulence and mixing in a viscoelastic fluid flowing inside a channel with a periodic array of cylindrical obstacles. Phys. Rev. Lett. 110, 174501 (2013).
Arora, K., Sureshkumar, R. & Khomami, B. Experimental investigation of purely elastic instabilities in periodic flows. J. Non-Newton. Fluid Mech. 108, 209–226 (2002).
Varshney, A. & Steinberg, V. Elastic wake instabilities in a creeping flow between two obstacles. Phys. Rev. Fluids 2, 051301(R) (2017).
Varshney, A. & Steinberg, V. Mixing layer instability and vorticity amplification in a creeping viscoelastic flow. Phys. Rev. Fluids 3, 103303 (2018).
Vázquez-Quesada, A. & Ellero, M. SPH simulations of a viscoelastic flow around a periodic array of cylinders confined in a channel. J. Non-Newton. Fluid Mech. 167-168, 1–8 (2012).
Afik, E. Measuring elastic properties of flow in dilute polymer solutions. M.Sc. Thesis, Weizmann Institute of Science (2009).
Jun, Y. & Steinberg, V. Power and pressure fluctuations in elastic turbulence over a wide range of polymer concentrations. Phys. Rev. Lett. 102, 124503 (2009).
Bird, R. B., Armstrong, R. C. & Hassager, O. Dynamics of Polymeric Liquids, Vol. 1, Fluid Mechanics, 2nd edn. (Wiley-Interscience, New York, 1987).
Liu, Y. & Steinberg, V. Molecular sensor of elastic stress in a random flow. Europhys. Lett. 90, 44002 (2010).
Rodd, L. E., Cooper-White, J. J., Boger, D. V. & McKinley, G. H. Role of the elasticity number in the entry flow of dilute polymer solutions in micro-fabricated contraction geometries. J. Non-Newton. Fluid Mech. 143, 170–191 (2007).
Shi, X., Kenney, S., Chapagain, G. & Christopher, G. F. Mechanisms of onset for moderate Mach number instabilities of viscoelastic flows around confined cylinders. Rheol. Acta 54, 805–815 (2015).
Burghelea, T., Steinberg, V. & Diamond, P. H. Internal viscoelastic waves in a circular Couette flow of a dilute polymer solution. Europhys. Lett. 60, 704 (2002).
Liu, Y., Jun, Y. & Steinberg, V. Concentration dependence of the longest relaxation times of dilute and semi-dilute polymer solutions. J. Rheol. 53, 1069–1085 (2009).
Thielicke, W. & Stamhuis, E. PIVlab-Towards user-friendly, affordable and accurate digital particle image velocimetry in MATLAB. J. Open Res. Softw. 2, p.e30 (2014).
Acknowledgements
We thank Guy Han and Yuri Burnishev for technical support. A.V. acknowledges support from the European Union’s Horizon 2020 research and innovation programme under the Marie Skłodowska-Curie grant agreement No. 754411. This work was partially supported by the Israel Science Foundation (ISF; grant #882/15) and the Binational USA-Israel Foundation (BSF; grant #2016145).
Author information
Authors and Affiliations
Contributions
A.V. and V.S. designed the experiment. A.V. performed the measurements and together with V.S. analyzed the data. Both authors discussed the results and wrote the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Journal peer review information: Nature Communications thanks the anonymous reviewers for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Varshney, A., Steinberg, V. Elastic Alfven waves in elastic turbulence. Nat Commun 10, 652 (2019). https://doi.org/10.1038/s41467-019-08551-0
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-019-08551-0
This article is cited by
-
Universal properties of non-Hermitian viscoelastic channel flows
Scientific Reports (2023)
-
Towards Predicting the Onset of Elastic Turbulence in Complex Geometries
Transport in Porous Media (2022)
-
Active open-loop control of elastic turbulence
Scientific Reports (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.