Introduction

Fundamental symmetry constraints determine allowable collective states in solids1. Of particular recent interest is broken inversion symmetry in non-centrosymmetric materials, which can lead to hidden topological order and odd-parity superconductivity1,2,3. With the added consideration of broken time-reversal invariance, exotic magnetic states can emerge. A quantum critical point, where quantum fluctuations tie together competing ground states, is a fertile region to investigate and manipulate intertwined charge, spin, and structural degrees of freedom under different symmetry conditions. However, many experimental systems either manifest first-order quantum phase transitions without critical behavior, as exemplified by itinerant ferromagnets4, or simply follow mean-field behavior5,6. Strongly-coupled systems with pronounced spin-orbit interactions provide a potential means to move to non-trivial criticality in three dimensions4,5. This scenario has been proposed for all-in-all-out (AIAO) antiferromagnetic order on a pyrochlore lattice4, but remains to be identified experimentally.

The AIAO arrangement of spins on the pyrochlore lattice is an unusual form of magnetism that preserves the underlying cubic lattice symmetry. With strong spin-orbit coupling and low itinerant electron density, compounds with AIAO spin order are desirable candidates to explore non-trivial quantum critical behavior in three dimensions5. AIAO spin order has been observed in FeF3, Nd2Zr2O7, A2Ir2O7 (A = Sm, Eu, and Nd), and Cd2Os2O7 (refs.7–12) and suggested for additional A2Ir2O7 systems with A = Y, Lu, Gd, Tb, Dy, Ho, and Yb13. For our purposes, Cd2Os2O7 is most desirable. With the transition temperature (TN = 227 K) roughly 60% higher than all other A2Ir2O7 members, Cd2Os2O7 should demonstrate the strongest correlation effects. Moreover, among spin-orbit coupled 5d compounds, only Cd2Os2O7 and Nd2Ir2O7 consistently manifest both an antiferromagnetic insulating phase and a metallic paramagnetic phase with dρ/dT > 0 (refs.13–15). It remains unclear whether iridates such as Eu2Ir2O7 and Sm2Ir2O7 are metallic or insulating in the paramagnetic phase, presumably due to vacancies and site disorder from the 3+/4+ valence condition16. By comparison, Cd2Os2O7 exhibits less site disorder from its 2+/5+ valence condition, with no extraneous spin order arising from the A site of the A2(Ir,Os)2O7 structure14. Both pressure14,17,18 and chemical tuning15,18 of the A site drive the insulating transition to lower temperature in the A2(Ir,Os)2O7 compounds, but little is known about the behavior of the AIAO magnetic order.

Here, to address this issue directly, we present results on the evolution of the spin, orbit, and lattice degrees of freedom, using polarization analysis of resonantly diffracted X-rays from Cd2Os2O7 under diamond anvil cell pressures. This resonant diffraction technique is highly challenging with regard to both maintaining the single-crystal quality at cryogenic temperatures and tens of giga-Pascal pressures, and efficiently detecting weak magnetic diffraction signals from miniature samples of 5 × 10−5 mm3 size. Nevertheless, AIAO magnetic order and 5d orbital order can be resolved directly and tracked to the highest pressures under resonant conditions in the polarization switching π−σ channel of the (6, 0, 0) and (4, 2, 0) forbidden lattice orders, respectively9. The lattice symmetry and space group assignments were detected via the π–π’ scattering channel.

Results

The magnetic quantum phase transition

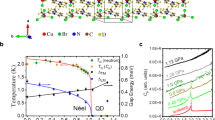
We summarize in Fig. 1 the symmetry evolution under pressure. At ambient pressure, Cd2Os2O7 has the pyrochlore structure of the Fd-3m space group (No. 227). Optical Raman scattering maps out a uniform Fd-3m phase up to P = 29 GPa and between T = 10 and 300 K. The phonon modes characteristic of that space group develop smoothly over the entire range (Fig. 2). The overall cubic symmetry is further verified by X-ray diffraction up to 41 GPa at 4 K (Fig. 3), as lineshapes of the (1, 1, 1), (2, 2, 2), (0, 2, 2), (0, 4, 4), and (4, 0, 0) diffraction orders remain single peak. The cubic lattice constant decreases smoothly under pressure, without discernable discontinuity at the quantum critical point Pc = 35.8 GPa (Fig. 1, discussed below). While the Fd-3m space group is uniquely determined by the unit cell’s lattice constant and one free coordinate x for oxygen position on 48f sites that characterizes the trigonal distortion of the OsO6 cluster14,19, diffraction intensities at (1, 1, 1) and (0, 2, 2) show a continuous evolution through the quantum phase transition, with x increasing by a small amount from 0.319 at P = 0 (ref.14) to ~0.325 at Pc (Fig. 3b, c). Neither the AIAO antiferromagnetic order nor the continuous space group evolution within the cubic symmetry (Fig. 1) would be detectable by macroscopic approaches such as electrical transport. Instead, we address these issues using resonant single crystal diffraction with polarization analysis at the Os L2 edge.

Fig. 1
figure 1

Symmetries across the P–T phase diagram. The Cd2Os2O7 lattice retains its cubic symmetry throughout the probed P–T phase space, but continuously transitions between Fd-3m and F-43m space groups. The Fd-3m lattice symmetry was verified by optical Raman scattering from 0–29 GPa and 10–300 K (gray crosses), while both phases of magnetism and structure (pink and blue shading) were inferred from X-ray diffraction measurements at T = 4 K. The metallic paramagnetic phase in the low-pressure Fd-3m space group has both spatial inversion (\(\cal{I}\)) and time reversal (\(\cal{T}\)) symmetries. For the spin degrees of freedom, time reversal symmetry is broken in the low-pressure AIAO phase. On the high-pressure side, the F-43m space group breaks the spatial inversion symmetry, introduces a tetrahedral breathing distortion (inset), and restores the time reversal symmetry with disordered spins. The coincidence of continuous magnetic and structural phase transitions at a single quantum critical point suggests strong coupling between spin, orbit, lattice, and (potentially) charge degrees of freedom in this 5d pyrochlore compound

Full size image
Fig. 2
figure 2

Optical Raman scattering. Raw Raman spectra of Cd2Os2O7 at various temperatures from 10 to 300 K and our highest measured pressure, P ~ 29 GPa. We only observe the six modes consistent with the Fd-3m space group. The T2g(3) and A1g modes merge under their broadened peaks

Full size image
Fig. 3
figure 3

Lattice evolution under pressure. a Pressure evolution of the lattice constant (red solid circles) was fit to a two-parameter Birch equation of state with B = 190.4 ± 3.6 GPa, and B’ = 4.2 ± 0.2. Vertical error bars represent 1σ s.d. counting statistics, and horizontal error bars represent the full range of pressure inhomogeneity during a measurement. (insets) Longitudinal (θ/2θ) scans of (1, 1, 1) and (0, 2, 2) orders measured at various pressures using 12.387 keV X-rays verify the cubic symmetry. b–c Pressure evolution of integrated diffraction intensities of (0, 2, 2) and (1, 1, 1) orders, normalized by (0, 4, 4) and (2, 2, 2) orders, respectively. The measurement was performed under either resonant (E = 12.387 keV, solid circles) or off-resonant (E = 12.355 keV, open circles) conditions. These two orders are sensitive to O 48 f sites in the unit cell, and develop in opposite fashion up to 40 GPa. d Simulated (0, 2, 2) and (1, 1, 1) intensities as a function of x. The overall percentage changes of (0, 2, 2) and (1, 1, 1) give an x increasing from 0.319 at P = 0 (ref.14) to approximately 0.325 at Pc

Full size image

Resonant diffraction at both (6, 0, 0) and (4, 2, 0) in the π−σ and π−π’ polarization channels are displayed in Fig. 4 for pressures that traverse the quantum phase boundary. The magnetic diffraction intensity I(6, 0, 0) in the π−σ channel at E = 12.387 keV, which scales to the ordered staggered moment <m> as I ~ <m>2, decreases continuously with increasing pressure (Fig. 5a). The scatter in the data makes it difficult to identify definitively the quantum critical region, but a phenomenological fit of the intensity data as I~ <m>2~(Pc–P)2β over the whole pressure range gives a critical pressure Pc = 35.8 ± 0.7 GPa and an exponent β = 0.40 ± 0.04 for the order parameter <m>. From energetic considerations of localized 3d-5d spins20,21, our magnetic diffraction results at the low temperature limit of 4 K also provide a means to estimate the AIAO magnetic phase boundary through TN ~ ΔL ~ <m>2, where ΔL is the external magnetostriction that develops under <m>. In AIAO-ordered pyrochlores where the cubic lattice symmetry is preserved by the magnetic order and the phase transition is continuous, the external magnetostriction ΔL is difficult to observe over the lattice’s thermal expansion. Nevertheless, a non-monotonic evolution of the lattice constant with temperature, a(T), for AIAO order at very low T has been demonstrated in Nd2Ir2O7 (TN = 33.5 K and Δa/a ~1 10−4)22. Following this logic, we identify the magnetic phase boundary TN(P) of Cd2Os2O7 in Fig. 1 via the relationship TN ~ I ~ <m>2. At high pressure, for P > Pc, the presence of a charge resonance at (4, 2, 0) verifies that the unoccupied t2g orbitals remain the same in promoting the resonance behavior (Fig. 4e, f). At the same time, the absence of a resonance at (6, 0, 0) importantly marks the vanishing of the staggered moment <m> and the long-range antiferromagnetic order (Fig. 4c).

Fig. 4
figure 4

Polarization-sensitive resonant diffraction data under pressure. Raw energy scan profiles at both (a–c) (6, 0, 0) and (d–f) (4, 2, 0) orders from two separate polarization channels (π–σ in red/pink and π–π’ in navy/aqua solid circles), expressed in counts s−1 for 100 mA synchrotron storage ring current. The azimuthal angel ψ relative to the (0, 0, 1) vector is specified for each order. At (6, 0, 0), the π–σ channel manifests magnetic resonant spectra whose intensity decreases continuously with pressure. Beyond 36 GPa (c), the sharp resonance disappears, and a background similar to the Os L2 absorption edge shape remains, which comes from X-ray fluorescence that passed through the polarization analyzer. Its origin is demonstrated by a comparison with an energy scan at (5.98, 0, 0) (gray line, c), where no magnetic diffraction is expected, and also with an Os L2 absorption spectrum at 39.3 GPa (black line, c). The fluorescence part of the background intensity is dependent on the detector slit size. At (4, 2, 0), the π–σ channel reveals the orbital ordering via the anisotropic tensor susceptibility resonance. For both (6, 0, 0) and (4, 2, 0) orders, the π-π’ charge diffraction intensities rise dramatically at pressures above the magnetic phase boundary, with a small leakage into the π-σ channel becoming apparent in f through the polarization analyzer (Methods)

Full size image
Fig. 5
figure 5

Continuous magnetic and structural quantum phase transitions. a Magnetic diffraction intensity was measured at (6, 0, 0) and in the π−σ channel, with a power law fit (solid line) to model the evolution over the whole pressure range. b Lattice diffraction intensities, measured at both the (6, 0, 0) and (4, 2, 0) orders in the π–π’ channel, indicate a continuous switching between the Fd-3m and F-43m space groups with a phase boundary that rises effectively exponentially. All intensities were measured at 4.0 ± 0.5 K with integration of sample mosaic profiles at specified azimuthal angle ψ using 12.387 keV X-rays. Vertical error bars represent 1σ s.d. counting statistics, and horizontal error bars represent the full range of pressure inhomogeneity during a measurement

Full size image

The lattice quantum phase transition

With the disappearance of AIAO order at the magnetic critical point Pc, the charge diffraction starts to gain intensity in the π–π’ channel for both (6, 0, 0) and (4, 2, 0) (Fig. 4), and grows continuously for P > Pc in an effectively exponential manner (Fig. 5b). We emphasize that these are forbidden diffraction orders in the Fd-3m space group. The energy dependence manifests no resonance, but is instead consistent with the inverted shape of the Os L2 absorption edge. At 41 GPa, 15% above Pc, there is no diffraction intensity at forbidden orders (3, 0, 0), (5, 0, 0), and (2, 1, 1). With preserved cubic symmetry, F-43m is the only maximal non-isomorphic subgroup of the original Fd-3m space group that is consistent with the measured diffraction results. We note that the pressure-induced AIAO quantum phase transition in Cd2Os2O7 differs from the chemical tuning scenario of the A2Ir2O7 series (A from Sm to Pr), where the lattice inversion symmetry is preserved throughout13.

The space group F-43m breaks lattice inversion symmetry, opening possibilities for a number of exotic states. We did not detect further symmetry breaking such as the small tetragonal distortion in Cd2Re2O7 that would remove the three-fold rotational symmetry23. The broken inversion symmetry derives from differently sized adjacent tetrahedra of the Os and Cd sublattices, which are no longer centrosymmetric and can be regarded as fully-softened breathing modes3 with spontaneous symmetry breaking. These breathing modes disappear above Tc ~ K|ΔOs|2, with the lower F-43m symmetry replaced by the higher Fd-3m symmetry with adjacent tetrahedra of equal size. Here K is the vibrational elastic constant and ΔOs is the amplitude of the Os tetrahedron breathing mode. We expect Tc ~ I, given that the measured X-ray diffraction intensities (Fig. 5b) depend on these lattice distortions as I ~ |f OsΔOs + fCdΔCd|2, where fOs/Cd are X-ray atomic form factors. Hence Tc increases two orders of magnitude in a short pressure interval: from approximately 5 K at 37 GPa to ~500 K at 41 GPa, leading to the striking concave high-pressure phase boundary in Fig. 1.

Discussion

The reduction of lattice symmetry from Fd-3m to F-43m in Cd2Os2O7 has direct implications for phonon coupling3 to spin fluctuations at the quantum critical point. Although spin-phonon coupling is known to generate unequal bond lengths in spin-Peierls dimers and antiferromagnetic superlattices20, the breathing phonons are not fully softened in the AIAO phase and, at least in the static limit, AIAO order only induces an external magnetostriction with a homogenous expansion22. In the high-pressure phase, a breathing lattice could in principle still permit AIAO spin configurations on different sized tetrahedra, despite a loss of site inversion symmetry in the F-43m space group. Nevertheless, as no long-range commensurate antiferromagnetic order was observed experimentally (Fig. 4c), the magnetic ground state at high pressure is likely spin-disordered, as ferromagnetically interacting Ising moments along local <1,1,1> axes would generate a high level of frustration7. Tuning by either chemical doping or pressure drives the ratio of the magnetic interaction strength to the hopping integral smaller in A2(Os,Ir)2O7. With an increasing electron density under 15% volume reduction by pressure, and moving away from the strong interaction strength limit13, one would not naturally expect a ferromagnetic ground state. Furthermore, ferromagnetic quantum phase transitions, as well as commensurate-incommensurate antiferromagnetic transitions, are first order, which would contrast with the continuous AIAO quantum phase transition observed in our experiment.

The increased bandwidth under pressure suggests that the electronic properties of Cd2Os2O7 in the high-pressure F-43m state become more metallic, potentially even superconducting in analogy to superconducting Cd2Re2O7 with its broken inversion symmetry (ref.23). The relationship between the spin and charge transitions is also intriguing. AIAO magnetic order and the metal-insulator transition respond similarly to compression across the P–T phase diagram. Our projected magnetic phase boundary in Fig. 1 gives dTN/dP ~ −5.0 K GPa−1 at P = 0, which is consistent with dTMIT/dP = −4 K GPa−1 in Cd2Os2O7 measured over the first 2 GPa range14. Comparing the two 5d AIAO ordered compounds with clear high-temperature metallic states, we find an average dTN/dP ~ −6.5 K GPa−1 over the whole AIAO phase in Cd2Os2O7 and a dTMIT/dP ~ −5.8 K GPa−1 in Nd2Ir2O717,18. This comparison holds true despite large differences in TN of 227 K and 33.5 K, respectively.

The experimentally observed coincidence of magnetic and structural phase transitions at Pc = 35.8 GPa, along with the similarities in the pressure evolution of the spin and charge degrees of freedom, point to one critical point within our experimental resolution (~1 GPa in the region around Pc) between the insulating AIAO order and the spin-disordered metallic phase. As Landau’s phase transition theory would dictate two quantum phase transitions to be separated or to share a first-order phase line. If the transitions are separated, then our results point to a narrow intermediate region where the various spin, charge, and structural modes can couple across phases. If the phase boundaries actually meet at one critical point, then we may be in the realm of deconfined quantum criticality24. In any case, the concurrence of magnetic and structural phase transitions within our experimental resolution, and the clear deviation from mean-field behavior of the high-pressure phase, stimulate a discussion of strong coupling in the quantum critical region.

From the band structure perspective, the Os 5d t2g band in Cd2Os2O7 is neither degenerate and forming a S = 3/2 state under Hund’s rule, as indicated by the reduced staggered moment <m> = 0.59 μB Os−1, nor cleanly separated into several narrow bands as demonstrated for a perfect OsO6 octahedron14,25,26. Instead, the t2g orbitals in Cd2Os2O7 extend continuously over a spectral width of order 2 eV from the combined effect of U (~1 eV)27, spin-orbit coupling (~0.35 eV)26, and trigonal distortion (~0.3 eV) on the OsO6 octahedron19. Through the continuous quantum phase transition, the overall stability of the empty t2g band is verified by the constant charge resonance profile at (4, 2, 0), with a coarse energy resolution slightly above 1 eV. From a metallic paramagnetic state, the formation of antiferromagnetic order would influence the oscillating dynamic component of the quasiparticle self-energy28, and in turn introduce a spin-order dependent repulsion between empty and filled states that could account for the insulating state below Pc. This effective exchange field due to neighboring opposite spins is a generalized Slater mechanism, even without introducing Brillouin zone folding28. If the gap opens through a critical state of singular points at the Fermi surface, instead of a removal of states altogether, its thermodynamics could fit the Lifshitz description27,29.

The essentially concurrent, continuous quantum phase transitions of antiferromagnetism, structure, and (apparently) charge in Cd2Os2O7 provides the necessary ingredients for a generic approach to strongly-coupled, non-mean-field quantum criticality in three-dimensions5. With the Fermi surface fully gapped, quasiparticle fluctuations would involve all itinerant states in reducing the screening on Coulomb U, and the increased interaction range would then help stabilize a continuous quantum phase transition5. Indeed, at the ambient-pressure metal-insulator transition in Cd2Os2O7, an increase in U from 0.8 to 1.5 eV in the theoretical modeling is consistent with the observed spectral weight shift in infrared conductivity over the broad range of 0–4 eV (ref.30). Above the quantum critical point and in the spin-disordered phase space of the Fd-3m space group, soft AIAO spin fluctuations and lattice breathing modes could exist and compete, and further couple to quasiparticle fluctuations. The competition between spin and lattice degrees of freedom might explain the remarkable concave-shaped phase line at high pressure, as Tc scales to pressure with a non-trivial exponent much larger than one, a characteristic of strongly-coupled quantum criticality5. We note as well that the quantum critical region is asymmetric in P–T phase space, as the magnetic and structural phase lines approach Pc with different asymptotic behavior.

The quantum phase transition in Cd2Os2O7, with its interwoven spin, orbit, lattice, and charge degrees of freedom, contrasts sharply with systems that have a partially gapped Fermi surface, exemplified by itinerant spin density waves where persistent carriers screen spin fluctuations and lead to mean-field behavior4,5,6,31. The cubic AIAO antiferromagnet also differs from itinerant ferromagnets, where strong spin and charge mode coupling at wave vector q = 0 categorically induces first-order quantum phase transitions4. Spin-orbit coupling in 5d systems is regarded as intermediately strong13, and pressure drives U/t smaller with increasing kinetic energy t, away from the strong-correlation limit. Pressure tuning thus likely induces a continuous quantum phase transition while still preserving non-trivial quantum criticality in this 5d antiferromagnet. By Luttinger’s theorem, a continuous insulator-metal transition would result in either a carrier-mass enhancement or non-Fermi liquid behavior32 in Cd2Os2O7’s high-pressure phase, and the broken inversion symmetry sets the conditions for odd-parity superconductivity. A microscopic theory remains to be developed to describe the interaction between the AIAO spin fluctuations, breathing phonon modes, and quasiparticle excitations, especially taking into consideration the symmetry, chirality, and wave vector characteristics of each.

Methods

Resonant X-ray diffraction under high-pressure

Both charge orbital order and magnetic order can induce resonant behavior at an absorption edge in X-ray diffraction9,12,31. At the Os L2 edge, these two types of resonances share the empty part of the t2g band as the intermediate state, and exhibit the same resonance profiles. The charge resonance originates from the anisotropic tensor susceptibility. In general, it can be observed at many forbidden lattice orders and, specifically, at both (4, 2, 0) and (6, 0, 0) in Cd2Os2O7. To avoid the charge anisotropic tensor susceptibility resonance and to isolate the magnetic resonance of the AIAO order, X-ray diffraction of the (6, 0, 0) order in Cd2Os2O7 was performed with a limited azimuthal angle range around 45° relative to the (0, 0, 1) vector, using a horizontal diffraction geometry for both π–σ and π–π’ polarization analyses9.

For the diamond anvil cell high-pressure environment33, we used a transmission (Laue) diffraction geometry in contrast to the typical reflection (Bragg) geometry at ambient pressure9. To achieve the diffraction geometry with the specified azimuthal condition at (6, 0, 0), single crystal samples were prepared in thin plate form with a surface normal along (0, −1, 1). This allows access to other diffraction orders such as (1, 1, 1), (4, 0, 0), (0, 2, 2), and (4, 2, 0) within the confined diamond anvil cell geometry, with the forbidden order (4, 2, 0) providing access to the charge-based anisotropic tensor susceptibility resonance. Several plates were polished down to 13 μm thickness, equivalent to one absorption length in Cd2Os2O7 for X-rays at the Os L2 edge (E = 12.387 keV). Unlike the iridates, resonance behavior at both the L2 and L3 edges are present in osmates. We chose the resonance at the L2 edge at high P because the higher energy X-rays had a longer X-ray penetration length through both the pressure environment and the sample.

We used the low-temperature, high-pressure diffraction setup at beamline 4-ID-D of the Advanced Photon Source33. To reduce absorption and enhance the signal-to-background ratio, a pair of wide-angle perforated Boehler diamond anvils33 (SYNTEK Co. LTD., Japan) were used, with culet size varying from 800 to 550 μm. A methanol/ethanol 4:1 mixture was used as the pressure medium inside rhenium gaskets. Pressure was calibrated by a Ag manometer in situ at 4.0 ± 0.5 K using a two-parameter Birch equation of state, with B0 = 108.85 GPa and B’ = dB/dP = 5.7 over the large pressure range. For X-ray polarization analysis, a highly oriented pyrolytic graphite (HOPG) plate of 5 mm thickness and 0.35° FWHM mosaic was used as the polarization analyzer. The (0, 0, 10) diffraction order of graphite at the Os L2 edge of 12.387 keV introduces a leakage of approximately 1.3% of the intensity from the π–π’ channel to the π–σ channel, and vice versa. Data presented here were collected from a total of 8 samples under pressure for spin (7) and charge (4) resonances. The absence of high-pressure commensurate antiferromagnetic order was verified on two crystals.

Optical Raman scattering

Shards of single crystal Cd2Os2O7 with original growth surfaces were individually loaded with a Neon pressure medium in a diamond anvil cell, and subsequently thermally cycled in a liquid helium flow cryostat. Optical Raman scattering was performed using a Horiba LabRam HR Evolution system in the MRSEC facilities at the University of Chicago, equipped with a 633 nm wavelength laser for excitation.

Data availability

The data that support the findings of this study are available from the corresponding authors upon request.