Abstract
A quantum spin liquid state has long been predicted to arise in spin-1/2 Heisenberg square-lattice antiferromagnets at the boundary region between Néel (nearest-neighbor interaction dominates) and columnar (next-nearest-neighbor interaction dominates) antiferromagnetic order. However, there are no known compounds in this region. Here we use d10–d0 cation mixing to tune the magnetic interactions on the square lattice while simultaneously introducing disorder. We find spin-liquid-like behavior in the double perovskite Sr2Cu(Te0.5W0.5)O6, where the isostructural end phases Sr2CuTeO6 and Sr2CuWO6 are Néel and columnar type antiferromagnets, respectively. We show that magnetism in Sr2Cu(Te0.5W0.5)O6 is entirely dynamic down to 19 mK. Additionally, we observe at low temperatures for Sr2Cu(Te0.5W0.5)O6—similar to several spin liquid candidates—a plateau in muon spin relaxation rate and a strong T-linear dependence in specific heat. Our observations for Sr2Cu(Te0.5W0.5)O6 highlight the role of disorder in addition to magnetic frustration in spin liquid physics.
Similar content being viewed by others
Introduction
Antiferromagnetic interactions on simple geometric lattices, such as triangular, square or tetrahedral, can give rise to magnetic frustration, because not all interactions between neighboring spins can be satisfied. These frustrated magnets have been widely studied in the search for exotic ground states such as quantum spin liquid (QSL) and quantum spin ice1. The square lattice has been of special interest due to its connection to high-temperature superconductivity2. Frustrated magnetism on a square lattice can be described using the spin-1/2 Heisenberg square-lattice model (J1–J2 model). This model has two interactions: nearest-neighbor interaction J1 along the side of the square and next-nearest-neighbor interaction J2 along the diagonal of the square (Fig. 1a). The J1–J2 model has three classical ground states: ferromagnetic (FM), Néel antiferromagnetic (NAF) and columnar antiferromagnetic (CAF) order. The Néel order occurs when the J1 interaction is antiferromagnetic and dominates (J2/J1 « 0.5), while the columnar order requires a dominant antiferromagnetic J2 interaction (J2/J1 » 0.5)3.
Spin-1/2 Heisenberg square-lattice model in Sr2CuTeO6 and Sr2CuWO6. a Phase diagram of the J1–J2 square-lattice model. J1 is the nearest-neighbor interaction and J2 the next-nearest-neighbor interaction. The classical ground states are ferromagnetic (FM), Néel antiferromagnetic (NAF) and columnar antiferromagnetic (CAF) ordering. The highly frustrated J2/J1 ≈ 0.5 and J2/J1 ≈ –0.5 regions are located at the NAF–CAF and CAF–FM boundaries, respectively. b The double perovskite structure of Sr2CuTeO6 and Sr2CuWO6. Sr, Cu, B” (Te/W) and O are represented by green, blue, dark yellow and red spheres, respectively. The blue (dark yellow) octahedra represent CuO6 (B”O6). c The Néel antiferromagnetic structure of Sr2CuTeO6 and the columnar antiferromagnetic structure of Sr2CuWO6 with the view down the c-axis. The dominant antiferromagnetic interactions are shown
The nature of the ground state in the highly frustrated region at the NAF–CAF boundary near J2/J1 ≈ 0.5 is under debate. Anderson4 famously proposed that a QSL state emerges when Néel order is frustrated by including an antiferromagnetic J2 interaction. Quantum spin liquids are highly entangled states, in which spins remain dynamic even at absolute zero1, 5. Experimental QSL candidates are known with several different structure types6,7,8,9,10,11, typically Kagomé lattices, but a square-lattice QSL has not been realized. The other ground state suggested for the J2/J1 ≈ 0.5 region is a valence bond solid12,13,14, in which spins form dimer or plaquette singlets with a static pattern. Despite these theoretical predictions for the square-lattice antiferromagnets, no experimental evidence of a compound in the J2/J1 ≈ 0.5 region exists.
Isostructural A2B’B”O6 double perovskite antiferromagnets Sr2CuTeO6 and Sr2CuWO6, where A = Sr2+, B’ = Cu2+ and B” = Te6+/W6+ (Fig. 1b), are well described by the J1–J2 model15,16,17,18,19,20. A Jahn–Teller distortion and an accompanying orbital ordering result in a square lattice of Cu2+ (S = 1/2) ions with highly two-dimensional magnetic interactions15, 21. The two B” cations, Te6+ and W6+, have nearly the same size22, and thus the bond distances and angles in Sr2CuTeO6 and Sr2CuWO6 are very similar21. Nevertheless, the diamagnetic B” cation has a significant effect on the magnetic properties. Recent neutron scattering studies have revealed NAF ordering at TN = 29 K with J1 = −7.18 and J2 = −0.21 meV (J2/J1 = 0.03) for Sr2CuTeO6, whereas Sr2CuWO6 has CAF ordering at TN = 24 K with J1 = –1.2 and J2 = –9.5 meV (J2/J1 = 7.92)18, 19, 23, 24 (Fig. 1c). This dramatic change in exchange interactions is driven by differences in orbital hybridization. In Sr2CuWO6, the dominant 180° Cu–O–W–O–Cu J2 exchange pathway is enabled by significant W 5d0–O 2p hybridization19, 25. In contrast, the filled 4d10 states in Sr2CuTeO6 are core-like and do not hybridize18, 25, resulting in a weak J2. The origin of the dominant 90° J1 interaction is under debate: Babkevich et al.18 proposed a Cu–O–O–Cu exchange pathway without a contribution from Te, whereas Xu et al.25 proposed that some Te 5p–O 2p hybridization does occur affecting the J1 interaction. Since Sr2CuTeO6 has a dominant J1 interaction and Sr2CuWO6 a dominant J2, it is natural to ask whether the J2/J1 ≈ 0.5 region could be reached by making a Sr2Cu(Te1-xW x )O6 solid solution.
Recently, Zhu et al.26 showed that Te6+–W6+ (d10–d0) cation mixing can be used to tune the magnetic ground state of Cr3+ (S = 3/2) inverse trirutiles Cr2TeO6 and Cr2WO6. Similar to Sr2CuWO6, W 5d0–O 2p hybridization in Cr2WO6 allows an exchange pathway not observed in Cr2TeO6, resulting in different magnetic structures for the two compounds. Magnetic interactions can be tuned by making a Cr2(Te1-xW x )O6 solid solution; a change in magnetic structure occurs at x = 0.7. Differences in the magnetic properties of isostructural d10 and d0 compounds have also been observed in perovskite-like Ni2+ (S = 1)27, 28 and Os6+ (S = 1)29 compounds.
Here we show that the magnetic ground state of a spin-1/2 square-lattice antiferromagnet can be tuned by d10–d0 cation mixing. In the solid solution Sr2Cu(Te0.5W0.5)O6 spins remain entirely dynamic down to 19 mK. This represents a suppression of TN by at least three orders of magnitude compared to the antiferromagnetic parent phases. Moreover, the magnetic specific heat shows T-linear behavior at low temperatures, despite the material itself being an insulator. These results indicate a spin-liquid-like ground state. A special property of Sr2Cu(Te0.5W0.5)O6 is the high amount of quenched disorder in the magnetic interactions.
Results
Crystal structure
Polycrystalline samples of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6 with crystallite size in the micrometer range were synthesized via a conventional solid state reaction. Sample color ranged from light green to yellow, indicating that the materials are insulating. This was confirmed with a room-temperature four-probe electrical conductivity measurement. Xray diffraction analysis found the samples to be of high quality with a trace SrWO4 impurity in Sr2Cu(Te0.5W0.5)O6 and Sr2CuWO6; the relatively stable SrWO4 is a common impurity in Sr2CuWO615,21. Sr2Cu(Te0.5W0.5)O6 retains the I4/m double perovskite structure of the parent phases with little difference in lattice parameters or bond distances; Rietveld refinement results are presented in Supplementary Fig. 1 and Supplementary Table 1. Cation order with respect to B’ (Cu2+) and B” (Te6+/W6+) sites is complete within experimental accuracy, but tellurium and tungsten are randomly distributed on the B” site. This results in quenched disorder in the J1 and J2 interactions between the Cu2+ ions.
Magnetic properties
Magnetic properties of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6 are summarized in Table 1. DC magnetic susceptibilities as a function of temperature are presented in Fig. 2. The zero-field cooled (ZFC) and field cooled (FC) curves fully overlap in all samples, and therefore we present only the ZFC results. The magnetic susceptibilities of Sr2CuTeO6 and Sr2CuWO6 do not feature a cusp at TN. Instead, in all three compounds we observe a broad maximum in the susceptibility, which is a common feature of two-dimensional magnets and QSL candidates5. This maximum can be characterized by two parameters: its position Tmax and height χmax. In the frustrated region of the square-lattice model near J2/J1 ≈ 0.5 Tmax is predicted to be lower than in either the NAF (J2/J1 « 0.5) or CAF (J2/J1 » 0.5) regions30,31. Our magnetic data are consistent with this theoretical prediction: Tmax in Sr2Cu(Te0.5W0.5)O6 shifts to a lower temperature than in Sr2CuTeO6 or Sr2CuWO6. This would place Sr2Cu(Te0.5W0.5)O6 close to the highly frustrated region, although the structural disorder present in Sr2Cu(Te0.5W0.5)O6 is not included in the theoretical model. In the related solid solution series Sr2Cu(W1-xMo x )O6, where both end members have a dominating J2 interaction and less frustration is expected, Tmax depends linearly on composition and never goes below those of the end members15,17. A Curie tail is observed in Sr2Cu(Te0.5W0.5)O6 at low temperatures. This is likely to be from a paramagnetic impurity, which are known to be relatively common in the end phases15,16,17,24.
Magnetic susceptibility. DC magnetic susceptibility of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6 measured in a 1 T field. Néel temperatures of Sr2CuTeO6 and Sr2CuWO6 are marked with TN, whereas the position of the maximum in magnetic susceptibility is marked with Tmax. Zero-field cooled and field cooled curves fully overlap and only the former is shown. Inset: Inverse magnetic susceptibility and fits to Curie–Weiss law
Magnetic susceptibilities were fitted to the Curie–Weiss law χ = C / (T − Θcw), where C is the Curie constant and Θcw is the Weiss constant. The inverse susceptibilities deviate from the linear Curie–Weiss behavior below relatively high temperatures of ≈ 200 K (inset in Fig. 2). For this reason, we performed the fitting in the temperature range 250–300 K. The Weiss constant Θcw gives an indication of the total strength of magnetic interactions in a material. For Sr2Cu(Te0.5W0.5)O6 we obtain Θcw = −71 K revealing mainly antiferromagnetic interactions similar in strength to those in Sr2CuTeO6 (Θcw = −80 K). In contrast, the antiferromagnetic interactions in Sr2CuWO6 are significantly stronger with Θcw = −165 K. Effective paramagnetic moments obtained from the Curie–Weiss fits are essentially the same for all samples and typical for Cu2+ (Table 1). In DC susceptibility, the ZFC and FC curves were found to overlap for all samples, which indicates the lack of a spin glass transition. AC susceptibility of Sr2Cu(Te0.5W0.5)O6 was measured (Supplementary Fig. 2) to support this conclusion. No frequency dependent peak was observed in the real part χ’ (dispersion) of the AC susceptibility indicating that Sr2Cu(Te0.5W0.5)O6 is not a spin glass. Moreover, the imaginary part χ” (absorption) remains practically zero.
Specific heat
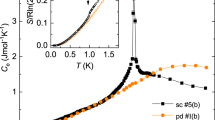
Results of specific heat measurements of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6 are shown in Fig. 3a. Similar to the magnetic susceptibility, TN cannot be simply determined from the specific heat of Sr2CuTeO6 or Sr2CuWO6 as no lambda anomalies are observed. Likewise, no lambda anomaly is seen for Sr2Cu(Te0.5W0.5)O6 down to 2 K. Moreover, we do not observe a low-temperature maximum typical of spin-gapped systems such as valence bond solids32,33 or the valence bond glass Ba2YMoO634. The main difference between the compounds is that the reduced specific heat capacities of Sr2CuTeO6 and Sr2CuWO6 approach zero with decreasing temperature, as is expected for insulators, whereas the reduced specific heat of Sr2Cu(Te0.5W0.5)O6 appears to remain finite.
Specific heat measurements. a Specific heat of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6. Inset: Low-temperature Cp/T vs. T2 plot. b Specific heat of Sr2Cu(Te0.5W0.5)O6, lattice standard Sr2Zn(Te0.5W0.5)O6 and the subtracted magnetic specific heat of Sr2Cu(Te0.5W0.5)O6. c Log–log plot of magnetic specific heat of Sr2Cu(Te0.5W0.5)O6. The red line is a fit to γTα, which yields α = 1.02(1) confirming T-linear behavior. d Magnetic specific heat (black) and integrated entropy (blue) of Sr2Cu(Te0.5W0.5)O6
At temperatures below ≈ 10 K, linear behavior is observed in a Cp/T vs. T2 plot (inset in Fig. 3a). Specific heat in the range 2–10 K was fitted using the function Cp = γT + βDT3, where γ is the T-linear electronic term and βD the Debye-like phononic term. The T-linear γ terms obtained were 54.2(5), 2.2(2) and 0.7(4) mJ/molK2 for Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6, respectively. Sr2Cu(Te0.5W0.5)O6 has a notably large γ term for an insulator with no free electrons. There are two main possibilities for a significant γ term in an insulator. In a gapless quantum spin liquid the γ term arises from collective excitations of entangled spins8,35. On the other hand, the γ term is also an archetypical feature of spin glasses. In spin glasses, γ is associated with intrinsic spin disorder36. The γ term can also develop above the spin freezing temperature TF37,38.
The specific heat of Sr2Cu(Te0.5W0.5)O6 was also measured in a field of μ0H = 8 T (Fig. 3a) and found to be nearly identical with the zero field measurements; a γ term of 56.5(7) mJ/molK2 was obtained. This lack of magnetic field dependency rules out a spin glass state39, since both specific heat and the γ term depend on the applied field in spin glasses37. The lack of field dependency indicates that the γ term could be related to a fermionic density of states as is the case in quantum spin liquids35. The properties of the predicted spin-liquid state at J2/J1 = 0.5 are under debate. Recent theoretical work suggests either a gapless Z2 QSL40,41,42 or a topological e.g. gapped Z2 QSL43,44. Sr2Cu(Te0.5W0.5)O6 appears to have gapless excitations based on our specific heat measurements, but the existence of a small spin gap14 cannot be ruled out.
For comparison, we measured the specific heat of similar double perovskite solid solutions (Supplementary Fig. 3). In non-magnetic Sr2Zn(Te0.5W0.5)O6, the reduced specific heat approaches zero with decreasing temperature, yielding a small γ term of 0.2(1) mJ/molK2. This shows that the finite electronic term is related to the magnetism of Cu2+ ions. We also measured the molybdenum analog Sr2Cu(Mo0.5W0.5)O6, where both end members Sr2CuWO6 and Sr2CuMoO6 have a dominant J2 interaction and thus less frustration is expected17. Here too the reduced specific heat approaches zero as temperature is lowered and the electronic term is small, 0.7(4) mJ/molK2. We conclude that the significant T-linear term in the specific heat of Sr2Cu(Te0.5W0.5)O6 is related to magnetic frustration and not solely to structural disorder.
An estimate of the magnetic specific heat of Sr2Cu(Te0.5W0.5)O6 (Fig. 3b) was obtained by subtracting the specific heat of the closest non-magnetic analog Sr2Zn(Te0.5W0.5)O6 (Fig. 3b). Unfortunately, the structure of Sr2ZnTe0.5W0.5O6 is slightly different, because it lacks the Jahn–Teller distortion present in Sr2Cu(Te0.5W0.5)O6. This and weighting errors lead to some uncertainty in the removal of the phononic contribution, and thus we have scaled the lattice standard data to match the specific heat of both compounds in the high-temperature paramagnetic region34. The magnetic specific heat of Sr2Cu(Te0.5W0.5)O6 increases with temperature up to ≈ 20 K. In a gapless quantum spin liquid a linear increase in magnetic specific heat is expected at low temperatures35,39. In order to conclusively show this linear relationship in Sr2Cu(Te0.5W0.5)O6, we present a log–log plot of magnetic specific heat as a function of temperature (Fig. 3c). A low-temperature fit to γTα yields α = 1.02(1), confirming the T-linear dependence of magnetic specific heat and γ = 55(1) mJ/K consistent with the Cp/T vs. T2 fit. Magnetic entropy was integrated (Fig. 3d) and found to reach 2.51 J/molK at 90 K. This represents 44% of the expected Rln(2) for spin-1/2. Similar values have been reported for some other QSL candidates39,45,46. The large amount of spin entropy retained at low temperatures is a common feature of quantum spin liquids39.
Muon spin rotation and relaxation
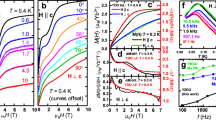
No magnetic ordering or spin freezing could be observed for any of the samples in either magnetic susceptibility or specific heat measurements. For this reason, we measured muon spin rotation and relaxation (μSR) of Sr2Cu(Te0.5W0.5)O6. μSR is a sensitive local probe of static and dynamic magnetism, and has been previously used to trace the onset of antiferromagnetic order in Sr2CuWO617. We measured the μSR signal in zero field (ZF), weak transverse field (wTF) and longitudinal field (LF) modes down to 19 mK. Representative spectra taken in ZF mode at various temperatures are shown in Fig. 4a. We have plotted the time dependent polarization Gz(t) of the initially 100% polarized muon spins measured via the asymmetry of decay positron count rates a(t) = a(t = 0)·Gz(t). Even at 19 mK there is no indication of a spontaneous muon spin rotation signal (oscillations of asymmetry) expected for a magnetically ordered system. In contrast, clear oscillations are observed below TN in Sr2CuWO617. Qualitatively, an increase in depolarization is observed with decreasing temperature. This means that the muon spins sense a distribution of local fields that is widened at lower temperatures. If these fields were of static origin, the distribution would correspond only to fields of a few 10−4 T, i.e., far less than expected for static local fields of Cu2+ such as the ≈ 0.1 T fields observed in Sr2CuWO617. Moreover, the curves are clearly different from those of a magnetically frozen static spin system, where one expects a residual polarization of Gz(t) ≈ 1/3 at long times. Thus, the muon spin relaxation appears to be mainly due to dynamic electronic spin fluctuations down to 19 mK.
μSR measurements of Sr2Cu(Te0.5W0.5)O6. a Zero-field muon spin relaxation function Gz(t) measured at different temperatures. b Muon spin rotation spectra a(t) measured with a 5 mT transverse-field at 19 mK and 0.5 K. c Muon spin relaxation rate λ and power β as a function of temperature from fits of the zero-field data using exp(-(λt)β) as the depolarization function. d Longitudinal-field muon spin relaxation function Gz(t) at 19 mK measured with different applied longitudinal fields. The error bars in a, b and d represent 1 s.d. and in (c) the maximum possible variation due to correlation of parameters
In order to conclusively rule out magnetic ordering, we measured muon spin precession in a weak transverse field of 5 mT (Fig. 4b). The onset of magnetic ordering of Cu2+ moments would cause a distinct loss of asymmetry in transverse field experiments due to randomly adding strong local magnetic fields to the weak applied transverse field. We find no loss of initial asymmetry a(t = 0) in the wTF experiments down to 19 mK. Damping increases upon decreasing temperature and tends to saturate below 0.5 K in parallel to the behavior observed in ZF (see below).
A quantitative description of the ZF spectra was obtained using a power law function Gz(t) = exp(−(λt)β) as the depolarization function, where λ is the relaxation rate and β is a power factor. This approach has also been used in the analysis of the Kagomé-lattice spin-liquid system SrCr8Ga4O1947. The fits shown in Fig. 4a were obtained using this phenomenological approach, which should be applicable when the field fluctuation rates are larger than the damping rates by static fields. The spectra above 2 K reveal close to exponential damping with β = 1, indicating fast fluctuating local fields, whereas β increases from 1 to ≈1.8 (Fig. 4c) at lower temperatures as seen from the more Gaussian-like appearance of the spectra. A Gaussian shaped (β = 2) depolarization function is typical for a static Gaussian local field distribution caused by disordered magnetic moments in the nearest neighbors of muons. The observed increase of β shows that the fluctuation rates of local fields are slowing down at lower temperatures. The relaxation rate λ increases at lower temperatures (Fig. 4c), which also corresponds to a decrease in the local field fluctuation rates sensed by the muon spins. At very low temperatures, between 0.5 K and 19 mK, λ levels are off. Notably, this plateau in λ is expected behavior in quantum spin liquid candidates9,48,49.
Further proof of the persistence of relatively fast electronic spin dynamics even at 19 mK is provided by LF measurements (Fig. 4d). Longitudinal fields can suppress muon spin depolarization caused by weaker local static fields. Weak randomly oriented static fields of the order of mT or less are typically due to nuclear dipole moments (in the present case mainly from 63Cu and 65Cu nuclei). Depolarization by fast fluctuating local fields from atomic spins, however, may only be affected by much larger applied fields. The LF spectra in Fig. 4d show that a 5 mT LF is only enough for a partial suppression of the depolarization of muon spins. This means that these muon spins are in positions where weak static random local fields from nearby nuclear spins are acting. The depolarization of the majority of muon spins, however, is only gradually reduced by much higher fields. Depolarization becomes nearly completely suppressed by an applied LF of 1 T. This is typical for depolarization caused by fast fluctuating local fields. Whether the observation of two (or several) muon ensembles is related to local inhomogeneities in the solid solution or different muon sites in the lattice is an open question. This situation also precludes a detailed quantitative analysis of the muon spin relaxation under applied field using, e.g., Keren’s function involving a single fluctuation rate and a single static damping50. From the variation of damping as a function of applied field, we may, however, estimate the field fluctuation rates at 19 mK to be on the order of 80–100 MHz.
Discussion
We have successfully used d10–d0 cation mixing to tune the magnetic ground state of the spin-1/2 square-lattice antiferromagnet Sr2Cu(Te0.5W0.5)O6, which retains the double perovskite structure of its parent phases Sr2CuTeO6 and Sr2CuWO6. The broad maximum in the magnetic susceptibility shifts to a lower temperature in the solid solution indicating increased frustration. The specific heat of Sr2Cu(Te0.5W0.5)O6 has a significant T-linear term of γ = 54.2(5) mJ/molK2 despite the phase being an insulator. A complete lack of static magnetism down to 19 mK is revealed by muon spin rotation and relaxation measurements. This corresponds to a frustration factor f = |Θcw|/TN of over 3700. Moreover, the muon spin relaxation rate has a clear plateau at low temperatures. Our experimental results therefore indicate a spin-liquid-like ground state in Sr2Cu(Te0.5W0.5)O6. This is the first observation of such a ground state in a square-lattice compound.
The origin of the spin-liquid-like state in Sr2Cu(Te0.5W0.5)O6 remains unclear. The J1–J2 model provides an appealingly simple explanation for our experimental observations, as they are consistent with the Z2 gapless quantum spin liquid state predicted for J2/J1 = 0.5. However, neither the average values of magnetic interactions J1 and J2 nor the applicability of the model itself due to disorder is known at this time. An alternative origin could be in the combination of disorder and magnetic frustration in the material. Disorder and magnetic frustration are inherently linked in Sr2Cu(Te0.5W0.5)O6: each elementary Cu2+ square, or plaquette, has randomly either a Te6+ (d10) or a W6+ (d0) cation in its center promoting a dominant J1 or J2 interaction, respectively. As a consequence, Sr2Cu(Te0.5W0.5)O6 has very significant quenched disorder in the magnetic interactions between spin-1/2 sites. Disorder has been shown to induce a gapless spin-liquid state in a spin-1/2 triangular compound51. Recently, Kawamura and coworkers52,53,54,55 proposed that a disorder-induced gapless quantum spin liquid state, a so-called random-singlet state, forms on spin-1/2 triangular, Kagomé and honeycomb lattices when there is a high amount of randomness in the magnetic interactions in addition to frustration. They suggested that the random-singlet state could be found in many frustrated compounds with disorder in a wide parameter range without needing an exact match of magnetic interactions55. Sr2Cu(Te0.5W0.5)O6, with its unique combination of significant disorder and magnetic frustration, could be a realization of this random-singlet state on the square lattice.
The d10–d0 cation mixing approach utilized here could be used to tune the ground state in other quantum materials such as those close to a quantum critical point. In this work, we have utilized the similar size of d10 Te6+ and d0 W6+ cations. We can identify the additional d10/d0 cation pairs of Zn2+/Mg2+, Cd2+/Ca2+, In3+/Sc3+ and Sb5+/Nb5+ based on ionic radii22 and previous work by Marjerrison and coworkers29.
Methods
Sample synthesis
Polycrystalline powders of Sr2Cu(Te0.5W0.5)O6, Sr2CuTeO6 and Sr2CuWO6 were prepared by a solid-state reaction method. Stoichiometric amounts of SrCO3, CuO, TeO2 and WO3 (all 99.995% or greater purity, Alfa Aesar) were thoroughly ground in an agate mortar with ethanol. The samples were calcined at 900 °C in air for 12 h, thoroughly ground, pressed into pellets, and fired at 1050 °C in air for 72 h with intermittent grindings.
Xray diffraction
Crystal structure and phase purity were evaluated by powder xray diffraction. Diffraction patterns were collected at room temperature with a Panalytical X’Pert Pro MPD instrument (Cu Kα1 radiation). Refinement of the crystal structure was carried out using FULLPROF56 software. Crystallite size was evaluated using line broadening analysis57. The crystal structures were visualized using VESTA58.
Magnetic characterization
Magnetic properties were investigated using a Quantum Design MPMS XL SQUID magnetometer. 100 mg of the samples were enclosed in gelatin capsules and placed in plastic straws. DC magnetic susceptibility was measured in zero-field cooled and field cooled modes between 5 K and 300 K in an applied field of μ0H = 1 T. AC susceptibility measurements were performed using a Quantum Design PPMS-9 on a 41.5 mg pressed pellet sample of Sr2Cu(Te0.5W0.5)O6. The sample was cooled in zero magnetic field and then measured at a range of frequencies between 215 and 9899 Hz, with an AC amplitude of 1 mT and a DC field of 0.5 T, as a function of temperature.
Specific heat
Specific heat capacity measurements were done with a Quantum Design PPMS between 2 K and 150 K using a thermal relaxation method. The samples were pieces of pellets with masses of 8–10 mg. Specific heat was measured in zero field and for Sr2Cu(Te0.5W0.5)O6 also in μ0H = 8 T. Magnetic specific heat of Sr2Cu(Te0.5W0.5)O6 was obtained by subtracting the specific heat of Sr2Zn(Te0.5W0.5)O6 after scaling the latter to fit the high-temperature paramagnetic part.
Muon spin rotation and relaxation (µSR)
The experiments were performed on a polycrystalline powder sample of Sr2Cu(Te0.5W0.5)O6 using the 100% spin polarized surface muon beam at the Dolly (3He cryostat down to 0.25 K) and LTF (3He/4He dilution refrigerator down to 19 mK) facilities of the Swiss Muon Source at the Paul Scherrer Institut, Switzerland. The measurements were made in zero-field, longitudinal field (along initial muon spin) and transverse field (perpendicular to initial muon spin) modes.
Data availability
All the data supporting the conclusions of this article are available from the authors upon reasonable request.
References
Balents, L. Spin liquids in frustrated magnets. Nature 464, 199–208 (2010).
Keimer, B., Kivelson, S. A., Norman, M. R., Uchida, S. & Zaanen, J. From quantum matter to high-temperature superconductivity in copper oxides. Nature 518, 179–186 (2015).
Misguich, G. & Lhuillier, C. in Frustrated Spin Systems (ed Diep, H. T.) 229–306 (World Scientific, 2004).
Anderson, P. W. The resonating valence bond state in La2CuO4 and superconductivity. Science 235, 1196–1198 (1987).
Zhou, Y., Kanoda, K. & Ng, T.-K. Quantum spin liquid states. Rev. Mod. Phys. 89, 025003 (2017).
Lee, S.-H. et al. Quantum-spin-liquid states in the two-dimensional kagome antiferromagnets ZnxCu4−x(OD)6Cl2. Nat. Mater. 6, 853–857 (2007).
Fåk, B. et al. Kapellasite: A kagome quantum spin liquid with competing interactions. Phys. Rev. Lett. 109, 037208 (2012).
Clark, L. et al. Gapless spin liquid ground state in the S=1/2 vanadium oxyfluoride kagome antiferromagnet [NH4]2[C7H14N][V7O6F18]. Phys. Rev. Lett. 110, 207208 (2013).
Balz, C. et al. Physical realization of a quantum spin liquid based on a novel frustration mechanism. Nat. Phys. 12, 942–949 (2016).
Terasaki, I. et al. Absence of magnetic long range order in Ba3ZnRu2O9: a spin-liquid candidate in the S=3/2 dimer lattice. J. Phys. Soc. Jpn. 86, 033702 (2017).
Dutton, S. E. et al. Quantum spin liquid in frustrated one-dimensional LiCuSbO4. Phys. Rev. Lett. 108, 187206 (2012).
Read, N. & Sachdev, S. Valence-bond and spin-Peierls ground states of low-dimensional quantum antiferromagnets. Phys. Rev. Lett. 62, 1694–1697 (1989).
Zhitomirsky, M. E. & Ueda, K. Valence-bond crystal phase of a frustrated spin-1/2 square-lattice antiferromagnet. Phys. Rev. B 54, 9007–9010 (1996).
Gong, S.-S., Zhu, W., Sheng, D. N., Motrunich, O. I. & Fisher, M. P. A. Plaquette ordered phase and quantum phase diagram in the spin-1/2 J1-J2 square Heisenberg model. Phys. Rev. Lett. 113, 027201 (2014).
Vasala, S., Cheng, J.-G., Yamauchi, H., Goodenough, J. B. & Karppinen, M. Synthesis and characterization of Sr2Cu(W1-xMox)O6: A quasi-two-dimensional magnetic system. Chem. Mater. 24, 2764–2774 (2012).
Koga, T., Kurita, N. & Tanaka, H. Strong suppression of magnetic ordering in an S=1/2 square-lattice Heisenberg antiferromagnet Sr2CuTeO6. J. Phys. Soc. Jpn. 83, 115001 (2014).
Vasala, S. et al. Characterization of magnetic properties of Sr2CuWO6 and Sr2CuMoO6. Phys. Rev. B 89, 134419 (2014).
Babkevich, P. et al. Magnetic excitations and electronic interactions in Sr2CuTeO6: A spin-1/2 square lattice Heisenberg antiferromagnet. Phys. Rev. Lett. 117, 237203 (2016).
Walker, H. C. et al. Spin wave excitations in the tetragonal double perovskite Sr2CuWO6. Phys. Rev. B 94, 064411 (2016).
Vasala, S. & Karppinen, M. A2B′B″O6 perovskites: A review. Prog. Solid State Chem. 43, 1–36 (2015).
Iwanaga, D., Inaguma, Y. & Itoh, M. Crystal structure and magnetic properties of B-site ordered perovskite-type oxides A2CuB’O6 (A=Ba, Sr; B’=W, Te). J. Solid State Chem. 147, 291–295 (1999).
Shannon, R. D. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Cryst. A32, 751–767 (1976).
Vasala, S., Avdeev, M., Danilkin, S., Chmaissem, O. & Karppinen, M. Magnetic structure of Sr2CuWO6. J. Phys. Condens. Matter 26, 496001 (2014).
Koga, T. et al. Magnetic structure of the S=1/2 quasi-two-dimensional square-lattice Heisenberg antiferromagnet Sr2CuTeO6. Phys. Rev. B 93, 054426 (2016).
Xu, Y. et al. Comparative description of magnetic interactions in Sr2CuTeO6 and Sr2CuWO6. J. Phys. Condens. Matter 29, 105801 (2017).
Zhu, M. et al. Tuning the magnetic exchange via a control of orbital hybridization in Cr2(Te1-xWx)O6. Phys. Rev. Lett. 113, 076406 (2014).
Paria Sena, R., Hadermann, J., Chin, C.-M., Hunter, E. C. & Battle, P. D. Structural chemistry and magnetic properties of the perovskite SrLa2Ni2TeO9. J. Solid State Chem. 243, 304–311 (2016).
Chin, C.-M., Paria Sena, R., Hunter, E. C., Hadermann, J. & Battle, P. D. Interplay of structural chemistry and magnetism in perovskites; a study of CaLn2Ni2WO9; Ln=La, Pr, Nd. J. Solid State Chem. 251, 224–232 (2017).
Marjerrison, C. A. et al. Magnetic ground states in the three Os6+ (5d2) double perovskites Ba2MOsO6 (M=Mg, Zn, and Cd) from Néel order to its suppression. Phys. Rev. B 94, 134429 (2016).
Shannon, N., Schmidt, B., Penc, K. & Thalmeier, P. Finite temperature properties and frustrated ferromagnetism in a square lattice Heisenberg model. Eur. Phys. J. B 38, 599–616 (2004).
Richter, J., Lohmann, A., Schmidt, H.-J. & Johnston, D. C. Magnetic susceptibility of frustrated spin-s J1-J2 quantum Heisenberg magnets: High-temperature expansion and exact diagonalization data. J. Phys. Conf. Ser. 529, 012023 (2014).
Weihong, Z., Hamer, C. J. & Oitmaa, J. Series expansions for a Heisenberg antiferromagnetic model for SrCu2(BO3)2. Phys. Rev. B 60, 6608–6616 (1999).
Kageyama, H. et al. Low-temperature specific heat study of SrCu2(BO3)2 with an exactly solvable ground state. J. Exp. Theor. Phys. 90, 129–132 (2000).
De Vries, M. A., McLaughlin, A. C. & Bos, J.-W. G. Valence bond glass on an fcc lattice in the double perovskite Ba2YMoO6. Phys. Rev. Lett. 104, 177202 (2010).
Yamashita, S. et al. Thermodynamic properties of a spin-1/2 spin-liquid state in a κ-type organic salt. Nat. Phys. 4, 459–462 (2008).
Mydosh, J. A. Spin glasses: redux: an updated experimental/materials survey. Rep. Prog. Phys. 78, 052501 (2015).
Meschede, D., Steglich, F., Felsch, W., Maletta, H. & Zinn, W. Specific heat of insulating spin-glasses, (Eu,Sr)S, near the onset of ferromagnetism. Phys. Rev. Lett. 44, 102–105 (1980).
LaForge, A. D., Pulido, S. H., Cava, R. J., Chan, B. C. & Ramirez, A. P. Quasispin glass in a geometrically frustrated magnet. Phys. Rev. Lett. 110, 017203 (2013).
Yamashita, S., Nakazawa, Y., Ueda, A. & Mori, H. Thermodynamics of the quantum spin liquid state of the single-component dimer Mott system κ-H3(Cat-EDT-TTF)2. Phys. Rev. B 95, 184425 (2017).
Hu, W.-J., Becca, F., Parola, A. & Sorella, S. Direct evidence for a gapless Z2 spin liquid by frustrating Néel antiferromagnetism. Phys. Rev. B 88, 060402 (2013).
Wang, L., Poilblanc, D., Gu, Z.-C., Wen, X.-G. & Verstraete, F. Constructing a gapless spin-liquid state for the spin-1/2 J1-J2 Heisenberg model on a square lattice. Phys. Rev. Lett. 111, 037202 (2013).
Richter, J., Zinke, R. & Farnell, D. J. J. The spin-1/2 square-lattice J1-J2 model: the spin-gap issue. Eur. Phys. J. B 88, 2 (2015).
Mezzacapo, F. Ground-state phase diagram of the quantum J1-J2 model on the square lattice. Phys. Rev. B 86, 045115 (2012).
Jiang, H.-C., Yao, H. & Balents, L. Spin liquid ground state of the spin-1/2 square J1-J2 Heisenberg model. Phys. Rev. B 86, 024424 (2012).
Cheng, J. G. et al. High-pressure sequence of Ba3NiSb2O9 structural phases: New S=1 quantum spin liquids based on Ni2+. Phys. Rev. Lett. 107, 197204 (2011).
Zhou, H. D. et al. Spin Liquid State in the S=1/2 Triangular Lattice Ba3CuSb2O9. Phys. Rev. Lett. 106, 147204 (2011).
Uemura, Y. J. et al. Spin fluctuations in frustrated Kagomé lattice system SrCr8Ga4O19 studied by muon spin relaxation. Phys. Rev. Lett. 73, 3306–3309 (1994).
Mendels, P. et al. Quantum magnetism in the paratacamite family: towards an ideal Kagomé lattice. Phys. Rev. Lett. 98, 077204 (2007).
Quilliam, J. A. et al. Gapless quantum spin liquid ground state in the spin-1 antiferromagnet 6HB-Ba3NiSb2O9. Phys. Rev. B 93, 214432 (2016).
Keren, A. Generalization of the Abragam relaxation function to a longitudinal field. Phys. Rev. B 50, 10039–10042 (1994).
Furukawa, T. et al. Quantum spin liquid emerging from antiferromagnetic order by introducing disorder. Phys. Rev. Lett. 115, 077001 (2015).
Watanabe, K., Kawamura, H., Nakano, H. & Sakai, T. Quantum spin-liquid behavior in the spin-1/2 random Heisenberg antiferromagnet on the triangular lattice. J. Phys. Soc. Jpn. 83, 034714 (2014).
Kawamura, H., Watanabe, K. & Shimokawa, T. Quantum spin-liquid behavior in the spin-1/2 random-bond Heisenberg antiferromagnet on the kagome lattice. J. Phys. Soc. Jpn. 83, 103704 (2014).
Shimokawa, T., Watanabe, K. & Kawamura, H. Static and dynamical spin correlations of the S=1/2 random-bond antiferromagnetic Heisenberg model on the triangular and kagome lattices. Phys. Rev. B 92, 134407 (2015).
Uematsu, K. & Kawamura, H. Randomness-induced quantum spin liquid behavior in the s=1/2 random J1–J2 Heisenberg antiferromagnet on the honeycomb lattice. J. Phys. Soc. Jpn. 86, 044704 (2017).
Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B 192, 55–69 (1993).
Rodríguez-Carvajal, J. & Roisnel, T. Line broadening analysis using FullProf*: determination of microstructural properties. Mater. Sci. Forum 443–444, 123–126 (2004).
Momma, K. & Izumi, F. VESTA3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 44, 1272–1276 (2011).
Acknowledgements
Dr. J-C. Orain is thanked for technical assistance with the μSR measurements at Dolly. Dr. E.M. Bittar is thanked for the use of laboratory facilities. We thank Dr. G. Stenning for help with AC susceptibility measurements in the Materials Characterization Laboratory at the ISIS Neutron and Muon Source. O.M. is grateful for an Aalto CHEM funded doctoral student position. S.V. is thankful for the support of the Brazilian funding agencies CNPq (grants no. 150503/2016-4 and 152331/2016-6) and FAPERJ (grant no. 202842/2016). F.J.L., E.S. and E.B-S. are grateful for financial support by a joint DFG-FAPERJ project DFG Li- 244/12. In addition, E.B-S. acknowledges support from FAPERJ through several grants, including Emeritus Professor fellow and CNPq for BPA and corresponding grants.
Author information
Authors and Affiliations
Contributions
O.M., S.V. and M.K. conceived and planned the study. The samples were synthesized by O.M. and S.V. Structural characterization and analysis was performed by O.M. and S.V. Magnetic properties were measured by O.M. and H.C.W. Specific heat was measured by S.V. and E.B-S. Magnetic and specific heat data were analyzed and interpreted by O.M., S.V., H.C.W., I.T., E.B-S. and M.K. Muon spin rotation and relaxation was measured by O.M., E.S., K.P.S., C.B. and F.J.L. with the main analysis by F.J.L. and K.P.S. O.M., S.V. and F.J.L. wrote the manuscript with contributions from all authors.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Electronic supplementary material
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Mustonen, O., Vasala, S., Sadrollahi, E. et al. Spin-liquid-like state in a spin-1/2 square-lattice antiferromagnet perovskite induced by d10–d0 cation mixing. Nat Commun 9, 1085 (2018). https://doi.org/10.1038/s41467-018-03435-1
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s41467-018-03435-1
This article is cited by
-
Observation of Mermin-Wagner behavior in LaFeO3/SrTiO3 superlattices
Nature Communications (2024)
-
Candidate spin-liquid ground state in CsNdSe2 with an effective spin-1/2 triangular lattice
Communications Materials (2024)
-
Strain-tuned incompatible magnetic exchange-interaction in La2NiO4
Communications Physics (2024)
-
Superexchange-stabilized long-distance Cu sites in rock-salt-ordered double perovskite oxides for CO2 electromethanation
Nature Communications (2024)
-
Classical spin liquid state in a rhombic lattice metal-organic framework
Nano Research (2024)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.