Abstract
In all archetypical reported (001)-oriented perovskite heterostructures, it has been deduced that the preferential occupation of two-dimensional electron gases is in-plane dxy state. In sharp contrast to this, the investigated electronic structure of a spinel-perovskite heterostructure γ-Al2O3/SrTiO3 by resonant soft X-ray linear dichroism, demonstrates that the preferential occupation is in out-of-plane dxz/dyz states for interfacial electrons. Moreover, the impact of strain further corroborates that this anomalous orbital structure can be linked to the altered crystal field at the interface and symmetry breaking of the interfacial structural units. Our findings provide another interesting route to engineer emergent quantum states with deterministic orbital symmetry.
Similar content being viewed by others
Introduction
Identifying the orbital symmetry of electrons near the Fermi edge is fundamentally important for understanding phase components of the order parameter,1–5 the coexistence of superconductivity and ferromagnetism,6–9 and the high mobility of interfacial conduction electrons.10–12 For example, at titanates based perovskite–perovskite interfaces, the preferential occupation of an in-plane dxy state by conduction electrons defines the isotropy of the Fermi surface13–25 of the two-dimensional (2D) electron gas. Theoretical calculations have predicted the possibility of out-of-plane dxz/dyz orbital symmetry of conduction carriers in titanates25 that can lead to an unusual one-dimensional (1D) electronic structure marked by either two orthogonal 1D bands26–28 with degenerate dxz/dyz orbitals or a single 1D band with non-degenerate dxz and dyz orbitals.12,19,29 Despite extensive experimental search thus far, however, this anomalous orbital configuration has not been observed at (001)-oriented interfaces.18
Very recently with the observation of interface enhanced high-temperature superconductivity (60–100 K)30,31 and high-mobility conduction electrons (~1.4×105 cm2 V−1 s−1)10 in SrTiO3 (STO)-based interfaces, probing the interactions between charge, spin, orbital, and structural degrees of freedom at the interfaces became fundamentally important to understand interface enhanced emergent electronic states. Specifically, the orbital symmetry of conduction carriers is primarily linked to the symmetry of the superconducting gap4 and the mobility of electrons.10–12 To this end, the orbital configuration, responsible for the high mobility of electrons in a spinel–perovskite interface (for example γ-Al2O3/SrTiO3, AlO/STO)10,11,32 is still an open question. From the experimental point of view, surface sensitive angle-resolved photoemission spectroscopy with polarized photons is a suitable probe of symmetry of the surface electronic structure,19 but it has limited applicability for the electronically active buried interfaces. In contrast to angle-resolved photoemission spectroscopy, interface sensitive linearly polarized X-ray absorption spectroscopy (XAS) has proven to be a powerful tool to resolve the orbital symmetry.6,9,13–18,33,34
In this work, using the AlO/STO heterostructure as a model system, we report on unique orbital symmetry and orbital occupancy, which is reversed compared with other well-known 2D electron gases based on perovskite titanates. Resonant soft X-ray linear dichroism (XLD) studies combined with direct current transport measurements have confirmed the orbital symmetry inversion driven by the altered crystal field at the interface and symmetry breaking of the TiO6 octahedral units.
Results
High-quality AlO/STO heterostructures were synthesized by pulsed laser deposition (see Supplementary Figure 1 and Materials and Methods for details). With its spinel structure, bulk γ-Al2O3 is cubic (space group ) with a lattice parameter a=7.911 Å,35,36 which is close to twice of the lattice parameter of bulk SrTiO3 a=3.905 Å. It is interesting to note that γ-Al2O3 is generally regarded as a defect spinel Al8/3O4 (32 oxygen ions, 64/3 Al cations, and 8/3 vacancies for one unit cell γ-Al2O3), which has the analogous structure to the conventional spinel MgAl2O4;36 owing to the Al vacancies, polar mismatch may be present at AlO/STO interfaces.37 The conductivity of the AlO/STO heterostructures used in this work is in good agreement with the previous reports,10,32,37–39 as shown in Supplementary Figure 2.
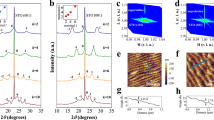
Figure 1 shows the spectra obtained by linearly polarized X-rays at the Ti L2,3-edge of AlO/STO heterostructures. The features of XAS spectra is the result of transitions from core levels to unoccupied valence states (e.g., 2p→3d for Ti L-edge). Owing to the crystal field, the Ti 3d state splits into t2g (dxy, dxz, and dyz) and eg ( and ) subbands with a crystal field gap as large as ~2 eV in the octahedral symmetry.9,13–18 In addition, the strong spin–orbit interaction induces the splitting of the Ti 2p core level into 2p1/2 and 2p3/2 states. Therefore, four main features are commonly observed in Ti L-edge XAS spectra (Figure 1b,c; Supplementary Figure 3). With lower crystal symmetry (e.g., tetragonal or orthorhombic symmetry as compared with cubic symmetry),19 the degeneracy of t2g and eg states can be further lifted, leading to an in-plane dxy subband with possibly lower energy than the out-of-plane dxz/dyz subband and available as the lowest energy state at the interface.9,13–17 To investigate the orbital configuration, XAS with linearly polarized X-rays, used in this work, has been proven to be one of the most powerful available probes applied to various interfaces.9,13–18 The utility of the probe stems from the strong dependence of absorption on the direction of the photon polarization vector (E) with respect to the crystal lattice axis (Figure 1a); Thus, excited by linearly polarized X-rays, electronic transitions from Ti core levels to the unoccupied d orbital bands contains important information about the orbital symmetry of those states. In general, when the linear X-ray polarization is oriented along the direction of unoccupied orbital lobes, the contribution of these orbitals to the XAS signal is largest.6,33 Therefore, the X-ray absorption at the Ti L2,3-edge for E||a b and E||c arises mainly from the unoccupied in-plane Ti (IV) and out-of-plane (IH) states, respectively. In general, the orbital character of a subband can be determined from the sign of XLD~(IH–IV).
Linearly polarized XAS of AlO/STO at Ti L2,3-edge. (a) Schematic of experimental setup. In-plane (IV, EV||ab and E is the linear polarization vector of the photon) and out-of-plane (IH, θ is the angle between EH and c) linearly polarized X-ray were used to measure XAS of AlO/STO (thickness of AlO film is ~5.5 unit cells or 4.35 nm) at Ti L2,3-edge with total electron yield (TEY, interface sensitive) detection mode at room temperature. The contribution of linearly polarized XAS signal at Ti L2,3-edge for t2g (or eg) band mainly arises from the unoccupied Ti dxy(or ) states by in-plane IV and dxz/dyz (or ) states by out-of-plane IH. Here the signal of XLD is defined as XLD=(IH−IV). (b) XAS at Ti L2,3-edge with normal incident angle θ=90°. Both EV||ab and EH||ab. (c) XAS at Ti L2,3-edge with grazing incident angle θ=20°. As seen in a, EV||ab, whereas EH||c. All collected spectra are repeated more than six times.
For precise determination of orbital polarization the knowledge of background can be important. To confirm the low background noise level of XLD and the absence of artifacts, a normal incidence geometry (θ=90°, Figure 1b) was utilized. In this geometry, both EH and EV are parallel to the interfacial plane and the intensities of linearly polarized XAS should be practically identical for both X-ray polarizations (i.e., (IH–IV)~0). As seen in Figure 1b, no significant XLD signal at Ti L2,3-edge is observed in agreement with the expectation.17 With the sample set at θ=20°, a strong XLD signal appears (~15% of XAS; Figure 1c) indicating the splitting of eg and t2g subbands with the lineshape that agrees well with the previous measurements and calculations.9,13–18
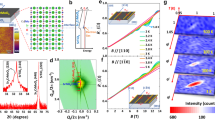
However, as seen in Figure 2, the XLD spectra for our AlO/STO system is atypical and has the reverse XLD lineshape compared with the results reported for prototypical 2D electron gases at titanate interfaces (e.g., LaAlO3/SrTiO3 (LAO/STO) and LaTiO3/SrTiO3 (LTO/STO)).9,14–17 Specifically, for the Ti t2g state of the perovsite–perovskite interfaces the negative sign of the first main XLD feature implies that the dxy subband is the lowest energy state in agreement with reported results.9,13–17 In sharp contrast to this, for AlO/STO, the sign of XLD is reversed (see blue and red arrows in Figure 2a), i.e., the first feature at ~457.85 eV has a positive sign, whereas the second feature at ~458.15 eV is negative, immediately implying that dxz/dyz orbitals are the first available states for interfacial electrons. Therefore, the relative energy position of Ti 3d subbands is unusual . In order to understand this anomalous behavior, epitaxial strain was induced by utilizing a large mismatch between the substrates and film (i.e., NdGaO3 (NGO) substrate for compressive strain ~−1.16 % and TbScO3 (TSO) substrate for tensile strain ~+1.29 %). As shown in Figure 2b, for the AlO/STO heterostructure on NGO (compressive strain) the lineshape of XLD is similar to that observed for AlO/STO except that the very first feature (at ~ 457.85 eV) is suppressed. However, for tensile strain on TSO, surprisingly almost all the spectral features are killed and no significant XLD signal is observed.
Symmetry inversion of XLD spectra in titanate interfaces. (a) The perovskite-perovskite interfaces (i.e., LaAlO3/SrTiO3 and LaTiO3/SrTiO3, red arrow) show negative sign for the first feature (at ~457.85 eV), whereas spinel-perovskite heterostructure (AlO/STO, blue arrow) displays positive sign indicating dxz/dyz is the preferential state of interfacial electrons for the later structure. The spectra of LaAlO3/SrTiO3 was adapted with permission from reference 16. Theoretically, to show the reversed lineshape of XLD for different orbital configurations, the calculation data were adapted with permission from reference 17. (b) Strain effects for AlO/STO/NGO and AlO/STO/TSO to XLD signal (compressive strain ~−1.16 % on NdGaO3 (NGO) and tensile strain ~+1.29 % on TbScO3 (TSO) substrates, respectively; thickness of STO layer is ~10 unit cells or 3.9 nm) and effects of oxygen vacancies in annealed STO single crystal to XLD signal. Comparing with the contributions from oxygen vacancies (annealed STO substrate) and bare STO substrate itself, the XLD signal at AlO/STO is robust. (Copyrighted by the American Physical Society).
Next, we quantify the strain effect on the splitting and peak energy shift (Figure 3). Generally, the size of the band splitting can be estimated from the peak energy difference of XAS obtained with linear polarized X-rays. First, we analyze the splitting of eg and t2g subbands at the AlO/STO interface. As shown in Figure 3a and Supplementary Figure 4, a direct comparison of the energy position for XAS with in-plane (IV) and out-of-plane (IH) orientation of the X-ray polarization reveal that the most pronounced XAS feature for IH is lower in energy than the IV absorption. For AlO/STO without external strain, it yields t2g (L3) band splitting Δt2g~50 meV and the eg(L3) band splitting Δeg~80 meV. Unexpectedly, as shown in Figure 3a, under tensile strain (~+1.29 %, on TSO substrate) the splitting of both t2g and eg bands is suppressed and practically vanished while the splitting is enhanced under compressive strain (~−1.16 %, NGO substrate). Compared to the band splitting of AlO/STO without external strain, the eg (L3) band splitting Δeg under compressive strain (~−1.16 %) increases from ~80 to 150 meV, whereas the t2g (L3) band splitting Δt2g~30 meV is only weakly decreased. Besides the subband splitting, strain also alters the peak energy position (Figure 3b). Specifically, for tensile strain though the splitting is strongly suppressed (Figure 3a; Supplementary Figure 4) the peak energy moves to the positive direction i.e., higher photon energies by ~+75 meV for t2g (L3) and +35 meV for eg (L3), respectively. On the other hand, as shown in Figure 3b, under compressive strain with enhanced band splitting, the four main peaks of Ti XAS at L2,3-edge shift towards negative direction by about −38 meV for t2g (L3) and −170 meV for eg (L3), respectively.
Strain effects of Ti 3d subband splitting and peak energy shift. (a) Subband splitting of Ti 3d state at AlO/STO interfaces under compressive strain ~−1.16 % (by NdGaO3 substrate, NGO), without external strain, and tensile strain ~+1.29 % (by TbScO3 substrate, TSO). (b) Relative peak energy shift (with unpolarized X-rays) of Ti L2,3-band in AlO/STO interfaces under compressive strain ~−1.16 % (negative energy direction) and tensile strain ~+1.29 % (positive energy direction).
Discussion
Next, we discuss the atomic structure of AlO/STO interfaces as a key factor to produce the inverse orbital symmetry. As schematically shown in Supplementary Figure 5, based on the interfacial atomic structure data,32,39 for the case of γ-Al2O3 spinel and in contrast to the previously reported perovskite–perovskite interfaces, the apical oxygen of Ti–O octahedra is not stable in a spinel–perovskite heterostructure. Thus, at the AlO/STO interface a unique Ti–O pyramid coordination is formed; in this distorted pyramid-like structure, dxz/dyz subband becomes the preferable state for the interfacial electrons (Supplementary Figure 5b,c). More importantly, the degenerate dxz/dyz subband can be further split owing to the cooperative efefct of spin–orbit coupling and crystal-field distortion, yielding an energy separation as large as 60–100 meV.12,19,29 Therefore, in contrast with all reported data on the (001)-oriented perovskite interfaces with in-plane dxy subband as the lowest energy state, the dxz or dyz subband becomes the lowest energy state for the case of spinel–perovskite heterojunction. As the consequence of the dxz or dyz orbital character of mobile electrons amplified by the spatial confinement along z,40,41 and regardless of which dxz or dyz is the preferred state, the forbidden electron hopping along the y (for dxz) or x (for dyz) direction may result in the emergence of the extremely anisotropic ‘1D’ electron gas (Supplementary Figure 5c).
Furthermore, to understand the impact of epitaxial strain on the XLD signals, we propose a simple model shown in Figure 4. As seen, under compressive strain (~−1.16%) the contraction of the in-plane four oxygens together with the elongation of the apical oxygen ion increases the energy of the in-plane and dxy orbitals, whereas the energy decreases for out-of-plane and dxz/dyz orbitals.42 As the result, the energy splitting Δeg between and orbitals, as well as the splitting Δt2g between dxy and dxz/dyz orbitals of Ti ions is increased. This model agrees well with the experimental observation that both Δeg and Δt2g under compressive strain are increased. On the other hand, under tensile strain, the elongation of the in-plane four oxygen ions and the contraction of the apical oxygen ion pulls the Ti ion inside the pyramid,42 leading to the reversed effect on the Ti 3d orbital sequence. Therefore, the energy splitting within both eg and t2g bands is expected to decrease; the corresponding XLD signal will be significantly suppressed due to the strain induced degeneracy.
In conclusion, we have demonstrated that in the spinel-perovskite heterostructure—AlO/STO the out-of-plane dxz/dyz states are the lowest lying energy states, which is in the sharp contrast to titanate based perovskite–perovskite heterostructures where the in-plane dxy state is always the ground state of the 2D conduction carriers. Moreover, the impact of strain corroborates that this unusual orbital configuration is directly linked with the altered crystal field at the interface and lattice symmetry breaking of the interfacial TiO6 octahedra. Our findings provide another interesting route to engineer unusual quantum states with deterministic orbital symmetry beyond those attainable in all (001)-oriented perovskite heterojunctions.
Materials and methods
Sample synthesis and characterization
Heterostructures γ-Al2O3 (~4.35 nm)/SrTiO3 (001), γ-Al2O3 (~4.35 nm)/SrTiO3 (~3.9 nm)/NdGaO3 (110), and γ-Al2O3 (~4.35 nm)/SrTiO3 (~3.9 nm)/TbScO3 (110) were layer-by-layer epitaxially grown with pulsed laser deposition (PLD), using a KrF excimer laser operating at λ=248 nm and 2 Hz pulse rate with 2 J/cm2 fluence. The layer-by-layer growth was monitored by in-situ reflection high-energy electron diffraction. During growth we utilized low oxygen pressure (~7.5×10−5 torr) and the temperature of the substrates was held at 700 °C. After growth, all samples were cooled at about 15 °C/min rate to room temperature keeping oxygen pressure constant. Annealed bulk SrTiO3 was prepared in vacuum (~1×10−6 torr) at 750 °C for 1 h. The lattice parameters of substrates are a=3.905 Å for SrTiO3 (STO); a=5.43 Å, b=5.50 Å, c=7.71 Å for NdGaO3 (NGO); a=5.46 Å, b=5.72 Å, c=7.91 Å for TbScO3 (TSO). The sheet-resistances of samples were measured in van-der-Pauw geometry by Physical Properties Measurement System (Quantum Design) from 300 to 2 K. X-ray diffraction was carried out at the 6-ID-B beamline of the Advanced Photon Source at Argonne National Laboratory.
Spectroscopy
XAS/XLD (at room temperature) at Ti L2,3-edge with total electron yield detection mode (interface sensitive) were carried out at beamline 4.0.2 of the Advanced Light Source (Lawrence Berkeley National Laboratory). In successive scans, spectra were captured with the order of polarization rotation reversed (e. g., horizontal, vertical, vertical, and horizontal) so as to eliminate systematic artifacts in the signal that drift with time. The residual artifact intensity is plotted in Figure 1 and labeled as background.
References
Tsuel, C. C. & Kirtley, J. R. Pairing symmetry in cuprate superconductors. Rev. Mod. Phys. 72, 969–1016 (2000).
Mackenzie, A. P. & Maeno, Y. The superconductivity of Sr2RuO4 and the physics of spin-triplet pairing. Rev. Mod. Phys. 75, 657–712 (2003).
Mazin, I. I. Superconductivity gets an iron boost. Nature 464, 183–186 (2010).
Leggett, A. J. What do we know about high T C? Nat. Phys. 2, 134–136 (2006).
Borisenko, S. V. et al. One-sign order parameter in iron based superconductor. Symmetry 4, 251–264 (2012).
Chakhalian, J., Freeland, J. W., Millis, A. J., Panagopoulos, C. & Rondinelli, J. M. Colloquium: Emergent properties in plane view: Strong correlations at oxide interfaces. Rev. Mod. Phys. 86, 1189–1202 (2014).
Dikin, D. A. et al. Coexistence of superconductivity and ferromagnetism in two dimensions. Phys. Rev. Lett. 107, 056802 (2011).
Michaeli, K., Potter, A. C. & Lee, P. A. Superconducting and ferromagnetic phases in SrTiO3/LaAlO3 oxide interface structures: possibility of finite momentum pairing. Phys. Rev. Lett. 108, 117003 (2012).
Lee, J. S. et al. Titanium d xy ferromagnetism at the LaAlO3/SrTiO3 interface. Nat. Mater. 12, 703–706 (2013).
Chen, Y. Z. et al. A high-mobility two-dimensional electron gas at the spinel/perovskite interface of γ-Al2O3/SrTiO3 . Nat. Commun. 4, 1371 (2013).
Chen, Y. Z. et al. Room temperature formation of high-mobility two-dimensional electron gases at crystalline complex oxide interfaces. Adv. Mater. 26, 1462–1467 (2014).
Fête, A. et al. Large modulation of the Shubnikov-de Haas oscillations by the Rashba interaction at the LaAlO3/SrTiO3 interface. New. J. Phys. 16, 112002 (2014).
Cao, Y. et al. Magnetic interactions at the nanoscale in trilayer titanates. Phys. Rev. Lett. 116, 076802 (2016).
Salluzzo, M. et al. Orbital reconstruction and the two-dimensional electron gas at the LaAlO3/SrTiO3 Interface. Phys. Rev. Lett. 102, 166804 (2009).
Salluzzo, M. et al. Structural and electronic reconstructions at the LaAlO3/SrTiO3 interface. Adv. Mater. 25, 2333–2338 (2013).
Salluzzo, M. et al. Origin of interface magnetism in BiMnO3/SrTiO3 and LaAlO3/SrTiO3 heterostructures. Phys. Rev. Lett. 111, 087204 (2013).
Pesquera, D. et al. Two-dimensional electron gases at LaAlO3/SrTiO3 Interfaces: Orbital symmetry and hierarchy engineered by crystal orientation. Phys. Rev. Lett. 113, 156802 (2014).
Herranz, G. et al. Engineering two-dimensional superconductivity and Rashba spin-orbit coupling in LaAlO3/SrTiO3 quantum wells by selective orbital occupancy. Nat. Commun. 6, 6028 (2015).
Santander-Syro, A. F. et al. Two-dimensional electron gas with universal subbands at the surface of SrTiO3 . Nature 469, 189–193 (2011).
Meevasana, W. et al. Creation and control of a two-dimensional electron liquid at the bare SrTiO3 surface. Nat. Mater. 10, 114–118 (2011).
McKeown Walker, S. et al. Control of a two-dimensional electron gas on SrTiO3(111) by atomic oxygen. Phys. Rev. Lett. 113, 177601 (2014).
Plumb, N. C. et al. Mixed dimensionality of confined conducting electrons in the surface region of SrTiO3 . Phys. Rev. Lett. 113, 086801 (2014).
Chang, Y. J. et al. Layer-by-layer evolution of a two-dimensional electron gas near an oxide interface. Phys. Rev. Lett. 111, 126401 (2013).
Zhong, Z., Tóth, A. & Held, K. Theory of spin-orbit coupling at LaAlO3/SrTiO3 interfaces and SrTiO3 surfaces. Phys. Rev. B 87, 161102(R) (2013).
Zhong, Z., Wissgott, P., Held, K. & Sangiovanni, G. Microscopic understanding of the orbital splitting and its tuning at oxide interfaces. Europhys. Lett. 99, 37011 (2012).
Coey, J. M. D., Ariando & Pickett, W. E. Magnetism at the edge: New phenomena at oxide interfaces. MRS Bull. 38, 1040–1047 (2013).
Zhou, X. J. et al. One-dimensional electronic structure and suppression of d-wave node state in (La1.28Nd0.6Sr0.12)CuO4 . Science 286, 268–272 (1999).
Yoo, H. K. et al. Thickness-dependent electronic structure in ultrathin LaNiO3 films under tensile strain. Phys. Rev. B 93, 035141 (2016).
Zhou, K. J. et al. Localized and delocalized Ti 3d carriers in LaAlO3/SrTiO3 superlattices revealed by resonant inelastic x-ray scattering. Phys. Rev. B 83, 201402 (R) (2011).
Wang, Q. Y. et al. Interface-induced high-temperature superconductivity in single unit-cell FeSe films on SrTiO3 . Chin. Phys. Lett. 29, 037402 (2012).
Ge, G. F. et al. Superconductivity above 100 K in single-layer FeSe films on doped SrTiO3 . Nat. Mater. 14, 285–289 (2015).
Kormondy, K. J. et al. Quasi-two-dimensional electron gas at the epitaxial alumina/SrTiO3 interface: Control of oxygen vacancies. J. Appl. Phys. 117, 095303 (2015).
Chakhalian, J. et al. Orbital reconstruction and covalent bonding at an oxide interface. Science 318, 1114–1117 (2007).
Cao, Y. et al. Engineered Mott ground state in a LaTiO3+δ/LaNiO3 heterostructure. Nat. Commun. 7, 10418 (2016).
Zhou, R. S. & Snyder, R. L. Structures and transformation mechanisms of the η, γ and θ transition aluminas. Acta Crystallogr. B47, 617–630 (1991).
Jiang, K., Music, D., Sarakinos, K. & Schneider, J. M. Ab initio study of effects of substitutional additives on the phase stability of γ-alumina. J. Phys. Condens. 22, 505502 (2010).
Schütz, P. et al. Band bending and alignment at the spinel/perovskite γ-Al2O3/SrTiO3 heterointerface. Phys. Rev. B 91, 165118 (2015).
Ngo, T. Q. et al. Quasi-two-dimensional electron gas at the interface of γ-Al2O3/SrTiO3 heterostructures grown by atomic layer deposition. J. Appl. Phys. 118, 115303 (2015).
Lu, S. et al. Spectrum and phase mapping across the epitaxial γ-Al2O3/SrTiO3 interface. Appl. Phys. Lett. 108, 051606 (2016).
Ohtomo, A., Muller, D. A., Grazul, J. L. & Hwang, H. Y. Artificial charge-modulationin atomic-scale perovskite titanate superlattices. Nature 419, 378–380 (2002).
You, J. H. & Lee, J. H. Critical thickness for the two-dimensional electron gas in LaTiO3/SrTiO3 . Phys. Rev. B 88, 155111 (2013).
Khomskii, D. I. Transition Metal Compounds. Cambridge Univ. Press, (2014).
Acknowledgements
We acknowledge numerous insightful theory discussions with Daniel Khomskii. J.C. was supported by the Gordon and Betty Moore Foundation EPiQS Initiative through Grant No. GBMF4534. Y.C., S.M. and M.K. were supported by the DOD-ARO under Grant No. 0402-17291. X.L. was supported by the Department of Energy Grant No. DE-SC0012375. The Advanced Light Source is supported by the Director, Office of Science, Office of Basic Energy Sciences, of the U.S. Department of Energy under Contract No. DE-AC02-05CH11231. This research used resources of the Advanced Photon Source, a U.S. Department of Energy (DOE) Office of Science User Facility operated for the DOE Office of Science by Argonne National Laboratory under Contract No. DE-AC02-06CH11357.
Author information
Authors and Affiliations
Contributions
Y.C. and J.C. conceived and designed the experiments. Y.C., X.L., D.M., P.S. and E.A. acquired the XAS/XLD data. Y.C., X.L., S.M. and D.M. measured the electrical transport. Y.C., X.L., S.M., D.M., J.K. and P.R. measured the X-ray diffraction. M.K. and Y.C. prepared and characterized the samples. Y.C., P.S., E.A. and J.C. analyzed the data. All authors discussed the results.
Corresponding author
Ethics declarations
Competing interests
The authors declare no conflict of interest.
Additional information
Supplementary Information accompanies the paper on the npj Quantum Materials website (http://www.nature.com/npjquantmats)
Supplementary information
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Cao, Y., Liu, X., Shafer, P. et al. Anomalous orbital structure in a spinel–perovskite interface. npj Quant Mater 1, 16009 (2016). https://doi.org/10.1038/npjquantmats.2016.9
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1038/npjquantmats.2016.9
This article is cited by
-
Strain-tunable magnetism at oxide domain walls
Nature Physics (2019)