Abstract
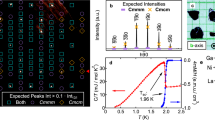
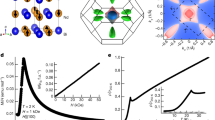
The topological properties of fermions arise from their low-energy Dirac-like band dispersion and associated chirality. Initially confined to points, extensions of the Dirac dispersion to lines, and even loops, have now been uncovered, and semimetals hosting such features have been identified. However, experimental evidence for the enhanced correlation effects predicted to occur in these topological semimetals has been lacking. Here, we report a quantum oscillation study of the nodal-loop semimetal ZrSiS in high magnetic fields that reveals significant enhancement in the effective mass of the quasiparticles residing near the nodal loop. Above a threshold field, magnetic breakdown occurs across gaps in the loop structure with orbits that enclose different windings around its vertices, each winding accompanied by an additional π Berry phase. The amplitudes of these breakdown orbits exhibit an anomalous temperature dependence. These findings demonstrate the emergence of novel, correlation-driven physics in ZrSiS associated with the Dirac-like quasiparticles.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Volovik, G. E. The Universe in a Helium Droplet (Oxford Univ. Press, 2007).
Kane, C. L. & Mele, E. J. Z2 topological order and the quantum spin Hall effect. Phys. Rev. Lett. 95, 146802 (2005).
Bernevig, B. A., Hughes, T. L. & Zhang, S.-C. Quantum spin Hall effect and topological phase transition in HgTe quantum wells. Science 314, 1757–1761 (2006).
Moore, J. E. & Balents, L. Topological invariants of time-reversal-invariant band structures. Phys. Rev. B 75, 121306 (2007).
Konig, M. et al. Quantum spin Hall insulator state in HgTe quantum wells. Science 318, 766–770 (2007).
Young, S. M. et al. Dirac semimetal in three dimensions. Phys. Rev. Lett. 108, 140405 (2012).
Young, S. M. & Kane, C. L. Dirac semimetals in two dimensions. Phys. Rev. Lett. 115, 126803 (2015).
Weng, H. et al. Topological node-line semimetal in three-dimensional graphene networks. Phys. Rev. B 92, 045108 (2015).
Wu, Y. et al. Dirac node arcs in PtSn4 . Nat. Phys. 12, 667–671 (2016).
Bian, G. et al. Topological nodal-line fermions in spin-orbit metal PbTaSe2 . Nat. Commun. 7, 10556 (2016).
Neupane, M. et al. Observation of topological nodal fermion semimetal phase in ZrSiS. Phys. Rev. B 93, 201104(R) (2016).
Schoop, L. M. et al. Dirac cone protected by non-symmorphic symmetry and three-dimensional Dirac line node in ZrSiS. Nat. Commun. 7, 11696 (2016).
Burkov, A. A. Topological semimetals. Nat. Mater. 15, 1145–1148 (2016).
Fang, C., Weng, H., Dai, X. & Fang, Z. Topological nodal line semimetals. Chin. Phys. B 25, 117106 (2016).
Huh, Y., Moon, E.-G. & Kim, Y.-B. Long-range Coulomb interaction in nodal-ring semimetals. Phys. Rev. B 93, 035138 (2016).
Roy, B. Interacting line-node semimetal and spontaneous symmetry breaking. Phys. Rev. B 96, 041113 (2017).
Liu, J. & Balents, L. Correlation and transport phenomena in topological nodal-loop semi-metals. Phys. Rev. B 95, 075426 (2017).
Wang, X. et al. Evidence of both surface and bulk Dirac bands and anisotropic non-saturating magnetoresistance in ZrSiS. Adv. Electron. Mater. 2, 1600228 (2016).
Ali, M. N. et al. Butterfly magnetoresistance, quasi-2D Dirac Fermi surfaces and a topological phase transition in ZrSiS. Sci. Adv. 2, e1601742 (2016).
Hu, J. et al. Evidence of topological nodal-line fermions in ZrSiSe and ZrSiTe. Phys. Rev. Lett. 117, 016602 (2016).
Singha, R., Pariari, A., Satpati, B. & Mandal, P. Large non-saturating magnetoresistance and signature of non-degenerate Dirac nodes in ZrSiS. Proc. Natl Acad. Sci. USA 114, 2468–2473 (2017).
Hu, J. et al. Nearly massless Dirac fermions and strong Zeeman splitting in the nodal-line semimetal ZrSiS probed by de Haas–van Alphen quantum oscillations. Phys. Rev. B 96, 045127 (2017).
Topp, A. et al. Non-symmorphic band degeneracy at the Fermi level in ZrSiTe. New J. Phys. 13, 125014 (2016).
Tan, B. S. et al. Unconventional Fermi surface in an insulating state. Science 349, 287–290 (2015).
Klein Haneveld, A. J. & Jellinek, F. Zirconium silicide and germanide chalcogenides preparation and crystal structures. Rec. Trav. Chim. Pays-Bas 83, 776–783 (1964).
Xu, Q. et al. Two-dimensional oxide topological insulator with iron-pnictide superconductor LiFeAs structure. Phys. Rev. B 92, 205310 (2015).
Lv, Y.-Y. et al. Extremely large and significantly anisotropic magnetoresistance in ZrSiS single crystals. Appl. Phys. Lett. 108, 244101 (2016).
Matusiak, M., Cooper, J. R. & Kaczorowski, D. Thermoelectric quantum oscillations in ZrSiS. Nat. Commun. 8, 15219 (2017).
Gvozdikov, V. M., Pershin, Y. V., Steep, E., Jansen, A. G. M. & Wyder, P. de Haas–van Alphen oscillations in the quasi-two-dimensional organic conductor κ-(ET)2Cu(NCS)2: the magnetic breakdown approach. Phys. Rev. B 65, 165102 (2002).
Elias, D. C. et al. Dirac cones reshaped by interaction effects in suspended graphene. Nat. Phys. 7, 701–704 (2011).
Yu, G. L. et al. Interaction phenomena in graphene seen through quantum capacitance. Proc. Natl Acad. Sci. USA 110, 3282–3286 (2013).
Gegenwart, P. et al. Magnetic-field induced quantum critical point in YbRh2Si2 . Phys. Rev. Lett. 89, 056402 (2002).
Paglione, J. et al. Field-induced quantum critical point in CeCoIn5 . Phys. Rev. Lett. 91, 246405 (2003).
Shoenberg, D. Magnetic Oscillations in Metals (Cambridge Univ. Press, 1984).
Schrieffer, J. R. Theory of Superconductivity (Perseus Books, 1983).
Kishigi, K., Nakano, M., Machida, K. & Hori, Y. dHvA effect with quantum interference oscillation due to magnetic breakdown. J. Phys. Soc. Jpn 64, 3043–3059 (1995).
Pal, H. K., Piechon, F., Fuchs, J.-N., Goerbig, M. & Montambaux, G. Chemical asymmetry and quantum oscillations in insulators. Phys. Rev. B 94, 125140 (2016).
Dzero, M., Sun, K., Galitski, V. & Coleman, P. Topological Kondo insulators. Phys. Rev. Lett. 104, 106408 (2010).
Knolle, J. & Cooper, N. R. Quantum oscillations without a Fermi surface and the anomalous de Haas–van Alphen effect. Phys. Rev. Lett. 115, 146401 (2015).
Knolle, J. & Cooper, N. R. Anomalous de Haas–van Alphen effect in InAs/GaSb quantum wells. Phys. Rev. Lett. 118, 176801 (2017).
Virosztek, A. & Ruvalds, J. Nested Fermi-liquid theory. Phys. Rev. B 42, 4064–4074 (1990).
Blaha, P., Schwarz, K., Madsen, G. K. H., Kvasnicka, D. & Luitz, J. WIEN2k, An Augmented Plane Wave + Local Orbitals Program for Calculating Crystal Properties (Karlheinz Schwarz, Techn. Universität Wien, 2001).
Perdew, J. P., Burke, S. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996).
Acknowledgements
We acknowledge enlightening discussions with Y.-B. Kim and A. McCollam. We also acknowledge the support of the HFML-RU/FOM, member of the European Magnetic Field Laboratory (EMFL). A portion of this work was supported by the Engineering and Physical Sciences Research Council (grant no. EP/K016709/1).
Author information
Authors and Affiliations
Contributions
S.W. initiated the project in collaboration with L.M.S. S.P., M.R.v.D. and S.W. performed the magnetotransport measurements. L.M.S. and B.V.L. synthesized the ZrSiS single crystals. A.C. performed the electronic band-structure calculations. S.P., M.R.v.D., S.W., A.C., M.I.K. and N.E.H. analysed the data. N.E.H. wrote the manuscript with input from all the co-authors.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 1081 kb)
Rights and permissions
About this article
Cite this article
Pezzini, S., van Delft, M., Schoop, L. et al. Unconventional mass enhancement around the Dirac nodal loop in ZrSiS. Nat. Phys. 14, 178–183 (2018). https://doi.org/10.1038/nphys4306
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys4306
This article is cited by
-
Comprehensive investigation of quantum oscillations in semimetal using an ac composite magnetoelectric technique with ultrahigh sensitivity
npj Quantum Materials (2024)
-
Magnetic-field-induced electronic instability of Weyl-like fermions in compressed black phosphorus
Science China Physics, Mechanics & Astronomy (2023)
-
Topological non-Hermitian skin effect
Frontiers of Physics (2023)
-
Quantum transport evidence of isolated topological nodal-line fermions
Nature Communications (2022)
-
Quasi-symmetry-protected topology in a semi-metal
Nature Physics (2022)