Abstract
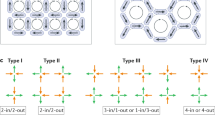
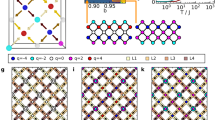
Recent advances in nanotechnology allow model systems to be constructed, in which frustrated interactions can be tuned at will, such as artificial spin ice. The symmetry of the square ice lattice leads to the emergence of a long-range-ordered ground state from the manifold of frustrated states. However, it is experimentally very difficult to access using the effective thermodynamics of rotating-field demagnetization protocols, because the energy barriers to thermal equilibrium are extremely large. Here we study an as-fabricated sample that approaches the ground state very closely. We identify the small localized departures from the ground state as elementary excitations of the system, at frequencies that follow a Boltzmann law. We therefore identify the state we observe as the frozen-in residue of true thermodynamics that occurred during the fabrication of the sample. The relative proportions of different excitations are suggestive of monopole interactions during thermalization.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Vedmedenko, E. Competing Interactions and Pattern Formation in Nanoworld (Wiley, 2007).
Pauling, L. The structure and entropy of ice and of other crystals with some randomness of atomic arrangement. J. Am. Chem. Soc. 57, 2680–2684 (1935).
Harris, M. J., Bramwell, S. T., McMorrow, D. F., Zeiske, T. & Godfrey, K. W. Geometrical frustration in the ferromagnetic pyrochlore Ho2Ti2O7 . Phys. Rev. Lett. 79, 2554–2557 (1997).
Bramwell, S. T. & Gingras, M. J. P. Spin ice state in frustrated magnetic pyrochlore materials. Science 294, 1495–1501 (2001).
Wang, R. F. et al. Artificial ‘spin ice’ in a geometrically frustrated lattice of nanoscale ferromagnetic islands. Nature 439, 303–306 (2006).
Remhof, A. et al. Magnetostatic interactions on a square lattice. Phys. Rev. B 77, 134409 (2008).
Tanaka, M., Saitoh, E., Miyajima, H., Yamaoka, T. & Iye, Y. Magnetic interaction in a ferromagnetic honeycomb nanoscale network. Phys. Rev. B 73, 052411 (2006).
Mengotti, E. et al. Building blocks of an artificial kagome spin ice: Photoemission electron microscopy of arrays of ferromagnetic islands. Phys. Rev. B 78, 144402 (2008).
Qi, Y., Brintlonger, T. & Cumings, J. Direct observation of the ice rule in an artificial kagome spin ice. Phys. Rev. B 77, 094418 (2008).
Ladak, S., Read, D. E., Perkins, G. K., Cohen, L. F. & Branford, W. R. Direct observation of magnetic monopole defects in an artificial spin-ice system. Nature Phys. 6, 359–363 (2010).
Schumann, A., Sothmann, B., Szary, P. & Zabel, H. Charge ordering of magnetic monopoles in triangular spin ice patterns. Appl. Phys. Lett. 97, 022509 (2010).
Mengotti, E. et al. Real-space observation of emergent magnetic monopoles and associated Dirac strings in artificial kagome spin ice. Nature Phys. advance online publication, 10.1038/nphys1794 (17 October 2010).
Nisoli, C. et al. Ground state lost but degeneracy found: The effective thermodynamics of ‘artificial spin ice’. Phys. Rev. Lett. 98, 217103 (2007).
Ke, X. et al. Energy minimization and ac demagnetization in a nanomagnet array. Phys. Rev. Lett. 101, 037205 (2008).
Castelnovo, C., Moessner, R. & Sondhi, S. L. Magnetic monopoles in spin ice. Nature 451, 42–45 (2008).
Jaubert, L. D. C. & Holdsworth, P. C. W. Signatures of magnetic monopole and Dirac string dynamics in spin ice. Nature Phys. 5, 258–261 (2009).
Fennell, T. et al. Magnetic Coulomb phase in the spin ice Ho2Ti2O7 . Science 326, 415–417 (2009).
Morris, D. J. P. et al. Dirac strings and magnetic monopoles in the spin ice Dy2Ti2O7 . Science 326, 411–414 (2009).
Kadowaki, H. et al. Observation of magnetic monopoles in spin ice. J. Phys. Soc. Jpn 78, 103706 (2009).
Bramwell, S. T. et al. Measurement of the charge and current of magnetic monopoles in spin ice. Nature 461, 956–959 (2009).
Möller, G. & Moessner, R. Magnetic multipole analysis of kagome and artificial ice dipolar arrays. Phys. Rev. B 80, 140409(R) (2009).
Mól, L. A. et al. Magnetic monopole and string excitations in two-dimensional spin ice. J. Appl. Phys. 106, 063913 (2009).
Mól, L. A., Moura-Melo, W. A. & Pereira, A. R. Conditions for free magnetic monopoles in synthetic square ice dipolar nanoarrays. Phys. Rev. B 82, 054434 (2010).
Jaeger, H. M., Nagel, S. R. & Behringer, R. P. Granular solids, liquids, and gases. Rev. Mod. Phys. 68, 1259–1273 (1996).
D’Anna, G., Mayor, P., Barrat, A., Loreto, V. & Nori, F. Observing Brownian motion in vibration-fluidized granular matter. Nature 424, 909–912 (2003).
Wang, R. F. et al. Demagnetization protocols for frustrated interacting nanomagnet arrays. J. Appl. Phys. 101, 09J104 (2007).
Nisoli, C. et al. Effective temperature in an interacting, externally driven, vertex system: Theory and experiment on artificial spin ice. Phys. Rev. Lett. 105, 047205 (2010).
Möller, G. & Moessner, R. Artificial square ice and related dipolar nanoarrays. Phys. Rev. Lett. 96, 237202 (2006).
Li, J. et al. Comparing artificial frustrated magnets by tuning the symmetry of nanoscale permalloy arrays. Phys. Rev. B 81, 092406 (2010).
Melko, R. G., den Hertog, B. C. & Gingras, M. P. Long-range order at low temperatures in dipolar spin ice. Phys. Rev. Lett. 87, 067203 (2001).
Joseph, R. I. & Schlömann, E. Demagnetizing field in nonellipsoidal bodies. J. Appl. Phys. 36, 1579–1593 (1965).
Libál, A., Reichhardt, C. & Olson Reichhardt, C. J. Realizing colloidal artificial ice on arrays of optical traps. Phys. Rev. Lett. 97, 228302 (2006).
Libál, A., Olson Reichhardt, C. J. & Reichhardt, C. Creating artificial ice states using vortices in nanostructured superconductors. Phys. Rev. Lett. 102, 237004 (2009).
Davidović, D. et al. Correlations and disorder in arrays of magnetically coupled superconducting rings. Phys. Rev. Lett. 76, 815–818 (1996).
Davidović, D. et al. Magnetic correlations, geometrical frustration, and tunable disorder in arrays of superconducting rings. Phys. Rev. B 55, 6518–6540 (1997).
Hilgenkamp, H. et al. Ordering and manipulation of the magnetic moments in large-scale superconducting π-loop arrays. Nature 422, 50–53 (2003).
Kirtley, J. R., Tsuei, C. C., Ariando, Smilde, H. J. H. & Hilgenkamp, H. Antiferromagnetic ordering in arrays of superconducting π-rings. Phys. Rev. B 72, 214521 (2005).
Han, Y. et al. Geometrical frustration in buckled colloidal monolayers. Nature 456, 898–903 (2008).
Shokef, Y. & Lubensky, T. C. Stripes, zigzags, and slow dynamics in buckled hard spheres. Phys. Rev. Lett. 102, 048303 (2009).
Mengotti, E. et al. Dipolar energy states in clusters of perpendicular magnetic nanoislands. J. Appl. Phys. 105, 113113 (2009).
Budrikis, Z., Politi, P. & Stamps, R. L. Vertex dynamics in finite two-dimensional square spin ices. Phys. Rev. Lett. 105, 017201 (2010).
Zhu, Y. (ed.) Modern Techniques for Characterizing Magnetic Materials (Springer, 2005).
Shpyrko, O. G. et al. Direct measurement of antiferromagnetic domain fluctuations. Nature 447, 68–71 (2007).
Pierce, M. S. et al. Quasistatic X-ray speckle metrology of microscopic magnetic return-point memory. Phys. Rev. Lett. 90, 175502 (2003).
Horcas, I. et al. WSxM: A software for scanning probe microscopy and a tool for nanotechnology. Rev. Sci. Inst. 78, 013705 (2007).
Acknowledgements
This work was supported financially by EPSRC and the STFC Centre for Materials Physics and Chemistry. The research was carried out in part at the Center for Functional Nanomaterials, Brookhaven National Laboratory, which is supported by the US Department of Energy, Office of Basic Energy Sciences, under Contract No. DE-AC02-98CH10886.
Author information
Authors and Affiliations
Contributions
J.P.M. and A.S. conducted sample fabrication. J.P.M conducted the MFM, data processing, calculations and analysis. C.H.M. and S.L. supervised the work, and contributed to discussion and direction. All authors discussed the results and commented on the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Information (PDF 2602 kb)
Rights and permissions
About this article
Cite this article
Morgan, J., Stein, A., Langridge, S. et al. Thermal ground-state ordering and elementary excitations in artificial magnetic square ice. Nature Phys 7, 75–79 (2011). https://doi.org/10.1038/nphys1853
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphys1853
This article is cited by
-
Defect-induced monopole injection and manipulation in artificial spin ice
Nature Communications (2022)
-
Reconfigurable training and reservoir computing in an artificial spin-vortex ice via spin-wave fingerprinting
Nature Nanotechnology (2022)
-
Controlled creation and annihilation of isolated robust emergent magnetic monopole like charged vertices in square artificial spin ice
Scientific Reports (2021)
-
Magnetic charge propagation upon a 3D artificial spin-ice
Nature Communications (2021)
-
Realization of macroscopic ratchet effect based on nonperiodic and uneven potentials
Scientific Reports (2021)