Abstract
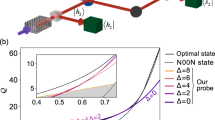
Quantum metrology utilizes entanglement to improve the sensitivity of measurements1,2,3. To date, the focus has been on the measurement of a single observable. Its orthogonal observable, however, may contain additional information, the knowledge of which can be used to further improve the measurement result beyond what is possible with state-of-the-art quantum metrology. Here we demonstrate a laser interferometer that provides information about two non-commuting observables, with uncertainties below the meter's quantum ground state. Our experiment is a proof of principle of what we call ‘quantum-dense metrology’, referring to its increased measurement information and its analogy to quantum-dense coding in quantum information science. We propose to use the additional information to discriminate between the actual science signal and parasitic signals originating from scattered photons. Our approach can be readily applied to improve squeezed-light enhanced gravitational-wave detectors at non-quantum noise-limited detection frequencies by providing a sub-shot-noise veto trigger against stray-light-induced signals.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 12 print issues and online access
$209.00 per year
only $17.42 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Giovannetti, V., Lloyd, S. & Maccone, L. Quantum-enhanced measurements: beating the standard quantum limit. Science 306, 1330–1336 (2004).
Giovannetti, V., Lloyd, S. & Maccone, L. Quantum metrology. Phys. Rev. Lett. 96, 010401 (2006).
Schnabel, R., Mavalvala, N., McClelland, D. E. & Lam, P. K. Quantum metrology for gravitational wave astronomy. Nat. Commun. 1, 121 (2010).
Kimble, H. J., Levin, Y., Matsko, A. B., Thorne, K. S. & Vyatchanin, S. P. Conversion of conventional gravitational-wave interferometers into quantum nondemolition interferometers by modifying their input and/or output optics. Phys. Rev. D 65, 22002 (2001).
Rarity, J. et al. Two-photon interference in a Mach–Zehnder interferometer. Phys. Rev. Lett. 65, 1348–1351 (1990).
Mitchell, M., Lundeen, J. & Steinberg, A. Super-resolving phase measurements with a multiphoton entangled state. Nature 429, 161–164 (2004).
Afek, I., Ambar, O. & Silberberg, Y. High-NOON states by mixing quantum and classical light. Science 328, 879–881 (2010).
Leibfried, D. et al. Toward Heisenberg-limited spectroscopy with multiparticle entangled states. Science 304, 1476–1478 (2004).
Gross, C., Zibold, T., Nicklas, E., Estève, J. & Oberthaler, M. K. Nonlinear atom interferometer surpasses classical precision limit. Nature 464, 1165–1169 (2010).
Lücke, B. et al. Twin matter waves for interferometry beyond the classical limit. Science 334, 773–776 (2011).
Cappellaro, P. et al. Entanglement assisted metrology. Phys. Rev. Lett. 94, 020502 (2005).
Wasilewski, W. et al. Quantum noise limited and entanglement-assisted magnetometry. Phys. Rev. Lett. 104, 133601 (2010).
Abadie, J. et al. A gravitational wave observatory operating beyond the quantum shot-noise limit. Nature Phys. 7, 962–965 (2011).
Einstein, A., Podolsky, B. & Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47, 777–780 (1935).
D'Ariano, G. M., Lo Presti, P. & Paris, M. G. A. Using entanglement improves the precision of quantum measurements. Phys. Rev. Lett. 87, 270404 (2001).
Tsang, M. & Caves, C. Evading quantum mechanics: engineering a classical subsystem within a quantum environment. Phys. Rev. X 2, 031016 (2012).
Ou, Z. Y., Pereira, S. F., Kimble, H. J. & Peng, K. C. Realization of the Einstein–Podolsky–Rosen paradox for continuous variables. Phys. Rev. Lett. 68, 3663–3666 (1992).
Furusawa, A. et al. Unconditional quantum teleportation. Science 282, 706–709 (1998).
Silberhorn, C. et al. Generation of continuous variable Einstein–Podolsky–Rosen entanglement via the Kerr nonlinearity in an optical fiber. Phys. Rev. Lett. 86, 4267–4270 (2001).
Bowen, W. P., Schnabel, R. & Lam, P. K. Experimental investigation of criteria for continuous variable entanglement. Phys. Rev. Lett. 90, 043601 (2003).
Bennett, C. H. & Wiesner, S. J. Communication via one-and two-particle operators on Einstein–Podolsky–Rosen states. Phys. Rev. Lett. 69, 2881–2884 (1992).
Braunstein, S. L. & Kimble, H. J. Dense coding for continuous variables. Phys. Rev. A 61, 042302 (2000).
Arthurs, E. & Kelly, J. L. B.S.T.J. Briefs: On the simultaneous measurement of a pair of conjugate observables. Bell Syst. Tech. J. 44, 725–729 (1965).
Leonhardt, U. Measuring the Quantum State of Light Ch. 6 (Cambridge Univ. Press, 1997).
Vahlbruch, H., Chelkowski, S., Danzmann, K. & Schnabel, R. Quantum engineering of squeezed states for quantum communication and metrology. New J. Phys. 9, 371 (2007).
Abramovici, A. et al. LIGO: the laser interferometer gravitational-wave observatory. Science 256, 325–333 (1992).
Vinet, J-Y., Brisson, V. & Braccini, S. Scattered light noise in gravitational wave interferometric detectors: coherent effects. Phys. Rev. D 54, 1276–1286 (1996).
Ottaway, D. J., Fritschel, P. & Waldman, S. J. Impact of upconverted scattered light on advanced interferometric gravitational wave detectors. Opt. Express 20, 8329–8336 (2012).
Sathyaprakash, B. & Schutz, B. Physics, astrophysics and cosmology with gravitational waves. Living Rev. Relativity 12, 2 (2009).
Steinlechner, S., Bauchrowitz, J., Eberle, T. & Schnabel, R. Strong Einstein–Podolsky–Rosen steering with unconditional entangled states. Phys. Rev. A 87, 022104 (2013).
Acknowledgements
The authors acknowledge discussions with T. Eberle, V. Händchen and H. Lück. This research was financed by the Deutsche Forschungsgemeinschaft (Sonderforschungsbereich Transregio 7, project C8), the European Union Seventh Framework Programme for Research (FP7), project ‘Quantum Interfaces, Sensors and Communication based on Entanglement’ (Q-ESSENCE), and supported by the Centre for Quantum Engineering and Space–Time Research (QUEST) and the International Max Planck Research School (IMPRS) on Gravitational Wave Astronomy.
Author information
Authors and Affiliations
Contributions
R.S. developed the initial idea for this work. H.M-E. and S.S. contributed to the theoretical background. S.S., J.B. and R.S. conceived the experiment. S.S., J.B. and M.M. conducted the experiment under supervision from K.D. and R.S.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary information
Supplementary information (PDF 225 kb)
Rights and permissions
About this article
Cite this article
Steinlechner, S., Bauchrowitz, J., Meinders, M. et al. Quantum-dense metrology. Nature Photon 7, 626–630 (2013). https://doi.org/10.1038/nphoton.2013.150
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nphoton.2013.150
This article is cited by
-
Speed limit of quantum metrology
Scientific Reports (2023)
-
Two-colour high-purity Einstein-Podolsky-Rosen photonic state
Nature Communications (2022)
-
Adaptive optical phase estimation for real-time sensing of fast-varying signals
Scientific Reports (2022)
-
High-performance cavity-enhanced quantum memory with warm atomic cell
Nature Communications (2022)
-
Entanglement of orbital angular momentum in non-sequential double ionization
Nature Communications (2022)