Abstract
Chiral magnetic domain walls are of great interest because lifting the energetic degeneracy of left- and right-handed spin textures in magnetic domain walls enables fast current-driven domain wall propagation. Although two types of magnetic domain walls are known to exist in magnetic thin films, Bloch- and Néel-walls, up to now the stabilization of homochirality was restricted to Néel-type domain walls. Since the driving mechanism of thin-film magnetic chirality, the interfacial Dzyaloshinskii–Moriya interaction, is thought to vanish in Bloch-type walls, homochiral Bloch walls have remained elusive. Here we use real-space imaging of the spin texture in iron/nickel bilayers on tungsten to show that chiral domain walls of mixed Bloch-type and Néel-type can indeed be stabilized by adding uniaxial strain in the presence of interfacial Dzyaloshinskii–Moriya interaction. Our findings introduce Bloch-type chirality as a new spin texture, which may open up new opportunities to design spin–orbitronics devices.
Similar content being viewed by others
Introduction
The formation of magnetic domains and domain walls (DWs) results from the interplay between the exchange interaction, the dipolar interaction and magnetic anisotropy. In perpendicularly magnetized thin-film systems, DWs can be classified as two canonical types: in Bloch walls, the spin rotates like a helical spiral around an axis which is parallel or antiparallel to the DW normal, whereas in Néel walls the spin rotates like a cycloidal spiral. A magnetic film is called chiral when the rotational sense of these spirals is the same in all DWs. In non-chiral films, both rotation senses exist in different sections of DWs, with the same probability overall. The conventional textbook view predicts non-chiral Bloch walls as the ground state in magnetic thin films1,2,3. In this picture chirality, that is, one preferred spin rotational sense in DWs, does not emerge because the magnetic energy contributions are all symmetric with respect to the rotation direction between to spins.
An asymmetric exchange interaction term, known as the Dzyaloshinskii–Moriya interaction (DMI), can be induced when inversion symmetry is broken in the system4,5. The inversion symmetry can break in the lattice (bulk DMI) or at surfaces and interfaces (interfacial DMI) of magnetic films6,7,8. The DMI term between two atomic spin Si and Sj on neighbouring atomic sites i and j can be written as EDM=−Dij·(Si × Sj), where Dij is the DMI vector. In the case of interfacial DMI, Dij is restricted to be perpendicular to the position vector rij=ri−rj (refs 6, 7, 8, 9). For this reason, interfacial DMI alone can only stabilize Néel-type chiral spin textures, such as cycloidal spin spirals10,11,12, skyrmions13,14 or chiral Néel walls15,16,17. In Bloch-type spin textures, the cross-product (Si × Sj) is parallel to the position vector rij and EDM vanishes, consequently Bloch walls are usually non-chiral.
It was recently found that chiral Néel walls in film and multilayer structures enable fast current-driven DW motion and spin texture-dependent DW propagation direction15,18,19,20,21. A numerical study also predicts that introducing chirality of Bloch walls into magnetic films can extend possibilities to manipulate DW propagation behaviours to new geometries18. Developing experimental evidence that allows us to tailor the spin structure of chiral DWs via interfacial DMI6,22 is crucial for engineering methods to control current- or field-driven DW dynamics in film and multilayer structures, since these phenomena hold great potential for information storage and spintronics. In particular, direct observations of DW spin structure under the combined effects of interfacial DMI and in-plane uniaxial anisotropy are still missing.
In the following, we demonstrate how the introduction of in-plane uniaxial anisotropy allows us to tailor the DMI-stabilized chiral DW spin textures. We focus on Fe/Ni bilayer grown on W(110) substrates, where the very large spin Hall angle of tungsten23 is combined with two-fold symmetry at the (110) interface and perpendicular magnetic anisotropy of the magnetic layer24,25,26. Using spin-polarized low-energy electron microscopy (SPLEEM)27,28,29, we observe anisotropic chiral DW spin structures with mixed components of chiral Bloch- and chiral Néel-character. We find that, as a function of the relative orientation of the DWs with respect to the [001] substrate surface direction, the Fe/Ni/W(110) system features chiral Néel walls, mixed chiral walls containing both Néel and Bloch components or non-chiral Bloch walls. The chirality of the Néel wall components is always left-handed, whereas the chirality of the Bloch wall components is either left- or right-handed. Bloch-component handedness as well as the ratio of the Bloch- versus Néel- components depends on the orientation relationship between the DW direction and the substrate lattice. Supported by Monte Carlo simulations, we propose that the origin of anisotropic chirality is the interplay between the DMI, the dipolar interaction and the in-plane uniaxial anisotropy. Our findings experimentally demonstrate the Bloch-type chirality as a new type of chiral spin texture stabilized by interfacial DMI, which may open up new opportunities to design spin–orbitronics devices.
Results
Visualizing chiral DWs
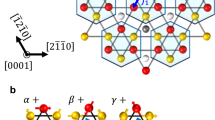
Within DWs in perpendicularly magnetized systems, spins usually rotate from one domain to another in one of the four basic spin textures, which are Bloch- or Néel walls with left- or right-handed chirality. Figure 1 shows sketches of a left-handed (a) and right-handed (b) chiral Néel wall and a left-handed (c) and right-handed (d) chiral Bloch wall. Experimentally, chirality in magnetic DWs can be determined by imaging three-dimensional spin textures. Figure 2 shows a SPLEEM measurement of the DW spin structure in a Fe/Ni bilayer grown on W(110) substrate. The structure of the thin film is sketched in Fig. 2a, and a typical compound SPLEEM image (see Methods) is shown in Fig. 2b where blue/yellow indicates magnetization components along [1–10]/[−110] and cyan/red indicates components along [001]/[00-1]. In this rendering scheme, all DWs are highlighted in colour and, in Fig. 2b, we notice that all DWs are either cyan or red, which means that the in-plane components of all DWs are either parallel or antiparallel to W[001], even though the directions of DWs are oriented along all possible directions within the film plane. These findings are reproduced in all images of other areas of this and a number of additional samples (see Supplementary Fig. 1). This indicates that the inner spin structure of DWs in the Fe/Ni/W(110) system is coupled to the direction of the DW with respect to the W[001] lattice direction.
Sketch of left-handed (a) and right-handed (b) chiral Néel walls in perpendicularly magnetized thin film, directions of arrows correspond to the magnetization direction. The spin structure of the DW is simplified by highlighting the in-plane component in the centre of the walls. Sketch of left-handed (c) and right-handed (d) chiral Bloch walls in a perpendicularly magnetized thin film.
(a) Profile sketch of Fe/Ni bilayer grown on W(110) substrate. (b) Compound SPLEEM image, colourized DW highlights the in-plane orientation of the magnetization inside the DW; scale bar, 1 μm. (c) Definition of angles α and φ, m indicates the in-plane component of the DW, blue arrow indicates normal vector n, grey arrow indicates W[001] direction. (d) Examples of different DW orientations, data are cropped from b. DW tangent directions are indicated by white dashed lines, scale bars are 100 nm. (e–h) φ-dependent histograms of angle α in DW centre, counted pixel-by-pixel in four SPLEEM compound images including b. The histograms are normalized with respect to the total number of evaluated states. Left h., left-handed; ML, monolayer; right h., right-handed.
To understand the micromagnetic properties of these DWs, we analyse the spin texture of the DWs in more detail. We start by identifying the image pixels on the centerlines of all DWs in a representative set of SPLEEM images (see Methods). At all centreline-pixels of all the DWs, we measure the local direction of the magnetization, expressed as the magnetization unit vector m, and the direction of the in-plane normal vector n of the DW. We represent these data in terms of two angles: to capture the DW orientation, we define the angle φ as the angle of the normal vector n of the DW with respect to the W[001] lattice direction, and to capture the local DW spin texture, we define the angle α as the angle between the magnetization unit vector m and the in-plane normal vector n of the DW; the geometry of these angles is sketched in Fig. 2c. The magnitude of the angle α indicates whether the wall is in Bloch configuration (α=±90°) or in Néel-configuration (α=0° or α=180°)17. Our measurements show that not only do both of these DW types occur, but there are also parts of the DWs which exhibit mixed types (for example, α=±45°). We notice that the magnitude of the angle α, and thus the type of the wall, is a function of the orientation of the wall φ. Figure 2d reproduces SPLEEM images cropped from Fig. 2b to show DW sections, highlighted by dashed lines, where certain DW orientations are prevalent (red and blue arrows in the SPLEEM images indicate directions of DW magnetization unit vector m and DW normal vector n within these DW sections). Next to these images, Fig. 2e–h shows histograms of the angle α at DW sections with corresponding orientations. Key points for these data demonstrated are as follows: when DW tangential direction is parallel to [1–10], that is, φ=0°±3°, then the histogram plotted in Fig. 2e shows that the angle α is scattered about a narrow distribution centred near 0°, confirming that these DWs are Néel type with left-handed chirality17. When φ=90°±3° (DW tangential direction is parallel to [001], Fig. 2f), then the distribution of α has two peaks with comparable heights centred at −90° and +90°, indicating right- and left-handed Bloch wall sections. This distribution corresponds to the conventional case of non-chiral Bloch walls17. The distribution for φ=−45°±3° is shown in Fig. 2g, where a peak showing a narrow distribution of the angle α appears at ~+45°. Although this is clearly a chiral spin structure, it neither corresponds to chiral Néel wall15,16,17 nor to non-chiral Bloch wall1,2,3,16,17. This DW spin texture can be understood as a superposition of the left-handed chiral Néel structure and left-handed chiral Bloch structure, that is, as in Néel walls the spin vector tilts towards the in-plane normal direction of the DW while, at the same time, it rotates around the DW normal as it does in Bloch walls. We will refer to this texture as a mixed DW. Similarly, when φ=45°±3°, as shown in Fig. 2h, then the single peak at −45° indicates a mixed chiral wall type composed of a left-handed Néel component and a right-handed Bloch-component.
Quantitative picture of anisotropic chirality
To analyse the orientation-dependent DW structure systematically, we test how Bloch-type chirality evolves from left-handed to non-chiral to right-handed chiral order as seen, for example, in Fig. 2f–h. A two-dimensional histogram reproduced in Fig. 3a shows the statistical likeliness of DW spin configurations α and DW orientations φ; on our colour scale, rare α/φ combinations are darker and common α/φ combinations are brighter. To guide interpretation, Fig. 3b shows the sketches of DW configurations corresponding to the α−φ histogram plotted in Fig. 3a. As defined in the inset in Fig. 2, red arrows in Fig. 3b indicate the in-plane direction of magnetization inside the DW and blue arrows correspond to the DW normal n. Vertical cuts along green dashed lines through the two-dimensional histogram in Fig. 3a correspond to the histograms of α as shown in Fig. 2e–h. The bright regions in Fig. 3a (emerald-coloured regions in Fig. 3b) represent the most common DW spin textures found experimentally. The most prominent feature in this histogram is a diagonal streak in the lower half. This streak forms for two reasons. First, in this region of the histogram, the orientations of in-plane components of DW magnetization (red arrows) are aligned with in-plane uniaxial magnetic anisotropy Ku, that is, parallel to the W[001] direction. Second, the Néel components of spin textures in the lower half of the histogram (−90°<α<90°) are always left handed. By contrast, a similar diagonal steak is suppressed in the upper half of the histogram (90°<α<270°) because, although a corresponding region exists where DW magnetization is aligned with Ku, the Néel components of spin textures in this part of the histogram are right-handed and thus energetically unfavourable as a result of the DMI of this system. Besides the prominent diagonal steak, six additional DW textures are observed where both α and φ are right angles (four bright regions in the four corners of the histogram and two bright regions where α=90° and φ=±90°). These α/φ combinations correspond to pure Bloch textures. The fact that for φ=−90° and +90° we simultaneously observe textures with α=90° (left-handed) and with α=−90° or α=270° (right-handed) indicates that the system is achiral in the limit of pure Bloch texture.
(a) Two-dimensional histogram of experimental DW magnetization data in α−φ space derived from four SPLEEM compound images including Fig. 2b. Colour bar corresponds to the number of pixels on DW centrelines with a given φ and α. Green dashed lines correspond to the histograms in Fig. 2e–h. (b) Sketch of DW configurations with respect to DW orientation, in α−φ space: each circle contains a black and a white domain, blue arrows show DW normal vectors n, red arrows show orientation of in-plane magnetization of the DWs, grey arrows show direction of Ku. Emerald-coloured regions highlight the preferred configurations realized in the Fe/Ni/W(110) system (that is, left-handed DMI and Ku along [001]). Left h., left-handed; right h., right-handed.
To clarify how the system evolves from homochirality for most α/φ combinations to non-chiral Bloch textures at φ=−90° and +90° we quantify the magnetic chirality of mixed DWs by decomposing the magnetization into Bloch- and Néel- components. By plotting the projections of the magnetization unit vector onto the DW tangent- and normal directions the two-dimensional histogram shown in Fig. 3a can be converted into separate histograms of the Bloch and Néel components: for all points on DW centrelines, the projection of the local magnetization unit vector onto the DW tangent, given by sin(α), contributes to the Bloch-type histogram and the projection onto the DW normal direction, given by cos(α), contributes to the Néel-type histogram. The resulting histograms are shown in Fig. 4a,b. From these data, we can quantify average chiralities γB and γN of the Bloch-type components and Néel-type components by averaging the magnetization projections over all angles α.
The sign of the average chiralities γB and γN reflects rotational sense and, following the conventions used by Heide et al.30, the Bloch-type component is called left-handed (right-handed) for positive (negative) γB, and the Néel-type component is left-handed (right-handed) for positive (negative) γN. Figure 4c plots the dependence of γB and γN on φ, the angle between the DW normal and Ku. The average Néel chirality is always positive and follows a cosine curve (light blue dashed line), indicating that Néel components of DW spin textures in this system are always left-handed, regardless of the orientation of local DW sections. The average Bloch chirality of DW spin textures follows a sin curve (dark blue dashed line) in the middle region of the plot, where the magnitude of φ is less than ~60°. This indicates that Bloch wall spin texture can be either left-handed or right-handed, depending on the orientation of the DW with respect to the substrate induced anisotropy Ku. The sinusoidal φ-dependence suggests that left- and right-handed Bloch components occur with equal likeliness in this system, similar to the case of non-chiral magnets where the DMI can be neglected. Yet this system is clearly different from non-chiral magnets, in which case one would expect the quantities plotted in Fig. 4c to scatter about the flat line where average chirality vanishes, γ=0 (purple dashed line). By contrast, the sinusoidal dependence of average chirality on φ is a result of the anisotropic DMI in the Fe/Ni/W(110) system. Here Bloch chirality gradually vanishes when φ approaches ±90° (ref. 16). The gradual deviation from sinusoidal behaviour is interesting because, given that the DMI stabilizes the Bloch-type chirality, measuring how the system evolves from mixed chiral textures at small φ to non-chiral Bloch DWs at φ=±90° offers a way to estimate the strength of the DMI (see Supplementary Note 1).
A model for the origin of anisotropic chirality
Prior work on a similar system, Fe/8 monolayer Ni/W(110), established that in-plane anisotropic strain due to lattice mismatch31 gives rise to an in-plane uniaxial magnetic anisotropy Ku with easy axis along W[001], through magneto-elastic contributions to the magnetic anisotropy25. We also confirmed the presence of uniaxial anisotropy Ku by SPLEEM observations on thicker in-plane magnetized Fe/Ni films, where the orientation of magnetization of the in-plane domains is always parallel or antiparallel to the W[001] direction. Thus, in these systems, spin structure inside the DWs is a result of interplay between the exchange interaction, the interfacial DMI, the dipolar interaction, the perpendicular magnetic anisotropy, as well as Ku. The interfacial DMI favours chiral Néel DWs, and the dipolar interaction favours non-chiral Bloch DWs. Because of its two-fold symmetry, uniaxial anisotropy itself does not influence the chirality of the DWs. However, Ku provides an additional force favouring alignment of the magnetization within DWs towards the easy magnetization axis [001]. This additional force lifts the left-/right-handed degeneracy of Bloch-type spin structures whenever DWs are at a non-zero angle with respect to the [001] direction.
Simulation for the anisotropic DW
To test this model and clarify the role of Ku in a DMI system, we performed Monte Carlo simulations32,33. A two-dimensional Heisenberg model was constructed to simulate DW configurations in the presence of the interfacial DMI and Ku (see Supplementary Note 1). Figure 5a shows typical simulated DWs. The coloured bars represent straight DW segments, each in a different orientation φ, that is, we sweep the angle φ by changing the easy axis of Ku with respect to the DW orientation. As φ varies from 0° to 90°, simulated DWs evolve from chiral Néel-type wall to non-chiral Bloch-type DWs. Quantitative values for the average chiralities γB and γN of the Néel- and Bloch- components can be derived from simulation results. Results are shown in Fig. 5b, where the φ dependence of γB and γN nicely reproduces the experimental results (see also Supplementary Fig. 2b). The simulated results suggest that this type of Bloch-component chirality may be a general feature of DMI systems with uniaxial in-plane anisotropy, beyond Fe/Ni/W(110).
(a) Monte Carlo simulation results with variable DW orientation φ. White and black regions correspond to perpendicularly magnetized domains, coloured region in between corresponds to DW, and orientation of in-plane component of the DW is highlighted by colour wheel. (b) φ-dependent chiralities γN and γB derived from the simulation, −1 corresponds to right-handed and +1 corresponds to left-handed.
Towards a homochiral Bloch-type component
In the measurements reported above, both left- and right-handed DW segments coexist within each sample, and the handedness of the chiral Bloch component in DWs in this system is solely determined by the DW orientation φ. This suggests the possibility that the system might be driven into a homochiral state if one can experimentally confine the directions of the DW orientation. One possibility to tune DW orientations might be to fabricate nanowires on W(110). For energetic reasons, DWs in nanowires tend to be oriented orthogonal to the wire direction. By controlling nanowire orientation with respect to the lattice orientation of the tungsten crystal, it may be possible to select preferred DW orientations and thus preferred handedness (and weight) of the Bloch component. Another way to control DW orientations is to exploit the interaction of atomic surface steps with DWs. Results on related thin-film systems have shown how substrate step arrays can be used to orient DWs34,35. We have confirmed the viability of this approach. Preparing thinner Fe/Ni bilayers, we find that most of the DWs are aligned along surface step directions, as shown in Fig. 6. Evaluating all DWs in this image shows that the histogram of the angle α has only one peak at −40°, indicating that the Bloch-type chirality in these DWs is right-handed homochiral. Considering that direction and density of atomic surface steps can be controlled by polishing substrates at controlled vicinal angles near the (110) lattice plane, this result suggests that it is indeed possible to prepare Fe/Ni/W(110) structures featuring homochiral DW with tailored Bloch-component spin textures.
(a) Sketch of Fe/Ni bilayer grown on W(110) substrate. (b) Compound SPLEEM image highlighting the direction of DW in-plane magnetization. The colour wheel indicates the direction of in-plane magnetization on the DWs. White arrows additionally show the in-plane spin orientations in the DWs; scale bar, 1 μm. (c) Histogram of angle α in DW counted pixel-by-pixel in (b) shows a single peak pointing at ~−40°. Left h., left-handed; right h., right-handed.
Our experimental results on iron/nickel bilayers epitaxially grown on (110) tungsten surfaces demonstrate that anisotropic chiral magnetism can be stabilized by combining the interfacial DMI with in-plane uniaxial anisotropy. Under these conditions, a family of DW spin textures is stabilized: chiral Néel-type DWs, chiral mixed-type DWs composed of Néel- and Bloch components and non-chiral Bloch-type DWs. The handedness of the Bloch-type component is a function of DW orientation with respect to the substrate lattice, which provides unique opportunities to systematically study in detail the transition between chiral DWs and non-chiral DWs to further understand the interplay between the DMI, magnetic anisotropy and the dipolar interaction.
These experimental observations demonstrate how the Bloch-type chirality emerges as a new type of DW spin texture when the interfacial DMI is combined with magnetic anisotropy, thus adding a new degree of freedom to tailor a diverse family of chiral spin textures. The results introduce rich possibilities to influence DW dynamics by combining intrinsic phenomena such as spin Hall effects18,19,20,21,36,37, Rashba effects18,36,38,39 or the DMI15,18, not only with magnetoelastically induced anisotropy (as we have shown in this work), but also with piezo-induced strain40,41, nanowire shape-induced anisotropy42 or external magnetic fields19,20,21,43,44, which may open up new opportunities to design spin–orbitronics devices.
Methods
Sample preparation
The W(110) substrate was cleaned by flashing at 1,950 °C in 3 × 10−8 Torr O2 and final annealing at 1,900 °C under ultrahigh vacuum with base pressure 4 × 10−11 Torr. Fe and Ni film thickness was calibrated by monitoring low-energy electron microscopy (LEEM) image intensity oscillations associated with atomic layer-by-layer growth. Fe and Ni layers were grown at 300 K by electron beam evaporation, and the sample was annealed to 900 K for several minutes after growth of one monolayer Ni to develop a well-ordered interface24,25.
Image vector field analysis
Two LEEM images, I+p(i, k) and I−p(i, k), are acquired with spin of the illuminating electron beam aligned and antialigned with a chosen polarization axis p. We typically use image integration times of 1 s. The LEEM images are used to calculate a SPLEEM asymmetry Ap(i, k)=(I+p(i, k)−I−p(i, k))/(I+p(i, k)+I−p(i, k)). The asymmetry value Ap(i, k) at pixel coordinates (i, k) within a SPLEEM image is proportional to the projection of the magnetization vector m(i, k) of the sample on the chosen polarization axis p, AP(i, k)~(m(i, k)·p). We choose three orthonormal vectors x, y and z to form a cartesian coordinate system with z being normal to the sample surface. From sets of three SPLEEM images shown in Supplementary Fig. 3a–c, each with p set to be parallel to one of the three components of the sample surface coordinates, the direction of magnetization unit vector m can be determined at all pixel coordinates. To obtain low-noise three-dimensional vector fields A(i, k) used for this study, we aligned and averaged 40 such images for the component in the out-of-plane direction (z) and 100 each for the in-plane directions (x, y). To represent these vector images in colour, we mapped the in-plane angle on hue and the out-of-plane component to the brightness of the image (Supplementary Fig. 3d). For higher contrast within DWs, the contrast range of the out-of-plane domains was reduced to 50%. The centrelines of the DWs were determined by thresholding at Az(i, k)=0 and subtracting the thresholded image from its binary dilation. The DW normal vectors were determined by applying Gaussian blur with a width of 2px and then evaluating the two-dimensional gradient on the centreline. To determine the direction of m(i, k), we evaluated and normalized m(i, k)=(Ax(i, k),Ay(i, k),0)/(Ax(i, k)2+Ay(i, k)2)1/2 so that the blur of the strong signal of the z component overlapping with the DW does not add noise to the data. Determining m(i, k) from single-pixel centrelines and from three-, five- and seven-pixel-wide ribbons straddling the DW centrelines, we had found that the histograms, characterizing DW magnetization direction as a function of the angles α and φ come out nearly identical; therefore, we use DW centre line pixels in the analysis.
Additional information
How to cite this article: Chen, G. et al. Unlocking Bloch-type chirality in ultrathin magnets through uniaxial strain. Nat. Commun. 6:6598 doi: 10.1038/ncomms7598 (2015).
References
Hubert, A. & Schäfer, R. Magnetic Domains: The Analysis of Magnetic Microstructures Springer (1998) .
Chikazumi, S. Physics of Ferromagnetism Oxford Univ. Press (1999) .
Yafet, Y. & Gyorgy, E. M. Ferromagnetic stripe domains in an atomic monolayer. Phys. Rev. B 38, 9145–9151 (1988) .
Dzyaloshinskii, I. E. A thermodynamic theory of “weak” ferromagnetism of antiferromagnetics. J. Phys. Chem. Solids 4, 241–255 (1958) .
Moriya, T. Anisotropic superexchange interaction and weak ferromagnetism. Phys. Rev. 120, 91–98 (1960) .
Fert, A. Magnetic and transport properties of metallic multilayers. Mat. Sci. Forum 59–60, 439–480 (1990) .
Crépieux, A. & Lacroix, C. Dzyaloshinskii–Moriya interactions induced by symmetry breaking at a surface. J. Magn. Magn. Mater. 182, 341–349 (1998) .
Fert, A., Cros, V. & Sampaio, J. Skyrmions on the track. Nat. Nanotechnol. 8, 152–156 (2013) .
Vedmedenko, E. Y., Udvardi, L., Weinberger, P. & Wiesendanger, R. Chiral magnetic ordering in two-dimensional ferromagnets with competing Dzyaloshinsky-Moriya interactions. Phys. Rev. B 75, 104431 (2007) .
Bode, M. et al. Chiral magnetic order at surfaces driven by inversion asymmetry. Nature 447, 190–193 (2007) .
Ferriani, P. et al. Atomic-scale spin spiral with a unique rotational sense: Mn monolayer on W(001). Phys. Rev. Lett. 101, 027201 (2008) .
Meckler, S. et al. Real-space observation of a right-rotating inhomogeneous cycloidal spin spiral by spin-polarized scanning tunneling microscopy in a triple axes vector magnet. Phys. Rev. Lett. 103, 157201 (2009) .
Heinze, S. et al. Spontaneous atomic-scale magnetic skyrmion lattice in two dimensions. Nat. Phys. 7, 713–718 (2011) .
Romming, N. et al. Writing and deleting single magnetic skyrmions. Science 341, 636–639 (2013) .
Thiaville, A. et al. Dynamics of Dzyaloshinskii domain walls in ultrathin magnetic films. Europhys. Lett. 100, 57002 (2012) .
Chen, G. et al. Novel chiral magnetic domain wall structure in Fe/Ni/Cu(001) films. Phys. Rev. Lett. 110, 177204 (2013) .
Chen, G. et al. Tailoring the chirality of magnetic domain walls by interface engineering. Nat. Commun. 4, 2671 (2013) .
Khvalkovskiy, A. V. et al. Matching domain-wall configuration and spin-orbit torques for efficient domain-wall motion. Phys. Rev. B 87, 020402(R) (2013) .
Ryu, K.-S., Thomas, L., Yang, S.-H. & Parkin, S. S. P. Chiral spin torque at magnetic domain walls. Nat. Nanotechnol. 8, 527–533 (2013) .
Emori, S., Bauer, U., Ahn, S.-M., Martinez, E. & Beach, G. S. D. Current-driven dynamics of chiral ferromagnetic domain walls. Nat. Mater. 12, 611–616 (2013) .
Torrejon, J. et al. Interface control of the magnetic chirality in CoFeB|MgO heterosctructures with heavy metal underlayers. Nat. Commun. 5, 4655 (2014) .
Fert, A. & Levy, P. M. Role of anisotropic exchange interactions in determining the properties of spin-glasses. Phys. Rev. Lett. 44, 1538–1541 (1980) .
Pai, C.-F. et al. Spin transfer torque devices utilizing the giant spin Hall effect of tungsten. Appl. Phys. Lett. 101, 122404 (2012) .
Sander, D. et al. Structure and perpendicular magnetization of Fe/Ni(111) bilayers on W(110). J. Appl. Phys. 81, 4702–4704 (1997) .
Meyerheim, H. L. et al. Spin reorientation and structural relaxation of atomic layers: pushing the limits of accuracy. Phys. Rev. Lett. 93, 156105 (2004) .
Lee, J.-S. et al. Interfacial nanostructure induced spin-reorientation transition in Ni/Fe/Ni/W(110). Phys. Rev. B 83, 144420 (2011) .
Rougemaille, N. & Schmid, A. K. Magnetic imaging with spin-polarized low-energy electron microscopy. Eur. Phys. J. Appl. Phys. 50, 20101 (2010) .
Ding, H. F., Schmid, A. K., Li, D., Guslienko, K. Y. & Bader, S. D. Magnetic bistability of Co nanodots. Phys. Rev. Lett. 94, 157202 (2005) .
El Gabaly, F. et al. Imaging spin-reorientation transitions in consecutive atomic Co layers on Ru(0001). Phys. Rev. Lett. 96, 147202 (2006) .
Heide, M., Bihlmayer, G. & Blügel, S. Dzyaloshinskii-Moriya interaction accounting for the orientation of magnetic domain in ultrathin films: Fe/W(110). Phys. Rev. B 78, 140403(R) (2008) .
Sander, D., Schmidthals, C., Enders, A. & Kirschner, J. Stress and structure of Ni monolayers on W(110): The importance of lattice mismatch. Phys. Rev. B 57, 1406–1409 (1998) .
Kwon, H. Y. & Won, C. Effects of Dzyaloshinskii–Moriya interaction on magnetic stripe domains. J. Magn. Magn. Mater. 351, 8–15 (2014) .
Kwon, H. Y. et al. A Study of the stripe domain phase at the spin reorientation transition of two-dimensional magnetic system. J. Magn. Magn. Mater. 322, 2742–2748 (2010) .
Ramchal, R., Schmid, A. K., Farle, M. & Poppa, H. Spiral-like continuous spin-reorientation transition of Fe/Ni bilayers on Cu(100). Phys. Rev. B 69, 214401 (2004) .
Klein, C., Ramchal, R., Farle, M. & Schmid, A. K. Direct imaging of spin-reorientation transitions in ultrathin Ni films by spin-polarized low-energy electron microscopy. Surf. Interface Anal. 38, 1550–1553 (2006) .
Kim, J. et al. Layer thickness dependence of the current-induced effective field vector in Ta|CoFeB|MgO. Nat. Mater 12, 240–245 (2013) .
Haazen, P. P. J. et al. Domain wall depinning governed by the spin Hall effect. Nature Mater 12, 299–303 (2013) .
Miron, I. M. et al. Current-driven spin torque induced by the Rashba effect in a ferromagnetic metal layer. Nat. Mater. 9, 230–234 (2010) .
Miron, I. M. et al. Fast current-induced domain-wall motion controlled by the Rashba effect. Nat. Mater. 10, 419–423 (2011) .
Lei, N. et al. Strain-controlled magnetic domain wall propagation in hybrid piezoelectric/ferromagnetic structures. Nat. Commun. 4, 1378 (2013) .
De Ranieri, E. et al. Piezoelectric control of the mobility of a domain wall driven by adiabatic and non-adiabatic torques. Nat. Mater. 12, 808–814 (2013) .
Koyama, T. et al. Observation of the intrinsic pinning of a magnetic domain wall in a ferromagnetic nanowire. Nat. Mater. 10, 194–197 (2011) .
Rushforth, A. W. Domain wall motion driven by spin Hall effect—Tuning with in-plane magnetic anisotropy. Appl. Phys. Lett. 104, 162408 (2014) .
Franken, J. H., Herps, M., Swagten, H. J. M. & Koopmans, B. Tunable chiral spin texture in magnetic domain-walls. Sci. Rep. 4, 5248 (2014) .
Acknowledgements
We acknowledge Dr Colin Ophus for helpful discussions. Experiments were performed at the Molecular Foundry, Lawrence Berkeley National Laboratory, supported by the Office of Science, Office of Basic Energy Sciences, Scientific User Facilities Division, of the U.S. Department of Energy under Contract No. DE-AC02—05CH11231. This work was also supported by the National Research Foundation of Korea Grant funded by the Korean Government (2012R1A1A2007524), by the National Key Basic Research Program (No. 2015CB921401 and No. 2011CB921801) and the National Science Foundation (No. 11434003 and No. 11474066) of China, by National Science Foundation DMR-1210167 and NRF through Global Research Laboratory project of Korea.
Author information
Authors and Affiliations
Contributions
G.C. was responsible for the concept of the experiment and carried out the measurements. A.K.S. supervised the SPLEEM facility. A.T.N. developed and implemented algorithms for the quantitative analysis of SPLEEM data. G.C., A.T.N., A.K.S., Y.W. and Z.Q.Q. analysed and interpreted the results. S.P.K. performed the Monte Carlo simulations. C.W. supervised the Monte Carlo simulations. H.Y.K. contributed to the development of the simulation code. G.C. and A.K.S. prepared the manuscript. All authors commented on the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-3, Supplementary Note 1 and Supplementary References (PDF 627 kb)
Rights and permissions
About this article
Cite this article
Chen, G., N’Diaye, A., Kang, S. et al. Unlocking Bloch-type chirality in ultrathin magnets through uniaxial strain. Nat Commun 6, 6598 (2015). https://doi.org/10.1038/ncomms7598
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms7598
This article is cited by
-
Super-resolution of magnetic systems using deep learning
Scientific Reports (2023)
-
The emergence of three-dimensional chiral domain walls in polar vortices
Nature Communications (2023)
-
Dense skyrmion crystal stabilized through interfacial exchange coupling: Role of in-plane anisotropy
Frontiers of Physics (2021)
-
The spin structures of interlayer coupled magnetic films with opposite chirality
Scientific Reports (2018)
-
Significant Dzyaloshinskii–Moriya interaction at graphene–ferromagnet interfaces due to the Rashba effect
Nature Materials (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.