Abstract
In underdoped cuprate superconductors, the Fermi surface undergoes a reconstruction that produces a small electron pocket, but whether there is another, as yet, undetected portion to the Fermi surface is unknown. Establishing the complete topology of the Fermi surface is key to identifying the mechanism responsible for its reconstruction. Here we report evidence for a second Fermi pocket in underdoped YBa2Cu3Oy, detected as a small quantum oscillation frequency in the thermoelectric response and in the c-axis resistance. The field-angle dependence of the frequency shows that it is a distinct Fermi surface, and the normal-state thermopower requires it to be a hole pocket. A Fermi surface consisting of one electron pocket and two hole pockets with the measured areas and masses is consistent with a Fermi-surface reconstruction by the charge–density–wave order observed in YBa2Cu3Oy, provided other parts of the reconstructed Fermi surface are removed by a separate mechanism, possibly the pseudogap.
Similar content being viewed by others
Introduction
The phase diagram of cuprate superconductors is shaped by ordered states, and their identification is essential for understanding high-temperature superconductivity. Evidence for a new state with broken symmetry in cuprates recently came from two major developments. The observation of quantum oscillations in underdoped YBa2Cu3Oy (YBCO)1 and HgBa2CuO4+d (Hg1201) (ref. 2), combined with negative Hall3,4,5 and Seebeck5,6,7 coefficients, showed that the Fermi surface contains a small closed electron pocket and is therefore reconstructed at low temperature, implying that translational symmetry is broken. The detailed similarity of the Fermi-surface reconstruction in YBCO and La1.6−xEu0.4SrxCuO4 (Eu-LSCO)6,7,8 revealed that YBCO must host a density–wave order similar to the stripe order of Eu-LSCO9. More recently, charge–density–wave (CDW) modulations were observed directly, first by NMR in YBCO10 and then by X-ray diffraction in YBCO11,12,13 and Hg1201 (ref. 14). In YBCO, a thermodynamic signature of the CDW order was detected in the sound velocity at low temperature and finite magnetic field15. These CDW modulations are reminiscent of the checkerboard pattern previously observed by STM on Bi2Sr2CaCu2O8+d (refs 16, 17), for instance.
Fermi-surface reconstruction and CDW modulations are therefore two universal signatures of underdoped cuprates, which begs the following question: is the Fermi surface seen by quantum oscillations compatible with a reconstruction by the observed CDW modulations? This issue requires a detailed knowledge of the Fermi surface, to be compared with Fermi surface calculations based on the measured parameters of the CDW order, in the same material at the same doping. In this Article, we report quantum oscillations measurements that reveal an additional, hole-like Fermi pocket in underdoped YBCO. As we discuss below, a Fermi surface consisting of one electron and two hole pockets of the measured sizes and masses is consistent with a reconstruction by the observed CDW.
Results
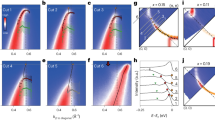
We have measured quantum oscillations in the thermoelectric response and c-axis resistance of underdoped YBCO. Our samples were chosen to have a doping p=0.11–0.12, at which the amplitude of quantum oscillations is maximal18. In the doping-temperature phase diagram, this is also where the CDW modulations are strongest19,20 (Fig. 1a) and where the critical magnetic field Bc2 needed to suppress superconductivity is at a local minimum21 (Fig. 1b). In the T=0 limit, the Seebeck (S) and Nernst (ν=N/B) coefficients are inversely proportional to the Fermi energy22,23 and are therefore expected to be enhanced for small Fermi surfaces. In Fig. 2a, we show isotherms of S and N at T=2 K measured up to B=45 T in a YBCO sample with p=0.11. Above Bc2=24 T (ref. 21), both S and N are negative; the fact that S<0 is consistent with an electron pocket dominating the transport at low temperature6,7. The normal-state signal displays exceptionally large quantum oscillations, with a main frequency Fa=540 T and a beat pattern indicative of other, nearby, frequencies. In Fig. 2, we also show the c-axis resistance of two YBCO samples at p=0.11 and 0.12, measured in pulsed fields up to 68 T. The overall behaviour of the c-axis magnetoresistance at p=0.11 is consistent with previous reports24,25. Quantum oscillations are clearly visible and the three distinct frequencies Fa1=540 T, Fa2=450 T and Fa3=630 T in the Fourier spectrum at p=0.11 (Fig. 1d) agree with reported values26.
(a) Temperature-doping phase diagram of YBCO, showing the superconducting transition temperature Tc (grey dome, data from ref. 46), the CDW onset temperature TCDW (green diamonds, from ref. 19; blue diamonds, from ref. 20). (b) Upper critical field Hc2 of YBCO as a function of doping (red dots, from ref. 21). In both a,b, the arrows indicate the two dopings of the samples used in our study. All error bars in a,b are reproduced from the original references. (c) Sketch of the reconstructed Fermi surface adapted from ref. 42 using the CDW wavevectors (arrows) measured in YBCO19,20, showing a diamond-shaped nodal electron pocket (red) and two hole-like ellipses (blue and green). The dashed line is the antiferromagnetic Brillouin zone; the dotted line is the original large Fermi surface; the black dots mark the so-called ‘hot spots’, where those two lines intersect. (d) Fourier transform of our c-axis resistance data (at p=0.11), showing the new ‘low’ frequency Fb=95±10 T reported here, and the three main ‘high’ frequencies Fa1 (blue), Fa2 (red) and Fa3 (green).
(a) Seebeck (S; red) and Nernst (N; blue) signals in YBCO p=0.11 as a function of magnetic field B at T=2 K. (b) Seebeck coefficient S, plotted as S/T versus B, at temperatures as indicated. (c,d) c axis electrical resistance Rc of YBCO samples with p=0.11 and p=0.12, as a function of B up to 68 T, at different temperatures as indicated. For all data, the field B is along the c axis.
With increasing temperature, the amplitude of these ‘fast’ oscillations decreases rapidly and above T~10 K we are left with a slowly undulating normal-state signal, clearly seen in the raw Seebeck data (Figs 2b and 3a). In Fig. 3b, the oscillatory part of that signal, obtained by subtracting a smooth background, is plotted as a function of inverse magnetic field. Although the ‘slow oscillations’ at 18 K are 20 times weaker than the fast oscillations at 2 K, they are clearly resolved and periodic in 1/B. After their discovery in the Seebeck signal, the slow oscillations were also detected in the c-axis resistance, as shown in Fig. 3c. In both the Seebeck and c-axis resistance data, the frequency of these slow oscillations is Fb=95±10 T (p=0.11). Similar oscillations were also detected in the c-axis resistance of a sample at p=0.12 (Fig. 3d), with Fb=120±15 T. In Fig. 4a, we show the derivative dRc/dB, which unambiguously reveals Fb, without the need for a background subtraction. (Note that in the c-axis resistance data, the amplitude of Fb is about 0.1% of the total signal and is more sensitive to the background subtraction.) This slow frequency persists up to 30 K and its amplitude follows the usual Lifshitz–Kosevich formula (Fig. 4b), with a small effective mass m*=0.45±0.1 m0, where m0 is the free electron mass.
(a) Seebeck coefficient in YBCO p=0.11 (blue), plotted as S/T, as a function of field B at T=18 K, showing slow oscillations about a linear background (red). (b) Oscillatory part of the Seebeck coefficient ΔS/T (obtained by subtracting a 2nd order polynomial from the raw data) as a function of 1/B, showing the usual fast quantum oscillations at T=2 K (red), and the new slow oscillations with Fb=95±10 T at T=18 K (blue, multiplied by 20). For clarity, the slow frequency Fb was removed from the data at T=2 K. (c,d) Oscillatory part of the c-axis electrical resistance ΔRc in YBCO (obtained by subtracting a third-order polynomial from the raw data) at p=0.11 and p=0.12 as a function of 1/B, at temperatures as indicated. The oscillations are periodic in 1/B, with a frequency Fb=95±10 T and 120±15 T at p=0.11 and 0.12, respectively.
(a) Derivative of the c-axis resistance of sample p=0.11 with respect to field B, plotted versus 1/B at temperatures as indicated. This confirms the presence of the slow frequency Fb, irrespective of background subtraction. (b) Amplitude of Fb oscillations as a function of temperature (dots). The line is a Lifshitz–Kosevich fit to the data, giving an effective mass m*=0.45±0.1 m0. (c) Oscillatory part of the c axis resistance at different angles θ between the field and the c axis, as indicated, as a function of 1/Bcos(θ) at T=15 K. A second-order polynomial background was subtracted from the raw data to extract ΔRc. (d) Slow frequency Fb as a function of θ (dots). The red line is the function 1/cos(θ). The error bars are a convolution of s.d. in the value of Fb, for different fitting ranges and different orders of the polynomial background.
Using the c-axis resistance, we have measured the dependence of Fb on the angle θ at which the field is tilted away from the c axis. In Fig. 4c, the oscillatory part of the c axis resistance for p=0.11 at T=15 K is plotted versus 1/Bcos(θ), and the angular dependence of Fb is displayed in Fig. 4d. Fb(θ) varies approximately as 1/cos(θ), indicating that the Fermi surface associated with Fb is a warped cylinder along the c axis, as expected for a quasi-two-dimensional system.
Discussion
The slow frequency Fb~100 T reported here bears the key signatures of quantum oscillations and in the following discussion we argue that it comes from a small hole-like Fermi surface, distinct from the larger electron-like Fermi pocket responsible for the main frequency Fa1=540 T.
We note that the frequency Fb is nearly equal to the difference between the main frequency of the electron pocket Fa1 and its satellites Fa2 and Fa3. While the identification of the multiple Fa frequencies is not definitive, it is likely that two of them are associated with the two separate Fermi surfaces that come from the two CuO2 planes (bilayer) in the unit cell of YBCO. The third frequency could then either come from magnetic breakdown between these two Fermi surfaces27 or from a warping due to c-axis dispersion26,28. In layered quasi-two-dimensional materials, slow quantum oscillations can appear in the c-axis transport as a result of interlayer coupling29,30. Two observations allow us to rule out this scenario in the present context. First, Fb is observed in the in-plane Seebeck coefficient, which does not depend on the c-axis conductivity. Second, at a special field-angle θ, called the Yamaji angle, where the c-axis velocity vanishes on average along a cyclotron orbit, one should see a vanishing Fb. This is not seen in our field-angle dependence of Fb, which, if anything, only deviates upward from a cylindrical 1/Bcos(θ) dependence (Fig. 4d).
Quantum interference from magnetic breakdown between two bilayer-split orbits could in principle produce a difference frequency close to Fb. In this scenario, however, the amplitudes of the two nearby frequencies Fa2 and Fa3 should be identical, irrespective of the field range, in disagreement with torque26 and c-axis resistance measurements (see Fig. 1d). Furthermore, in a magnetic breakdown scenario, we would expect Fb to scale with Fa, since both frequencies originate from the same cyclotron orbits. This is not what we observe in our thermoelectric data: as seen in Fig. 2a, the amplitude of Fa is larger in the Nernst effect than in the Seebeck effect, yet Fb is only detected in the latter. This is strong evidence that Fa and Fb do not involve cyclotron orbits on the same Fermi surface. We therefore conclude that Fb must come from a distinct Fermi pocket, in contrast with the interpretation of ref. 31 in terms of quantum interference.
For a number of reasons, we infer that this second pocket in the reconstructed Fermi surface of YBCO is hole-like. The first reason is the strong dependence of resistivity ρ and Hall coefficient RH on magnetic field B, as observed in YBCO and in YBa2Cu4O8 (ref. 3), a closely related material with similar quantum oscillations32,33. For instance, RH(B) goes from positive at low field to negative at high field3 and ρ(B) exhibits a significant magnetoresistance25. These are natural consequences of having both electron and hole carriers. In YBa2Cu4O8, the Hall and resistivity data were successfully fit in detail to a two-band model of electrons and holes34.
A second indication that both the electron and hole carriers are present in underdoped YBCO is the fact that quantum oscillations are observed in the Hall coefficient35,36. In an isotropic single-band model, the Hall coefficient is simply given by RH=1/ne, where n is the carrier density and e the electron charge. Quantum oscillations in RH appear via the scattering rate, which enters RH either when two or more bands of different mobility are present or when the scattering rate on a single band is strongly anisotropic. At low temperatures, however, where impurity scattering dominates, the latter scenario is improbable.
The most compelling evidence for the presence of hole-like carriers in underdoped YBCO comes from the magnitude of the Seebeck coefficient. In the T=0 limit and for a single band, it is given by22:

where kB is Boltzmann’s constant, TF is the Fermi temperature and ζ=0 or −1/2 depending on whether the relaxation time or the mean free path is assumed to be energy independent, respectively. The sign of S/T depends on whether the carriers are holes (+) or electrons (−). This expression has been found to work very well in a variety of correlated electron metals22. We stress that S/T (in the T=0 limit) is governed solely by TF, which allows a direct quantitative comparison with quantum oscillation data, with no assumption on pocket multiplicity. This contrasts with the specific heat, which depends on the number of Fermi pockets (see below).
In Fig. 5a, we reproduce normal-state Seebeck data in YBCO at four dopings, plotted as S/T versus T (from ref. 7). S/T goes from positive at high T to negative at low T, in agreement with a similar sign change in RH(T) (refs 3, 4), both evidence that the dominant carriers at low T are electron like. Extrapolating S/T to T=0 as shown by the dashed lines in Fig. 5a, we obtain the residual values and plot them as a function of doping in Fig. 5b (Smeasured, red squares). We see that the size of the residual term is largest (that is, is most strongly negative) at p=0.11, and that it decreases on both sides.
(a) Normal-state Seebeck coefficient S/T as a function of temperature for YBCO at four dopings, as indicated (adapted from ref. 7). The squares and dots are data in zero field and in 28 T, respectively. The dotted lines are extrapolations of S/T to T→0, whose values are plotted in b,c (red squares). The lines are a guide to the eye. (b) Extrapolated value of S/T at T=0 as a function of doping (Smeasured, red squares; from data and extrapolations in a). The error bars represent the uncertainty in extrapolating to T=0. The blue dots (Se) indicate S/T (T→0) for the electron pocket alone, calculated from the main quantum oscillation frequency Fa1 and mass m* (from refs 24, 37, 38) (using equations (1) and (2)). The blue line is a guide to the eye. (c) The red squares (Smeasured) are identical to those in b. Using a two-band model (equation (3)), we include the contribution of the hole pocket (Sh) using the measured Fermi temperature associated with the slow frequency Fb (TF=280±80 K; see text). With the conductivity ratio σe/σh as the only fit parameter, this model (black line) reproduces the measured data (red squares). (d) Ratio of electron-to-hole conductivities, σe/σh (black circles), from a fit (black line, c) to the measured values of S/T at T=0 (red squares, b,c). The black line is a guide to the eye. The error bars are derived from the errors on Smeasured.
This doping-dependent S/T is to be compared with the Fermi temperature directly measured by quantum oscillations via:

where F is the frequency, m* the effective mass and assuming a parabolic dispersion. For YBCO at p=0.11, the electron pocket gives Fa1=540±20 T and m*=1.76m0, so that TF=410±20 K and hence Se/T=–1.0 μV K−2 (–0.7 μV K−2), for ζ=0 (–1/2). In Fig. 5a, the measured S/T extrapolated to T→0 gives a value of −0.9 μV K−2. The electron pocket alone therefore accounts by itself for essentially the entire measured Seebeck signal at p=0.11. From quantum oscillation measurements at different dopings24,37,38, we know the values of Fa1 and m* from p=0.09 to p=0.13, and can therefore determine the evolution of Se/T in that doping interval. The result is plotted as blue dots in Fig. 5b, where we see that the calculated |Se/T| increases by a factor 2.5 between p=0.11 and p=0.09. This is because the mass m* increases strongly as p→0.08 (ref. 38), while Fa1 decreases only slightly18. This strong increase in the calculated |Se/T| is in stark contrast with the measured value of |S/T|, which decreases by a factor of 3 between p=0.11 and p=0.09 (Fig. 5b). To account for the observed doping dependence of the thermopower in YBCO, we are led to conclude that there must be a hole-like contribution to S/T. We emphasize that the Hall coefficient RH (ref. 4) measured well above Bc2 (ref. 21) displays the same dome-like dependence on doping as the Seebeck coefficient7, which further confirms the presence of a hole-like Fermi pocket.
In a two-band model, the total Seebeck coefficient is given by

where the hole (h) and electron (e) contributions are weighted by their respective conductivities σh and σe. As shown in Fig. 5c, we can account for the measured S/T at T→0 by adding a hole-like contribution, Sh, which we estimate from Fb=95±10 T and m*=0.45m0, giving TF=280±80 K. Assuming for simplicity that Sh is doping independent leaves the ratio of conductivities, σe/σh, as the only adjustable parameter in the above two-band expression (equation (3)). In Fig. 5d, we plot the resulting σe/σh as a function of doping, and we see that it peaks at p=0.11 and drops on either side. This is consistent with the fact that the amplitude of the fast quantum oscillations is largest at p=0.11, and much smaller away from that doping18, direct evidence that the mobility of the electron pocket is maximal at p=0.11. This is clearly seen in the resistance data in Fig. 2c,d, where the amplitude of the quantum oscillations of the electron pocket is strongly reduced when going from p=0.11 to 0.12. In contrast, Fig. 3c,d shows that the amplitude of the oscillations from the hole pocket remains nearly constant: at T=4.2 K and H=68 T, their relative amplitude is ΔRc/Rc=0.036% at p=0.11 and 0.03% at p=0.12. The change in conductivity ratio therefore comes mostly from a change in σe.
To summarize, in addition to the two-band description of transport data in YBa2Cu4O8 (ref. 34), the doping dependence of the Seebeck7 and Hall4 coefficients in YBCO is firm evidence that the reconstructed Fermi surface of underdoped YBCO (for 0.08<p<0.18) contains not only the well-established electron pocket4, but also another hole-like surface (of lower mobility). We combine this evidence with our discovery of an additional small Fermi surface to conclude that this new pocket is hole like.
From the measured effective mass m*, the residual linear term γ in the electronic specific heat Ce(T) at T→0 can be estimated through the relation39

where ni is the multiplicity of the ith type of pocket in the first Brillouin zone (this expression assumes an isotropic Fermi liquid in two dimensions with a parabolic dispersion). For a Fermi surface containing one electron pocket and two hole pockets per CuO2 plane, we obtain a total mass of (1.7±0.2)+2 (0.45±0.1)=2.6±0.4 m0, giving γ=7.6±0.8 mJ K−2 mol (for two CuO2 planes per unit cell). High-field measurements of Ce at T→0 in YBCO at p~0.1 yield γ=5±1 mJ K−2 mol (ref. 39) at B>Bc2=30 T (ref. 21). We therefore find that the Fermi surface of YBCO can contain at most two of the small hole pockets reported here, in addition to only one electron pocket. No further sheet can realistically be present in the Fermi surface.
There is compelling evidence that the Fermi surface of YBCO is reconstructed by the CDW order detected by NMR and X-ray diffraction. In particular, Fermi-surface reconstruction4,7 and CDW modulations19,20 are detected in precisely the same region of the temperature-doping phase diagram. Because the CDW modulations are along both the a and b axes, the reconstruction naturally produces a small closed electron pocket along the Brillouin zone diagonal, at the so-called nodal position40,41. Given the wavevectors measured by X-ray diffraction, there will also be small closed hole-like ellipses located between the diamond-shaped nodal electron pockets. An example of the Fermi surface calculated42 using the measured CDW wavevectors is sketched in Fig. 1c. It contains two distinct closed pockets: a nodal electron pocket of area such that Fe~430 T and a hole-like ellipse such that Fh~90 T p=0.11 (ref. 42). Note that a reconstruction by a commensurate wavevector q=1/3 π/a, very close to the measured value, yields one electron and two hole pockets per Brillouin zone, as assumed in our calculation of γ above.
If, as indeed observed in YBCO at p=0.11 (ref. 20), the CDW modulations are anisotropic in the a–b plane, the ellipse pointing along the a axis will be different from that pointing along the b axis42. If one of the ellipses is close enough to the electron pocket, that is, if the gap between the two is small enough, magnetic breakdown will occur between the hole and the electron pockets, and this could explain the complex spectra of multiple quantum oscillations seen in underdoped YBCO (Fig. 1d).
In most models of Fermi-surface reconstruction by CDW order, the size of the Fermi pockets can be made to agree with experiments using a reasonable set of parameters. For example, a similar Fermi surface (with one electron pocket and two hole pockets) is also obtained if one considers a ‘criss-crossed’ stripe pattern instead of a checkerboard43. At this level, there is consistency between our quantum oscillation measurements and models of Fermi-surface reconstruction by the CDW order. However, in addition to the electron and hole pockets, the folding of the large Fermi surface produces other segments of Fermi surface whose total contribution to γ greatly exceeds that allowed by the specific heat data. Consequently, there must exist a mechanism that removes parts of the Fermi surface beyond the reconstruction by the CDW order. A possible mechanism is the pseudogap. The loss of the antinodal states caused by the pseudogap would certainly remove parts of the reconstructed Fermi surface.
Further theoretical investigations are needed to understand how pseudogap and CDW order are intertwined in underdoped cuprates. An important point in this respect is the fact that, unlike in Bi2Sr2−xLaxCuO6+d (ref. 44), the CDW wavevector measured by X-ray diffraction in YBCO and in Hg1201 (ref. 14) does not connect the hot spots where the large Fermi surface intersects the antiferromagnetic Brillouin zone (Fig. 1c), nor does it nest the flat antinodal parts of that large Fermi surface (Fig. 1c).
Methods
Samples
Single crystals of YBCO with y=6.54, 6.62 and 6.67 were obtained by flux growth at UBC45. The superconducting transition temperature Tc was determined as the temperature below which the zero-field resistance R=0. The hole doping p is obtained from Tc (ref. 46), giving p=0.11 for y=6.54 and 6.62, and p=0.12 for y=6.67. The samples with y=6.54 and 6.62 have a high degree of ortho-II oxygen order, and the sample with y=6.67 has ortho-VIII order. The samples are detwinned rectangular platelets, with the a axis parallel to the length (longest dimension) and the b axis parallel to the width. The electrical contacts are diffused evaporated gold pads with a contact resistance less than 1 Ω.
Thermoelectric measurements
The thermoelectric response of YBCO with y=6.54 (p=0.11) was measured at the National High Magnetic Field Laboratory (NHMFL) in Tallahassee, Florida, up to 45 T, in the temperature range from 2 to 40 K. The Seebeck and Nernst coefficients are given by S≡−∇Vx/∇Tx and ν≡N/B≡(∇Vy/∇Tx)/B, respectively, where ∇Vx (∇Vy) is the longitudinal (transverse) voltage gradient caused by a temperature gradient ∇Tx, in a magnetic field B||z. A constant heat current was sent along the a axis of the single crystal, generating a temperature difference ΔTx across the sample. ΔTx was measured with two uncalibrated Cernox chip thermometers (Lakeshore), referenced to a third, calibrated Cernox. The longitudinal and transverse electric fields were measured using nanovolt preamplifiers and nanovoltmeters. All measurements were performed with the temperature of the experiment stabilized within ±10 mK and the magnetic field B swept at a constant rate of 0.4–0.9 T min−1 between positive and negative maximal values, with the heat on. The field was applied normal to the CuO2 planes (B||z||c).
Since the Seebeck coefficient S is symmetric with respect to the magnetic field, it is obtained by taking the mean value between positive and negative fields:

where ΔVx is the difference in the voltage along x measured with and without thermal gradient. This procedure removes any transverse contribution that could appear due to slightly misaligned contacts. The longitudinal voltages and the thermal gradient being measured on the same pair of contacts, no geometric factor is involved.
The Nernst coefficient N is antisymmetric with respect to the magnetic field; therefore, it is obtained by the difference:

where L and w are the length and width of the sample, respectively, along x and y and Vy is the voltage along y measured with the heat current on. This antisymmetrization procedure removes any longitudinal thermoelectric contribution and a constant background from the measurement circuit. The uncertainty on N comes from the uncertainty in determining L and w, giving typically an error bar of ±10%.
Resistance measurements
The c-axis resistance was measured at the Laboratoire National des Champs Magnétiques Intenses (LNCMI) in Toulouse, France, in pulsed magnetic fields up to 68 T. Measurements were performed in a conventional four-point configuration, with a current excitation of 5 mA at a frequency of ~60 kHz. Electrical contacts to the sample were made with large current pads and small voltage pads mounted across the top and bottom so as to short out any in-plane current. A high-speed acquisition system was used to digitize the reference signal (current) and the voltage drop across the sample at a frequency of 500 kHz. The data were analysed with software that performs the phase comparison. θ is the angle between the magnetic field and the c axis, and measurements were done at θ=0° up to 68 T and at various angles θ up to 58 T. The uncertainty on the absolute value of the angle is about 1°.
Additional information
How to cite this article: Doiron-Leyraud, N. et al. Evidence for a small hole pocket in the Fermi surface of underdoped YBa2Cu3Oy. Nat. Commun. 6:6034 doi: 10.1038/ncomms7034 (2015).
References
Doiron-Leyraud, N. et al. Quantum oscillations and the Fermi surface in an underdoped high-Tc superconductor. Nature 447, 565–568 (2007).
Barišić, N. et al. Universal quantum oscillations in the underdoped cuprate superconductors. Nat. Phys. 9, 761–764 (2013).
LeBoeuf, D. et al. Electron pockets in the Fermi surface of hole-doped high-Tc superconductors. Nature 450, 533–536 (2007).
LeBoeuf, D. et al. Lifshitz critical point in the cuprate superconductor YBa2Cu3Oy from high-field Hall effect measurements. Phys. Rev. B 83, 054506 (2011).
Doiron-Leyraud, N. et al. Hall, Seebeck, and Nernst coefficients of underdoped HgBa2CuO4+δ: Fermi-surface reconstruction in an archetypal cuprate superconductor. Phys. Rev. X 3, 021019 (2013).
Chang, J. et al. Nernst and Seebeck coefficients of the cuprate superconductor YBa2Cu3O6.67: a study of Fermi surface reconstruction. Phys. Rev. Lett. 104, 057005 (2010).
Laliberté, F. et al. Fermi-surface reconstruction by stripe order in cuprate superconductors. Nat. Commun. 2, 432 (2011).
Taillefer, L. et al. Fermi-surface reconstruction in high-Tc superconductors. J. Phys.: Condens. Matter 21, 164212 (2009).
Fink, J. et al. Phase diagram of charge order in La1.8-xEu0.2SrxCuO4 from resonant soft x-ray diffraction. Phys. Rev. B 83, 092503 (2011).
Wu, T. et al. Magnetic-field-induced charge-stripe order in the high-temperature superconductor YBa2Cu3Oy . Nature 477, 191–194 (2011).
Ghiringhelli, G. et al. Long-range incommensurate charge fluctuations in (Y,Nd)Ba2Cu3O6+x . Science 337, 821–825 (2012).
Chang, J. et al. Direct observation of competition between superconductivity and charge density wave order in YBa2Cu3O6.67 . Nat. Phys. 8, 871–876 (2012).
Achkar, A. J. et al. Distinct charge orders in the planes and chains of ortho-III-ordered YBa2Cu3O6+δ superconductors identified by resonant elastic x-ray scattering. Phys. Rev. Lett. 109, 167001 (2012).
Tabis, W. et al. Charge order and its connection with Fermi-liquid charge transport in a pristine high-Tc cuprate. Nat. Commun. 5, 5875 (2014).
LeBoeuf, D. et al. Thermodynamic phase diagram of static charge order in underdoped YBa2Cu3Oy . Nat. Phys. 9, 79–83 (2013).
Hoffman, J. E. et al. A four unit cell periodic pattern of quasi-particle states surrounding vortex cores in Bi2Sr2CaCu2O8+δ . Science 295, 466–469 (2002).
Kohsaka, Y. et al. An intrinsic bond-centered electronic glass with unidirectional domains in underdoped cuprates. Science 315, 1380–1385 (2007).
Vignolle, B., Vignolles, D., Julien, M.-H. & Proust, C. From quantum oscillations to charge order in high-Tc copper oxides in high magnetic fields. C. R. Phys. 14, 39–52 (2013).
Hücker, M. et al. Competing charge, spin, and superconducting orders in underdoped YBa2Cu3Oy . Phys. Rev. B 90, 054514 (2014).
Blanco-Canosa, S. et al. Resonant x-ray scattering study of charge-density wave correlations in YBa2Cu3O6+x . Phys. Rev. B 90, 054513 (2014).
Grissonnanche, G. et al. Direct measurement of the upper critical field in cuprate superconductors. Nat. Commun. 5, 3280 (2014).
Behnia, K., Jaccard, D. & Flouquet, J. On the thermoelectricity of correlated electrons in the zero-temperature limit. J. Phys.: Condens. Matter 16, 5187 (2004).
Behnia, K. The Nernst effect and the boundaries of the Fermi liquid picture. J. Phys.: Condens. Matter 21, 113101 (2009).
Ramshaw, B. J. et al. Angle dependence of quantum oscillations in YBa2Cu3O6.59 shows free-spin behaviour of quasiparticles. Nat. Phys. 7, 234–238 (2010).
Vignolle, B. et al. Coherent c-axis transport in the underdoped cuprate superconductor YBa2Cu3Oy . Phys. Rev. B 85, 224524 (2012).
Audouard, A. et al. Multiple quantum oscillations in the de Haas–van Alphen spectra of the underdoped high-temperature superconductor YBa2Cu3O6.5 . Phys. Rev. Lett. 103, 157003 (2009).
Sebastian, S. E. et al. Quantum oscillations from nodal bilayer magnetic breakdown in the underdoped high temperature superconductor YBa2Cu3O6+x . Phys. Rev. Lett. 108, 196403 (2012).
Sebastian, S. E. et al. Fermi-liquid behavior in an underdoped high-Tc superconductor. Phys. Rev. B 81, 140505 (2010).
Grigoriev, P. et al. Anomalous beating phase of the oscillating interlayer magnetoresistance in layered metals. Phys. Rev. B 65, 060403(R) (2002).
Kartsovnik, M. et al. Slow oscillations of magnetoresistance in quasi-two-dimensional metals. Phys. Rev. Lett. 89, 126802 (2002).
Sebastian, S. E. et al. Normal-state nodal electronic structure in underdoped high-Tc copper oxides. Nature 511, 61–64 (2014).
Yelland, E. A. et al. Quantum oscillations in the underdoped cuprate YBa2Cu4O8 . Phys. Rev. Lett. 100, 047003 (2008).
Bangura, A. F. et al. Small Fermi surface pockets in underdoped high temperature superconductors: observation of Shubnikov–de Haas oscillations in YBa2Cu4O8 . Phys. Rev. Lett. 100, 047004 (2008).
Rourke, P. M. C. et al. Fermi-surface reconstruction and two-carrier model for the Hall effect in YBa2Cu4O8 . Phys. Rev. B 82, 020514 (2010).
Chakravarty, S. & Kee, H.-Y. Fermi pockets and quantum oscillations of the Hall coefficient in high-temperature superconductors. Proc. Natl Acad. Sci. USA 105, 8835–8839 (2008).
Kikugawa, N., Rost, A.W., Hicks, C. W., Schofield, A. J. & Mackenzie, A. P. Ca3Ru2O7: density wave formation and quantum oscillations in the Hall resistivity. J. Phys. Soc. Jpn 79, 024704 (2010).
Jaudet, C. et al. de Haas–van Alphen oscillations in the underdoped high-temperature superconductor YBa2Cu3O6.5 . Phys. Rev. Lett. 100, 187005 (2008).
Sebastian, S. E. et al. Metal-insulator quantum critical point beneath the high-Tc superconducting dome. Proc. Natl Acad. Sci. USA 107, 6175–6179 (2010).
Riggs, S. C. et al. Heat capacity through the magnetic-field-induced resistive transition in an underdoped high-temperature superconductor. Nat. Phys. 7, 332–335 (2011).
Harrison, N. & Sebastian, S. E. Protected nodal electron pocket from multiple-Q ordering in underdoped high temperature superconductors. Phys. Rev. Lett. 106, 226402 (2011).
Sebastian, S. E., Harrison, N. & Lonzarich, G. G. Towards resolution of the Fermi surface in underdoped high-Tc superconductors. Rep. Prog. Phys. 75, 102501 (2012).
Allais, A., Chowdhury, D. & Sachdev, S. Connecting high-field quantum oscillations to the pseudogap in the underdoped cuprates.Nat. Commun. 5, 5771 (2014).
Maharaj, A. V., Hosur, P. & Raghu, S. Crisscrossed stripe order from interlayer tunneling in hole-doped cuprates. Phys. Rev. B 90, 125108 (2014).
Comin, R. et al. Charge order driven by Fermi-arc instability in Bi2Sr2−xLaxCuO6+δ . Science 343, 390–392 (2014).
Liang, R., Bonn, D. A. & Hardy, W. N. Growth of YBCO single crystals by the self-flux technique. Phil. Mag. 92, 2563 (2012).
Liang, R., Bonn, D. A. & Hardy, W. N. Evaluation of CuO2 plane hole doping in YBa2Cu3O6+x single crystals. Phys. Rev. B 73, 180505 (2006).
Acknowledgements
We thank A. Allais, K. Behnia, A. Carrington, S. Chakravarty, B. Fauqué, A. Georges, N. E. Hussey, M.-H. Julien, S.A. Kivelson, M.R. Norman, S. Raghu, S. Sachdev, A.-M. Tremblay and C. Varma for fruitful discussions. We thank J. Béard and P. Frings for their assistance with the experiments at the LNCMI. The work in Toulouse was supported by the French ANR SUPERFIELD, the EMFL and the LABEX NEXT. A portion of this work was performed at the National High Magnetic Field Laboratory, which is supported by the National Science Foundation Cooperative Agreement No. DMR-1157490, the State of Florida, and the U.S. Department of Energy. R.L., D.A.B. and W.N.H. acknowledge support from NSERC. L.T. acknowledges support from the Canadian Institute for Advanced Research and funding from NSERC, FRQNT, the Canada Foundation for Innovation and a Canada Research Chair.
Author information
Authors and Affiliations
Contributions
N.D.-L., S.R.d.C. and J.-H.P. performed the Seebeck and Nernst measurements at the NHMFL in Tallahassee. N.D.-L., F.L. and E.H. analysed the Seebeck data. S.B., S.L., D.L.B., D.V., B.V. and C.P. performed and analysed the resistance measurements at the LNCMI in Toulouse. B.J.R., R.L., D.A.B. and W.N.H. prepared the YBCO single crystals at UBC (crystal growth, annealing, detwinning and contacts). L.T. and N.D.-L. supervised the thermoelectric measurements. C.P. supervised the pulsed-field measurements. N.D.-L., L.T. and C.P. wrote the manuscript with input from all authors.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Doiron-Leyraud, N., Badoux, S., René de Cotret, S. et al. Evidence for a small hole pocket in the Fermi surface of underdoped YBa2Cu3Oy. Nat Commun 6, 6034 (2015). https://doi.org/10.1038/ncomms7034
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms7034
This article is cited by
-
High-T\(_c\) Cuprates: a Story of Two Electronic Subsystems
Journal of Superconductivity and Novel Magnetism (2022)
-
Phonons, electrons and thermal transport in Planckian high Tc materials
npj Quantum Materials (2021)
-
Hard antinodal gap revealed by quantum oscillations in the pseudogap regime of underdoped high-Tc superconductors
Nature Physics (2020)
-
Pauli-limit upper critical field of high-temperature superconductor La1.84Sr0.16CuO4
Scientific Reports (2019)
-
Effect of Short-Range Correlations on Spectral Properties of Doped Mott Insulators
Journal of Superconductivity and Novel Magnetism (2019)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.