Abstract
Conflict with conspecifics from neighbouring groups over territory, mating opportunities and other resources is observed in many social organisms, including humans. Here we investigate the evolutionary origins of social instincts, as shaped by selection resulting from between-group conflict in the presence of a collective action problem. We focus on the effects of the differences between individuals on the evolutionary dynamics. Our theoretical models predict that high-rank individuals, who are able to usurp a disproportional share of resources in within-group interactions, will act seemingly altruistically in between-group conflict, expending more effort and often having lower reproductive success than their low-rank group-mates. Similar behaviour is expected for individuals with higher motivation, higher strengths or lower costs, or for individuals in a leadership position. Our theory also provides an evolutionary foundation for classical equity theory, and it has implications for the origin of coercive leadership and for reproductive skew theory.
Similar content being viewed by others
Introduction
Social organisms living in stable groups often engage in aggressive interactions with conspecifics from neighbouring groups over territory, mating opportunities and other resources. The outcome of these interactions can significantly affect both individual fitness and group survival. Examples include border patrols in chimpanzees1,2, raiding parties in spider monkeys3, clan wars in hyenas4 and between-group fights in lions5, free-ranging dogs6, meerkats7, Capuchin monkeys8, blue monkeys9, black howler monkeys10 and ring-tail lemurs11.
As in other collective activities that lead to the production of a public good, aggressive between-group interactions result in a collective action problem: if a group member benefits from the action of group-mates and individual effort is costly, then there is an incentive to ‘free ride’, that is, to reduce one’s effort or to withdraw it completely. However, if individuals follow this logic, the public good is not produced and all group members suffer. Overcoming the collective action problem is a major challenge facing social groups across a range of species, including humans12,13,14. Theoretical studies of cooperation have identified several possible solutions for reducing free-riding tendencies, such as altruism, relatedness, direct and indirect reciprocity, and punishment15,16. Although these mechanisms should in general also apply to cooperation in between-group conflict, theoretical and empirical work is limited in scope17.
Here we investigate the collective action problem in between-group conflict within an evolutionary context. In particular, we study the effect of within-group inequality on provision of the public good, that is, differences between group-mates in dominance rank, status, motivation, valuation of the reward, costs paid and strength. We identify a mechanism that can overcome the collective action problem in groups of unrelated individuals without recourse to altruism, reciprocity or punishment.
Our focus is on the evolution of social instincts. As first argued by Darwin in On the Origins of Species, social instincts—that is, genetically based propensities that govern the behaviour of individuals in social interactions—have evolved by natural and sexual selection. In modern perspective, these instinctive social behaviours are plastic, resulting from the interaction of the genotype with the social environment (including the behaviour of social partners)18.
By focusing on the origin of social instincts, the framework we develop here aims to place the debate19 on between-group conflict in human evolution within a broad cross-specific perspective. A crucial evolutionary question, discussed already by Darwin in The Descent of Man, is how important between-group conflict was in shaping the human behavioural repertoire. Existing theoretical work suggests that relatively modest levels of mortality in between-group conflict could have sufficiently large evolutionary effects, driving the emergence of characteristic human abilities, biases and preferences (for example, cooperation, altruism, belligerence, parochialism and ethnocentrism), as well as social and cultural norms and institutions16,20,21,22,23,24. Like Darwin’s account, these theoretical approaches rely on factors that are exclusive to our species, such as cultural transmission aided by language, culturally enforced sharing norms or group decisions and particular within- and between-group interaction patterns (for example, cultural group selection)16,22. Given that our approach does not rely on these factors, our insights may apply to any species with between-group conflict.
This body of theoretical work is informed by inferences drawn from comparison with the social systems of other primate species, or from archaeological and ethnographic data16,25,26,27,28,29,30. Many of these inferences are contentious19. For example, the ethnographic data typically pertain to present-day mobile forager groups, which are characterized by an egalitarian social structure31. However, it is not clear to what extent this social system is a good ‘model’ for early human groups. Other types of small-scale societies may also provide clues to early human social systems, but they have been largely neglected in the literature on the evolution of cooperation. This includes semisedentary and sedentary forager groups with varying degrees of hierarchy and other types of inequality. Our work may provide insights into the dynamics of these groups and guide discussions of human cooperation towards a broader conceptual framework.
Our models are grounded in contest theory, an approach used widely in economics32. We extend in several important directions existing work on the evolution of cooperation in the context of between-group conflict. First, rather than allowing for only two discrete strategies (for example, cooperate and defect), we use a more general and realistic framework in which individual efforts and costs are treated as continuous variables; this is also more in line with experimental economic games, where subjects can typically vary their contributions continuously. Second, existing models of between-group conflict use standard public goods games, where individual benefit is a linear function of the number of contributors (or of their total effort). However, it is now recognized that this assumption can lead to misleading conclusions32,33. In our approach, benefits are specified by a nonlinear function explicitly capturing the efforts of both focal and competing groups. Third, existing approaches postulate a completely egalitarian division of spoils and disregard any differences between individuals except for the strategy they use. In contrast, as noted above we explicitly aim to capture the effects of differences between individuals on their strategies and the fitness consequences of their actions (for example, differences with respect to their dominance rank, status, motivation, valuation of the reward, costs paid and strength). Finally, compared with related work in the economics literature32,34, our approach adds biological realism by focusing on evolution by small mutations, by explicitly allowing for group extinction and multiplication and by investigating the effects of migration and genetic relatedness.
Here we study a series of mathematical models describing competition between groups comprising heterogenous individuals. Our models show that within-group inequality leads dominant individuals (or those with higher strengths or lower costs) to contribute the effort required to produce the public good (that is, to succeed in between-group competition) while the rest of the group free rides12. This is because they can ‘afford’ higher costs of contribution, owing to their larger shares of the public good. Their behaviour is seemingly altruistic, in the sense that they contribute more effort than their group-mates and often have lower fitness as a result. Their contribution is driven by competition with their counterparts in other groups rather than with their own group-mates. This mechanism can overcome the collective action problem in groups of unrelated individuals without resorting to altruism, reciprocity or punishment.
Results
Basic model
We consider a population of individuals living in a large number of groups G of constant size n. The groups engage in competition for resources, which affects their survival and multiplication as well as the reproduction of members of surviving groups. Groups can be egalitarian, so that each individual gets an equal share of the resources that the group obtains, or hierarchical, so that each individual’s share depends on its dominance rank.
Each group faces a collective action problem. The amount of resources obtained by each group depends on the total effort of its members towards the group success; at the same time, individuals pay fitness costs that increase with their efforts. Each individual’s effort is controlled genetically and is modelled as a non-negative continuous variable. In the case of hierarchical groups, individual efforts are conditioned on the dominance rank, which is assigned to group members randomly at each generation (for example, based on their strength). There is no coercion. We allow for mutation, recombination, migration and genetic relatedness between individuals.
To introduce our approach and results, we start with a simple model. Let Pj be a share of the resources obtained by group j in competition with other groups, and xij the effort of individual i in group j towards the group’s success (i=1,…,n; j=1,…,G). We specify the total group effort as

where the sum is taken over all group members, and define the group success probability Pj as

where the sum is taken over all groups32,35. We assume that the groups survive to leave offspring to the next generation with probabilities equal to Pj. For members of such groups, we define individual fertility as

where f0 is a constant baseline fertility (which can be set to 1 without loss of generality), B is the total (normalized) resource contested by the groups, c is the cost parameter and vi is the share of the group’s reward allocated to individual i. We treat valuations vi as constants dependent on the within-group dominance rank (v1≥v2≥…≥vn; ∑ vi=1).
The groups that do not survive are replaced by the offspring of surviving groups. Generations are discrete and non-overlapping. Specifically, we assume that each group in the current generation descends from a group in the previous generation chosen randomly and independently with probabilities Pj. Individuals in each group descend from individuals in their parental group independently with probabilities  , where
, where  is the average fertility in group j (=Σifij/n). Under these assumptions, the fitness of individual i from group j is
is the average fertility in group j (=Σifij/n). Under these assumptions, the fitness of individual i from group j is

In what follows it will be convenient to use parameter b=B/(nG), which is the expected benefit per individual in an egalitarian group if all individuals in the population are identical. Below we describe our key findings. A technical explanation and derivations of our results are given in the Methods.
Egalitarian groups
Consider first the case when groups are egalitarian so that vi=1/n for all i. Then, the population is predicted to evolve to an equilibrium at which the total group effort is

while each individual effort is  =X*/n. As expected, both individual and group efforts increase with benefit b and decrease with cost c. Increasing the group size n (while keeping b constant) does not change the total group effort, but it decreases the individual effort as a result of increasing free riding. If the benefit per individual b decreases with group size n, then the total group effort
=X*/n. As expected, both individual and group efforts increase with benefit b and decrease with cost c. Increasing the group size n (while keeping b constant) does not change the total group effort, but it decreases the individual effort as a result of increasing free riding. If the benefit per individual b decreases with group size n, then the total group effort  will decrease with increasing n.
will decrease with increasing n.
These results assume that the groups are formed uniformly at random each generation, implying that group members are no more genetically related to one another than they are to members of other groups. The evolution of cooperation in a public goods game as studied here is driven by the overlapping interests of group-mates36 and does not require genetic relatedness (or reciprocity, coercion or punishment)33,37. Genetic relatedness does however increase cooperation15. In the model considered here, with average within-group relatedness r and no between-group relatedness, each individual effort increases by factor

For example, let female offspring disperse randomly between groups while male offspring stay in the natal group (as in chimpanzees and, likely, in our ancestors38). This will result in a ~30% increase in the individual and group efforts relative to the case of zero relatedness (assuming that n≥5).
Hierarchical groups
The results outlined above assume a completely egalitarian division of the spoils. In hierarchical groups, the predicted collective action behaviour is strikingly different. With random group formation, only individuals with valuations vi higher than a certain threshold will make a non-zero effort, while low valuators will free ride contributing nothing.
All ranks will contribute only if, for each i,

Note that the threshold value vcrit is close to 1/n if the group size n and/or benefit b are large. If all ranks do contribute, then the total group effort X*=(1+b)/c, which is the same as in egalitarian groups.
Ranks for which vi<vcrit will contribute nothing. Counter-intuitively, the total group effort X* increases as the number of contributing ranks ne decreases. This happens because the remaining high-valuation contributors increase their efforts, effectively overcompensating for the lack of effort by the free-riding ranks.
Individual effort xi* increases with valuation vi; counter-intuitively, however, the individual fertility  of contributing ranks decreases with valuation. That is, high-rank group members have lower fertility than their low-rank group-mates. This results from their increasing efforts and the correspondingly higher costs they pay, which negate the higher share of the reward that they claim. (For non-contributing ranks, fertility
of contributing ranks decreases with valuation. That is, high-rank group members have lower fertility than their low-rank group-mates. This results from their increasing efforts and the correspondingly higher costs they pay, which negate the higher share of the reward that they claim. (For non-contributing ranks, fertility  increases with valuation vi).
increases with valuation vi).
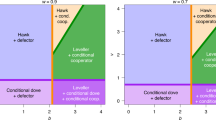
The above inequality is a necessary but not sufficient condition for rank i to contribute. (A recursive sufficient condition is given in the Methods). Because of this threshold effect, small differences in valuation can result in large differences in efforts and reproductive success. In the extreme case when only the highest valuator contributes effort, its share of group reproduction is the smallest in the group (and it is smaller than 1/n), in spite of this individual getting the highest share of the reward. Figure 1 illustrates our results for the case of only two individuals per group (n=2), which is a common set-up in reproductive skew theory39,40. Note that increasing the valuation increases fitness only if the individual free rides or if it is the only contributor. Otherwise, increasing the valuation decreases its share of reproduction.
Increasing the value of the resource b while keeping n fixed increases both individual efforts and the share of the effort contributed by rank-1 individuals. At the same time, this decreases their reproductive success because of increasing costs. Increasing the degree of inequality increases the group effort  and can, counter-intuitively, increase the reproductive success of low-rank individuals.
and can, counter-intuitively, increase the reproductive success of low-rank individuals.
The key insight is that high-rank individuals behave seemingly altruistically: they contribute effort to the collective action at a cost, which reduces their fitness compared with their free-riding lower-rank group-mates. This behaviour is however not altruistic: they are maximizing their fitness by contributing, because they would not be better off by reducing their contribution or by not contributing. These individuals are essentially competing with high-rank individuals in the other groups, rather than with their own group-mates.
Numerical simulations
To confirm our conclusions using a more realistic description of the population structure, limited dispersal and the resulting genetic relatedness, we used individual-based simulations (see Methods). All individuals were haploid. The effort at each rank was controlled by an independent unlinked locus with a continuum of effects. Each group comprised n males and n females. Only males contributed to group competition and received benefits proportionally to their valuations. Females from surviving groups had equal reproductive success and each female produced two offspring. The fathers were chosen among the group males proportionally to their fertilities fi. The offspring sex was assigned randomly, but an equal sex ratio was enforced within each group. Female offspring dispersed randomly while male offspring stayed in the natal group.
Numerical simulations show that, relative to the case of random group formation, genetic relatedness increases individual and group efforts and causes more low valuators to start contributing towards the group’s success. The overall effect is however not large because relatedness remains relatively low (see Supplementary Figs 1–6). Figures 2, 3, 4 illustrate our results using a model in which individual valuations are proportional to a power function of individual rank: vi~(n+1−i)δ. Here δ is a parameter measuring the degree of inequality: with δ=0, all valuations are equal to 1/n, with δ=1 valuation vi is a linear function of individual rank i and with δ>1 it is a decreasing, concave-up function of rank. In simulations, we used five values of δ=0.25, 0.5, 1, 2, 4.
Results over 200,000 generations for a particular run with group size n=8 and within-group inequality δ=2. The values are averages over individuals of rank i in all groups in the population. Colours describe different ranks, from rank 1 (red) to rank 8 (dark green). (a) Individual efforts xi for rank i; the average individual effort over all ranks is shown with the dashed line. (b) Relative fertilities  for individuals of rank i.
for individuals of rank i.
Summary results of the last 20,000 generations for a particular set of 10 runs with group size n=12 and within-group inequality δ=1, as a function of individual valuation vi. For each run, the values are averages over individuals of rank i in all groups in the population. Values for individual runs are given by circles, the average over the 10 runs is given by a solid line. (a) Individual efforts xi for rank i. (b) Relative fertilities  for individuals of rank i.
for individuals of rank i.
Summary results over all runs for one set of parameters, with group size n=4 (a,b) and n=8 (c,d) and cost c=0.5. For each set of runs, the values are averages over individuals of rank i in all groups in the population. Colours show the relevant amounts for individuals of different ranks, from the rank-1 individual at the bottom (red) to the rank-n individual at the top. Each set of bars corresponds to a specific value of benefit b. Each bar within a set corresponds to a specific value of within-group inequality δ, from the smallest on the left (δ=0.25; low inequality) to the largest on the right (δ=4; high inequality). (a) Individual efforts xi for rank i with group size n=4; the height of the bar is the total group effort X*. (b) Share of reproduction for individuals of rank i with group size n=4. (c) Individual efforts xi for rank i with group size n=8; the height of the bar is the total group effort X*. (d) Share of reproduction for individuals of rank i with group size n=8.
Figure 2 illustrates the dynamics of individual efforts and fertilities with n=8. In this case, only the top three ranks make substantial contributions. (Note that because of mutation individual contributions always remain positive). Figure 3 shows the average efforts and fertilities for different ranks with n=12. The ranks with the lowest valuations contribute almost nothing and their fertilities increase with valuation. The contributions of the high valuators increases linearly with vi but their fertilities decrease linearly with vi. Figure 4 is a summary graph showing that as within-group inequality increases (measured by δ), the individual efforts of high valuators increase while their shares of reproduction decrease. They also show that the total group effort grows with within-group inequality and benefit b.
Generalizations
To check the robustness of our conclusions, we have also studied two modifications of the basic model. In the first version, the probability of group success was specified as

where β is a positive parameter measuring the ‘decisiveness’ of the group strength: β>1 implies that stronger groups will get disproportionately large shares of the reward32. Increasing β increases both the individual and group efforts (as expected), but it results in no other qualitative differences (Fig. 5).
Group size n=8 and cost c=0.5. (a,b) Summary results of the last 20,000 generations for a particular set of 10 runs with within-group inequality δ=1, as a function of individual valuation vi. For each set of runs, the values are averages over individuals of rank i in all groups in the population. Values for individual runs are given by circles, the average over the 10 runs is given by a solid line. (a) Individual efforts xi for rank i. (b) Relative fertilities  for individuals of rank i. (c,d) Summary results over all runs for one set of parameters. For each run, the values are averages over individuals of rank i in all groups in the population. Colours show the relevant amounts for individuals of different ranks, from the rank-1 individual at the bottom (red) to the rank-8 individual at the top (dark green). Each set of bars corresponds to a specific value of benefit b. Each bar within a set corresponds to a specific value of within-group inequality δ, from the smallest on the left (δ=0.25; low inequality) to the largest on the right (δ=4; high inequality). (c) Individual efforts xi for rank i; the height of the bar is the total group effort X*. (d) Share of reproduction for individuals of rank i.
for individuals of rank i. (c,d) Summary results over all runs for one set of parameters. For each run, the values are averages over individuals of rank i in all groups in the population. Colours show the relevant amounts for individuals of different ranks, from the rank-1 individual at the bottom (red) to the rank-8 individual at the top (dark green). Each set of bars corresponds to a specific value of benefit b. Each bar within a set corresponds to a specific value of within-group inequality δ, from the smallest on the left (δ=0.25; low inequality) to the largest on the right (δ=4; high inequality). (c) Individual efforts xi for rank i; the height of the bar is the total group effort X*. (d) Share of reproduction for individuals of rank i.
In another modification, we assumed that group-mates share the reward equally (vi=1/n) but differ with respect to the cost coefficient ci. This may be the case if some individuals are stronger than others, so that the same amount of effort can be less costly for them in fitness terms. In our simulations, we used costs ci that increased linearly with rank i. Specifically, we used n equally spaced values of ci from (1−d)/2 to (1+d)/2 with five different values of d: 0.05 (small differences in costs), 0.1, 0.2, 0.4, 0.8 (large differences in costs). Our major conclusions remain qualitatively valid, with low-cost individuals playing the role of high-valuation group members in the basic model (Fig. 6). Note that the model with differences in costs is mathematically equivalent to a model with a constant cost c but with differences in fighting abilities si and the total group effort defined as
Group size n=8 and egalitarian division of spoils (that is, the individual shares of the reward are vi=1/n for all i). (a,b) Summary results of the last 20,000 generations for a particular set of five runs with within-group inequality d=0.2, as a function of individual cost ci. For each run, the values are averages over individuals of rank i in all groups in the population. Values for individual runs are given by circles, the average over the five runs is given by a solid line. (a) Individual efforts xi for rank i. (b) Relative fertilities  for individuals of rank i. (c,d) Summary results over all runs for one set of parameters. For each set of runs, the values are averages over individuals of rank i in all groups in the population. Colours show the relevant amounts for individuals of different ranks, from the rank-1 individual at the bottom (red) to the rank-8 individual at the top (dark green). Each set of bars corresponds to a specific value of benefit b. Each bar within a set corresponds to a specific value of parameter d controlling differences in costs, from the smallest on the left (d=0.05; small difference in costs) to the largest on the right (d=0.8; high difference in costs). We used n equally spaced values of individual costs ci from c × (1−d)/2 to c × (1+d)/2. (c) Individual efforts xi for rank i; the height of the bar is the total group effort X*. (d) Share of reproduction for individuals of rank i.
for individuals of rank i. (c,d) Summary results over all runs for one set of parameters. For each set of runs, the values are averages over individuals of rank i in all groups in the population. Colours show the relevant amounts for individuals of different ranks, from the rank-1 individual at the bottom (red) to the rank-8 individual at the top (dark green). Each set of bars corresponds to a specific value of benefit b. Each bar within a set corresponds to a specific value of parameter d controlling differences in costs, from the smallest on the left (d=0.05; small difference in costs) to the largest on the right (d=0.8; high difference in costs). We used n equally spaced values of individual costs ci from c × (1−d)/2 to c × (1+d)/2. (c) Individual efforts xi for rank i; the height of the bar is the total group effort X*. (d) Share of reproduction for individuals of rank i.

In this model, individuals with larger fighting abilities si will, ceteris paribus, contribute more to X. Therefore, our conclusions are applicable to the case of within-group variation in strengths. That is, lower-cost or higher-strength individuals are predicted to contribute more effort than their group-mates, and they can have lower reproductive success as a result.
Discussion
We have studied the collective action problem in between-group conflict within an evolutionary context, focusing on the effect of within-group inequality in dominance rank, status, motivation, valuation of the reward, costs paid and strength. We have shown that inequality in rank can lead dominant individuals to act seemingly altruistically towards their group-mates by contributing the effort required to succeed in between-group competition while others free ride. As a result, these individuals have reduced reproductive success compared with their free-riding subordinate group-mates. Analogous reasoning applies when individuals differ in the costs incurred from contributing to the public good or when they differ in strength: lower-cost or higher-strength individuals contribute more effort to the public good, and they have reduced reproductive success compared with their free-riding group-mates as a result.
This behaviour may seem altruistic but actually it is not. In the case of hierarchical groups, for example, dominant individuals maximize their fitness by contributing; given the subordinates’ lack of contribution, dominants will not be better off by reducing their contribution or by withholding it completely. Thus, the non-contributors are indeed free riding, but the contributors are not altruistic; paradoxically, they are acting in their own interest by contributing to the public good. What is driving their contribution is that they are essentially competing with their counterparts in other groups rather than with their own group-mates.
The outcome of seemingly altruistic behaviour by dominant individuals (or those with lower costs or higher strength) is consistent with Olson’s controversial conclusion that individuals who obtain the greatest share of a public good bear a disproportionate share of the costs, leading to ‘a systematic tendency for ‘exploitation’ of the great by the small!’ [12, (p. 29)]. Olson’s insight relates to the phenomenon of collective action within a single group. Here we have shown that this effect can be even more extreme when the driving force of collective action is between-group conflict coupled with within-group inequality. In this case, groups of unrelated individuals can ‘solve’ the collective action problem without resorting to altruism, reciprocity or punishment.
Note that we assumed that individual efforts are only limited by the requirement of non-negative fertility (cxi<1+bnvi). However, if there is a smaller upper limit on xi (for example, due to physiological, energetic or time constraints), then a single individual (or a small group) may not be able to contribute the optimum effort predicted by our theory. In this case, we expect that the number of contributors will increase34 relative to our predictions.
Our work has connections with several other classes of models and results in behavioural biology; here we outline some of the similarities, differences and their implications. The mechanism for the evolution of seemingly altruistic behaviour we identify is analogous to the emergence of ‘altruistic’ soma in multicellular organisms41, but without the need for genetic relatedness. Our results show that within-group genetic relatedness increases the ‘altruistic’ effort of dominant individuals but is not required for the mechanism to operate.
The system state with heterogenous groups in which only some individuals make an effort while the rest free ride is somewhat similar to polymorphic states observed in producer–scrounger models42,43. In these models, some individuals pay fitness costs to discover resource items, while the rest avoid these costs by using the resources discovered by others. The major difference is that while in classical producer–scrounger models the two strategies have equal fitness at equilibrium, in between-group conflict ‘producers’ (that is, contributors) have lower fitness than their free-riding group-mates.
Our insights on hierarchically structured groups bear on work on reproductive skew, the unequal partitioning of reproduction within a group39,40. Models in reproductive skew theory focus on the evolution of investment in within-group competition (for example, by dominant versus subordinates), given its negative effect on between-group competition and given genetic relatedness between group members. In contrast, we assume that the within-group division of benefits is set by factors endogenous to the model (for example, based on rank), and we study the evolution of costly investment in between-group competition. In our models, genetic relatedness is of secondary importance. Most of the modelling work on reproductive skew involves groups with two reproductive classes and linear production functions. Our results and techniques for groups of arbitrary size and nonlinear production functions can be used to obtain additional insights into the evolution of reproductive skew. For example, a key question in this area is why some individuals (for example, subordinates) ‘stick around’ in stable groups when other individuals (for example, dominants) monopolize a disproportionate share of reproductive opportunities39,40. Qualitatively, one insight from our work is that, in between-group conflict scenarios, subordinates can reap the benefits of collective action without bearing any costs, which are borne instead by dominant individuals. This may provide an incentive for subordinates to stay in the group. Thus, while in reproductive skew theory subordinate individuals are commonly viewed as making ‘the best of a bad job’, in our models they are in fact better off than dominant individuals.
In hierarchical groups, dominant individuals are able to bully their group-mates, typically usurping a disproportional share of material resources and mating opportunities. Reducing the negative effects of dominant bullies on their group-mates requires the formation of coalitions between subordinates31,44,45,46. Our theory predicts that, at the same time, dominant bullies may act ‘altruistically’ towards their group-mates in between-group conflict situations. As we saw above, this is because, once a within-group hierarchy is established, alpha-individuals effectively compete against alpha-individuals in other groups rather than against their own group-mates; the competition can drive the within-group fitness of alpha-individuals to very low values. Note that this only applies to public goods contested by groups: if a group survives the between-group conflict, then there are other resources that dominant individuals can seize from their group-mates in within-group interactions. Therefore, high-rank individuals are not necessarily worse off in terms of overall fitness.
A final connection is with the growing literature on the evolution of leadership and followership and on the psychological mechanisms underlying these traits in humans. One of the roles ascribed to leaders in this literature is punishment of defectors in collective action47,48 or punishment of individuals who disrupt group cohesion49. Our models do not allow for punishment. However, our results suggest that, were this included, dominant individuals in hierarchical groups may evolve the tendency to punish low-rank free riders to increase their effort in collective action. This expectation is reinforced by the results of experimental economic games showing that between-group competition enhances punishment of free riders50; they also show that, when individuals are heterogenous with respect to the costs of administering punishment, the tacit expectation is that punishment will be carried out by the group member with the smallest cost51. An open question is how exactly individuals will partition their effort between punishing their group-mates and contributing directly to between-group competition.
Overall, our models predict higher effort for high-rank individuals towards collective action in between-group competition. This is consistent with empirical observations across a range of species, including humans. In chimpanzees, high-rank males travel further into the periphery during border patrols2 and males with higher mating success are more likely to engage in this activity1, which is energetically costly52. In ring-tail lemurs11 and blue monkeys9, high-rank females participate more in the defence of communal feeding territories than low-rank females; note that in both species, males disperse from the natal group, that is, the dispersal pattern is reversed compared with the scenario captured by our simulation set-up. In meerkats, dominant males respond more strongly to intruder scent marks53. High-rank chacma baboon males are more likely than low-rank males to join inter-group loud call displays17. In some human groups, the most aggressive warriors have lower reproductive success than other men, as documented for the horticulturalist/forager Waorani of Ecuador54.
Our results are also relevant for explaining the propensity to sacrifice (that is, to expend relatively more effort) of individuals in leadership positions in warfare and other between-group competition scenarios. For example, the highest mortality in the US Army in the Iraq War was among First and Second Lieutenants, who typically lead combat patrols55. Note that we predict higher efforts and costs not only for individuals who actually hold or have been assigned high rank or status, but also for ‘potential alphas’ who have higher valuation of the prize, lower costs or higher strength. Finally, our work suggests that bullish and ‘unethical’ behaviour by high-rank individuals towards their group-mates56 will be accompanied by their higher contributions towards between-group competition. Although experimental studies of public good games with between-group competition57,58,59 typically do not allow for within-group variation in valuation, the data available for humans do show that more competitive, individualistic players contribute more to public goods with group competition60 and that individuals of high status contribute more towards group goals61.
We conclude our discussion by outlining some implications that apply specifically to human behaviour. One set of implications relates to the emergence of an evolved psychology for egalitarian tendencies in hierarchical groups46. This process is linked to the intentional eradication of dominant bullies (and their genes) by their group-mates, as in the ‘reverse dominance hierarchies’ of present-day mobile forager groups31,44. In related studies, Bowles and co-authors have argued that genes for altruism can proliferate relatively easily in the presence of culturally enforced leveling mechanisms, such as meat sharing. This would have resulted in greater efficiency of egalitarian groups in between-group conflict, leading to the emergence of an egalitarian social structure via cultural group selection62,63.
Our work offers a different perspective. First, our results show that high-rank bullies make key contributions in between-group conflict and that ‘despots’ can dialectically become ‘altruists’. Such individuals may have played an important role in human social evolution and genes for such behaviour may have been maintained in the population by selection. Second, in our models the total group effort typically increases with the degree of inequality. (If all group members contribute, then the total group effort does not depend on the degree of inequality). This implies that hierarchical groups will expend more effort in between-group conflict and are thus likely to outcompete more egalitarian groups. Overall, our theory suggests that humans may have stronger innate preferences for an egalitarian social structure in the absence of between-group conflict46 and for a hierarchical social structure in its presence. Egalitarian groups are subject to stronger overall free riding and as a result they may allocate less effort to between-group conflict. More broadly, our work adds a new mechanism to those commonly invoked to explain the evolution of cooperation in humans. This mechanism does not rely on factors that are exclusive to our species (for example, cultural transmission aided by language) or traits characteristic of a particular type of human social system (for example, the leveling mechanisms attributed to mobile forager groups). Our work thus extends the range of information that can be brought to bear on questions about human social evolution.
A final implication of our work that applies specifically to human behaviour relates to the origin of fairness norms. Social instincts that evolved under ancestral conditions of between-group conflict may also affect preferences and biases exhibited in peaceful collective activities, such as one’s day job. If people feel that they are fairly compensated, then they are generally happier at work and more motivated to continue contributing input at the same level. But what is the ‘fair’ compensation when individuals involved in a collective project are unequal in their roles and types of inputs? The answer provided by classical equity theory64,65 is that employees seek to maintain equity between their input-to-output ratio and that of others. (The theory does recognize that the ‘input’ of workers includes many factors besides working hours, and that their ‘output’ includes many rewards besides money). Although equity theory has attracted a number of consistent criticisms, including the lack of evolutionary foundations65, it remains one of the more important and successful theories in the field of organizational behaviour. While the expectation of a positive relationship between output and input seems quite natural, why this relationship should be linear (as postulated in equity theory) rather than, say, quadratic is a priori unclear. In our models, evolution adjusts the efforts of contributing individuals so that they become linearly aligned with the individual shares of the reward emerging from the collective action (Figs 3a and 5a). Thus, our results provide a theoretical evolutionary foundation for a major assumption of classical equity theory.
Clearly, in collective action as in other domains, human behaviour is greatly influenced by cultural institutions, societal norms, the possibility of punishment, preferences, emotions and so on31,44. Human history provides many examples of norms and institutions that reduce free riding in more egalitarian groups, making them efficient in competition. For instance, early eighteenth century pirates formed effective predatory groups and had a relatively ‘flat’ structure: the captain’s share of the booty was only twice as large as the share of an ordinary crew member66. In the context of business organizations, the advantages of hierarchical versus more egalitarian firms in competition with one another are currently hotly debated67,68. Hence, the theory outlined here, with its exclusive focus on the emergence of genetically controlled social instincts, is only part of the picture. However, we contend that this is an important part—one that helps us ground the evolution of human behaviour within a broad cross-specific perspective.
Methods
Fitness
In our models, the fitness of individual i from group j can be written as

To study our models, we use the evolutionary invasion analysis (adaptive dynamics69) approach, which focuses on the invasion fitness w(y|x), that is, the fitness of a rare mutant with trait y in a monomorphic population of individuals with trait x. We assume that the effort for each rank is specified genetically and that there is only a single segregating mutation across all the ranks at any one time.
Egalitarian groups
Assume that group members share the reward equally so that nvi=1 for each i. To evaluate the change in individual effort x per generation, one needs to calculate the selection gradient of the invasion function  at y=x. To find the invasion fitness w(y|x), we need to set xij=y,
at y=x. To find the invasion fitness w(y|x), we need to set xij=y,  , fij=1+bGPj−cy,
, fij=1+bGPj−cy,  and
and

in equation (10). In the limit of large G, the selection gradient  at y=x is proportional to 1+b−cnx. Then, one finds that at equilibrium
at y=x is proportional to 1+b−cnx. Then, one finds that at equilibrium

Summing up the individual contributions, the total group effort X*=(1+b)/c and thus it does not depend on n.
Let r0 and r1 be the probabilities that a randomly chosen group-mate of a mutant and a randomly chosen individual from a different group have the same mutation. Then, the equilibrium effort x* is increased by a factor

Note that if female offspring disperse randomly between groups while male offspring stay in the natal group, the males within the group will be genetically related with average relatedness70  . If the number of groups is large, r1 will be very close to zero.
. If the number of groups is large, r1 will be very close to zero.
Assume that the probability of success is  . Then,
. Then,

One can show that with relatedness and decisiveness, the total group effort X* increases with the group size n (assuming r1<<r0).
Hierarchical groups
Assume that group members differ in their valuations vi and that each group is characterized by exactly the same set of vi values. To find the invasion fitness w(yi|xi) for the effort xi at rank i, we need to set xij=yi, fij=1+bGPjnvi−cyi,  ,
,  and
and

in equation (10). Here X=∑jxj and X−i=X−xi.
Then, in the large G limit, the selection gradient  at y=x for xi is proportional to
at y=x for xi is proportional to

This equation is linear in xi. It follows that effort xi at each rank i evolves either to a positive value or to zero.
Assume first that the effort at each rank evolves to a positive value  . Summing up equation (16) over all i with account that ∑vi=1 and ∑xi=X, one finds that X satisfies to a quadratic equation
. Summing up equation (16) over all i with account that ∑vi=1 and ∑xi=X, one finds that X satisfies to a quadratic equation

Therefore, at equilibrium

(The other solution, X*=n(1+b)/c, results in zero average fertility and thus is not biologically relevant). That is, the total group effort does not depend on the group size or on the distribution of the valuations. The equilibrium individual efforts are

This equilibrium is feasible (that is, all xi*>0) if for all i

Note that increasing the value of the resource b or the group size n moves vcrit closer to 1/n, so that individuals with a valuation smaller than the average will not contribute but will free ride.
Equation (19) shows that individual efforts  increase with valuation vi. In contrast, individual reproductive success at equilibrium is
increase with valuation vi. In contrast, individual reproductive success at equilibrium is

and thus decreases with valuation. That is, the higher costs paid by high-rank individuals negate their larger shares of the reward.
If not all group members make a positive effort, then the derivations become more complex. Let there be ne contributors and v be their total valuation (v=∑ vi where the sum is over ne contributors). Then, summing up equation (16) over all contributors, at equilibrium their total effort X satisfies to a quadratic equation

Of the two solutions of this quadratic, only the smallest one, X*, is relevant biologically (the other solution leads to negative fertilities). Note that cX*≤ne+bnΣvi<n(1+b). (The first inequality follows from the requirement that all fi values are non-negative:  , which one then sums up over all contributing individuals).
, which one then sums up over all contributing individuals).
The individual efforts  can be found from equation (16) by substituting X* into
can be found from equation (16) by substituting X* into

This equilibrium is biologically feasible (that is, all  ), if
), if

Equation (23) shows that individual effort increases with valuation. However, within-group reproductive success

and is decreasing with valuation vi because cX*≤n(b+1).
By differentiating the expression for X*, one can show that X* always increases with v and generally decreases with ne. (The latter requires that v exceeds a certain threshold that depends on b and n but is relatively small). That is, decreasing the number of contributors and/or increasing their total share of the reward results in increasing group effort. Moreover, analytical derivation and numerical simulations strongly suggest that the derivative of vcrit with respect to X is positive, so that the threshold valuation increases with decreasing the number of contributors. This leads to a recursive procedure for finding the equilibrium: (i) set  for all ranks for which inequality (20) is not satisfied; (ii) using appropriate values of ne and v, find X* from equation (22); (iii) if there are ranks for which inequality (24) is not satisfied, set their contributions
for all ranks for which inequality (20) is not satisfied; (ii) using appropriate values of ne and v, find X* from equation (22); (iii) if there are ranks for which inequality (24) is not satisfied, set their contributions  to zero, recompute ne and v, and return to step (ii).
to zero, recompute ne and v, and return to step (ii).
Assume there is only one contributor per group, say, individual of rank 1 so that ne=1 and  . By evaluating the left-hand side of equation (22) at
. By evaluating the left-hand side of equation (22) at  , one can show that the resulting expression is positive and thus the corresponding root of equation (22), that is,
, one can show that the resulting expression is positive and thus the corresponding root of equation (22), that is,  , is larger than bnv1. In this case, the contributor's fertility
, is larger than bnv1. In this case, the contributor's fertility  , while for all other group members fj≥1. This means that the share of reproduction
, while for all other group members fj≥1. This means that the share of reproduction  of the dominant individual is smaller than 1/n.
of the dominant individual is smaller than 1/n.
As another illustration, assume that there are only two individuals per group (n=2), which is a common set-up in reproductive skew theory39,40. Then, individual i contributes a positive effort only if its valuation

If both individuals contribute, their joint and individual efforts are given by equations (18) and (19) with n=2. If only individual i contributes, its effort can be found from equation (22) with n=2, ne=1, v=vi and X=xi. Figure 1 illustrates the dependence of individual contributions xi and the shares of reproduction fi/(f1+f2) on valuation.
Numerical results
We have not been able to get analytical results for models that would include both between-individual heterogeneity (in costs or valuations) and genetic relatedness. Instead, we studied the effects of these factors numerically.
To produce the next generation, first we identified a ‘parental group’ for each ancestral group (by random and independent sampling with probabilities Pj). Then, for each female in the parental group, we identified two fathers for her two children (by random and independent sampling with probabilities  ). To produce each offspring, free recombination was followed by mutation with a small probability. Mutational effects at each locus were drawn randomly and independently from a truncated normal distribution with zero mean.
). To produce each offspring, free recombination was followed by mutation with a small probability. Mutational effects at each locus were drawn randomly and independently from a truncated normal distribution with zero mean.
In numerical studies of the basic model, we used all possible combinations of the following parameter values: expected benefit per individual b=0.25, 0.5, 1.0; cost coefficient c=0.25, 0.5, 1.0; group size n=4, 8, 12; inequality parameter δ=0.25, 0.5, 1.0, 2.0, 4.0. We performed 10 runs for each parameter combination. Some parameters did not change: number of groups G=1,000, mutation rate μ=0.001 per gene per generation, standard deviation of the mutational effect σμ=0.1. In models with hierarchical groups, all n genes controlling the efforts at different ranks were unlinked. The initial individual efforts were chosen randomly and independently from a uniform distribution on [0,0.05]. Simulations ran for 200,000 generations. To avoid the appearance of negative fitness values in numerical simulations, we introduced upper boundaries on individual efforts xi,max=(1+bnvi)/c. We used a zero lower boundary on xi.
Supplementary Figs 1–6 summarize the results for three different sets of simulations: (i) the basic model as outlined above (Supplementary Figs 1 and 2), (ii) the model with decisiveness coefficient β=2 (Supplementary Figs 3 and 4) and (iii) the model of an egalitarian population with the cost coefficients ci increasing linearly with rank (Supplementary Figs 5 and 6). Within each set, we show separately the results for the case of no genetic relatedness (Supplementary Figs 1, 3 and 5) and for the case of genetic relatedness resulting from male philopatry (Supplementary Figs 2, 4 and 6). No genetic relatedness was obtained by enforcing random dispersal of male offspring in addition to random dispersal of female offspring.
Additional information
How to cite this article: Gavrilets, S. & Fortunato, L. A solution to the collective action problem in between-group conflict with within-group inequality. Nat. Commun. 5:3526 doi: 10.1038/ncomms4526 (2014).
References
Watts, D. P. & Mitani, J. C. Boundary patrols and intergroup encounters in wild chimpanzees. Behaviour 138, 299–327 (2001).
Wilson, M. L., Kahlenberg, S. M., Wells, M. & Wrangham, R. W. Ecological and social factors affect the occurrence and outcomes of intergroup encounters in chimpanzees. Anim. Behav. 83, 277–291 (2012).
Aureli, F., Schaffner, C., Verpooten, J., Slater, K. & Ramos-Fernandez, G. Raiding parties of male spider monkeys: Insights into human warfare? Am. J. Phys. Anthropol. 131, 486–497 (2006).
Boydson, E. E., Morelli, T. L. & Holekamp, K. E. Sex differences in territorial behavior exhibited by the spotted hyena (Hyaenidae, Crocuta crocuta). Ethology 107, 369–385 (2001).
Mosser, A. & Packer, C. Group territoriality and the benefits of sociality in the African lion, Panthera leo. Anim. Behav. 78, 359–370 (2009).
Bonanni, R., Valsecchi, P. & Natoli, E. Pattern of individual participation and cheating in conflicts between groups of free-ranging dogs. Anim. Behav. 79, 957–968 (2010).
Mares, R., Young, A. J. & Clutton-Brock, T. H. Individual contributions to territory defence in a cooperative breeder: weighing up the benefits and the costs. Proc. R. Soc. Lond. B 279, 3989–3995 (2012).
Perry, S. Intergroup encounters in wild white-faced capuchins (Cebus capucinus). Int. J. Primatol. 17, 309–330 (1996).
Cords, M. Variable participation in the defense of communal feeding territories by blue monkeys in the Kakamega Forest, Kenya. Behaviour 144, 1537–1550 (2007).
Kitchen, D. M., Horwich, R. H. & James, R. A. Subordinate male black howler monkey (Alouatta pigra) responses to loud calls: experimental evidence for the effects of intra-group male relationships and age. Behaviour 141, 703–723 (2004).
Nunn, C. L. & Deaner, R. O. Patterns of participation and free riding in territorial conflicts among ringtailed lemurs (Lemur catta). Behav. Ecol. Sociobiol. 57, 50–61 (2004).
Olson, M. The Logic Of Collective Action: Public Goods And The Theory Of Groups Harvard University Press (1965).
Nunn, C. L. in Primate Males: Causes and Consequences of Variation in Group Composition (ed. Kappeler, P. M.), 192–204 (Cambridge University Press, 2000).
Willems, E. P., Hellriegel, B. & van Schaik, C. P. The collective action problem in primate territory economics. Proc. R. Soc. Lond. B 280, 20130081 (2013).
McElreath, R. & Boyd, R. Mathematical Models Of Social Evolution: A Guide For The Perplexed Chicago University Press (2007).
Bowles, S. & Gintis, H. A Cooperative Species: Human Reciprocity and Its Evolution Princeton University Press (2011).
Kitchen, D. M. & Beehner, J. C. Factors affecting individual participation in group-level aggression among non-human primates. Behaviour 144, 1551–1581 (2007).
Robinson, G. E., Fernald, R. D. & Clayton, D. F. Genes and social behavior. Science 322, 896–900 (2008).
Culotta, E. Latest skirmish over ancestral violence strikes blow for peace. Science 341, 224 (2013).
Bowles, S. Did warfare among ancestral hunter-gatherers affect the evolution of human social behaviors? Science 324, 1293–1298 (2009).
Bowles, S. Group competition, reproductive leveling, and the evolution of human altruism. Science 314, 1569–1572 (2006).
Lehmann, L. & Feldman, M. W. War and the evolution of belligerence and bravery. Proc. R. Soc. Lond. B 275, 2877–2885 (2008).
Gavrilets, S., Anderson, D. G. & Turchin, P. Cycling in the complexity of early societies. Cliodynamics 1, 5536t55r (2010).
Turchin, P., Currie, T. E., Turner, E. A. L. & Gavrilets, S. War, space, and the evolution of Old World complex societies. Proc. Natl Acad. Sci. USA 110, 16384–16389 (2013).
Chagnon, N. A. Life histories, blood revenge, and warfare in a tribal population. Science 239, 985–992 (1988).
Wrangham, R. W. & Glowacki, L. Intergroup aggression in chimpanzees and war in nomadic hunter-gatherers: evaluating the chimpanzee model. Hum. Nat. 23, 5–29 (2012).
Walker, R. S. & Bailey, D. H. Body counts in lowland South American violence. Evol. Hum. Behav. 34, 29–34 (2013).
Ferguson, R. B. in War, Peace, and Human Nature: the Convergence of Evolutionary and Cultural Views (ed. Fry, D. P.), 112–131 (Oxford University Press, 2013).
Fry, D. P. & Söderberg, P. Lethal aggression in mobile forager bands and implications for the origins of war. Science 341, 270–273 (2013).
Wilson, M. L. in War, Peace, and Human Nature: the Convergence of Evolutionary and Cultural Views (ed. Fry, D. P.), 361–388 (Oxford University Press, 2013).
Boehm, C. Hierarchy in the Forest: The Evolution of Egalitarian Behavior Harvard University Press (1999).
Konrad, K. A. Strategy and Dynamics in Contests Oxford University Press (2009).
Archetti, M. & Scheuring, I. Coexistence of cooperation and defection in public goods games. Evolution 65, 1140–1148 (2011).
Baik, K. H. Contests with group-specific public-good prizes. Soc. Choice Welfare 30, 103–117 (2008).
Tullock, G. in Toward a Theory of the Rent-Seeking Society (eds Buchanan, J. M., Tollison, R. D. & Tullock, G.), 97–112 (Texas A & M University Press, 1980).
Glowacki, L. & Wrangham, R. W. The role of rewards in motivating participation in simple warfare. Hum. Nat. 24, 444–460 (2013).
Souza, M. O., Pacheco, J. M. & Santos, F. C. Evolution of cooperation under N-person snowdrift games. J. Theor. Biol. 260, 581–588 (2009).
Chapais, B. Primeval Kinship: How Pair-Bonding Gave Birth to Human Society Harvard University Press (2008).
Nonacs, P. & Hager, R. The past, present and future of reproductive skew theory and experiments. Biol. Rev. 86, 271–298 (2011).
Reeve, H. K. & Shen, S.-F. Unity and disunity in the search for a unified reproductive skew theory. Anim. Behav. 85, 1137–1144 (2013).
Gavrilets, S. Rapid transition towards the division of labor via evolution of developmental plasticity. PLoS Comput. Biol. 6, e1000805 (2010).
Barta, Z. & Giraldeau, L.-A. The effect of dominance hierarchy on the use of alternative foraging tactics: a phenotype-limited producing-scrounging game. Behav. Ecol. Sociobiol. 42, 217–223 (1998).
Mathot, K. J. & Giraldeau, L.-A. Within-group relatedness can lead to higher levels of exploitation: a model and empirical test. Behav. Ecol. 21, 843–850 (2010).
Boehm, C. Moral Origins: The Evolution of Virtue, Altruism, and Shame Basic Books (2012).
Gavrilets, S., Duenez-Guzman, E. A. & Vose, M. D. Dynamics of alliance formation and the egalitarian revolution. PLoS ONE 3, e3293 (2008).
Gavrilets, S. On the evolutionary origins of the egalitarian syndrome. Proc. Natl Acad. Sci. USA 109, 14069–14074 (2012).
King, A. J., Johnson, D. D. P. & Van Vugt, M. The origins and evolution of leadership. Curr. Biol. 19, R911–R916 (2009).
Hooper, P. L., Kaplan, H. S. & Boone, J. L. A theory of leadership in human cooperative groups. J. Theor. Biol. 265, 633–646 (2010).
Flack, J. C., Girvan, M., de Waal, F. B. M. & Krakauer, D. C. Policing stabilizes construction of social niches in primates. Nature 439, 426–429 (2006).
Sa¨a¨ksvuori, L., Mappes, T. & Puurtinen, M. Costly punishment prevails in intergroup conflict. Proc. R. Soc. Lond. B 278, 3428–3436 (2011).
Przepiorka, W. & Diekmann, A. Individual heterogeneity and costly punishment: a volunteer’s dilemma. Proc. R. Soc. Lond. B 280, 20130247 (2013).
Amsler, S. J. Energetic costs of territorial boundary patrols by wild chimpanzees. Am. J. Primatol. 72, 93–103 (2010).
Mares, R., Young, A. J., Levesque, D. L., Harrison, N. & Clutton-Brock, T. H. Responses to intruder scents in the cooperatively breeding meerkat: sex and social status differences and temporal variation. Behav. Ecol. 22, 594–600 (2011).
Beckerman, S. et al. Life histories, blood revenge, and reproductive success among the Waorani of Ecuador. Proc. Natl Acad. Sci. USA 106, 8134–8139 (2009).
Buzzell, E. & Preston, S. H. Mortality of American troops in the Iraq War. Popul. Dev. Rev. 33, 555–566 (2007).
Piff, P. K., Stancato, D. M., Cóte, S., Mendoza-Denton, R. & Keltner, D. Higher social class predicts increased unethical behavior. Proc. Natl Acad. Sci. USA 109, 4086–4091 (2012).
Tan, J. H. W. & Bolle, F. Team competition and the public goods game. Econ. Lett. 96, 133–139 (2007).
Burton-Chellew, M. N., Ross-Gillespie, A. & West, S. A. Cooperation in humans: competition between groups and proximate emotions. Evol. Hum. Behav. 31, 104–108 (2010).
Egas, M., Kats, R., van der Sar, X., Reuben, E. & Sabelis, M. W. Human cooperation by lethal group competition. Sci. Rep. 3, 1373 (2013).
Probst, T. M., Carnevale, P. J. & Triandis, H. C. Cultural values in intergroup and single-group social dilemmas. Organ. Behav. Hum. Decis. Process. 77, 171–191 (1999).
Simpson, B., Willer, R. & Ridgeway, C. L. Status hierarchies and the organization of collective action. Sociol. Theor. 30, 149–166 (2012).
Wilson, D. S. Hunting, sharing, and multilevel selection: the tolerated-theft model revisited. Curr. Anthropol. 39, 73–97 (1998).
Richerson, P. J. & Boyd, R. Not By Genes Alone: How Culture Transformed Human Evolution University of Chicago Press (2005).
Adams, J. S. Toward an understanding of inequity. J. Abnorm. Soc. Phsychol. 67, 422–436 (1963).
Price, M. E. Free riders as a blind spot of equity theory: an evolutionary correction. in Managerial Ethics: Managing the Psychology of Morality (ed. Schminke, M.), 235–256 (Routledge, 2010).
Defoe, D. A General History of the Pyrates London (1724).
Anderson, C. & Brown, C. E. The functions and dysfunctions of hierarchy. Res. Organ. Behav. 30, 55–89 (2010).
Halevy, N., Chou, E. Y. & Galinsky, A. D. A functional model of hierarchy: why, how, and when vertical differentiation enhances group performance. Organ. Psychol. Rev. 1, 32–52 (2011).
Geritz, S. A. H., Kisdi, É., Meszéna, G. & Metz, J. A. J. Evolutionarily singular strategies and the adaptive growth and branching of the evolutionary tree. Evol. Ecol. 12, 35–57 (1998).
Taylor, P. D., Irwin, A. J. & Day, T. Inclusive fitness in finite deme-structured and stepping-stone populations. Selection 1–3, 153–163 (2000).
Acknowledgements
We thank E. Akçay, J. Auerbach, S. Bowles, M.F. Grady, J.C. Mitani, J. Van Cleve, P.W. Wiessner, M.L. Wilson and the reviewers for comments and suggestions. This work was supported by the National Institute for Mathematical and Biological Synthesis through NSF Award #EF-0830858, with additional support from the University of Tennessee, Knoxville.
Author information
Authors and Affiliations
Contributions
S.G. and L.F. designed the study and wrote the paper, and S.G. performed the analyses.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures 1-6 (PDF 389 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/
About this article
Cite this article
Gavrilets, S., Fortunato, L. A solution to the collective action problem in between-group conflict with within-group inequality. Nat Commun 5, 3526 (2014). https://doi.org/10.1038/ncomms4526
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms4526
This article is cited by
-
Leader–follower behavioural coordination and neural synchronization during intergroup conflict
Nature Human Behaviour (2023)
-
Constraints on cooperation shape hierarchical versus distributed structure in human groups
Scientific Reports (2023)
-
Characterizing group and individual engagement in intergroup encounters between small groups of ring-tailed lemurs (Lemur catta) on St. Catherines Island, USA
Primates (2023)
-
The dynamics of cooperation, power, and inequality in a group-structured society
Scientific Reports (2021)
-
Human large-scale cooperation as a product of competition between cultural groups
Nature Communications (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.