Abstract
Antarctic ice cores document glacial-interglacial and millennial-scale variability in atmospheric pCO2 over the past 800 kyr. The ocean, as the largest active carbon reservoir on this timescale, is thought to have played a dominant role in these pCO2 fluctuations, but it remains unclear how and where in the ocean CO2 was stored during glaciations and released during (de)glacial millennial-scale climate events. The evolution of surface ocean pCO2 in key locations can therefore provide important clues for understanding the ocean’s role in Pleistocene carbon cycling. Here we present a 135-kyr record of shallow subsurface pCO2 and nutrient levels from the Norwegian Sea, an area of intense CO2 uptake from the atmosphere today. Our results suggest that the Norwegian Sea probably acted as a CO2 source towards the end of Heinrich stadials HS1, HS4 and HS11, and may have contributed to the increase in atmospheric pCO2 at these times.
Similar content being viewed by others
Introduction
The ongoing rise in atmospheric pCO2 and associated observations of reduced Arctic winter sea-ice coverage are projected to suppress the high-latitude North Atlantic ocean circulation over the coming decades, in turn affecting regional climate and the large-scale atmospheric circulation1,2. Regional reconstructions of past changes in surface ocean pCO2 and temperature are important for understanding how climate, ocean circulation and the carbon cycle are linked. Greenland and Antarctic ice core records document a millennial-scale bipolar seesaw in air temperature changes during late Pleistocene glaciations and deglaciations3. Warm interstadial conditions over Greenland coincided with periods of gradual cooling over Antarctica, whereas cold stadial periods in Greenland coincided with warming over Antarctica3. In Greenland ice cores, these millennial-scale events have been termed Dansgaard–Oeschger events and are characterized by abrupt warming during the transitions to interstadials4. In contrast, Antarctic ice cores report only gradual climate changes3. The longest stadials include Heinrich events, and are called Heinrich Stadials (HS) (ref. 5). These interhemispheric climate patterns may be explained by variations in the Atlantic Meridional Overturning Circulation and associated changes in the northward heat export6.
Atmospheric pCO2 was ∼80–100 μatm lower during glacials compared with interglacial periods7. During the last deglaciation (∼20–10 ka), atmospheric pCO2 increased in two pronounced steps, by ∼50 μatm during HS1 (∼18–14.5 ka) and by another ∼30 μatm during the Younger Dryas (∼13–11.5 ka) (ref. 8). The last glacial period was furthermore characterized by millennial-scale variability in atmospheric pCO2, with an increase of roughly 25 μatm beginning during most of the Heinrich stadials, and peaking at or less than a thousand years after the onset of the interstadials9. Thereafter, pCO2 decreased gradually in phase with cooling in Antarctica9.
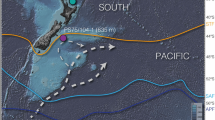
The high-latitude North Atlantic, north of 50°N, is one of the most efficient CO2 uptake areas in the modern ocean, because of cold sea surface temperatures, deep-water formation, strong primary productivity and high-wind speeds10,11,12. Therefore, it is an important region to study glacial-interglacial and millennial-scale variations in air-sea CO2 exchange. This study aims to quantify the evolution of shallow subsurface ocean carbonate chemistry in the Norwegian Sea over the past 135 kyr, using the boron isotopic composition (δ11B) recorded in fossil shells of the polar planktic foraminifer Neogloboquadrina pachyderma. To constrain nutrient utilization, a primary control on the pCO2 in the surface ocean, we also analysed Cd/Ca and δ13C in N. pachyderma. The study is based on sediment core JM11-FI-19PC retrieved from 1,179 m water depth in the Faroe-Shetland Channel (Fig. 1), in the main pathway of the exchange of surface and deep water masses between the Nordic Seas and eastern North Atlantic13 (Fig. 2a). Our results suggest that the Norwegian Sea remained a CO2 sink during most of the past 135 kyr, but during the latest parts of HS1, HS4 and HS11 the area acted as a source of CO2 to the atmosphere. To elucidate the causes of these variations in seawater carbonate chemistry, we compare our results with previously published reconstructions of temperature14,15, sea-ice cover, input of terrestrial organic matter and primary productivity16.
Oceanic CO2 sinks and sources are presented by negative and positive ΔpCO2sea-air values, respectively, and identify the high-latitude North Atlantic as a significant CO2 sink. The white star shows the location of the studied sediment core JM-FI-19PC. Map was generated using Ocean Data View66 based on modern data of Takahashi et al.10
(a) Map showing the major surface and bottom water currents in the northern North Atlantic and the Nordic Seas13. Figure modified after Ezat et al.14. The white star and circle indicate the location of sediment core JM11-FI-19PC (used in this study) and sediment cores studied in refs 32, 37, respectively. (b,c) pCO2-depth profiles from the Norwegian and Greenland Seas, respectively, calculated from hydrographic carbonate chemistry and nutrient data collected during 2002–2003 (ref. 21). Note that we chose only data collected during the growth seasons of N. pachyderma. The white and orange rectangles in (a) refer to the locations for the hydrographic sites used to construct the pCO2-depth profiles in (b,c), respectively. The exact locations of the hydrographic sites are shown in Supplementary Fig. 4. The purple vertical arrow on the y-axes in (b,c) refer to the average atmospheric pCO2 during 2002–2003.
Results
Geochemical proxies of ocean pCO2 and nutrient changes
Because the speciation and isotopic composition of dissolved boron in seawater depends on seawater pH, and borate ion is the dominant species incorporated into planktic foraminiferal shells, their recorded δ11B serves as a pH-proxy17, and paleo-pH can be quantified if temperature and salinity can be constrained independently (see Methods for details). When pH is paired with a second parameter of the carbon system, aqueous pCO2 can be estimated. Here we applied foraminiferal δ18O and Mg/Ca measurements to estimate foraminiferal calcification temperature and salinity, and then used the modern local relationship between salinity and total alkalinity to estimate coeval changes in total alkalinity (see Methods for details). Finally, we calculated the difference between our reconstructed shallow subsurface pCO2 and atmospheric pCO2 from ice core measurements18.
The ΔpCO2sea-air is a measure for the tendency of a water mass to absorb/release CO2 from/to the atmosphere10. However, because N. pachyderma lives below the sea surface, this difference represents the difference between atmospheric pCO2 (‘air’) and the seawater pCO2 (‘pCO2cal’) at the calcification depth and growth season of N. pachyderma (ΔpCO2cal-air). Neogloboquadrina pachyderma is thought to inhabit a wide and variable range of calcification depths in the Nordic Seas from 40 to 250 m water depth19. It migrates vertically in the water column19 and is most abundant during late spring to early autumn20. To assess the influence of the seasonal occurrence and calcification depth of N. pachyderma on our results, we calculated pCO2-depth profiles for the upper 250 m of the water column in the Norwegian Sea based on modern hydrographic data (total dissolved inorganic carbon, total alkalinity, temperature, salinity, phosphate and silicate) covering the late spring to early autumn21 (Fig. 2b). The resulting modern pCO2-profile (Fig. 2b) shows that the average pCO2 of the surface ocean (0–25 m water depth) is 30–50 μatm lower than atmospheric pCO2, but at the calcification depth of N. pachyderma (∼≥50 m water depth) average aqueous pCO2 is approximately equal to atmospheric pCO2. We thus calculated the difference in pCO2 between the surface ocean and the atmosphere (ΔpCO2sea-air) by subtracting 40 μatm from ΔpCO2cal-air, assuming that the pCO2 gradient between the surface ocean and calcification depth of N. pachyderma remained constant through time (see ‘Discussion’).
To characterize the changes in availability and utilization of nutrients, we measured Cd/Ca and δ13C in N. pachyderma. The Cd/Ca recorded by symbiont-barren planktic foraminifera such as N. pachyderma is sensitive to Cd concentrations in seawater22, an element that shows strong similarity to the seawater distribution of the nutrient phosphate23. Thus, foraminiferal Cd/Ca can be used to reconstruct the levels of phosphate in seawater, and provides clues for the abundance and utilization of phosphate through time24, albeit with a potential side control of temperature on the Cd incorporation into planktic foraminiferal shells25. In addition, planktic foraminiferal δ13C responds to changes in nutrient cycling, air-sea gas exchange, exchange between global carbon reservoirs26 and carbonate chemistry27.
Seawater pH and pCO2
The studied sediment core JM-FI-19PC spans the last 135 kyr (refs 14, 15, 16) and has been correlated closely to the age model of the Greenland ice core NGRIP (ref. 28) (see Methods and Supplementary Fig. 1). The δ11B record displays ∼1.5‰ higher glacial values compared with interglacials and the core top samples. In addition, negative δ11B excursions of up to −1.5‰ occurred during HS1 and HS4 (Fig. 3a). Correspondingly, glacial pH was elevated by ∼0.16 units in the shallow subsurface compared with the Holocene, similar to results from earlier studies of tropical regions29,30, but the record is punctuated by brief episodes of acidification during some Heinrich stadials (Fig. 3b). The reconstructed shallow subsurface pCO2 shows lowest values of ∼200 μatm during the Last Glacial Maximum (LGM) (∼24–19 ka), whereas it increased to 320 μatm during HS1 at ∼16.5 ka, and then gradually dropped to ∼230 μatm over the Bølling-Allerød interstadials (14.7–12.7 ka) (Fig. 3c). The ΔpCO2cal-air increased from ∼+5 μatm during the LGM to ∼+100 μatm during HS1 (at ∼16.5 ka) and gradually decreased towards the Bølling–Allerød (BA) interstadial (Fig. 3e). Because of the analytical effort required for boron isotope measurements, and inadequate sample sizes for high-resolution boron analyses in some Heinrich stadials, we chose to focus on HS4 (∼40–38 ka) as representative for the last glacial Heinrich stadials, because of the high sedimentation rate and good age control14 on this interval in our record. The shallow subsurface pCO2 increased from ∼220 μatm during interstadial 9 (∼40 ka) to ∼285 μatm during HS4 and then gradually decreased to ∼225 μatm during interstadial 8 (∼37.5 ka) (Fig. 3c). Similar to the late part of HS1 (∼16.5 ka) during Termination I, a prominent increase in the ΔpCO2cal-air (∼+100 μatm) is also seen during the late part of HS11 (at ∼133 ka) in Termination II (Fig. 3e). A Holocene-like shallow subsurface pCO2 is observed during the early and late Eemian interglacial (at ∼129 and at 116 ka, respectively), but shallow subsurface pCO2 was ∼30 μatm lower during the mid Eemian (125–122 ka) (Fig. 3c).
(a) δ11B measured in N. pachyderma with analytical uncertainty. (b) seawater-pH inferred from δ11B. (c) estimated seawater pCO2 at the calcification depth and growth season of N. pachyderma. The envelope reflects the uncertainty boundaries based on the propagated error of the individual uncertainties in the parameters used to calculate pCO2. (d) Atmospheric pCO2 from Antarctic ice cores18. (e) the difference between reconstructed shallow subsurface pCO2 at our site and atmospheric pCO2 (ΔpCO2cal-air). (f) ΔpCO2sea-air calculated as ΔpCO2cal-air minus the modern pCO2 gradient between the calcification depth of N. pachyderma (40–200 m water depth) and surface ocean (0–30 m water depth). The green circle indicates present day average ΔpCO2sea-air in the Norwegian Sea21. (g) Cd/Ca measured in N. pachyderma. (h) δ13C measured in N. pachyderma.
Cd/Ca and δ13C
The δ13C record shows minimum values (∼−0.4‰) during the Heinrich stadials HS1, HS3 and HS6, and ∼−0.1‰ during HS11, HS4 and some non-Heinrich stadials (Fig. 3h). The highest Cd/Ca values are recorded during HS1, HS11 (∼0.007 μmol mol−1), HS3, Younger Dryas (∼0.004 μmol mol−1) and HS4 (∼0.0025 μmol mol−1) (Fig. 3g). Although, the calcification temperature is found to have a secondary effect on the Cd incorporation into planktic foraminifera shells25, the absence of a correlation between our raw Mg/Ca values, a temperature proxy, and Cd/Ca data (R2=0.0001; Supplementary Fig. 2) supports the interpretation of the recorded Cd/Ca variability as changes in nutrient levels. However, it is notable that our Cd/Ca results show absolute values that are an order of magnitude lower than previous studies from the region31,32. We re-examined our Cd/Ca analyses closely and could not find any indication of analytical errors. The low Cd/Ca values can also not be attributed to the application of the intensive ‘full cleaning’ procedure to clean our foraminiferal samples before minor/trace element analyses (see Methods). Five duplicate samples of N. pachyderma cleaned with the standard cleaning protocol used in Cd/Ca studies yielded the same low Cd/Ca values (see Methods). Despite the low absolute values, our Cd/Ca data show strong consistency and agreement with the variations in δ13C values (Fig. 3g,h). In addition, our Cd/Ca trends are similar to previous studies, for example, similar Cd/Ca for both the Holocene and the LGM are obtained as in Keigwin and Boyle31 (Fig. 3g,h). As we cannot find the reason for the significantly lowered absolute values of our Cd/Ca, we refrain from quantifying the phosphate concentrations using Cd/Ca. Instead, we interpret their variations qualitatively to support the evidence from foraminiferal δ13C (Fig. 3) and other export productivity proxy-data (the concentration of phytoplankton-induced sterols) obtained from the same core and published in Hoff et al.16 (Fig. 4) (see Discussion).
(a) ΔpCO2sea-air. (b) Cd/Ca measured in N. pachyderma. (c) δ13C measured in N. pachyderma. (d) δ13C measured in organic matter (δ13Corg) (ref. 16). (e) concentration of brassicasterol16. (f) concentration of dinosterol16. (g) C25 isoprenoid lipid (IP25) (ref. 16). High concentration of IP25 suggests presence of seasonal sea ice, whereas absence of IP25 suggests either permanent sea-ice cover (when the concentration of sterols is low) or open ocean conditions (when the concentration of sterols is high) (see Hoff et al.16 for details). Note the break in the y-axes of plots e–g. (h) shallow subsurface (black) and bottom water (grey) temperature14,15. Bottom water temperatures are based on Mg/Ca in the benthic foraminiferal species Melonis barleeanus (triangles) and Cassidulina neoteretis (squares). Shallow subsurface temperatures are based on Mg/Ca in N. pachyderma. (i) North Greenland Ice Core Project (NGRIP) ice core δ18O values28,67. Red stars on the x-axis indicate tephra layers that are common to sediment core JM11-FI-19PC and Greenland ice cores (Supplementary Fig. 1).
Collectively, the δ13C and Cd/Ca records indicate an increase in the nutrient content during the Heinrich stadials studied herein. There is a ∼0.5‰ decrease in δ13C during the LGM and the Eemian compared with the Holocene (Fig. 3h), while the Cd/Ca values remain almost the same (Fig. 3g). The ∼0.5‰ lower δ13C values during the LGM with almost no concomitant change in Cd/Ca may be due to the transfer of isotopically light terrestrial carbon31, and elevated [CO32−] at the higher pH characteristic for the LGM (Fig. 3b). Elevated pH (and/or [CO32−]) has been observed to lower the δ13C recorded by planktic foraminifera relative to seawater δ13CDIC, but the sensitivity is species-specific and N. pachyderma has not yet been examined in this regard27. Compared with the Holocene, the lower δ13C values are likely due to a smaller air-sea gas exchange in response to the higher temperatures during the Eemian relative to the Holocene33 (0.1‰ decrease in δ13C per 1 °C increase; ref. 34) (see Discussion below).
Discussion
The most striking observation from these data is the large increase in ΔpCO2cal-air by +80 to +100 μatm during the final stages of HS1, HS4 and HS11. In the modern Norwegian Sea, the average pCO2 at the calcification depth of N. pachyderma is ∼40 μatm lower than in the surface ocean, where the CO2 exchange with the atmosphere actually occurs (Fig. 2b). If the paleo-pCO2 gradient between the calcification depth of N. pachyderma and the surface ocean was similar to the modern ocean (∼40 μatm), the re-calculated ΔpCO2sea-air values of +40 to +60 μatm during HS1, HS4 and HS11 (Fig. 3f) suggest that the Norwegian Sea, and perhaps the Nordic Seas in general, acted as a CO2 source during these intervals. This is very different from the modern ocean, where the core site region is characterized by intense CO2 uptake from the atmosphere (Figs 1 and 2b).
In contrast, the negative ΔpCO2sea-air (=∼−35 μatm) during the LGM and BA interstadial could be interpreted as enhanced CO2 uptake, similar to the Holocene (Fig. 3f). However, the lower aqueous pCO2 values during the mid Eemian relative to the Holocene are more likely explained by a decrease in the CO2 solubility because of increased sea surface temperatures. Mg/Ca temperature estimates in core JM11-FI-19PC indicate a 2 °C warming at the calcification depth of N. pachyderma15, but faunal assemblages, which may reflect temperatures in the mixed layer, where CO2 is exchanged, suggest an even greater warming up to ∼4 °C compared with the present33.
In the discussion above, we assumed that the pCO2 gradient between the calcification depth of N. pachyderma and the surface ocean (∼40 μatm) remained constant through time. We cannot provide evidence for past changes in this gradient; however, the modern spatial variability of this pCO2 gradient in the Nordic Seas combined with inferred past changes in ocean circulation can provide some insights. Importantly, previous studies from the Nordic Seas based on planktic foraminiferal assemblages35 and sea-ice proxies (IP25 and phytoplankton-based sterols) (ref. 16) suggest that the polar front moved towards our study area during cold stadial periods. A modern pCO2-depth profile from the polar frontal zone in the Greenland Sea21 (Fig. 2c) shows that the pCO2 gradient between the surface ocean and the calcification depth of N. pachyderma (=∼20 μatm on average) (as well as the upper water column pCO2 in general) is smaller at the polar front than in the Norwegian Sea (Fig. 2b,c). This pattern argues against the possibility that a larger than modern pCO2 gradient existed between the surface ocean and the calcification depth of N. pachyderma during Heinrich stadials. Our recalculated ΔpCO2sea-air (Fig. 3f) may therefore actually represent a minimum estimate of the ΔpCO2sea-air during these time intervals. It is notable that earlier findings by Yu et al.32 using evidence from B/Ca and a low-resolution δ11BN. pachyderma record from the Iceland Basin, suggested that the high-latitude North Atlantic region remained a CO2 sink throughout the last deglaciation. This result contrasts with our δ11B record despite the fact that our B/Ca record looks very similar to the B/Ca record of Yu et al.32 (Supplementary Fig. 3). However, because Pleistocene planktic B/Ca records typically display large variability that rarely relates to oceanic pH variations36, we suggest that the δ11B proxy is a more reliable pH proxy. The δ11B proxy has been validated against ice core CO2 data and consistent variations in δ11B have been reconstructed between different core sites, where CO2 is in equilibrium with the atmosphere29,30. Furthermore, the earlier δ11B study32 does not extend beyond HS1 and may therefore fail to capture the full glacial/interglacial variability (Supplementary Fig. 3). Nevertheless, because we reconstruct air-sea disequilibrium conditions, which may be spatially variable, the discrepancy between these two δ11B records across HS1 (Supplementary Fig. 3) warrants additional research to further explore the spatial extent of the high-latitude North Atlantic pCO2 source during Heinrich Stadials.
The increase in ΔpCO2sea-air during HS1, HS4 and HS11 in the Norwegian Sea could be the result of the following scenarios: (1) mixing with or surfacing of older water masses with accumulated CO2, (2) changes in primary productivity and nutrient concentrations, (3) increased rate of sea ice formation, (4) enriched CO2 content of the inflowing Atlantic water (that is, changes in the pCO2 of the source water at lower latitudes) and/or (5) slowdown of deep-water formation.
Concerning scenario (1), shallow subsurface radiocarbon reconstructions from the high-latitude North Atlantic37,38,39 display a prominent decrease in reservoir ages (that is, better ventilated ‘young’ water) at 16.5 ka, when our record shows an increase in pCO2. This comparison eliminates mixing with an aged, CO2-rich water mass as an explanation for our ΔpCO2sea-air record. For scenario (2), the increased ΔpCO2sea-air during HS1, HS4 and HS11 coincides with low δ13C and high Cd/Ca values, so we interpret our observations as a decrease in nutrient utilization and primary production at the sea surface. A decrease in primary productivity would reduce nutrients and CO2 utilization (that is, high Cd/Ca and high pCO2), and δ13CDIC would not be elevated by preferential photosynthetic removal of 12C (that is, low foraminiferal δ13C). A decrease in the concentration of phytoplankton-induced sterols during HS4 and to some extent during HS1 (ref. 16) support the scenario of diminished primary productivity (Fig. 4). The increase in seawater pCO2 and nutrients might also be caused by enhanced transfer of terrestrial carbon during Heinrich events and subsequent release via respiration. Hoff et al.16 recorded a relative decrease in δ13Corg during HS1 and HS4 (Fig. 4d), which may reflect a combination of both decreased primary productivity (that is, decrease in the relative proportion of marine organic matter) and increased proportion of terrigenous organic matter40.
Regarding scenario (3), studies from the modern East Greenland current region show that total dissolved inorganic carbon is rejected more efficiently than total alkalinity during sea-ice formation, causing the brines beneath the sea ice to be enriched in CO2 compared with normal seawater11. Furthermore, modern observations from the coastal Arctic zone show substantial seasonal variations in surface ocean pCO2 because of formation and melting of sea ice; with positive ΔpCO2sea-air during spring and negative ΔpCO2sea-air during the summer attributed to complex biogeochemical processes41. Because of the increased extent of sea ice during Heinrich stadials at our site16 (Fig. 4e–g), the effect of sea ice growth/decay may have exerted a longer-term and larger-scale influence on the surface ocean pCO2 in the Arctic Ocean and Nordic Seas. For scenario (4), reconstructions from the Nordic Seas of stadial ocean circulation patterns indicate a subsurface incursion of warm Atlantic water into the Nordic Seas below a well-developed halocline14,42. Thus, we cannot rule out that some of the pCO2 increase has occurred in the source water somewhere at lower latitudes. In addition, the increase in the subsurface temperature14,42 (Fig. 4h) may have enhanced the degradation of organic matter. Last, for scenario (5), a slow-down or cessation of deep-water formation in the Nordic Seas14,35,42 may have promoted the pCO2 increase in the shallow subsurface depth via slowing down of the carbon transfer from the sea surface to the ocean interior.
As illustrated above, several processes may have contributed to the pCO2 increase during HS1, HS4 and HS11 including decreased primary productivity, increased input of terrestrial organic matter, high rate of sea ice formation and suppressed deep water formation. Conversely, during the interstadials studied herein (interstadial 8 and the BA interstadial) increased primary productivity, decreased input of terrestrial organic carbon, melting of sea ice16 (Fig. 4) and enhanced deep water formation14,35, resulted in the consumption and/or dilution of the CO2 content. Heinrich stadials 3 and 6 are at least partially resolved in this study, but do not show similar changes in seawater carbonate chemistry as HS1, HS4 and HS11. It is notable, however, that nutrients, export productivity and sea-ice proxies suggest similar changes for all resolved Heinrich stadials (Fig. 4). We have measured δ11B only for the early part of HS3 (for example, no measurements at the Cd/Ca peak), which shows a tendency towards decreasing values similar to other Heinrich stadials (Fig. 3a). During HS6, our δ11B record displays an increase (that is, decrease in aqueous pCO2) based on one data point (Fig. 3a). One additional difference that characterizes HS6 is the increase in δ13Corg, which suggests a relative decrease in the input of terrestrial organic matter during this event compared with other Heinrich stadials (Fig. 4). Nevertheless, higher resolution δ11B records are required to assess the carbonate chemistry evolution across HS3 and HS6.
How was the oceanic CO2 released to the atmosphere during HS1, HS4 and HS11 in the Norwegian Sea? The presence of thick perennial or near-perennial sea ice cover during these times16 may have acted as a barrier for oceanic CO2 outgassing. Earlier studies have suggested that a gradual build-up of a heat reservoir occurred during stadial periods because of subsurface inflow of warm Atlantic water to the Nordic Seas14,35,42 (Fig. 4h). Surfacing of this warm water, evidenced by a large decrease in bottom water temperature14 (Fig. 4h), occurred during the rapid transition to interstadial periods14,42. We therefore suggest that the CO2 was released to the atmosphere, along with the advection of subsurface heat, at the terminations of the Heinrich stadials. The increases in surface pCO2 in the Nordic Seas may thus have contributed to the rapid increase in atmospheric pCO2 (∼10 μatm) that occurred at the terminations of some Heinrich stadials9,43,44.
In summary, we show significant changes in the marine carbon system in the Norwegian Sea associated with well-known regional climatic anomalies during the last 135 kyr. Our data indicate that the Norwegian Sea, and possibly the broader Nordic Seas, was an area for intense CO2 uptake from the atmosphere during the LGM and the interstadials investigated in this study (that is, interstadials 8 and Bølling-Allerød), similar to modern conditions, whereas it may have acted as a CO2 source during the ends of HS1, HS4 and HS11. Our shallow subsurface pCO2 record presents the first indication that changes in primary productivity and ocean circulation in the Nordic Seas may have played a role in the late Pleistocene variations in atmospheric pCO2.
Methods
Age model
The logging, scanning and sampling of the sediment core (JM-FI-19PC) are described in Ezat et al.14 The sediment core JM-FI-19PC is aligned to the Greenland ice core NGRIP based on the identification of common tephra layers and by tuning increases in magnetic susceptibility and/or increases in benthic foraminiferal δ18O values to the onset of DO interstadials in the Greenland ice cores14,15,16 (Supplementary Fig. 1). In support of the reconstructed age model, eleven calibrated radiocarbon dates measured in N. pachyderma (with no attempt to correct for past changes in near-surface reservoir ages) show strong consistency with the tuned age model for the past 50 kyr (ref. 14).
Boron isotope and minor/trace element analyses
Only pristine N. pachyderma specimens with no visible signs of dissolution were picked from the 150 to 250 μm size fractions for boron isotope (200–450 specimens) and minor/trace element (70–160 specimens) analyses. For boron isotope measurements, the foraminifer shells were gently crushed, and cleaned following Barker et al.45 This cleaning protocol includes clay removal, oxidative and weak acid leaching steps. Thereafter, the samples were dried and weighed to determine the amount of acid required for dissolution. Immediately before loading, samples were dissolved in ultrapure 2N HCl, and then centrifuged to separate out any insoluble mineral grains. One μl of boron-free seawater followed by an aliquot of sample solution (containing 1–1.5 ng B per aliquot) were loaded onto outgassed Rhenium filaments (zone refined), then slowly evaporated at an ion current of 0.5A and finally mounted into the mass spectrometer. Depending on sample size, five to ten replicates were loaded per sample. Boron isotopes were measured as BO2- ions on masses 43 and 42 using a Thermo Triton thermal ionization mass spectrometer at the Lamont-Doherty Earth Observatory (LDEO) of Columbia University. Each sample aliquot was heated up slowly to 1,000±20 °C and then 320 boron isotope ratios were acquired over ∼40 min46. Boron isotope ratios are reported relative to the boron isotopic composition of SRM 951 boric acid standard, where δ11B (‰)=(43/42sample/43/42standard−1) × 1,000. Analyses that fractionated >1‰ over the data acquisition time were discarded. The analysis of multiple replicates allows us to minimize analytical uncertainty, which is reported as 2s.e.=2s.d./✓n, where n is the number of sample aliquots analysed. The analytical uncertainty in δ11B of each sample was then compared with the long-term reproducibility of an in-house vaterite standard (±0.34‰ for n=3 to ±0.19‰ for n=10) and the larger of the two uncertainties is reported (Supplementary Table 1). Two samples were repeated using the oxidative-reductive cleaning procedure from Pena et al.47 and yielded indistinguishable δ11B values (Supplementary Table 1).
Trace and minor element analytical procedures followed cleaning after Martin and Lea48 and included clay removal, reductive, oxidative, alkaline chelation (with DTPA solution) and weak acid leaching steps with slight modifications15 from Pena et al.47 and Lea and Boyle49. These modifications included rinsing samples with NH4OH (ref. 49) instead of using 0.01 N NaOH (ref. 48) as a first step to remove the DTPA solution, followed by rinsing the samples three times with cold (room temperature) MilliQ water, 5-min immersion in hot (∼80 °C) MilliQ water and two more rinses with cold MilliQ water47. After cleaning, the samples were dissolved in 2% HNO3 and finally analysed by iCAPQ Inductively-Coupled Plasma Mass Spectrometry at LDEO. Based on repeated measurements of in-house standard solutions, the long-term precision is <1.4, 1.9 and 2.1% for Mg/Ca, B/Ca and Cd/Ca, respectively. Five samples were split after clay removal, reduction and oxidation steps; one half was cleaned by the full cleaning procedure, while the alkaline chelation step was omitted for the other half. This approach was applied to test the influence of the chelation step on Cd/Ca and B/Ca. The results with and without the alkaline chelation show an average difference of 0.0003 μmol mol−1 and 5 μmol mol−1 for Cd/Ca and B/Ca, respectively (Supplementary Table 2). The Mg/Ca values from the two cleaning methods are comparable, but two samples showed a significant decrease in Mg/Ca, Fe/Ca, Mn/Ca and Al/Ca values when the alkaline chelation step was applied (Supplementary Table 2). This might be due to a more efficient removal of contaminants that are rich in Mg, but not in Cd or B. All our Mn/Ca values from the full cleaning method are <105 μmol mol−1, indicating that our results are unlikely affected by diagenetic coatings50. Only minor/trace element results from the full cleaning method were used in this study. All cleaning and loading steps for boron isotope and minor/trace element analyses were done in boron-free filtered laminar flow benches and all used boron-free Milli-Q water.
Stable isotope analyses
Pristine specimens of the benthic foraminifera Melonis barleeanus (∼30 specimens, size fraction 150–315 μm) and the planktic foraminifera N. pachyderma (∼50 specimens, size fraction 150–250 μm) were picked for stable isotope analyses. The stable oxygen and carbon isotope analyses were performed using a Finnigan MAT 251 mass spectrometer with an automated carbonate preparation device at MARUM, University of Bremen. The external standard errors for the oxygen and carbon isotope analyses are ±0.07‰ and ±0.05‰, respectively. Values are reported relative to the Vienna Pee Dee Belemnite (VPDB), calibrated by using the National Bureau of Standards (NBS) 18, 19 and 20. The oxygen isotope data were previously presented14,15,16, while the carbon isotope results are presented here for the first time (Supplementary Data 1).
Salinity and temperature reconstructions
We used the calcification temperature and δ18OSW values from Ezat et al.15 based on parallel δ18O and Mg/Ca measurements in N. pachyderma (Supplementary Data 1). Previous studies suggested that carbonate chemistry may exert a significant secondary effect on Mg/Ca in N. pachyderma20. The possible influence of secondary factors on temperature reconstructions are discussed in detail in Ezat et al.15 In brief, the main effect of the secondary factors appears to be the elevated pH and carbonate ion concentration during the LGM; a correction for this effect may lower the temperatures by 0–2 °C. However, the exact effect remains uncertain15. Here we used the temperature and δ18OSW reconstructions with no correction for non-temperature factors on Mg/Ca (see section ‘Propagation of error’ below).
In the absence of a direct proxy for salinity, we estimated the salinity from our reconstructed δ18OSW. There is a quasi-linear regional relationship between salinity and δ18OSW in the modern ocean, as both parameters co-vary because of addition/removal of freshwater51. However, temporal changes in the δ18OSW composition of freshwater sources and/or their relative contribution to a specific region, as well as changes in ocean circulation complicate using a local modern δ18OSW-salinity relationship to infer past changes in salinity. We therefore estimate salinity using the δ18OSW-salinity mixing line from the Norwegian Sea51 for the Holocene and the Eemian, when the hydrological cycle and ocean circulation were likely similar to modern. For the deglacial and last glacial periods, we use the δ18OSW-salinity mixing line52 based on data from the Kangerdlugssuaq Fjord, East Greenland, where the dominant source of freshwater is glacial meltwater from tidewater glaciers with δ18OSW values ranging from −30 to −20‰. These conditions are probably more representative of the sources of glacial meltwater during deglacial and glacial times53. Our salinity estimates during the deglacial and last glacial periods would have been ∼1.5‰ lower if we had used the modern δ18OSW-salinity mixing line from the Norwegian Sea. Although this salinity difference may appear large, it has little consequence for our pH and pCO2 reconstructions and our conclusions (see ‘Sensitivity tests’ below).
pH and pCO2 estimations
The boron isotopic composition of biogenic carbonate is sensitive to seawater-pH (ref. 17), because the relative abundance and isotopic composition of the two dominant dissolved boron species in seawater, boric acid [B(OH)3] and borate [B(OH)4−] changes with pH (ref. 54), and borate is the species predominantly incorporated into marine carbonates. Culture experiments with planktic foraminifera provide empirical support for using their boron isotopic composition as a pH proxy30,55,56, but species-specific δ11B offsets are also observed, which are widely ascribed to ‘vital effects’57.
Linear regressions of δ11BCaCO3 versus δ11Bborate relationships allow to infer δ11Bborate from δ11BCaCO3 (ref. 30) as follows:

where ‘c’ is the intercept and ‘m’ is the slope of the regression. pH can then be estimated from foraminiferal δ11B-based δ11Bborate using the following equation17:

where pKB is the equilibrium constant for the dissociation of boric acid for a given temperature and salinity58, δ11BSW is the δ11B of seawater (modern δ11BSW=39.61‰; ref. 59), and α(B3-B4) is the fractionation factor for aqueous boron isotope exchange between boric acid and borate. Klochko et al.54 determined the boron isotope fractionation factor in seawater α(B3-B4)= 1.0272±0.0006.
Because δ11B in the symbiont-barren N. pachyderma has so far only been calibrated from core top sediments, with large uncertainties and over a very limited natural pH range32, the pH sensitivity of this species is uncertain. However, we can use evidence from other calibrated symbiont-barren planktic foraminifera species to further constrain the pH sensitivity of this species. Martínez-Botí et al.60 suggested a pH sensitivity for the symbiont-barren planktic foraminifera G. bulloides similar to values predicted from aqueous boron isotope fractionation (that is, slope m in eq. (1) =1.074). We therefore used a slope value of 1.074 in equation (1). In addition, we calculated the intercept c=2.053‰ in equation (1) for N . pachyderma by calibrating our core top foraminiferal δ11B to a calculated pre-industrial pH (that is, δ11Bborate). Pre-industrial pH was estimated from modern hydrographic carbonate data (total Dissolved Inorganic Carbon ‘DIC’, total alkalinity, phosphate, silicate, temperature, salinity; ref. 21) from the southern Norwegian Sea (Fig. 2a, Supplementary Fig. 4), and subtracting 50 μmol kg−1 from DIC (ref. 61) to correct for the anthropogenic CO2 effect. We used the hydrographic data collected during June 2002 and from the 22nd of September to the 13th of October 2003 (that is, within the assumed calcification season of N. pachyderma; refs 19, 20) and at our assumed calcification depth (that is, 40–120 m). This approach allows us to determine δ11Bborate from δ11BCaCO3 (equation 1), which can then be used to calculate pH based on equation 2.
Although the slope determined for G. bulloides60 is similar to the coretop calibration of N. pachyderma32, neither calibration encompasses a wide pH range, and the uncertainty of the slopes is therefore large. In contrast, laboratory culture experiments with (symbiont-bearing) planktic foraminifera cover a much wider pH-range but display a lesser pH sensitivity (slope in equation (1)=∼0.7) than predicted from aqueous boron isotope fractionation30,55,56. However, this difference in slope has little consequence for our pH and pCO2 reconstructions. A sensitivity test using slopes m=1.074 (ref. 60) and m=0.7 (refs 30, 55, 56) shows little difference between the two estimates (see section ‘Sensitivity tests’ below).
If two of the six carbonate parameters (total Dissolved Inorganic Carbon (DIC), total alkalinity, carbonate ion concentration, bicarbonate ion concentration, pH and CO2), are known in addition to temperature, pressure and salinity, the other parameters can be calculated62. We used the modern local salinity-total alkalinity relationship (Alkalinity=69.127 × Salinity−116.42, R2=0.76, ref. 21) to estimate total alkalinity. Because weathering processes are slow and alkalinity is relatively high in the ocean, alkalinity can be considered a quasi-conservative tracer on these time scales, and we do not consider potential past changes in the salinity-total alkalinity relationship. Nonetheless, if we use the modern alkalinity-salinity relationship from the polar region as a possible analogue for our area during the last glacial, this would decrease the error in total alkalinity (because of the uncertainty in salinity) by up to 65 μmol kg−1 (Supplementary Fig. 5). Aqueous pCO2 is then calculated using CO2sys.xls (ref. 63), with the equilibrium constants K1 and K2 from Millero et al.64, KSO4 is from Dickson59 and the seawater boron concentration from Lee et al.65
Sensitivity tests of pCO2 reconstructions
Supplementary Fig. 6 shows that pH and pCO2 reconstructions based on very different temperature, salinity and total alkalinity scenarios are very similar and do not significantly affect the large pCO2 increases during HS1, HS4 and HS11. Because the intercept ‘c’ in the \({\rm \delta }^{{\rm 11}} {\rm B}_{{\rm CaCO}_{\rm 3} } \) versus δ11Bborate calibrations (see Methods) is dependent on our choice of calcification depth for N. pachyderma, and corresponding selection of depths of hydrographic data to calculate the pre-industrial pH (after removing the anthropogenic carbon effect), we alternatively calculated the pre-industrial pH and the intercept ‘c’ based on hydrographic data from both 50 and 200 m water depths. This sensitivity test shows that the uncertainty in the calcification depth of N. pachyderma has insignificant effect on the amplitude of our down core pCO2 variations (Supplementary Fig. 7).
In addition, to assess the uncertainty in our pH and pCO2 estimations because of the uncertainty in the \({\rm \delta }^{{\rm 11}} {\rm B}_{{\rm CaCO}_{\rm 3} } \) versus pH sensitivity in N. pachyderma, we recalculated the δ11Bborate using slope value of m=0.7 instead of m=1.074 in equation (1) as suggested for some symbiont-bearing planktic foraminifera species30,55,56, and re-adjusted the intercept ‘c’ accordingly (=−4.2‰). This test shows that the uncertainty in species-specific pH-sensitivity has no effect on our pCO2 reconstructions for the Heinrich stadial events, while the main difference is an increase in the glacial/interglacial pCO2 by ∼30 μatm, when a slope value of m=0.7 is used (Supplementary Fig. 8). This brings ΔpCO2cal-air for the LGM to values of −30 μatm (and ΔpCO2sea-air=−70 μatm), strengthening our conclusion about enhanced oceanic CO2 uptake in our area during the LGM.
Finally, because our ΔpCO2cal-air record can be biased because of errors in the age model especially for the Heinrich stadials (times with increasing atmospheric pCO2), we performed a sensitivity study, in which 500 and 1,000 years were both added and subtracted from our age model (Supplementary Fig. 9). This arbitrary sensitivity study shows that such errors in the age model do not significantly affect the large increases in ΔpCO2cal-air during HS1, HS4 and HS11 (Supplementary Fig. 9).
Error propagation in pCO2 reconstructions
The uncertainty of each pCO2 value in our record (Fig. 3c) is based on the propagated error of the effect of individual uncertainties in δ11B, calcification depth of N. pachyderma, temperature, salinity and total alkalinity on the pH and pCO2 calculations. The error propagation (2σ) was calculated as the square root of the sum of the squared individual uncertainties. Note that total alkalinity has no effect on the pH estimations; it only affects the pCO2 calculations.
The analytical uncertainty in δ11B ranges from ±0.22 to ±0.43‰, which translates to ∼±10 to ±40 μatm in pCO2. The error in pCO2 due to the uncertainty in the calcification depth of N. pachyderma is equal to ±11 μatm on average (see previous Section and Supplementary Fig. 6). The uncertainty in salinity due to the choice of different salinity-δ18OSW mixing models for the last glacial period and the deglaciation is ∼±1.5‰, which translates to ∼±4 μatm pCO2. The error in total alkalinity due to the uncertainty in salinity estimations is up to ±100 μmol kg−1, which is equivalent to ∼±9 μatm pCO2.
For the assessment of uncertainty in our temperature estimates, one should ideally consider uncertainties associated with empirical calibrations and other non-temperature factors that affect Mg/Ca in N. pachyderma. Because the sensitivity of Mg/Ca in N. pachyderma to factors other than temperature (for example, carbonate chemistry) is not known20, we only include an error of ±0.7 °C, based on the calibration and analytical uncertainties of Mg/Ca (see ref. 15). This uncertainty translates to ±7 μatm pCO2 on average. Ezat et al.15 discussed that the correction for elevated carbonate ion concentration during the LGM on Mg/Ca may lower the LGM temperature by 0–2 °C; however, the exact effect is very uncertain. A decrease in LGM temperatures would decrease our reconstructed pCO2 values (∼−10 μatm decrease per 1 °C decrease), strengthening our conclusion that our study region was an intense area for CO2 uptake at that time.
Data availability
The data generated and analysed during the current study are available along the online version of this article at the publisher’s web-site.
Additional information
How to cite this article: Ezat, M. M. et al. Episodic release of CO2 from the high-latitude North Atlantic Ocean during the last 135 kyr. Nat. Commun. 8, 14498 doi: 10.1038/ncomms14498 (2017).
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
Stocker, T. F. et al. IPCC, 2013: climate change 2013: the physical science basis. Contribution of working group I to the fifth assessment report of the intergovernmental panel on climate change (2013).
Drijfhouta, S. et al. Catalogue of abrupt shifts in Intergovernmental Panel on Climate Change climate models. Proc. Natl Acad. Sci. 112, E5777–E5786 (2015).
EPICA. One-to-one coupling of glacial climate variability in Greenland and Antarctica. Nature 444, 195–198 (2006).
Dansgaard, W. et al. Evidence for general instability of past climate from a 250-kyr ice-core record. Nature 364, 218–220 (1993).
Hemming, S. R. Heinrich events: massive late Pleistocene detritus layers of the North Atlantic and their global climate imprint. Rev. Geophys. 42, RG1005 (2004).
Stocker, T. F. & Johnsen, S. J. A minimum thermodynamic model for the bipolar seesaw. Paleoceanography 18, 1087 (2003).
Petit, J. R. et al. Climate and atmospheric history of the past 420,000 years from the Vostok ice core, Antarctica. Nature 399, 429–436 (1999).
Monnin, E. et al. Atmospheric CO2 concentrations over the last glacial termination. Science 291, 112–114 (2001).
Bereiter, B. et al. Mode change of millennial CO2 variability during the last glacial cycle associated with a bipolar marine carbon seesaw. Proc. Natl Acad. Sci. 109, 9755–9760 (2012).
Takahashi, T. et al. Climatological mean and decadal change in surface ocean pCO2, and net sea–air CO2 flux over the global oceans. Deep-Sea Res. Part II: Top. Stud. Oceanogr. 56, 554–577 (2009).
Rysgaard, S., Bendtsen, J., Pedersen, L. T., Ramløv, H. & Glud, R. N. Increased CO2 uptake due to sea ice growth and decay in the Nordic Seas. J. Geophys. Res.: Oceans 114, C09011 (2009).
Watson, A. J. et al. Tracking the variable North Atlantic sink for atmospheric CO2 . Science 326, 1391–1393 (2009).
Hansen, B. & Østerhus, S. North Atlantic–Nordic Seas exchanges. Prog. Oceanogr. 45, 109–208 (2000).
Ezat, M. M., Rasmussen, T. L. & Groeneveld, J. Persistent intermediate water warming during cold stadials in the southeastern Nordic seas during the past 65 k.y. Geology 42, 663–666 (2014).
Ezat, M. M., Rasmussen, T. L. & Groeneveld, J. Reconstruction of hydrographic changes in the southern Norwegian Sea during the past 135 kyr and the impact of different foraminiferal Mg/Ca cleaning protocols. Geochem. Geophys. Geosyst. 17, 3420–3436 (2016).
Hoff, U., Rasmussen, T. L., Stein, R., Ezat, M. M. & Fahl, K. Sea ice and millennial-scale climate variability in the Nordic seas 90 ka to present. Nat. Commun. 7, 12247 (2016).
Hemming, N. G. & Hanson, G. N. Boron isotopic composition and concentration in modern marine carbonates. Geochim. Cosmochim. Acta 56, 537–543 (1992).
Bereiter, B. et al. Revision of the EPICA Dome C CO2 record from 800 to 600 kyr before present. Geophys. Res. Lett. 42, 542–549 (2015).
Simstich, J., Sarnthein, M. & Erlenkeuser, H. Paired δ18O signals of Neogloboquadrina pachyderma (s) and Turborotalita quinqueloba show thermal stratification structure in Nordic Seas. Mar. Micropaleontol. 48, 107–125 (2003).
Jonkers, L., Jiménez-Amat, P., Mortyn, P. G. & Brummer, G.-J. A. Seasonal Mg/Ca variability of N. pachyderma (s) and G. bulloides: implications for seawater temperature reconstruction. Earth Planet. Sci. Lett. 376, 137–144 (2013).
Key, R. M. et al. The CARINA data synthesis project: introduction and overview. Earth Syst. Sci. Data 2, 105–121 (2010).
Mashiotta, T. A., Lea, D. W. & Spero, H. J. Experimental determination of cadmium uptake in shells of the planktonic foraminifera Orbulina universa and Globigerina bulloides: implications for surface water paleoreconstructions. Geochim. Cosmochim. Acta 61, 4053–4065 (1997).
Boyle, E. A., Sclater, F. & Edmond, J. M. On the marine geochemistry of cadmium. Nature 263, 42–44 (1976).
Elderfield, H. & Rickaby, R. E. M. Oceanic Cd/P ratio and nutrient utilization in the glacial Southern Ocean. Nature 405, 305–310 (2000).
Rickaby, R. E. M. & Elderfield, H. Planktonic foraminiferal Cd/Ca: Paleonutrients or paleotemperature? Paleoceanography 14, 293–303 (1999).
Broecker, W. S. & Maier-Reimer, E. The influence of air and sea exchange on the carbon isotope distribution in the sea. Glob. Biogeochem. Cycles 6, 315–320 (1992).
Spero, H. J., Bijma, J., Lea, D. W. & Bemis, B. E. Effect of seawater carbonate concentration on foraminiferal carbon and oxygen isotopes. Nature 390, 497–500 (1997).
Rasmussen, S. O. et al. A stratigraphic framework for abrupt climatic changes during the Last Glacial period based on three synchronized Greenland ice-core records: refining and extending the INTIMATE event stratigraphy. Quat. Sci. Rev. 106, 14–28 (2014).
Hönisch, B. & Hemming, N. G. Surface ocean pH response to variations in pCO2 through two full glacial cycles. Earth Planet. Sci. Lett. 236, 305–314 (2005).
Henehan, M. J. et al. Calibration of the boron isotope proxy in the planktonic foraminifera Globigerinoides ruber for use in palaeo-CO2 reconstruction. Earth Planet. Sci. Lett. 364, 111–122 (2013).
Keigwin, L. D. & Boyle, E. A. Late quaternary paleochemistry of high-latitude surface waters. Palaeogeogr. Palaeoclim. Palaeoecol. 73, 85–106 (1989).
Yu, J., Thornalley, D. J. R., Rae, J. W. B. & McCave, N. I. Calibration and application of B/Ca, Cd/Ca, and δ11B in Neogloboquadrina pachyderma (sinistral) to constrain CO2 uptake in the subpolar North Atlantic during the last deglaciation. Paleoceanography 28, 237–252 (2013).
Capron, E. et al. Temporal and spatial structure of multi-millennial temperature changes at high latitudes during the Last Interglacial. Quat. Sci. Rev. 103, 116–133 (2014).
Zhang, J., Quay, P. D. & Wilbur, D. O. Carbon isotope fractionation during gas-water exchange and dissolution of CO2 . Geochim. Cosmochim. Acta 59, 107–114 (1995).
Rasmussen, T. L., Thomsen, E., Labeyrie, L. & van Weering, T. C. E. Circulation changes in the Faeroe-Shetland Channel correlating with cold events during the last glacial period (58–10 ka). Geology 24, 937–940 (1996).
Allen, K. A. & Hönisch, B. The planktic foraminiferal B/Ca proxy for seawater carbonate chemistry: a critical evaluation. Earth Planet. Sci. Lett. 345, 203–211 (2012).
Thornalley, D. J. R., Barker, S., Broecker, W. S., Elderfield, H. & McCave, I. N. The Deglacial Evolution of North Atlantic Deep Convection. Science 331, 202–205 (2011).
Stern, J. V. & Lisiecki, L. E. North Atlantic circulation and reservoir age changes over the past 41,000 years. Geophys. Res. Lett. 40, 3693–3697 (2013).
Thornalley, D. J. R. et al. A warm and poorly ventilated deep Arctic Mediterranean during the last glacial period. Science 349, 706–710 (2015).
Meyers, P. A. Organic geochemical proxies of paleoceanographic, paleolimnologic and paleoclimatic processes. Org. Geochem. 27, 213–250 (1997).
Geilfus, N. X. et al. Dynamics of pCO2 and related air‐ice CO2 fluxes in the Arctic coastal zone (Amundsen Gulf, Beaufort Sea). J. Geophys. Res.: Oceans 117, C00G10 (2012).
Rasmussen, T. L. & Thomsen, E. The role of the North Atlantic Drift in the millennial timescale glacial climate fluctuations. Palaeogeogr. Palaeoclim. Palaeoecol. 210, 101–116 (2004).
Marcott, S. A. et al. Centennial-scale changes in the global carbon cycle during the last deglaciation. Nature 514, 616–619 (2014).
Bauska, T. K. et al. Carbon isotopes characterize rapid changes in atmospheric carbon dioxide during the last deglaciation. Proc. Natl Acad. Sci. 113, 3465–3470 (2016).
Barker, S., Greaves, M. & Elderfield, H. A study of cleaning procedures used for foraminiferal Mg/Ca paleothermometry. Geochem. Geophys. Geosyst. 4, 8407 (2003).
Hönisch, B. et al. Atmospheric carbon dioxide concentration across the mid-pleistocene transition. Science 324, 1551–1554 (2009).
Pena, L. D., Calvo, E., Cacho, I., Eggins, S. & Pelejero, C. Identification and removal of Mn-Mg-rich contaminant phases on foraminiferal tests: Implications for Mg/Ca past temperature reconstructions. Geochem. Geophys. Geosyst. 6, Q09P02 (2005).
Martin, P. A. & Lea, D. W. A simple evaluation of cleaning procedures on fossil benthic foraminiferal Mg/Ca. Geochem. Geophys. Geosyst. 3, 8401 (2002).
Lea, D. W. & Boyle, E. A. Determination of carbonate-bound barium in foraminifera and corals by isotope dilution plasma-mass spectrometry. Chem. Geol. 103, 73–84 (1993).
Boyle, E. A. Manganese carbonate overgrowths on foraminifera tests. Geochim. Cosmochim. Acta 47, 1815–1819 (1983).
LeGrande, A. N. & Schmidt, G. A. Global gridded data set of the oxygen isotopic composition in seawater. Geophys. Res. Lett. 33, L12604 (2006).
Azetsu-Scott, K. & Tan, F. C. Oxygen isotope studies from Iceland to an East Greenland Fjord: behaviour of glacial meltwater plume. Mar. Chem. 56, 239–251 (1997).
Tarasov, L. & Peltier, W. R. Arctic freshwater forcing of the Younger Dryas cold reversal. Nature 435, 662–665 (2005).
Klochko, K., Kaufman, A. J., Yao, W., Byrne, R. H. & Tossell, J. A. Experimental measurement of boron isotope fractionation in seawater. Earth Planet. Sci. Lett. 248, 276–285 (2006).
Sanyal, A. et al. Oceanic pH control on the boron isotopic composition of foraminifera: evidence from culture experiments. Paleoceanography 11, 513–517 (1996).
Sanyal, A., Bijma, J., Spero, H. & Lea, D. W. Empirical relationship between pH and the boron isotopic composition of Globigerinoides sacculifer: implications for the boron isotope paleo-pH proxy. Paleoceanography 16, 515–519 (2001).
Hönisch, B. et al. The influence of symbiont photosynthesis on the boron isotopic composition of foraminifera shells. Mar. Micropaleontol. 49, 87–96 (2003).
Dickson, A. G. Thermodynamics of the dissociation of boric acid in synthetic seawater from 273.15 to 318.15 K. Deep Sea Res. Part A. Oceanogr. Res. Pap. 37, 755–766 (1990).
Foster, G. L., Pogge von Strandmann, P. A. E. & Rae, J. W. B. Boron and magnesium isotopic composition of seawater. Geochem. Geophys. Geosyst. 11, Q08015 (2010).
Martínez-Botí, M. A. et al. Boron isotope evidence for oceanic carbon dioxide leakage during the last deglaciation. Nature 518, 219–222 (2015).
Jeansson, E. et al. The Nordic Seas carbon budget: Sources, sinks, and uncertainties. Glob. Biogeochem. Cycles 25, GB4010 (2011).
Zeebe, R. E. & Wolf-Gladrow, D. A. CO2 in Seawater: Equilibrium, Kinetics, Isotopes Elsevier (2001).
Pierrot, D., Lewis, E. & Wallace, D. W. R. MS Excel program developed for CO2 system calculations ORNL/CDIAC-105, Carbon Dioxide Information Analysis Center, Oak Ridge National Laboratory, U.S. Department of Energy (2006).
Millero, F. J. et al. Dissociation constants of carbonic acid in seawater as a function of salinity and temperature. Mar. Chem. 100, 80–94 (2006).
Lee, K. et al. The universal ratio of boron to chlorinity for the North Pacific and North Atlantic oceans. Geochim. Cosmochim. Acta 74, 1801–1811 (2010).
Schlitzer, R. Ocean Data View. http://odv.awi.de (2016).
Svensson, A. et al. A 60,000 year Greenland stratigraphic ice core chronology. Clim. Past 4, 47–57 (2008).
Acknowledgements
We sincerely thank J. Ruprecht, K. Esswein, U. Hoff, J. Farmer, T. Dahl, E. Ellingsen, I. Hald, K. Monsen, L. Pena, K. Allen, M. Segl and S. Pape for valuable support in the laboratory and L. Skinner, D. Thornalley, U. Hoff, J. McManus, J. Farmer and H. Spero for helpful discussions. We also thank the three anonymous reviewers for their very constructive comments and suggestions. This research was funded by the Research Council of Norway through its Centres of Excellence funding scheme, project number 223259. M.M. Ezat has also received funding from the Arctic University of Norway and the Mohn Foundation to the Paleo-CIRCUS project.
Author information
Authors and Affiliations
Contributions
M.M.E. sampled the core, performed the boron isotope analyses, cleaned the foraminiferal samples for the minor/trace analyses and wrote the first draft of the paper. T.L.R. conceived the study and contributed substantially to all aspects. B.H. supervised the boron isotope analyses, cleaning of foraminiferal samples and all carbonate chemistry calculations. All authors interpreted the results and contributed to the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
Supplementary Figures, Supplementary Tables and Supplementary References (PDF 1943 kb)
Supplementary Data 1
Isotope and trace element data in planktic and benthic foraminifera from sediment core JM11-FI-19PC. (XLSX 71 kb)
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Ezat, M., Rasmussen, T., Hönisch, B. et al. Episodic release of CO2 from the high-latitude North Atlantic Ocean during the last 135 kyr. Nat Commun 8, 14498 (2017). https://doi.org/10.1038/ncomms14498
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/ncomms14498
This article is cited by
-
Deglacial upwelling, productivity and CO2 outgassing in the North Pacific Ocean
Nature Geoscience (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.