Agriculture causes nitrate fertilization of remote alpine lakes

Abstract

Humans have altered Earth’s nitrogen cycle so dramatically that reactive nitrogen (Nr) has doubled. This has increased Nr in aquatic ecosystems, which can lead to reduced water quality and ecosystem health. Apportioning sources of Nr to specific ecosystems, however, continues to be challenging, despite this knowledge being critical for mitigation and protection of water resources. Here we use Δ¹⁷O, δ¹⁸O and δ¹⁵N from Uinta Mountain (Utah, USA) snow, inflow and lake nitrate in combination with a Bayesian-based stable isotope mixing model, to show that at least 70% of nitrates in aquatic systems are anthropogenic and arrive via the atmosphere. Moreover, agricultural activities, specifically nitrate- and ammonium-based fertilizer use, are contributing most (∼60%) Nr, and data from other North American alpine lakes suggest this is a widespread phenomenon. Our findings offer a pathway towards more effective mitigation, but point to challenges in balancing food production with protection of important water resources.

Introduction

The global nitrogen cycle has been drastically altered by human activities^1,2. Reactive nitrogen (Nr), or biologically available nitrogen, which includes the inorganic forms ammonia (NH₃), ammonium (NH₄⁺), nitrogen oxide (NO_x), nitric acid (HNO₃), nitrous oxide (N₂O), nitrate (NO₃⁻) and others (for example, peroxyacetyl nitrates, as well as organic compounds (for example, urea, amines, proteins and nucleic acids)), has been doubled by human activities³. Nr is added to the environment naturally by lightning (5 Tg N per year) and by terrestrial (58 Tg N per year) and marine (140 Tg N per year) biological nitrogen fixation for a total of 203 Tg N per year⁴. Anthropogenic activities, including production of synthetic fertilizers and byproducts of fossil fuel combustion (NO_x), add 210 Tg N per year of Nr (ref. 4). Terrestrial Nr emissions are estimated to be 100 Tg N per year (75 Tg N per year are anthropogenic)⁴, which, despite relatively short residence times, can be transported and deposited hundreds to thousands of kilometres from their source^5,6.

Owing to the transportable nature of Nr, even remote regions may receive increased inputs⁷. Because of a paucity of monitoring stations in isolated areas, the relative contribution of atmospheric Nr is unclear and its specific sources are uncertain. Alpine lakes can be limited by nitrogen^8,9,10,11 and thus even modest increases in nitrogen deposition can have significant effects including eutrophication, acidification and reduction of biodiversity¹². To protect alpine lake ecosystems, which are globally important water resources for adjacent lowlands¹³ and biodiversity hotspots¹⁴, identification of the specific contributions of different nitrogen sources to these remote regions is needed to implement successful mitigation policies.

Here we use stable isotope analysis of nitrates (δ¹⁵N, δ¹⁸O and Δ¹⁷O) in modern water (inflows and lakes) and snow samples in combination with a stable isotope mixing-model (SIAR, Stable Isotope Analysis in R)¹⁵, to determine the proportional contribution of various nitrate sources to the Uinta Mountains, Utah, USA. This mountain range, located in northeast Utah (40° to 41° N, 109° to 111° W), has experienced little direct human impact, making it ideal for investigating the influence of atmospheric nitrate¹⁶. The ‘triple oxygen isotope’ approach for the analysis of nitrate allows us to take advantage of the anomalous enrichment of ¹⁷O–NO₃⁻ that results from transfers of ¹⁷O from ozone during photochemical conversion of NO_x to NO₃ in the atmosphere¹⁷. This signature of a mass-independent oxygen isotope fractionation, quantified by the term Δ¹⁷O (Δ¹⁷O=δ¹⁷O−0.52 × δ¹⁸O), differs from the mass-dependent relationship between δ¹⁷O and δ¹⁸O characteristic of terrestrial processes, which is described by the terrestrial fractionation line. Therefore, using Δ¹⁷O–NO₃⁻ allows for clear differentiation of nitrate originating from fossil fuel burning, biomass burning and lightning that is oxidized in the atmosphere versus nitrate oxidized in freshwater or terrestrial systems. The ¹⁷O anomaly is measurable after wet or dry deposition of atmospherically oxidized (and ¹⁷O-enriched) NO₃⁻ at the Earth’s surface and through subsequent reactions affecting this nitrate (for example, assimilation and denitrification)¹⁸. Analysis of δ¹⁵N and δ¹⁸O provides further insight into the sources and/or processes that control nitrate isotopic composition^19,20,21,22. The δ¹⁵N is helpful in distinguishing various sources of nitrogen and, together with δ¹⁸O, can also be used to help identify reactions such as denitrification²³. Values of δ¹⁵N–NO₃⁻ have been used similarly in previous research to classify sources^24,25,26. We have quantified the contributions of particular nitrate sources to inflow and lakewater samples using a stable isotope mixing model contained in the software package SIAR^27,28. SIAR is particularly useful for nitrate source allocation for three reasons. First, uncertainty in the system can be included by factoring in variability in source values. Second, solutions can be found for systems with more potential sources than previous approaches have allowed. Third, probability distributions are generated for each source¹⁵.

Our results show that atmospheric deposition of Nr from anthropogenic sources contributes at least 70% of the total nitrate to Uinta Mountain inflows and lakes; the majority of this is from atmospherically delivered fertilizers. Similarities in nitrate isotope compositions (δ¹⁵N and δ¹⁸O) between Uinta Mountain lakes and lakes throughout the US Rocky Mountains suggest that these findings apply to other mountain regions in western North America. Our research underlines the importance of careful management of fertilizer application in adjacent lowlands to protect alpine ecosystems and also demonstrates the power of applying a combined nitrogen- and triple oxygen-isotope approach to quantify human inputs to the nitrogen cycle.

Results

Water chemistry and stable isotope compositions

Water chemistry and stable isotope compositions were measured in six Uinta Mountain lakes and their inflows and snow from nearby sites (Fig. 1). Mean values of δ¹⁵N–NO₃⁻ for lakes (+0.8‰) and snow (+0.9‰) are higher than for inflows (−1.6‰) and δ¹⁵N–NO₃⁻ of snow spans a slightly larger range than inflow and lake samples (range for snow is −1.2 to +3.4‰; for lakes is −1.1 to +2.4‰ and for inflows is −3.3 to +1.0‰) (Figs 2 and 3, and Supplementary Table 1). Inflows have higher and more variable nitrate concentrations (mean NO₃⁻=28.7±16.78 μM) than do lakes (mean NO₃⁻=1.6±2.2 μM), whereas mean NH₄⁺ concentrations for inflows and lakes are identical (0.9 μM) (Fig. 2 and Supplementary Table 1). Mean values of δ¹⁸O–NO₃⁻ for lakes (+16.7‰) are higher than for inflows (+11.7‰) (Fig. 2 and Supplementary Table 1). Of 41 samples analysed here, all but one have Δ¹⁷O–NO₃⁻ that are elevated above the terrestrial fractional line (Δ¹⁷O=0±1‰) (Figs 3 and 4). Snow samples have particularly high Δ¹⁷O–NO₃⁻ with a mean value of +23.7‰ (Figs 2 and 3, and Supplementary Table 1). Snow samples are also characterized by high mean NH₄⁺ (5.8 μM) concentrations and δ¹⁸O–NO₃⁻ (+66.0‰) (Fig. 2).

Figure 1: Map of Uinta Mountain study area.

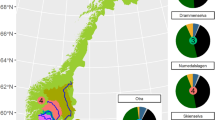
(a) Location of the Uinta Mountains in northeastern Utah and USA, including water sampling point in Great Salt Lake (see Supplementary Methods); (b) the locations of study lakes and snow sampling sites within the Uinta Mountains. Reproduced and modified from Hundey et al.¹⁶ with permission from John Wiley and Sons.

Figure 2: Summary boxplots of Uinta Mountain sample data.

(a) NO₃⁻ (μM), (b) NH₄⁺ (μM), (c) δ¹⁵N-NO₃⁻ (‰), (d) δ¹⁸O-NO₃⁻ (‰) and (e) Δ¹⁷O-NO₃⁻ (‰). The median is represented by the horizontal black bar, the lower and upper boundaries of the box represent the lower (25%) and upper (75%) quartiles of the data and the whiskers represent the minimum and maximum values, excluding outliers. Outliers are represented as points outside the whiskers. The NO₃⁻ sample concentrations reported here are higher than the true average for these Uinta Mountain sites, because sample selection for isotopic analysis was limited by nitrate concentration.

Figure 3: Δ¹⁷O-NO₃⁻ versus δ¹⁵N-NO₃⁻ of Uinta Mountain water samples relative to possible sources.

The SIAR model inputs for nitrate sources are represented by the blue and grey boxes. The lines shown below the x axis (δ¹⁵N) indicate source N isotopic ranges (see also Supplementary Table 2). The overlap in source values for δ¹⁵N (NH₄⁺ & NO₃⁻ Fertilizer+Rain NH₄⁺, Soil NO₃⁻ and Septic Effluent and Manure) is shown by the overlap of lines below the x axis. Model inputs for the nitrate sources are drawn from the literature²¹ other than for AON, which was derived from the mean and s.d. of the Uinta Mountain snow samples. Samples plotting above terrestrial source Δ¹⁷O (0±1‰) contain a significant contribution from AON.

Figure 4: δ¹⁷O- versus δ¹⁸O-NO₃⁻ of Uinta Mountain water samples compared with the terrestrial fractionation line.

Δ¹⁷O is the difference between the measured δ¹⁷O and the δ¹⁷O expected based on the terrestrial fractionation line (δ¹⁷O=0.52 × δ¹⁸O)⁵⁶.

Source contribution model

Potential sources of Nr to Uinta Mountain lakes and inflows are both distal and proximal (that is, within the catchment). Distal sources could include fossil fuel combustion from communities in the adjacent Uinta Basin and nearby (∼150 km) Wasatch Front and agriculture (including application of synthetic fertilizers, manure and urea) along the Wasatch Front or further upwind (for example, California) (Fig. 1). Catchment sources could include naturally biologically fixed nitrogen from soils or inflows and, although probably insignificant, manure from grazing sheep and cattle, which would have peaked in these catchments at the onset of the twentieth century¹⁶. Here we distinguish among four categories of sources for nitrates (Fig. 3 and Supplementary Table 2). The first is atmospherically oxidized nitrate (AON), which originates from natural (lightning, wildfire and soil emissions), anthropogenic (fossil fuel combustion and biomass burning) or mixed (for example, soil emissions from fertilized fields) sources that are oxidized atmospherically. The second is ‘NH₄⁺ and NO₃⁻ Fertilizer+Rain NH₄⁺’. This category includes NO₃⁻- and NH₄⁺-based synthetic fertilizers and rain NH₄⁺, which are combined because their sources are isotopically indistinguishable²¹. Rain NH₄⁺ is largely derived from fertilizer, although natural soil emissions and manure can also contribute^21,29. The third category is soil nitrate (Soil NO₃⁻), which largely represents unamended soil from natural systems, for example, beneath forest vegetation²¹. The final is ‘Septic Effluent and Manure’.

The proportional contribution of these sources to inflows and lakes are graphically summarized by SIAR using 95, 75 and 50% Bayesian credible intervals (Fig. 5). For inflows, NO₃⁻ and NH₄⁺ Fertilizer+Rain NH₄⁺ is the largest contributor of nitrate, at 62% modal probability estimate (MPE, the solution with the highest probability) (Fig. 5 and error terms in Table 1). AON contributes 23% MPE to the total nitrate in inflows, Soil NO₃⁻ contributes 14% MPE and Septic Effluent and Manure contributes 1% MPE (all P-values<0.01). NH₄⁺- and NO₃⁻-based fertilizers+rain NH₄⁺ is also the main contributor to lake nitrate (35% MPE), although a much greater proportional contribution comes from Soil NO₃⁻ (34% MPE) than for inflows. AON contributes 24% MPE to lakes, only slightly higher than inflows, and Septic Effluent and Manure contributes 2% MPE (Fig. 5). It is noteworthy that the Bayesian credible intervals for inflows are smaller compared with those for lakes, in particular for sources other than AON.

Figure 5: Mixing model estimates of nitrate source contributions.

Mixing model estimates of contributions of nitrate sources based on δ¹⁵N-NO₃⁻ and Δ¹⁷O-NO₃⁻ of samples are displayed for (a) inflows and (b) lakes. Bayesian credible intervals show estimated contributions of each source to inflows and lakes, as determined using the stable isotope mixing model SIAR. The 50, 75 and 95% Bayesian credible intervals for each source are shown by the dark, medium and light boxes, respectively.

Table 1 SIAR model results for various sources of nitrate to inflows and lakes.

A comparison of the isotopic compositions of Uinta Mountain lake nitrates with those from national parks of the North American Cordillera³⁰ shows similarity in lakewater NO₃⁻ concentrations and δ¹⁵N- and δ¹⁸O–NO₃⁻ isotopic compositions (Fig. 6). This suggests that our results may be applicable to other western US mountain ranges. If so, this would show that a large proportion of alpine Nr is arriving atmospherically from fertilizers.

Figure 6: Comparison of Uinta Mountain lake nitrate sample isotopic compositions from five national parks in the US Cordillera.

(a) δ¹⁸O- versus δ¹⁵N-NO₃⁻ and (b) NO₃⁻ concentration versus δ¹⁵N-NO₃⁻. The ellipses, which outline the 50% confidence level of each location (grey outline for Rocky Mountain sites, red for Uinta Mountains), are used to visualize each group of lakes and the overlap among the sites. Yellowstone National Park is represented by a single measurement. Data for sites other than the Uinta Mountains are from Nanus et al³⁰.

Discussion

Snow sample δ¹⁵N–NO₃⁻ is higher and spans a greater range than inflow samples (Figs 2 and 3). To explain this feature, we hypothesize that some fertilizer-derived N is still present as NH₄⁺ in the snowpack, where nitrification does not occur³¹. This is supported by the high concentrations of NH₄⁺ in snow samples relative to the inflows and lakes (Fig. 2), and is further corroborated by a calculated snow δ¹⁵N–NH₄⁺ of −4.5‰, which is within the known range for fertilizer ammonium (see Methods). On release from snow into soil, snowmelt or inflows, NH₄⁺ can be nitrified to NO₃⁻, resulting in the high NO₃⁻ concentrations and lower δ¹⁵N–NO₃⁻ that characterize inflows (Fig. 2).

There are several possible explanations for the higher δ¹⁵N–NO₃⁻ in lakes relative to inflows, including atmospheric acidity, denitrification, differences in nitrate sources and/or nitrate assimilation. Atmospheric acidity has the potential to increase δ¹⁵N–NO₃⁻ (ref. 32); however, spatial analyses of precipitation δ¹⁵N–NO₃ from across the midwestern and eastern USA suggest that δ¹⁵N–NO₃ is more influenced by source than precipitation pH, or SO₄²⁻ or NO₃⁻ concentrations²⁵. Furthermore, paleolimnological data from our Uinta Mountain sites supports only a minor role for atmospheric acidity. These data show the most rapid decrease in lake sediment δ¹⁵N beginning in the mid-1900s and continuing to the present¹⁶, whereas reductions in atmospheric pH and SO₄²⁻ or NO₃⁻ concentrations show slight declines after 1985 at western US sites³³.

During denitrification concentrations of NO₃⁻ decrease (as NO₃⁻ is converted to N₂), δ¹⁵N–NO₃ and δ¹⁸O–NO₃ increase³⁴, and Δ¹⁷O–NO₃ remains unaffected. Although these features are observed in our study (Fig. 2), anaerobic conditions favouring denitrification are unlikely in the waters of oligotrophic Uinta Mountain lakes^16,35. Moreover, previous studies suggest that 1:1 to 2:1 enrichment in ¹⁵N relative to ¹⁸O characterizes residual nitrate following denitrification²³, whereas we observe a greater enrichment of ¹⁸O relative to ¹⁵N.

Differences in nitrate sources could also explain the higher δ¹⁵N–NO₃⁻ in lakes than inflows. The variations in δ¹⁵N and Δ¹⁷O for both lake and inflow nitrates are small (Figs 2 and 3) considering the large range in nitrate concentrations (Fig. 2), site locations, inflow routes (for example, wetland and talus slopes) and sampling times (Supplementary Table 1). Given the many potential pathways travelled by NO₃⁻ and processes affecting NO₃⁻, such a small amount of variation is perhaps unexpected but is explained if source compositions are important in determining δ¹⁵N–NO₃. This observation suggests the dominance of a widespread and isotopically homogenous source. This is supported by nearly identical AON source contribution to Uinta Mountain lakes and inflows as determined using Δ¹⁷O–NO₃⁻ (MPEs of 23 and 24%, respectively) (Fig. 5). Snow samples, by comparison, have significantly higher δ¹⁸O- and Δ¹⁷O–NO₃⁻ than lakes and inflows (Fig. 2), which is expected, because the isotopic composition of snow should be most connected to the atmosphere and least affected by the surrounding terrestrial system.

The AON originates mostly from fossil fuel combustion, lightning, soil emissions and biomass burning (Table 2). Based on global approximations of tropospheric NO_x sources³⁶, we estimate that ∼10% of total nitrate inputs to Uinta Mountain lakes are derived from fossil fuel combustion, delivered as AON (Table 2). This may be an underestimation, as our sites lie upwind of two major sources of NO_x, oil and gas production to the southwest in the Uinta Basin³⁷ and the populous Wasatch Front to the west³⁸. In addition, peroxyacetyl nitrates formed by reactions of fossil fuel-derived NO₂ with peroxacyl radicals, themselves formed by oxidation and photolysis of volatile organic trace gases, could enable long-distance transport of NO_x, enhancing delivery of AON to the Uinta Mountains from these upwind sources³⁹.

Table 2 Sources of tropospheric NO_x and their estimated contribution to Uinta Mountain lake nitrates.

The outstanding question is the source for the rest of the nitrate inputs. The lower δ¹⁵N–NO₃⁻ of Uinta Mountain lakes and inflows indicates that a high proportion of the nitrate is derived from fertilizer (35% MPE for lakes and 62% MPE for inflows: NH₄⁺ and NO₃⁻ Fertilizer+Rain NH₄⁺ in Table 1). The difference between lakes and inflows in apportionment of the NH₄⁺ and NO₃⁻ Fertilizer+Rain NH₄⁺ (35% MPE versus 62% MPE) and Soil NO₃⁻ categories (33% MPE versus 14% MPE) (Fig. 5 and Table 1) is initially surprising. As most water in lakes and inflows originates from precipitation, source apportionment should be similar, as is the case for the well-constrained AON contributions (Fig. 5). However, if additional Soil NO₃⁻ in lakes originates from the catchment, then the AON contribution to lakes should have been lower than for inflows.

Nitrate assimilation in lakes can provide an explanation for their higher δ¹⁵N–NO₃⁻ and lower NO₃⁻ concentrations relative to inflows, and differences in source apportionment between lakes and inflows (Fig. 5). During the spring/summer periods sampled here, snowmelt results in peak nitrate inputs, which lead to increased lake production and thus nitrate assimilation^16,40. During assimilation, lake NO₃⁻ concentrations should decrease substantially relative to inflows, as is reported here (Fig. 2). Assimilation would also cause lake δ¹⁵N–NO₃⁻ to increase, because algae and aquatic plants preferentially use ¹⁴N (ref. 41). Such ¹⁵N enrichment would cause the Soil NO₃⁻ source category to be overrepresented in lakes relative to inflows, as is observed here.

Fertilizers, therefore, are currently the most important influence on nitrate concentrations in high elevation sites in the Uinta Mountains (Fig. 5). As neither NH₄⁺- nor NO₃⁻-based fertilizers are used directly in the catchments, this contribution is attributed to atmospherically delivered fertilizers from nearby to distant agricultural regions. Based on the main wind directions being south, southwest and west^42,43,44, the most probable source is the Wasatch Front, but it could be much further away (for example, California) (see Supplementary Methods for additional information on source areas).

Collectively, these arguments lead to the conclusion that anthropogenic sources (primarily atmospherically delivered fertilizers (∼60%) and fossil fuel combustion (∼10%)) currently comprise ∼70% MPE of the nitrates delivered to the Uinta Mountain alpine aquatic ecosystems. A sizeable fraction of this nitrate is being assimilated by Uinta Mountain lakes, with attendant implications for increased primary lacustrine productivity.

Can we extend these findings to other western alpine sites? The average snow Δ¹⁷O–NO₃⁻ from the Uinta Mountains (+23.7±5.6‰) is within the documented atmospheric range in non-polar regions of +24 to +33‰ (ref. 45) and comparable to the average Δ¹⁷O–NO₃⁻ of three samples from a small catchment in the Colorado Front Range (+28.6±0.2‰)⁴⁶. Considerable overlap also exists between Uinta Mountain snow nitrates (δ¹⁸O–NO₃⁻ range: +48.6 to +75.1‰, median: +69.4‰) and precipitation nitrate measured in several US Cordilleran national parks (δ¹⁸O–NO₃⁻ range: +71 to +78‰, median: +74.5‰)³⁰. Similarly, high δ¹⁸O–NO₃⁻ in several US Cordilleran lakes³⁰ shows considerable overlap with Uinta Mountain lake nitrates (Fig. 6). The high δ¹⁸O–NO₃⁻ (>0‰) and low δ¹⁵N–NO₃⁻ (<+5‰) of these US Cordilleran lakes suggest influence by wet deposition of dissolved inorganic nitrogen (NO₃⁻+NH₄⁺) and fertilizer sources of dissolved inorganic nitrogen³⁰. In the absence of Δ¹⁷O–NO₃ data for other alpine lakes, this comparison suggests widespread atmospheric inputs of oxidized nitrate to alpine settings in western North America and potential effects from such nitrogen fertilization.

Baseline (that is, before fossil fuel burning and synthetic fertilizer production) nitrate concentrations remain virtually unknown for North American alpine sites. In the absence of such information, our results provide evidence for proportionally large contributions of nitrogen from new, anthropogenic sources, most importantly from atmospheric deposition of fertilizers used in agriculture. Based on paleolimnological evidence, it has been suggested that increased nitrogen delivery from these additional sources began at the onset of the twentieth century but was most pronounced after the mid-twentieth century⁴⁷. Increased nitrogen inputs have led to increased lake primary production and other ecological changes in the Colorado Front Range^24,48, Beartooth Range⁴⁹ and Uinta Mountains¹⁶. Our study confirms these new sources of nitrogen. Taken together, and assuming that human populations continue to expand and agricultural fertilizer use continues to rise, these findings point to potential future of N-enrichment, decreased water quality and loss of biodiversity. The findings of our research, achieved by combining measurement of nitrate Δ¹⁷O, δ¹⁸O and δ¹⁵N with a Bayesian mixing model to discriminate among Nr sources, point to the challenges faced by society to balance needs for adequate food production with protection of critical water resources needed by our rapidly expanding population.

Methods

Study lake selection

Seven high elevation (>3,000 m.a.s.l.), oligotrophic lakes were selected for this study from the Uinta Mountains. These study lakes, with lake codes used in this study shown in bold and Utah Department of Wildlife Resources codes in parentheses, are: Denise UN07 (WR-9), Taylor UN08 (WR-8), Upper Carrol UN55 (X-18), East Carrol UN56 (X-21), No Name UN57 (X-26), Bluebell Pass UN58 (X-25) and Walk-Up Lake UN32 (WR-55) (Fig. 1). Lake water, inflow and snow samples were collected from each site in summer 2008, 2009 and 2012 (Supplementary Table 1). Lake and inflow samples represent a single day sample and therefore a snapshot view, whereas the snow samples represent seasonal accumulation. To evaluate the effect on the isotopic composition that could occur during snowmelt, snow samples were also collected around the time of maximum snow accumulation but before spring snowmelt (March 2009 and April 2011). For logistical reasons, snow sampling at the time of maximum snow accumulation was not possible at the lake sites, but was performed instead at nearby sites (Chepeta, Spirit Lake and Lake Fork sites are within 13 km of the lake sites) and at the west and east extent of the Uinta mountains (Trial Lake and Grizzly Ridge sites are within 50 km of the lake sites) (Fig. 1) with the support of the United States Department of Agriculture and the United States Geological Survey.

Water and snow sampling

For the lake water samples, the bottles were filled ∼0.5 m below the water surface at the deepest portion of the lake. Inflow samples were collected from streams and in some cases from rivulets from melting snow. Samples were collected for stable isotope analysis in 250 ml amber Nalgene bottles, kept cool in the field and frozen as soon as logistically possible (1–3 days). Summer snowpack samples were taken in zip lock bags after removing the outer ∼2 cm of surface snow. The snow samples were transferred to tightly sealed sample bottles in the field after melting and then frozen. Samples for stable isotope measurements were stored frozen until analysed at the Laboratory for Stable Isotope Science (LSIS), located at the University of Western Ontario in London, Canada.

The March 2009 snow samples (Lake Fork, Spirit Lake, Chepeta Lake and Trial Lake sites) were collected by the Natural Resources Conservation Services of the United States Department of Agriculture with a standard federal snow sampler using standard snow sampling techniques⁵⁰. The April 2011 samples (Grizzly Ridge and Lake Fork sites) were collected from snow pits following methods used by the United States Geological Survey⁵¹. The samples were shipped frozen in clean, 19-l paint buckets to Lakes and Reservoir Systems Research Facility, also at the University of Western Ontario, where they were melted and aliquots provided to LSIS for stable isotopic analysis. Samples for water chemistry analysis (NO₂, NH₄ and NO₂+NO₃) were taken concurrently with the isotope samples and filtered using a 0.7-μm Whatman GF/F filter. Samples were frozen and sent to Chesapeake Biological Laboratory in MD, USA, for chemical analyses.

Of the collected inflow, lake and snow samples, 34 had sufficiently high nitrate concentrations (>1.6 μM) for analysis of δ¹⁵N-, δ¹⁸O- and Δ¹⁷O–NO₃⁻. As only two lake samples met this nitrate concentration threshold, we analysed ten previously excluded lake samples (owing to nitrate concentrations <1.6 μM NO₃). Of these, we were able to obtain δ¹⁵N and δ¹⁸O for seven samples, three of which also yielded Δ¹⁷O data. In total, 41 Uinta Mountain samples and one sample from Great Salt Lake (see Supplementary Methods) were analysed for stable isotopes of nitrate (Supplementary Table 1).

Nitrate isotope analysis

We used the coupled cadmium-azide reduction method with some modifications to prepare nitrate for analysis of δ¹⁸O, Δ¹⁷O and δ¹⁵N. The chemical procedure involves two main steps. The first is conversion of nitrate to nitrite using activated cadmium and the second is conversion of nitrite to N₂O by injecting 0.8 ml of a 1:1 by volume mixture of 2 M sodium azide and 20% acetic acid⁵². The δ¹⁵N and δ¹⁸O values are measured from N₂O by monitoring the masses 44, 45 and 46 (¹⁴N¹⁴N¹⁶O, ¹⁴N¹⁵N¹⁶O+¹⁴N¹⁴N¹⁷O and ¹⁴N¹⁴N¹⁸O, respectively). The Δ¹⁷O values are measured on O₂ formed by thermal decomposition of N₂O using a gold catalyst at 875 °C (ref. 53) by monitoring the masses 32, 33 and 34. All measurements were made using a Thermo Finnigan Delta^PLUS XL isotope ratio mass spectrometer accessorized with a Gas Bench II and CTC CombiPal autosampler.

Corrections and calibration

A number of corrections are made to the raw δ-values to account for overlapping masses and memory effects⁵⁴. Briefly, for δ¹⁵N, two corrections are made to account for the mass overlap between ¹⁵N¹⁴N¹⁶O and ¹⁴N¹⁴N¹⁷O (mass=45). First, a ‘Craig Correction’ is applied by the isotope ratio mass spectrometer operating system (ISODAT) and accounts for the mass overlap of these isotopologues. Second, a correction is applied to account for the mass-independent (that is, Δ¹⁷O>0) overlap between these same isotopologues, for which the Craig correction does not account. Through experimentation, we have determined that uncorrected δ¹⁵N is increased by 0.1‰ (denoted X_corr below) for every 1‰ increase in Δ¹⁷O. This offset in δ¹⁵N was determined from equation (1):

where $d^{\text{15}}{\text{N}}_{{\text{N}}_{\text{2}}{\text{O}}_{\text{USGS35cal}}}$, δ¹⁵N_USGS35true and Δ¹⁷O_USGS35cal are the calibrated (cal) and true values of USGS-35 nitrate. To account for the non-zero Δ¹⁷O, we have applied equation (2):

where is the corrected value reported in this study, is the calibrated result produced using standards and Δ¹⁷O_sample is the Δ¹⁷O value of the sample. Therefore, for snow samples with Δ¹⁷O upwards of +30‰, this effect causes uncorrected δ¹⁵N to be higher than the true value by ∼+3‰. In the case of four lake water samples, we could not correct for the mass overlap between ¹⁵N¹⁴N¹⁶O and ¹⁴N¹⁴N¹⁷O, because the nitrate concentrations were too low to measure Δ¹⁷O accurately. In these cases, uncorrected δ¹⁵N–NO₃⁻ was used. Although the inclusion of uncorrected results is not ideal, the correction would have probably been minor, because the lakes tend to have low Δ¹⁷O–NO₃⁻ (mean lake Δ¹⁷O=+5.3‰). Corrections are also made to δ¹⁸O in each analytical run to account for exchange between sample water and laboratory water. This is necessary, because a fraction (on average 13%) of oxygen that ends up in the measured nitrous oxide product is derived from exchange with water oxygen rather than from the original nitrate oxygen.

Calibration of the raw isotopic ratios to AIR (nitrogen) and VSMOW (Standard Mean Ocean Water; oxygen) is achieved using international standards USGS-32, USGS-34 and USGS-35 (Supplementary Table 3), and an internal standard GSI-NO-3 (δ¹⁵N=+1.3‰, δ¹⁸O=+14.13‰, courtesy of the Geological Survey of Israel). Accuracy of isotopic compositions was determined by comparison with the value known for standard IAEA-NO-3. Mean δ¹⁸O, Δ¹⁷O and δ¹⁵N obtained on replicate measurements (n=10) of IAEA-NO3 are summarized and compared with accepted values⁵⁴ in Supplementary Table 3. Precision was calculated using duplicates from each analytical session (±0.04‰, ±0.23‰ and ±0.5‰ for Δ¹⁷O, δ¹⁸O and δ¹⁵N, respectively). Internal data from LSIS (N=48) has demonstrated long-term precision for dissolved nitrate sample duplicates to be ±0.50‰, ±1.00‰ and ±0.7‰ for Δ¹⁷O, δ¹⁸O and δ¹⁵N, respectively.

Stable isotopes analysis in R mixing model

Proportional contributions of nitrate sources to Uinta Mountain lakes and inflows were estimated using the stable isotope mixing model SIAR¹⁵. SIAR uses a Bayesian framework, to determine the probability distribution of the proportional contribution of various sources to a mixture. The mathematical definition used can be found in the Supplementary Methods. The model is fit via Markov chain Monte Carlo methods, which produces simulations of plausible source proportions for each sample group¹⁵. Models were run for 500,000 iterations. In this study, we used both δ¹⁵N and Δ¹⁷O in the SIAR model, to differentiate contributions from four source categories: AON, NH₄⁺ and NO₃⁻ Fertilizer+Rain NH₄⁺, Soil NO₃⁻ and Septic Effluent and Manure. Source parameters (mean and s.d.) for input into the SIAR model were estimated from ranges provided in the literature²¹ and are listed in Supplementary Table 2. Two snowmelt samples (Fig. 3) were not included in the SIAR model, because they were located directly below snowpack and were not clearly categorized into either the snowpack or inflow sample group. These data nonetheless provide information on transitional waters between snow and stream. The four lake samples for which we were unable to measure Δ¹⁷O were also not included in the SIAR model. We used the mean and s.d. of all Uinta Mountain snow sample nitrate isotope compositions (mean Δ¹⁷O–NO₃⁻ is +23.7±5.6‰) to represent the regional AON source; this value is comparable to the range in Δ¹⁷O–NO₃⁻ observed in non-polar regions (+24 to +33‰)⁴⁵. We acknowledge that only winter precipitation is represented in our sample set, and that atmospheric Δ¹⁷O can vary seasonally⁵⁵, but felt that our snow sample average was a more accurate representation of regional AON values than a global average.

After initial model runs, which contained five possible nitrate sources (NO₃⁻ Fertilizer was initially separate from NH₄⁺ Fertilizer+Rain NH₄⁺) and δ¹⁵N-, δ¹⁸O- and Δ¹⁷O–NO₃⁻ measurements, we simplified the model in two ways. First, NO₃⁻ Fertilizer and NH₄⁺ Fertilizer+Rain NH₄⁺ were aggregated into the single source described earlier and, second, the model inputs were reduced from three isotopes of nitrate to include only δ¹⁵N and Δ¹⁷O. We combined the NO₃⁻-based fertilizer and NH₄⁺-based fertilizer+rain NH₄⁺ source values into a single source, because they have similar nitrogen isotopic signatures. In addition, both NO₃⁻-based and NH₄⁺-based fertilizers are associated with intensive agricultural activity. NH₄⁺ in rain is also largely derived from fertilizer, although natural soil emissions and manure can also contribute^21,29. Given that Δ¹⁷O- and δ¹⁸O–NO₃⁻ are highly correlated (R²=0.94) and both tracking AON, we removed the parameter δ¹⁸O–NO₃⁻ from the models. This strengthens the model, as the potential variability in δ¹⁸O for terrestrial nitrate and atmospheric nitrate sources is much larger than for Δ¹⁷O. It is not possible to predict the correlation of Δ¹⁷O and δ¹⁸O without making measurements of both. If samples consisted of primarily terrestrial nitrates, the samples would track the terrestrial fractionation line, but this behaviour cannot be discerned from δ¹⁸O alone.

Estimation of snow δ¹⁵N–NH₄⁺

A large component of snow N is in the form NH₄⁺, but our analysis solely measures δ¹⁵N–NO₃⁻. To improve our understanding of the source values of NH₄⁺ in snow, we can calculate the approximate value of δ¹⁵N–NH₄⁺. Assuming that the inflow δ¹⁵N reflects the combined isotopic composition of snow ammonium and nitrate, the δ¹⁵N of snow NH₄⁺ is calculated by solving for $d^{\text{15}}{\text{N}}_{{\text{NH}}_{\text{4}}^{\text{+}}\text{snow}}$ in equation (3):

where δ¹⁵N_inflow and δ¹⁵N_snow are average values. Both NH₄⁺ and NO₃⁻ are reported as N in μM, [total_snow] is the sum of [NH_{4 snow}⁺] and [NO_{3 snow}⁻], and $d^{\text{15}}{\text{N}}_{{\text{NH}}_{\text{3}}^{\text{-}}\text{snow}}$ is assumed to be equal to δ¹⁵N_snow (for data, see Supplementary Table 1). This calculation also assumes that there is little fractionation during conversion from ammonium to nitrate.