Abstract
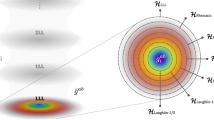
The quantum of thermal conductance of ballistic (collisionless) one-dimensional channels is a unique fundamental constant1. Although the quantization of the electrical conductance of one-dimensional ballistic conductors has long been experimentally established2, demonstrating the quantization of thermal conductance has been challenging as it necessitated an accurate measurement of very small temperature increase. It has been accomplished for weakly interacting systems of phonons3,4, photons5 and electronic Fermi liquids6,7,8; however, it should theoretically also hold in strongly interacting systems, such as those in which the fractional quantum Hall effect is observed. This effect describes the fractionalization of electrons into anyons and chargeless quasiparticles, which in some cases can be Majorana fermions2. Because the bulk is incompressible in the fractional quantum Hall regime, it is not expected to contribute substantially to the thermal conductance, which is instead determined by chiral, one-dimensional edge modes. The thermal conductance thus reflects the topological properties of the fractional quantum Hall electronic system, to which measurements of the electrical conductance give no access9,10,11,12. Here we report measurements of thermal conductance in particle-like (Laughlin–Jain series) states and the more complex (and less studied) hole-like states in a high-mobility two-dimensional electron gas in GaAs–AlGaAs heterostructures. Hole-like states, which have fractional Landau-level fillings of 1/2 to 1, support downstream charged modes as well as upstream neutral modes13, and are expected to have a thermal conductance that is determined by the net chirality of all of their downstream and upstream edge modes. Our results establish the universality of the quantization of thermal conductance for fractionally charged and neutral modes. Measurements of anyonic heat flow provide access to information that is not easily accessible from measurements of conductance.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Pendry, J. B. Quantum limits to the flow of information and entropy. J. Phys. A 16, 2161–2171 (1983)
Das Sarma, S. & Pinczuk, A. (eds) Perspectives in Quantum Hall Effects: Novel Quantum Liquids in Low-dimensional Semiconductor Structures (Wiley, 1996)
Schwab, K., Henriksen, E. A., Worlock, J. M. & Roukes, M. L. Measurement of the quantum of thermal conductance. Nature 404, 974–977 (2000)
Yung, C. S., Schmidt, D. R. & Cleland, A. N. Thermal conductance and electron–phonon coupling in mechanically suspended nanostructures. Appl. Phys. Lett. 81, 31–33 (2002)
Meschke, M., Guichard, W. & Pekola, J. P. Single-mode heat conduction by photons. Nature 444, 187–190 (2006)
Molenkamp, L. W., Gravier, Th., van Houten, H., Buijk, O. J. A. & Mabesoone, M. A. A. Peltier coefficient and thermal conductance of a quantum point contact. Phys. Rev. Lett. 68, 3765–3768 (1992)
Chiatti, O. et al. Quantum thermal conductance of electrons in a one-dimensional wire. Phys. Rev. Lett. 97, 056601 (2006)
Jezouin, S. et al. Quantum limit of heat flow across a single electronic channel. Science 342, 601–604 (2013)
Kane, C. L. & Fisher, M. P. A. Impurity scattering and transport of fractional quantum Hall edge states. Phys. Rev. B 51, 13449–13466 (1995)
Kane, C. L. & Fisher, M. P. A. Quantized thermal transport in the fractional quantum Hall effect. Phys. Rev. B 55, 15832–15837 (1997)
Cappelli, A., Huerta, M. & Zemba, G. R. Thermal transport in chiral conformal theories and hierarchical quantum Hall states. Nucl. Phys. B 636, 568–582 (2002)
Wen, X.-G. Quantum Field Theory of Many-body Systems: From the Origin of Sound to an Origin of Light and Electrons (Oxford Univ. Press, 2004)
Bid, A. et al. Observation of neutral modes in the fractional quantum Hall regime. Nature 466, 585–590 (2010)
Gross, Y., Dolev, M., Heiblum, M., Umansky, V. & Mahalu, D. Upstream neutral modes in the fractional quantum Hall effect regime: heat waves or coherent dipoles. Phys. Rev. Lett. 108, 226801 (2012)
Gurman, I., Sabo, S., Heiblum, M., Umansky, V. & Mahalu, D. Extracting net current from an upstream neutral mode in the fractional quantum Hall regime. Nat. Commun. 3, 1289 (2012)
Inoue, H. et al. Proliferation of neutral modes in fractional quantum Hall states. Nat. Commun. 5, 4067 (2014)
Wellstood, F. C., Urbina, C. & Clarke, J. Hot-electron effects in metals. Phys. Rev. B 49, 5942–5955 (1994)
Jain, J. K. Composite Fermions (Cambridge Univ. Press, 2007)
Meir, Y. Composite edge states in the v = 2/3 fractional quantum Hall regime. Phys. Rev. Lett. 72, 2624–2627 (1994)
Sabo, R. et al. A new paradigm for edge reconstruction in fractional quantum Hall states. Nat. Phys. 13, 4010–4016 (2017)
Bid, A., Ofek, N., Heiblum, M., Umansky, V. & Mahalu, D. Shot noise and charge at the 2/3 composite fractional quantum Hall state. Phys. Rev. Lett. 103, 236802 (2009)
Slobodeniuk, A. O., Levkivskyi, I. P. & Sukhorukov, E. V. Equilibration of quantum Hall edge states by an Ohmic contact. Phys. Rev. B 88, 165307 (2013)
Dahlem, F. Cryogenic scanning force microscopy of quantum Hall samples: adiabatic transport originating in anisotropic depletion at contact interfaces. Phys. Rev. B 82, 121305 (2010)
Buks, E., Heiblum, M., Levinson, Y. & Shtrikman, H. Scattering of a two-dimensional electron gas by a correlated system of ionized donors. Semicond. Sci. Technol. 9, 2031–2041 (1994)
Buks, E., Heiblum, M. & Shtrikman, H. Correlated charged donors and strong mobility enhancement in a two-dimensional electron gas. Phys. Rev. B 49, 14790(R)–14793(R) (1994)
Sivan, U. & Imry, Y. Multichannel Landauer formula for thermoelectric transport with application to thermopower near the mobility edge. Phys. Rev. B 33, 551–558 (1986)
Jiang, J.-H. & Imry, Y. Linear and nonlinear mesoscopic thermoelectric transport with coupling to heat bath. C. R. Phys. 17, 1047–1059 (2016)
Beenakker, C. W. J. & Buttiker, M. Suppression of shot noise in metallic diffusive conductors. Phys. Rev. B 46, 1889–1892 (1992)
Blanter, Y. M. & Sukhorukov, E. V. Semiclassical theory of conductance and noise in open chaotic cavities. Phys. Rev. Lett. 84, 1280–1283 (2000)
Acknowledgements
We acknowledge the help and advice of R. Sabo, I. Gurman, N. OfeK, H.-K. Choi and D. Mahalu. M.H. acknowledges the European Research Council under the European Community’s Seventh Framework Programme, grant agreement number 339070, the partial support of the Minerva Foundation, grant number 711752, and, together with V.U., the German Israeli Foundation (GIF), grant number I-1241-303.10/2014, and the Israeli Science Foundation (ISF). A.S. and Y.O. acknowledge the European Research Council under the European Community’s Seventh Framework Programme (FP7/2007-2013)/ERC, grant agreement number 339070, and the Israeli Science Foundation, ISF agreement number 13335/16. Y.O. acknowledges CRC 183 of the DFG. D.E.F. acknowledges support by the NSF under grant number DMR-1205715.
Author information
Authors and Affiliations
Contributions
M.B., A.R. and M.H. designed the experiment. M.B. and A.R. fabricated the devices with input from M.H. A.R. participated in initial measurements. M.B. and M.H. preformed the measurements, did the analysis and guided the experimental work. Y.O., D.E.F. and A.S. worked on the theoretical aspects. V.U. grew the heterostructures in which the two-dimensional electron gas (2DEG) is embedded. All authors contributed to writing the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
Reviewer Information Nature thanks G. Gervais and the other anonymous reviewer(s) for their contribution to the peer review of this work.
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
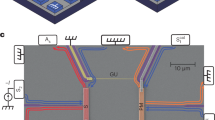
Extended Data Figure 1 Detailed schematic of the structure.
The SEM micrograph shows details away from the floating ohmic contact. In particular, note the two calibration regions, each hosting an added source contact (CS1 or CS3), a ground contact (CG1 or CG3) and a QPC (CQ1 or CQ3, which is fully pinched when thermal noise is measured). When QPC1 is fully pinched and CQ1 is partly pinched, shot noise is measured by the amplifier at D1 (in the same arm), with respect to ground. There are also voltage probes (VP) in each arm for low-frequency lock-in measurements. Q1–Q4 denote QPC1–QPC4.
Extended Data Figure 2 Current splitting among multiple arms.
a, v = 1/3. Current is sourced from S and measured by the amplifier at D1 (see notations in Fig. 1). The blue curve is the reflection coefficient rrel into D1 (a function of the pinching of QPC1) when all of the QPCs in the other arms are open. The other lines correspond to when the QPC in each of the arms pinches separately, while QPC1 is fully open. We see that each arm consumes the same current. VQPC represents the pinching of the relevant QPC. b, v = 3/5. A similar experiment as for a, but in this case the current is sourced from S and measured at D3 as each of the other QPCs pinch. The red and blue lines are the transmissions of QPC1 and QPC3 while both QPC2 and QPC4 were completely pinched; hence, the relative transmission starts from trel = 0.5 and goes down to zero. The other two lines are the transmissions of QPC2 while all QPCs except QPC4 were open, and of QPC4 while all QPCs except QPC2 were open. In these cases, the relative transmission starts from 0.33 and reaches 0.5 when QPC2 or QPC4 was completely pinched.
Extended Data Figure 3 Thermal noise and analysis at v = 1.
a, Thermal noise Sth as a function of IS = Iin for different numbers of arms (N). b, The normalized subtracted power dissipation λ (see main text) as function of  (Tm < 40 mK). Subtracting the contributions for different N cancels out the phonon contribution; the notation in the legend, for example, ‘N = 4 − N = 2’, indicates the power dissipation for four arms, less that for two arms. The fit lines lead to an average thermal conductance per channel of gQ = (0.9 ± 0.1)κ0T.
(Tm < 40 mK). Subtracting the contributions for different N cancels out the phonon contribution; the notation in the legend, for example, ‘N = 4 − N = 2’, indicates the power dissipation for four arms, less that for two arms. The fit lines lead to an average thermal conductance per channel of gQ = (0.9 ± 0.1)κ0T.
Extended Data Figure 4 Raw data of thermal noise Sth and Tm for hole-like states.
a, Thermal noise Sth for two different arm configurations and v = 2/3, as a function of source current IS. b, Deduced Tm as function of the dissipated power ΔP in the floating ohmic contact for the two arm configurations and v = 3/5 or v = 4/7 (T0 = 10 mK).
Extended Data Figure 5 Gain and temperature calibration via shot noise.
a, Transmission t of a QPC for v = 2/3 (blue, top), v = 3/5 (red, middle) and v = 4/7 (green, bottom). Plateaus are visible for the different chiral charge modes at the edge. b, Shot noise SI (green circles) measured for v = 2/3 at the t = 0.5 plateau to calibrate the gain. The black solid line shows a fit with the equation 2e*ISt(1 − t)coth[e*VS/(2kBT0) − 2kBT0/(e*VS)], with e* = 2e/3 and T0 = 10 mK. Linear extrapolation of this fit to zero noise from VS = (e2/h)IS = 2kBT0 (dashed magenta line) provides an exact measure of T0 that is independent of the gain. c, Shot noise SI (red circles) measured for v = 3/5 at the t = 0.55 plateau, with the blue solid line corresponding to a fit using the expression in b, but with e* = 3e/5 and T = 11 mK. d, Resonant response of the LCRH circuit (connected to the drain in parallel with the actual device) at different filling factors. Gains at different filling factors are compared by the ratio of the areas in the appropriate bandwidths. At smaller filling factors, the bandwidth is smaller (largest 30 kHz, smallest 10 kHz).
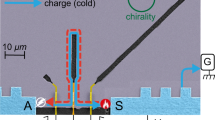
Extended Data Figure 6 Schematics aiding the calculation of the equilibration length in Methods (and the main text).
The schematic shows a single arm with nd downstream modes (in the chiral direction; solid arrows) and nu upstream modes (in the achiral direction; dashed arrows). The arrows marked as Jt represent energy exchange in the equilibration process. The relevant length of each arm is L = x0 − xm.
Rights and permissions
About this article
Cite this article
Banerjee, M., Heiblum, M., Rosenblatt, A. et al. Observed quantization of anyonic heat flow. Nature 545, 75–79 (2017). https://doi.org/10.1038/nature22052
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature22052
This article is cited by
-
Heat conductance of the quantum Hall bulk
Nature (2024)
-
Direct determination of the topological thermal conductance via local power measurement
Nature Physics (2023)
-
Nanoscale electrostatic control in ultraclean van der Waals heterostructures by local anodic oxidation of graphite gates
Nature Physics (2023)
-
Anomalous quantized plateaus in two-dimensional electron gas with gate confinement
Nature Communications (2023)
-
Verification of Wiedemann–Franz Law in Silver with Moderate Residual Resistivity Ratio
Journal of Low Temperature Physics (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.