Abstract
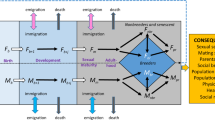
The Trivers–Willard theory1 proposes that the sex ratio of offspring should vary with maternal condition when it has sex‐specific influences on offspring fitness. In particular, mothers in good condition in polygynous and dimorphic species are predicted to produce an excess of sons, whereas mothers in poor condition should do the opposite. Despite the elegance of the theory, support for it has been limited2,3. Here we extend and generalize the Trivers–Willard theory to explain the disparity between predictions and observations of offspring sex ratio. In polygynous species, males typically have higher mortality rates4, different age‐specific reproductive schedules and more risk‐prone life history tactics than females; however, these differences are not currently incorporated into the Trivers–Willard theory. Using two‐sex models parameterized with data from free‐living mammal populations with contrasting levels of sex differences in demography, we demonstrate how sex differences in life history traits over the entire lifespan can lead to a wide range of sex allocation tactics, and show that correlations between maternal condition and offspring sex ratio alone are insufficient to conclude that mothers adaptively adjust offspring sex ratio.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Trivers, R. L. & Willard, D. E. Natural selection of parental ability to vary the sex ratio of offspring. Science 179, 90–92 (1973)
Hewison, A. J. M. & Gaillard, J.‐M. Successful sons or advantaged daughters? The Trivers–Willard model and sex‐biased maternal investment in ungulates. Trends Ecol. Evol. 14, 229–234 (1999)
Sheldon, B. C. & West, S. A. Maternal dominance, maternal condition, and offspring sex ratio in ungulate mammals. Am. Nat. 163, 40–54 (2004)
Clutton‐Brock, T. H. & Isvaran, K. Sex differences in ageing in natural populations of vertebrates. Proc. Biol. Sci. 274, 3097–3104 (2007)
Williams, G. C. Question of adaptive sex‐ratio in outcrossed vertebrates. Proc. Biol. Sci. 205, 567–580 (1979)
Schwanz, L. E., Bragg, J. G. & Charnov, E. L. Maternal condition and facultative sex ratios in populations with overlapping generations. Am. Nat. 168, 521–530 (2006)
Wiebe, K. L. & Bortolotti, G. R. Facultative sex ratio manipulation in American kestrels. Behav. Ecol. Sociobiol. 30, 379–386 (1992)
Ruckstuhl, K. E., Colijn, G. P., Amiot, V. & Vinish, E. Mother’s occupation and sex ratio at birth. BMC Public Health 10, 269 (2010)
Brown, G. R. Sex‐biased investment in nonhuman primates: can Trivers & Willard’s theory be tested?. Anim. Behav. 61, 683–694 (2001)
Cockburn, A., Legge, S. & Double, M. in Sex Ratios: Concepts and Research Methods, (ed. Hardy, I. C. W. ) Ch. 13, 266–286 (Cambridge Univ. Press, 2002)
Leimar, O. Life‐history analysis of the Trivers and Willard sex‐ratio problem. Behav. Ecol. 7, 316–325 (1996)
Taylor, P. D. Allele‐frequency change in a class‐structured population. Am. Nat. 135, 95–106 (1990)
Fisher, R. The Genetical Theory of Natural Selection (Oxford University Press, 1930)
Loison, A., Festa‐Bianchet, M., Gaillard, J.‐M., Jorgenson, J. & Jullien, J. Age‐specific survival in five populations of ungulates: evidence of senescence. Ecology 80, 2539–2554 (1999)
Lawson Handley, L. & Perrin, N. Advances in our understanding of mammalian sex‐biased dispersal. Mol. Ecol. 16, 1559–1578 (2007)
Kappeler, P. Verhaltensbiologie (Springer, 2006)
Charnov, E. L. The Theory of Sex Allocation (Princeton Univ. Press, 1982)
Schindler, S., Neuhaus, P., Gaillard, J.‐M. & Coulson, T. The influence of nonrandom mating on population growth. Am. Nat. 182, 28–41 (2013)
Gedir, J. V. & Michener, G. R. Litter sex ratios in Richardsons ground squirrels: long‐term data support random sex allocation and homeostasis. Oecologia 174, 1225–1239 (2014)
Traill, L. W., Schindler, S. & Coulson, T. Demography, not inheritance, drives phenotypic change in hunted bighorn sheep. Proc. Natl Acad. Sci. USA 111, 13223–13228 (2014)
Blanchard, P., Festa‐Bianchet, M., Gaillard, J.‐M. & Jorgenson, J. T. Maternal condition and offspring sex ratio in polygynous ungulates: a case study of bighorn sheep. Behav. Ecol. 16, 274–279 (2005)
Martin, J. G. A. & Festa‐Bianchet, M. Sex ratio bias and reproductive strategies: what sex to produce when?. Ecology 92, 441–449 (2011)
Festa‐Bianchet, M. The social system of bighorn sheep: grouping patterns, kinship and female dominance rank. Anim. Behav. 42, 71–82 (1991)
Hewison, A. J. M. et al. Big mothers invest more in daughters – reversed sex allocation in a weakly polygynous mammal. Ecol. Lett. 8, 430–437 (2005)
Hewison, A. J. M. & Gaillard, J.‐M. Birth‐sex ratios and local resource competition in roe deer, Capreolus capreolus. Behav. Ecol. 7, 461–464 (1996)
Jones, P. H., Van Zant, J. L. & Dobson, F. S. Variation in reproductive success of male and female Columbian ground squirrels (Urocitellus columbianus). Can. J. Zool. 90, 736–743 (2012)
Murie, J. O. Mating behavior of Columbian ground squirrels. I. Multiple mating by females and multiple paternity. Can. J. Zool. 73, 1819–1826 (1995)
Neuhaus, P. & Pelletier, N. Mortality in relation to season, age, sex, and reproduction in Columbian ground squirrels (Spermophilus columbianus). Can. J. Zool. 79, 465–470 (2001)
Coltman, D. W., Festa‐Bianchet, M., Jorgenson, J. T. & Strobeck, C. Age‐dependent sexual selection in bighorn rams. Proc. Biol. Sci. 269, 165–172 (2002)
Neuhaus, P. Weight comparisons and litter size manipulation in Columbian ground squirrels (Spermophilus columbianus) show evidence of costs of reproduction. Behav. Ecol. Sociobiol. 48, 75–83 (2000)
Nussey, D. H. et al. Patterns of body mass senescence and selective disappearance differ among three species of free‐living ungulates. Ecology 92, 1936–1947 (2011)
Bronson, M. T. Altitudinal variation in the life history of the golden‐mantled ground squirrel (Spermophilus lateralis). Ecology 60, 272–279 (1979)
Festa‐Bianchet, M., Gaillard, J. & Jorgenson, J. Mass‐ and density‐dependent reproductive success and reproductive costs in a capital breeder. Am. Nat. 152, 367–379 (1998)
Steiner, U. K., Tuljapurkar, S. & Coulson, T. Generation time, net reproductive rate, and growth in stage‐age‐structured populations. Am. Nat. 183, 771–783 (2014)
Edwards, A. M. & Cameron, E. Z. Forgotten fathers: paternal influences on mammalian sex allocation. Trends Ecol. Evol. 29, 158–164 (2014)
McGraw, J. B. & Caswell, H. Estimation of individual fitness from life‐history data. Am. Nat. 147, 47–64 (1996)
Acknowledgements
We thank Y. Vindenes, S. Cubaynes, S. West, R. K. Kanda, J. A. Deere, J. Barthold, M. Brouard, R. A. Pozo, and E. G. Simmonds for comments. We thank M. Festa‐Bianchet and F. Pelletier for access to Bighorn sheep data and feedback. We acknowledge the use of the University of Oxford Advanced Research Computing facility. S.S. was funded by an ERC Advanced Grant to T.C., P.N. is funded by a Swiss National Science Foundation grant (SNF 3100AO‐109816), and L.T. was funded by grants from the European Commission (Marie Curie Fellowship 254442) and the Carnegie Corporation of New York (B8749.R01).
Author information
Authors and Affiliations
Contributions
S.S., J.M.G. and T.C. conceived and designed the study. S.S. developed the models and, with S.T., derived the formulas. S.S. and T.C. wrote the manuscript. S.S., A.G. and S.T. contributed to the mathematical formulation of the model. P.N. collated data on Columbian ground squirrels. L.T. parameterized data for bighorn sheep. T.C. parameterized data for squirrels. All authors edited the manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Extended data figures and tables
Extended Data Figure 1 Mean female age at reproduction affects optimal sex allocation.
a, Slope of the difference between male and female RV as a function of the size threshold above which females reproduce. The male size threshold is fixed at 279 g. Negative values indicate a reversed Trivers–Willard effect, positive values a Trivers–Willard effect. Dashed lines indicate a type 4 effect. When the population growth rate λ is greater than 1 (growing population), increasing female age at reproduction selects towards a Trivers–Willard effect. In contrast, when the population is shrinking (λ < 1), reproducing at a later age increases fitness36 and selects towards a reversed Trivers–Willard effect with increasing female age at reproduction. b, Mean maternal (red) and paternal (blue) age at reproduction as a function of the size threshold at which females reproduce. Dashed lines indicate the range of size thresholds that cause a type 4 effect.
Extended Data Figure 2 Strength of Trivers–Willard and reversed Trivers–Willard effects in squirrels as a function of the male to female survival ratio.
The x‐axis plots the ratio of male to female survival rate (independent of size and age) to the slope of the difference between male and female reproductive value, Δva(s). Positive values indicate a Trivers–Willard effect (grey background), negative values a reversed Trivers–Willard effect (red background). The more positive (or negative) the slope of Δva(s) the more the expected sex ratio in offspring to good‐condition mothers is biased towards males (or females). Solid line, no sex differences in mortality; dashed line, strength of mate selection has been increased from ρ = 0.1 to ρ = 0.25 (see Supplementary Table 1). Points highlighted with arrows indicate the settings that are used in Fig. 2 to plot Δva(s) against maternal size s.
Extended Data Figure 3 Trade‐off between survival and reproduction in squirrels.
a, Females (red) have higher survival rates than males (blue) at all ages. b, Small females are expected to produce more offspring than small males, while large females produce less offspring than large males.
Extended Data Figure 4 Sensitivity of the reversed Trivers–Willard effect to parameter perturbations in squirrels.
When bars lie in the positive (or negative) range, then a change in parameters works towards a Trivers–Willard effect (or strengthening the reversed Trivers–Willard effect). The horizontal dashed line shows the difference in slope needed to neutralise the reversed Trivers–Willard effect. The bar above the dashed line indicates a Trivers–Willard effect (black); bars below indicate a reversed Trivers–Willard effect (red). Filled fractions of the bars indicate the contribution of change caused by parameter perturbation owing to change of female RV, and, in the white fractions, to change in male RV (see also Extended Data Fig. 5). Bars 1 to 4 show the sensitivity of the Trivers–Willard effect in squirrels to perturbations of the following parameters by 1% downwards (which corresponds to higher mortality in the sex affected): (1) female survival intercept; (2) female survival slope; (3) male survival intercept, parameter change resulted in curved Δva which we indicate with ‘TW effect type 3’ and omit the bar; (4) male survival slope. Bars from 5 to 14 show the sensitivity of the Trivers–Willard effect in squirrels to perturbations of the following parameters by 1% upwards (which corresponds to higher rates in the affected sex): (5) female growth (mean intercept); (6) female growth (mean slope); (7) female growth variance; (8) male growth (mean intercept); (9) male growth (mean slope); (10) male growth variance; (11) inheritance (mean intercept); (12) inheritance (mean slope); (13) inheritance variance; (14) expected offspring number. All parameters are listed in Supplementary Table 1.
Extended Data Figure 5 Female (red) and male (blue) variance in RV in original model (bar 0) and when parameters are perturbed (bars 1–14) in squirrels.
Number 1 above bars indicates a Trivers–Willard effect, number 2 a reversed Trivers–Willard effect, and number 3 a type 3 Trivers–Willard effect. Bars 1 to 4 show variances in RV when the survival parameters are perturbed by 1% downwards (which corresponds to higher mortality in the affected sex): (1) female survival intercept; (2) female survival slope; (3) male survival intercept; and (4) male survival slope. Bars 5 to 14 show variances in RV when the following parameters are perturbed by 1% upwards (which corresponds to higher rates in the affected sex): (5) female growth (mean intercept); (6) female growth (mean slope); (7) female growth variance; (8) male growth (mean intercept); (9) male growth (mean slope); (10) male growth variance; (11) inheritance (mean intercept); (12) inheritance (mean slope); (13) inheritance variance; and (14) expected offspring number. All parameters are listed in Supplementary Table 1.
Extended Data Figure 6 Reproductive value (RV) of female (solid line) and male (dashed line) offspring in bighorn sheep.
For small mothers, daughters have higher RV than sons. For large mothers, sons have higher RV than daughters. We scaled the RV of females and males such that the female RV of the smallest reproductive size class is 1.
Extended Data Figure 7 Sensitivity of the Trivers–Willard effect to parameter perturbations in sheep.
Bars in the positive range indicate that the Trivers–Willard effect is strengthened; bars in the negative range indicate a weakened Trivers–Willard effect. The horizontal dotted line marks the sensitivity needed to reverse the Trivers–Willard effect. The parameters are: 1–8 female survival (2 parameters each for the stages lamb, yearling, adult, and senescent); 9–16 male survival (2 parameters for each stage); 17–36 female growth (5 parameters for each stage); 37–56 male growth (5 parameters for each stage); 57–60 female inheritance (inh) (2 intercepts for mean and variance, 2 slopes for female contribution to mean and variance); 61–62 male inheritance (male contributions to mean and variance); and 63–68 fecundity (fecund). All parameters are listed in Supplementary Tables 2 and 3.
Extended Data Figure 8 Sensitivity of Trivers–Willard effect to size‐specific male mortality increases of 1% in sheep.
a–d, The survival probability of each size class in each stage (lamb (a), yearling (b), adult (c), and senescent (d)) has been independently lowered by 1%. The vertical dashed black line denotes the mean body weight of male sheep in the corresponding stage. In the early stages (a and b, lamb and yearling) we find that male‐mortality increases in small size classes strengthen the Trivers–Willard effect, whereas male‐mortality increases in heavy size classes weaken the Trivers–Willard effect. In the later stages (c and d, adult and senescent), mortality increases hardly affect the Trivers–Willard effect (note that adult rams usually weigh above 60 kg).
Supplementary information
Supplementary Information
This file contains Supplementary Tables 1-3, Supplementary Text and Data and additional references. (PDF 447 kb)
Rights and permissions
About this article
Cite this article
Schindler, S., Gaillard, J., Grüning, A. et al. Sex‐specific demography and generalization of the Trivers–Willard theory. Nature 526, 249–252 (2015). https://doi.org/10.1038/nature14968
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature14968
This article is cited by
-
The Trivers-Willard Effect for Educational Investment: Evidence from an African Sample
Evolutionary Psychological Science (2023)
-
Reformulation of Trivers–Willard hypothesis for parental investment
Communications Biology (2022)
-
Parasite intensity drives fetal development and sex allocation in a wild ungulate
Scientific Reports (2020)
-
Variation in the sex ratio of pouch young and adult hairy-nosed wombats (Lasiorhinus latifrons and Lasiorhinus krefftii)
Behavioral Ecology and Sociobiology (2020)
-
Predictions of children’s emotionality from evolutionary and epigenetic hypotheses
Scientific Reports (2019)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.