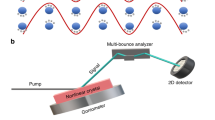
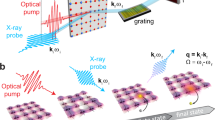
Abstract
Light–matter interactions are ubiquitous, and underpin a wide range of basic research fields and applied technologies. Although optical interactions have been intensively studied, their microscopic details are often poorly understood and have so far not been directly measurable. X-ray and optical wave mixing was proposed nearly half a century ago as an atomic-scale probe of optical interactions but has not yet been observed owing to a lack of sufficiently intense X-ray sources. Here we use an X-ray laser to demonstrate X-ray and optical sum-frequency generation. The underlying nonlinearity is a reciprocal-space probe of the optically induced charges and associated microscopic fields that arise in an illuminated material. To within the experimental errors, the measured efficiency is consistent with first-principles calculations of microscopic optical polarization in diamond. The ability to probe optical interactions on the atomic scale offers new opportunities in both basic and applied areas of science.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout



Similar content being viewed by others
References
Freund . I & Levine, B. F. Optically modulated X-ray diffraction. Phys. Rev. Lett. 25, 1241–1245 (1970)
Eisenberger, P. M. & McCall, S. L. Mixing of X-ray and optical photons. Phys. Rev. A 3, 1145–1151 (1971)
Jackson, J. D. Classical Electrodynamics (Wiley, 1975)
Arya, K. & Jha, S. S. Microscopic optical fields and mixing coefficients of x-ray and optical frequencies in solids. Pramana 2, 116–125 (1974)
Adler, S. L. Quantum theory of the dielectric constant in real solids. Phys. Rev. 126, 413–420 (1962)
Pine, A. S. Self-consistent-field theory of linear and nonlinear crystalline dielectrics including local-field effects. Phys. Rev. 139, A901–A911 (1965)
Woo, J. W. F. & Jha, S. S. Inelastic scattering of x rays from optically induced charge-density oscillations. Phys. Rev. B 6, 4081–4082 (1972)
Freund, I. Nonlinear X-ray diffraction: determination of valence electron charge distributions. Chem. Phys. Lett. 12, 583–588 (1972)
Van Vechten, J. A. &. Martin, R. M. Calculation of local effective fields: optical spectrum of diamond. Phys. Rev. Lett. 28, 446–449 (1972)
Freund, I. &. Levine, B. F. Surface effects in the nonlinear interaction of X-ray and optical fields. Phys. Rev. B 6, 3059–3060 (1973)
Arya, K. & Jha, S. S. Microscopic optical fields in diamond and germanium: molecular-orbital approach. Phys. Rev. B 10, 4485–4487 (1974)
Johnson, D. L. Local field effects and the dielectric response matrix of insulators: A model. Phys. Rev. B 9, 4475–4484 (1974)
Schweigert, I. V. & Mukamel, S. Coherent ultrafast core-hole correlation spectroscopy: X-ray analogues of multidimensional NMR. Phys. Rev. Lett. 99, 163001 (2007)
Tanaka, S. & Mukamel, S. Probing exciton dynamics using Raman resonances in femtosecond X-ray four-wave mixing. Phys. Rev. A 67, 033818 (2003)
Nazarkin, A., Podorov, S., Uschmann, I., Forster, E. & Sauerbrey, R. Nonlinear optics in the angstrom regime: hard-X-ray frequency doubling in perfect crystals. Phys. Rev. A 67, 041804(R) (2003)
Tanaka, S. & Mukamel, S. Coherent X-ray Raman spectroscopy: a nonlinear local probe for electronic excitations. Phys. Rev. Lett. 89, 043001 (2002)
Hudis, E., Shkolnikov, P. L. & Kaplan, A. E. X-ray stimulated Raman scattering in Li and He. Appl. Phys. Lett. 64, 818–820 (1994)
Freund, I. & Levine, B. F. Parametric conversion of X rays. Phys. Rev. Lett. 23, 854–857 (1969)
Eisenberger, P. & McCall, S. L. X-ray parametric conversion. Phys. Rev. Lett. 26, 684–688 (1971)
Danino, H. & Freund, I. Parametric down conversion of X rays into the extreme ultraviolet. Phys. Rev. Lett. 46, 1127–1130 (1981)
Yoda, Y., Suzuki, T., Zhang, X. W., Hirano, K. & Kikuta, S. X-ray parametric scattering by a diamond crystal. J. Synchrotron Radiat. 5, 980–982 (1998)
Adams, B. et al. Parametric down conversion of X-ray photons. J. Synchrotron Radiat. 7, 81–88 (2000)
Tamasaku, K. Sawada, K. Nishibori, E. & Ishikawa, T. Visualizing the local optical response to extreme-ultraviolet radiation with a resolution of λ/380. Nature Phys. 7, 705–708 (2011)
Platzman, P. M. & Isaacs, E. D. Resonant inelastic X-ray scattering. Phys. Rev. B 57, 11107–11114 (1998)
Kotani, A. & Shin, S. Resonant inelastic X-ray scattering spectra for electrons in solids. Rev. Mod. Phys. 73, 203–246 (2001)
Emma, P. et al. First lasing and operation of an ångstrom-wavelength free-electron laser. Nature Photon. 4, 641–647 (2010)
Palik, E. D., ed. Handbook of Optical Constants of Solids 313–334 (Academic, 1998)
Spackman, M. A. The electron distribution in diamond: a comparison between experiment and theory. Acta Crystallogr. A 47, 420–427 (1991)
Shen, Y. R. The Principles of Nonlinear Optics 5–40 (Wiley, 1984)
Phillips, J. C. Covalent bond in crystals. I. Elements of a structural theory. Phys. Rev. 166, 832–838 (1968)
Levine, B. F. Electrodynamic bond-charge calculation of nonlinear optical susceptibilities. Phys. Rev. Lett. 22, 787–790 (1969)
Giannozzi, P. et al. Quantum Espresso: a modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Matter 21, 395502 (2009)
Souza, I. Iniguez, J. & Vanderbilt, D. First principles approach to insulators in finite electric fields. Phys. Rev. Lett. 89, 117602 (2002)
Ihee, H. et al. Ultrafast X-ray diffraction of transient molecular structures in solution. Science 309, 1223–1227 (2005)
Möhr-Vorobeva, E. et al. Nonthermal melting of a charge density wave in TiSe2 . Phys. Rev. Lett. 107, 036403 (2011)
Lindenberg, A. M. et al. Time-resolved X-ray diffraction from coherent phonons during a laser-induced phase transition. Phys. Rev. Lett. 84, 111–114 (2000)
Cavalleri, A. et al. Tracking the motion of charges in a terahertz light field by femtosecond X-ray diffraction. Nature 442, 664–666 (2006)
Fritz, D. M. et al. Ultrafast bond softening in bismuth: mapping a solid’s interatomic potential with X-rays. Science 315, 633–636 (2007)
Wu, J. S., Spence, J. C. H., O’Keeffe, M. & Groy, T. L. Application of a modified Oszlanyi and Suto ab initio charge-flipping algorithm to experimental data. Acta Crystallogr. A 60, 326–330 (2004)
Coppens, P. X-Ray Charge Densities and Chemical Bonding (Oxford Univ. Press, 1997)
Acknowledgements
We thank J. D. Jackson, D. Vanderbilt, and J. C. H. Spence for commenting on various aspects of this work. T.E.G. was supported by the Office of Science, Office of Basic Energy Sciences, of the US Department of Energy under contract no. DE-AC02-05CH11231. The work of S.E.H. and S.S. was supported by the US Air Force Office of Scientific Research and the US Army Research Office. D.A.R. and S.G. were supported as part of the AMOS programme within the Chemical Sciences, Geosciences, and Biosciences Division, Office of Basic Energy Sciences, US Department of Energy. Portions of this research were carried out at the LCLS at SLAC National Accelerator Laboratory. LCLS is an Office of Science User Facility operated for the US Department of Energy Office of Science by Stanford University. Preliminary experiments were performed at the Advanced Light Source at Lawrence Berkeley National Laboratory.
Author information
Authors and Affiliations
Contributions
T.E.G. and J.B.H. had the idea for the x-ray/optical SFG project. D.M.F., T.E.G., J.B.H., M.C., T.K.A., J.M.F. and D.A.R. contributed to the experiment design. D.M.F., M.C., H.L., D.Z. and R.N.C. were responsible for the X-ray pump–probe instrument and the optical laser. D.M.F., M.C., T.E.G., J.B.H., H.L., D.Z., T.K.A., J.M.F., Y.F., R.N.C., D.A.R., S.S., M.F., S.G. and J.C. collected data. S.E.H., S.S., T.E.G. and T.K.A. contributed to the wave equation calculations. Data analysis was done by T.E.G., H.L. and J.M.F. Construction of the bond charge model was done by T.E.G. Density functional theory calculations were done by S.C. T.E.G. wrote the manuscript. All authors contributed to the work presented here and to the final paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Supplementary information
Supplementary Information
This file contains Supplementary Text and Data 1-7, Supplementary Figure 1 and additional references (see page 1 for more details). (PDF 1636 kb)
Rights and permissions
About this article
Cite this article
Glover, T., Fritz, D., Cammarata, M. et al. X-ray and optical wave mixing. Nature 488, 603–608 (2012). https://doi.org/10.1038/nature11340
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature11340
This article is cited by
-
Remote focused encoding and decoding of electric fields through acoustoelectric heterodyning
Communications Physics (2023)
-
Emerging ultrafast techniques for studying quantum materials
Nature Reviews Materials (2023)
-
Progress and prospects in nonlinear extreme-ultraviolet and X-ray optics and spectroscopy
Nature Reviews Physics (2023)
-
Using X-ray free-electron lasers for spectroscopy of molecular catalysts and metalloenzymes
Nature Reviews Physics (2021)
-
Non-linear self-driven spectral tuning of Extreme Ultraviolet Femtosecond Pulses in monoatomic materials
Light: Science & Applications (2021)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.