Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

Coherent zero-state and π-state in an exciton–polariton condensate array

Nature volume 450, pages 529–532 (2007)Cite this article

Abstract

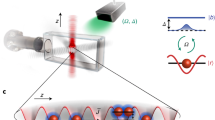
The effect of quantum statistics in quantum gases and liquids results in observable collective properties among many-particle systems. One prime example is Bose–Einstein condensation, whose onset in a quantum liquid leads to phenomena such as superfluidity and superconductivity. A Bose–Einstein condensate is generally defined as a macroscopic occupation of a single-particle quantum state, a phenomenon technically referred to as off-diagonal long-range order due to non-vanishing off-diagonal components of the single-particle density matrix1,2,3. The wavefunction of the condensate is an order parameter whose phase is essential in characterizing the coherence and superfluid phenomena4,5,6,7,8,9,10,11. The long-range spatial coherence leads to the existence of phase-locked multiple condensates in an array of superfluid helium12, superconducting Josephson junctions13,14,15 or atomic Bose–Einstein condensates15,16,17,18. Under certain circumstances, a quantum phase difference of π is predicted to develop among weakly coupled Josephson junctions19. Such a meta-stable π-state was discovered in a weak link of superfluid 3He, which is characterized by a ‘p-wave’ order parameter20. The possible existence of such a π-state in weakly coupled atomic Bose–Einstein condensates has also been proposed21, but remains undiscovered. Here we report the observation of spontaneous build-up of in-phase (‘zero-state’) and antiphase (‘π-state’) ‘superfluid’ states in a solid-state system; an array of exciton–polariton condensates connected by weak periodic potential barriers within a semiconductor microcavity. These in-phase and antiphase states reflect the band structure of the one-dimensional polariton array and the dynamic characteristics of metastable exciton–polariton condensates.

This is a preview of subscription content, access via your institution

Relevant articles

Open Access articles citing this article.

Access options

Rent or buy this article

Get just this article for as long as you need it

$39.95

Learn more

Prices may be subject to local taxes which are calculated during checkout

Figure 1: Formation of an exciton–polariton array.
Figure 2: Imaging and spectroscopy of exciton–polariton distribution in coordinate and momentum space.
Figure 3: Band structure and ‘superfluid’ states in an exciton–polariton condensate array.
Figure 4: Evolution of the ‘zero-state’ and ‘π-state’.

References

  1. Penrose, O. & Onsager, L. Bose-Einstein condensation and liquid helium. Phys. Rev. 104, 576–584 (1956)

    Article  ADS  CAS  Google Scholar 

  2. Beliaev, S. T. Application of the methods of quantum field theory to a system of bosons. J. Exp. Theor. Phys. 34, 417–432 (1958)

    Google Scholar 

  3. Yang, C. N. Concept of off-diagonal long-range order and the quantum phases of liquid He and of superconductors. Rev. Mod. Phys. 34, 694–704 (1962)

    Article  ADS  MathSciNet  CAS  Google Scholar 

  4. Tilley, D. R. & Tilley, J. Superfluidity and Superconductivity Chs 1–3, and 7 (Adam Hilger, New York, 1990)

    MATH  Google Scholar 

  5. Pitaevskii, L. P. & Stringari, S. Bose-Einstein Condensation (Clarendon, Oxford, 2003)

    MATH  Google Scholar 

  6. Leggett, A. J. Quantum Liquids: Bose Condensation and Cooper Pairing in Condensed-matter Systems Chs 1–4 (Oxford Univ. Press, Oxford, 2006)

    Book  Google Scholar 

  7. Andrews, M. R. et al. Observation of interference between two Bose condensates. Science 275, 637–641 (1997)

    Article  CAS  Google Scholar 

  8. Hagley, E. W. et al. Measurement of the coherence of a Bose-Einstein condensate. Phys. Rev. Lett. 83, 3112–3115 (1999)

    Article  ADS  CAS  Google Scholar 

  9. Bloch, I., Hansch, T. W. & Esslinger, T. Measurement of the spatial coherence of a trapped Bose gas at the phase transition. Nature 403, 166–170 (2000)

    Article  ADS  CAS  Google Scholar 

  10. Castin, Y. & Dalibard, J. Relative phase of two Bose-Einstein condensates. Phys. Rev. A. 55, 4330–4337 (1997)

    Article  ADS  CAS  Google Scholar 

  11. Naraschewski, M. & Glauber, R. J. Spatial coherence and density correlations of trapped Bose gases. Phys. Rev. A. 59, 4595–4607 (1999)

    Article  ADS  CAS  Google Scholar 

  12. Davis, J. C. & Packard, R. E. Superfluid 3He Josephson weak links. Rev. Mod. Phys. 74, 741–773 (2002)

    Article  ADS  CAS  Google Scholar 

  13. Hansen, J. B. & Lindelof, P. E. Static and dynamic interactions between Josephson junctions. Rev. Mod. Phys. 56, 431–459 (1984)

    Article  ADS  Google Scholar 

  14. Hadley, P. M., Beasley, R. & Wiesenfeld, K. Phase locking of Josephson-junction series arrays. Phys. Rev. B 38, 8712–8719 (1988)

    Article  ADS  CAS  Google Scholar 

  15. Cataliotti, F. S. et al. Josephson junction arrays with Bose-Einstein condensates. Science 293, 843–846 (2001)

    Article  ADS  CAS  Google Scholar 

  16. Anderson, B. P. & Kasevich, M. A. Macroscopic quantum interference from atomic tunnel arrays. Science 282, 1686–1689 (1998)

    Article  ADS  CAS  Google Scholar 

  17. Orzel, C. et al. Squeezed states in a Bose-Einstein condensate. Science 291, 2386–2389 (2001)

    Article  ADS  CAS  Google Scholar 

  18. Greiner, M. et al. Quantum phase transition from a superfluid to a Mott insulator in a gas of ultracold atoms. Nature 415, 39–44 (2002)

    Article  ADS  CAS  Google Scholar 

  19. Bulaevskii, L. N., Kuzii, V. V. & Sobyanin, A. A. Superconducting system with weak coupling to current in ground state. JETP Lett. 25, 290–294 (1977)

    ADS  Google Scholar 

  20. Backhaus, S. et al. Discovery of a metastable pi-state in a superfluid 3He weak link. Nature 392, 687–690 (1998)

    Article  ADS  CAS  Google Scholar 

  21. Smerzi, A. et al. Quantum coherent atomic tunneling between two trapped Bose-Einstein condensates. Phys. Rev. Lett. 79, 4950–4953 (1997)

    Article  ADS  CAS  Google Scholar 

  22. Weisbuch, C. et al. Observation of the coupled exciton-photon mode splitting in a semiconductor quantum microcavity. Phys. Rev. Lett. 69, 3314–3317 (1992)

    Article  CAS  Google Scholar 

  23. Houdre, R. et al. Measurement of cavity-polariton dispersion curve from angle-resolved photoluminescence experiments. Phys. Rev. Lett. 73, 2043–2046 (1994)

    Article  ADS  CAS  Google Scholar 

  24. Deng, H. et al. Polariton lasing versus photon lasing in a semiconductor microcavity. Proc. Natl Acad. Sci. USA 100, 15318–15323 (2003)

    Article  ADS  CAS  Google Scholar 

  25. Deng, H. et al. Quantum degenerate exciton-polaritons in thermal equilibrium. Phys. Rev. Lett. 97, 146402 (2006)

    Article  ADS  Google Scholar 

  26. Kasprzak, J. et al. Bose-Einstein condensation of exciton polaritons. Nature 443, 409–414 (2006)

    Article  ADS  CAS  Google Scholar 

  27. Yeh, P. Optical Waves in Layered Media Ch. 5 (John Wiley, Hoboken, 2005)

    Google Scholar 

  28. Pedri, P. et al. Expansion of a coherent array of Bose-Einstein condensates. Phys. Rev. Lett. 87, 220401 (2001)

    Article  ADS  CAS  Google Scholar 

  29. Madelung, O. Introduction to Solid-state Theory Ch. 2 (Springer, Berlin, 1996)

    Google Scholar 

Download references

Acknowledgements

This work was supported by the JST/SORST programme and by Special Coordination Funds for Promoting Science and Technology in Japan. The high-quality microcavity cavity sample is courtesy of G. S. Solomon, R. Hey, K. Ploog and A. Forchel. We thank T. Maruyama for support and S. Sasaki for the device fabrication. C.W.L. thanks Y. R. Shen for comments and discussions.

Author information

Authors and Affiliations

  1. E. L. Ginzton Laboratory, Stanford University, Stanford, California 94305, USA ,

    C. W. Lai, N. Y. Kim, G. Roumpos, H. Deng, M. D. Fraser, P. Recher & Y. Yamamoto

  2. Institute of Industrial Science, University of Tokyo, Meguro-ku, Tokyo 153-8505, Japan ,

    C. W. Lai, N. Y. Kim, T. Byrnes & P. Recher

  3. National Institute of Informatics, Hitotsubashi, Chiyoda-ku, Tokyo 101-8430, Japan ,

    C. W. Lai, S. Utsunomiya, T. Byrnes & Y. Yamamoto

  4. NTT basic research laboratories, NTT Corporation, Atugi, Kanagawa 243-0198, Japan ,

    S. Utsunomiya, N. Kumada & T. Fujisawa

Authors
  1. C. W. Lai
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. N. Y. Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. S. Utsunomiya
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. G. Roumpos
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. H. Deng
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. M. D. Fraser
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. T. Byrnes
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. P. Recher
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. N. Kumada
    View author publications

    You can also search for this author in PubMed Google Scholar

  10. T. Fujisawa
    View author publications

    You can also search for this author in PubMed Google Scholar

  11. Y. Yamamoto
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to C. W. Lai or Y. Yamamoto.

Supplementary information

Supplementary Information

The file contains Supplementary Notes with Supplementary Figures S1-S6 and additional references. The document describes the signatures of exciton-polariton condensation without a periodically modulated potential, focusing on the spatial coherence properties and condensation in momentum space. The characteristics of the exciton-polariton condensate form the basis of the study of the exciton-polariton condensate array. We also discuss the diffraction patterns of ‘zero-state’ and ‘π-state’ considering the finite transmittance through the metallic strips and spatial coherence. A rate-equation model is used to describe the dynamics and evolution of the ‘zero-state’ and ‘π-state’ as a function of the pumping rate. (PDF 3416 kb)

Rights and permissions

Reprints and Permissions

About this article

Cite this article

Lai, C., Kim, N., Utsunomiya, S. et al. Coherent zero-state and π-state in an exciton–polariton condensate array. Nature 450, 529–532 (2007). https://doi.org/10.1038/nature06334

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1038/nature06334

This article is cited by

Comments

By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing