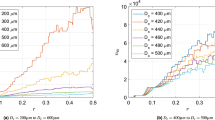
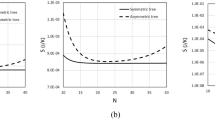
Abstract
The geometry and dimensions of branched structures such as blood vessels or airways are important factors in determining the efficiency of physiological processes. It has been shown that fractal trees can be space filling1 and can ensure minimal dissipation2,3,4. The bronchial tree of most mammalian lungs is a good example of an efficient distribution system with an approximate fractal structure5,6. Here we present a study of the compatibility between physical optimization and physiological robustness in the design of the human bronchial tree. We show that this physical optimization is critical in the sense that small variations in the geometry can induce very large variations in the net air flux. Maximum physical efficiency therefore cannot be a sufficient criterion for the physiological design of bronchial trees. Rather, the design of bronchial trees must be provided with a safety factor and the capacity for regulating airway calibre. Paradoxically, our results suggest that bronchial malfunction related to asthma is a necessary consequence of the optimized efficiency of the tree structure.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Mandelbrot, B. The Fractal Geometry of Nature (W. H. Freeman, San Francisco, CA, 1982)
West, G. B., Brown, J. H. & Enquist, B. J. A general model for the origin of allometric scaling laws in biology. Science 276, 122–126 (1997)
Brown, J. H., West, G. B. & Enquist, B. J. Scaling in Biology (Oxford Univ. Press, Oxford, UK, 2000)
Bejan, A. Shape and Structure, From Engineering to Nature (Cambridge Univ. Press, Cambridge, UK, 2000)
Nelson, T. R. & Manchester, D. K. Modeling of lung morphogenesis using fractal geometries. IEEE Trans. Med. Imaging 7, 321–327 (1988)
West, B. J., Barghava, V. & Goldberger, A. L. Beyond the principle of similitude: renormalization in the bronchial tree. J. Appl. Physiol. 60, 1089–1097 (1986)
Weibel, E. R. The Pathway for Oxygen (Harvard Univ. Press, Cambridge, MA, 1984)
Mauroy, B., Filoche, M., Andrade, J. S. & Sapoval, B. Interplay between geometry and flow distribution in an airway tree. Phys. Rev. Lett. 90, 1–4 (2003)
Hess, W. R. Das Prinzip des kleinsten Kraftverbrauchs im Dienste hämodynamischer Forschung. Archiv Anat. Physiol. 1914, 1–62 (1914)
Murray, C. D. The physiological principle of minimum work. I. The vascular system and the cost of blood. Proc. Natl Acad. Sci. USA 12, 207–214 (1926)
Weibel, E. R. in The Lung: Scientific Foundations 2nd edn Vol. 1 (eds Crystal, R. G., West, J. B., Weibel, E. R. & Barnes, P. J.) 1061–1071 (Lippincott-Raven, Philadelphia, PA, 1997)
Que, C. L., Kenyon, C. M., Olivenstein, R., Macklem, P. T. & Maksym, G. N. Homeokinesis and short-term variability of human airway caliber. J. Appl. Physiol. 91, 1131–1141 (2001)
Sapoval, B. Universalités et Fractales (Flammarion, Paris, 1997)
Kitaoka, H., Ryuji, T. & Suki, B. A three-dimensional model of the human airway tree. J. Appl. Physiol. 87, 2207–2217 (1999)
Sapoval, B., Filoche, M. & Weibel, E. R. Smaller is better—but not too small: a physical scale for the design of the mammalian pulmonary acinus. Proc. Natl Acad. Sci. USA 99, 10411–10416 (2002)
Wilber, R. L. et al. Incidence of exercise-induced bronchospasm in Olympic winter sport athletes. Med. Sci. Sports Exerc. 32, 732–737 (2000)
Weibel, E. R. Symmorphosis (Harvard Univ. Press, Cambridge, MA, 2000)
Hoppeler, H. & Fluck, M. Plasticity of skeletal muscle mitochondria: structure and function. Med. Sci. Sports Exerc. 35, 95–104 (2003)
Weibel, E. R. Morphometry of the Human Lung (Springer, Berlin, 1963)
Acknowledgements
The authors wish to thank J. M. Morel and M. Bernot for useful discussions.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing financial interests.
Rights and permissions
About this article
Cite this article
Mauroy, B., Filoche, M., Weibel, E. et al. An optimal bronchial tree may be dangerous. Nature 427, 633–636 (2004). https://doi.org/10.1038/nature02287
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature02287
This article is cited by
-
Water and heat exchanges in mammalian lungs
Scientific Reports (2023)
-
Asymmetric lung increases particle filtration by deposition
Scientific Reports (2023)
-
Historicity at the heart of biology
Theory in Biosciences (2022)
-
Theory of branching morphogenesis by local interactions and global guidance
Nature Communications (2021)
-
Pseudo-Darwinian evolution of physical flows in complex networks
Scientific Reports (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.