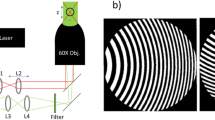
Abstract
Optical tweezers1 are commonly used for manipulating microscopic particles, with applications in cell manipulation2, colloid research3,4,5, manipulation of micromachines6 and studies of the properties of light beams7. Such tweezers work by the transfer of momentum from a tightly focused laser to the particle, which refracts and scatters the light and distorts the profile of the beam. The forces produced by this process cause the particle to be trapped near the beam focus. Conventional tweezers use gaussian light beams, which cannot trap particles in multiple locations more than a few micrometres apart in the axial direction, because of beam distortion by the particle and subsequent strong divergence from the focal plane. Bessel beams8,9, however, do not diverge and, furthermore, if part of the beam is obstructed or distorted the beam reconstructs itself after a characteristic propagation distance10. Here we show how this reconstructive property may be utilized within optical tweezers to trap particles in multiple, spatially separated sample cells with a single beam. Owing to the diffractionless nature of the Bessel beam, secondary trapped particles can reside in a second sample cell far removed (∼3 mm) from the first cell. Such tweezers could be used for the simultaneous study of identically prepared ensembles of colloids and biological matter, and potentially offer enhanced control of ‘lab-on-a-chip’ and optically driven microstructures.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Ashkin, A., Dziedzic, J. M., Bjorkholm, J. E. & Chu, S. Observation of a single-beam gradient force optical trap for dielectric particles. Opt. Lett. 11, 288–290 (1986)
Smith, S. B., Cui, Y. & Bustamante, C. Overstretching B-DNA: The elastic response of individual double strained and single stranded DNA molecules. Science 271, 795–799 (1996)
Crocker, J. C. & Grier, D. G. Methods of digital video microscopy for collodial studies. J. Colloid Interf. Sci. 179, 298–310 (1996)
Crocker, J. C., Matteo, J. A., Dinsmore, A. D. & Yodh, A. G. Entropic attraction and repulsion in binary colloids probed with a line optical tweezer. Phys. Rev. Lett. 82, 4352–4355 (1999)
Larsen, A. E. & Grier, D. G. Like charge attractions in metastable colloidal crystallites. Nature 385, 230–233 (1997)
Friese, M. E. J., Rubinsztein-Dunlop, H., Gold, J., Hagberg, P. & Hanstorp, D. Optically driven micromachine elements. Appl. Phys. Lett. 78, 547–549 (2001)
Volke-Sepulveda, K., Garcés-Chávez, V., Chávez-Cerda, S., Arlt, J. & Dholakia, K. Orbital angular momentum of a high-order Bessel light beam. J. Opt. B 4, 582–589 (2002)
Durnin, J., Miceli, J. J. Jr & Eberly, J. H. Diffraction-free beams. Phys. Rev. Lett. 58, 1499–1501 (1987)
McQueen, C. A., Arlt, J. & Dholakia, K. An experiment to study a “nondiffracting” light beam. Am. J. Phys. 67, 912–915 (1999)
Bouchal, Z., Wagner, J. & Chlup, M. Self-reconstruction of a distorted nondiffracting beam. Opt. Commun. 151, 207–211 (1998)
Durnin, J. Exact solutions for nondiffracting beams. I. The scalar theory. J. Opt. Soc. Am. A 4, 651–654 (1987)
Herman, R. M. & Wiggins, T. A. Production and uses of diffractionless beams. J. Opt. Soc. Am. A 8, 932–942 (1991)
MacDonald, R. P., Boothroyd, S. A., Okamato, T., Chrostowski, J. & Syrett, B. A. Interboard optical data distribution by Bessel beam shadowing. Opt. Commun. 122, 169–177 (1996)
Chávez-Cerda, S. et al. Holographic generation and orbital angular momentum of high-order Mathieu beams. J. Opt. B 4, S52–S57 (2002)
Curtis, J. E., Koss, B. A. & Grier, D. G. Dynamic holographic optical tweezers. Opt. Commun. 207, 169–175 (2002)
Arlt, J., Garcés-Chávez, V., Sibbett, W. & Dholakia, K. Optical micro-manipulation using a Bessel light beam. Opt. Commun. 197, 239–245 (2001)
Friese, M. E. J., Nieminen, T. A., Heckenberg, N. R. & Rubinsztein-Dunlop, H. Alignment or spinning of laser-trapped microscopic waveplates. Nature 394, 348–350 (1998)
Paterson, L. et al. Controlled rotation of optically trapped microscopic particles. Science 292, 912–914 (2001)
MacDonald, M. P. et al. Creation and manipulation of three-dimensional optically trapped structures. Science 296, 1101–1103 (2002)
Korda, P., Spalding, G. C., Dufresne, E. R. & Grier, D. G. Nanofabrication with holographic optical tweezers. Rev. Sci. Instrum. 73, 1956–1957 (2002)
Acknowledgements
We thank G. Spalding for discussions. This work was supported by the Leverhulme Trust, the UK Engineering and Physical Sciences Research Council and the Medical Research Council.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing financial interests.
Rights and permissions
About this article
Cite this article
Garcés-Chávez, V., McGloin, D., Melville, H. et al. Simultaneous micromanipulation in multiple planes using a self-reconstructing light beam. Nature 419, 145–147 (2002). https://doi.org/10.1038/nature01007
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/nature01007
This article is cited by
-
Tight focusing Lorenz–Gaussian vortex beams modulated by power order space-variant phase
Optical and Quantum Electronics (2024)
-
Distortion of Bessel Gaussian beams caused by atmospheric thermal blooming effect
Optical and Quantum Electronics (2024)
-
On-chip generation of Bessel–Gaussian beam via concentrically distributed grating arrays for long-range sensing
Light: Science & Applications (2023)
-
Fresnel diffraction of Humbert beam of type-II by a helical axicon
Optical and Quantum Electronics (2023)
-
Generation of elliptic helical Mathieu optical vortices
Applied Physics B (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.