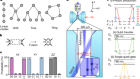
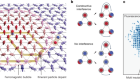
- NEWS AND VIEWS
A traffic jam of light
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Rent or buy this article
Prices vary by article type
from$1.95
to$39.95
Prices may be subject to local taxes which are calculated during checkout
Nature 566, 45-46 (2019)
doi: https://doi.org/10.1038/d41586-019-00348-x
References
Ma, R. et al. Nature 566, 51–57 (2019).
Kapit, E. Quantum Sci. Technol. 2, 033002 (2017).
Shankar, S. et al. Nature 504, 419–422 (2013).
Lin, Y. et al. Nature 504, 415–418 (2013)
Barreiro, J. T. et al. Nature 470, 486–491 (2011).
Fitzpatrick, M., Sundaresan, N. M., Li, A. C. Y., Koch, J. & Houck, A. A. Phys. Rev. X 7, 011016 (2017).
Potočnik, A. et al. Nature Commun. 9, 904 (2018).
Hacohen-Gourgy, S., Ramasesh, V. V., De Grandi, C., Siddiqi, I. & Girvin, S. M. Phys. Rev. Lett. 115, 240501 (2015).
Kapit, E., Hafezi, M. & Simon, S. H. Phys. Rev. X 4, 031039 (2014).
Bakr, W. S. et al. Science 329, 547–550 (2010).

 Read the paper: A dissipatively stabilized Mott insulator of photons
Read the paper: A dissipatively stabilized Mott insulator of photons
 Novel electronic states seen in graphene
Novel electronic states seen in graphene
 Superconducting electrons go missing
Superconducting electrons go missing