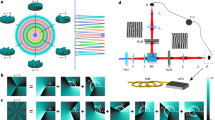
Abstract
Entangled quantum states are not separable, regardless of the spatial separation of their components. This is a manifestation of an aspect of quantum mechanics known as quantum non-locality1,2. An important consequence of this is that the measurement of the state of one particle in a two-particle entangled state defines the state of the second particle instantaneously, whereas neither particle possesses its own well-defined state before the measurement. Experimental realizations of entanglement have hitherto been restricted to two-state quantum systems3,4,5,6, involving, for example, the two orthogonal polarization states of photons. Here we demonstrate entanglement involving the spatial modes of the electromagnetic field carrying orbital angular momentum. As these modes can be used to define an infinitely dimensional discrete Hilbert space, this approach provides a practical route to entanglement that involves many orthogonal quantum states, rather than just two Multi-dimensional entangled states could be of considerable importance in the field of quantum information7,8, enabling, for example, more efficient use of communication channels in quantum cryptography9,10,11.
This is a preview of subscription content, access via your institution
Access options
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Schrödinger, E. Die gegenwärtige Situation in der Quantenmechanik. Naturwissenschaften 23, 807–812; 823–828; 844–849 (1935).
Schrödinger, E. Discussion of probability relations between separated systems. Proc. Camb. Phil. Soc. 31, 555–563 (1935).
Bouwmeester, D., Pan, J.-W., Daniell, M., Weinfurter, H. & Zeilinger, A. Observation of a three-photon Greenberger-Horne-Zeilinger state. Phys. Rev. Lett. 82, 1345–1349 (1999).
Pan, J.-W., Bouwmeester, D., Daniell, M., Weinfurter, H. & Zeilinger, A. Experimental test of quantum nonlocality in three-photon Greenberger-Horne-Zeilinger entanglement. Nature 403, 515–519 (2000).
Sackett, C. A. et al. Experimental entanglement of four particles. Nature 404, 256–259 (2000).
Pan, J.-W., Daniell, M., Gasparoni, S., Weihs, G. & Zeilinger, A. Experimental demonstration of four-photon entanglement and high-fidelity teleportation. Phys. Rev. Lett. 86, 4435–4438 (2001).
DiVincenzo, D. P., More, T., Shor, P. W., Smolin, J. A. & Terhal, B. M. Unextendible product bases, uncompletable product bases and bound entanglement. Preprint quant-ph/9908070 at 〈http://xxx.lanl.gov〉 (1999).
Bartlett, S. D., de Guise, H. & Sanders, B. C. Quantum computation with harmonic oscillators. Preprint quant-ph/0011080 at 〈http://xxx.lanl.gov〉 (2000).
Bechmann-Pasquinucci, H. & Peres, A. Quantum cryptography with 3-state systems. Phys. Rev. Lett. 85, 3313–3316 (2000).
Bechmann-Pasquinucci, H. & Tittel, W. Quantum cryptography using larger alphabets. Phys. Rev. A 61, 62308–62313 (2000).
Bourennane, M., Karlsson, A. & Björk, G. Quantum key distribution using multilevel encoding. Phys. Rev. A (in the press).
Reck, M., Zeilinger, A., Bernstein, H. J. & Bertani, P. Experimental realization of any discrete unitary operator. Phys. Rev. Lett. 73, 58–61 (1994).
Zukowski, M., Zeilinger, A. & Horne, M. Realizable higher-dimensional two-particle entanglements via multiport beam splitters. Phys. Rev. A 55, 2564–2579 (1997).
Reck, M. Quantum Interferometry with Multiports: Entangled Photons in Optical Fibers. Thesis, Univ. Innsbruck (1996).
Arnaut, H. H. & Barbosa, G. A. Orbital and angular momentum of single photons and entangled pairs of photons generated by parametric down-conversion. Phys. Rev. Lett. 85, 286–289 (2000).
Franke-Arnold, S., Barnett, S. M., Padgett, M. J. & Allen, L. Two-photon entanglement of orbital angular momentum states. Phys. Rev. A (in the press).
Allen, L., Beijersbergen, M. W., Spreeuw, R. J. C. & Woerdman, J. P. Orbital angular momentum of light and the transformation of laguerre-gaussian laser modes. Phys. Rev. A 45, 8185–8189 (1992).
He, H., Fries, M., Heckenberg, N. & Rubinsztein-Dunlop, H. Direct observation of transfer of angular momentum to absorbtive particles from a laser beam with a phase singularity. Phys. Rev. Lett. 75, 826–829 (1995).
Simpson, N. B., Dholakia, K., Allen, L. & Padgett, M. J. Mechanical equivalence of spin and orbital angular momentum of light: An optical spanner. Opt. Lett. 22, 52–54 (1997).
Galajda, P. & Ormos, P. Complex micromachines produced and driven by light. Appl. Phys. Lett. 78, 249–251 (2001).
Friese, M. E. J., Enger, J., Rubinsztein-Dunlop, H. & Heckenberg, N. Optical angular-momentum transfer to trapped absorbing particles. Phys. Rev. A 54, 1593–1596 (1996).
Bejersbergen, M. W., Allen, L., van der Veen, H. E. L. O. & Woerdman, J. P. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 96, 123–132 (1993).
Arlt, J., Dholakia, K., Allen, L. & Padgett, M. J. The production of multiringed laguerre-gaussian modes by computer-generated holograms. J. Mod. Opt. 45, 1231–1237 (1998).
Arlt, J., Dholakia, K., Allen, L. & Padgett, M. Parametric down-conversion for light beams possessing orbital angular momentum. Phys. Rev. A 59, 3950–3952 (1999).
Kaszlikowski, D., Gnacinski, P., Zukowski, M., Miklaszewski, W. & Zeilinger, A. Violation of local realism by two entangled n-dimensional systems are stronger than for two qubits. Phys. Rev. Lett. 85, 4418–4421 (2000).
Acknowledgements
This work was supported by the Austrian Fonds zur Förderung der wissenschaftlichen Forschung (FWF).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Mair, A., Vaziri, A., Weihs, G. et al. Entanglement of the orbital angular momentum states of photons. Nature 412, 313–316 (2001). https://doi.org/10.1038/35085529
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1038/35085529
This article is cited by
-
Qubit gate operations in elliptically trapped polariton condensates
Scientific Reports (2024)
-
Ultrafast all-optical second harmonic wavefront shaping
Nature Communications (2024)
-
Generation of optical vortices by flat-topped beam diffracted with a radial phase shift spiral zone plate
Optical and Quantum Electronics (2024)
-
Induced turbulence in the quantum channel of high dimensional QKD system using structured light
Applied Physics B (2024)
-
Arbitrarily structured quantum emission with a multifunctional metalens
eLight (2023)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.