Abstract
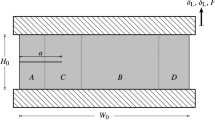
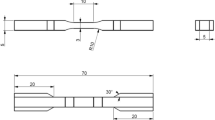
IT may be shown theoretically that when plane mechanical pulses of finite amplitude travel in non-dispersive media, the velocity of propagation in space is given by c + V where c = (S/ρ)1/2, S being the tangent modulus of the material and ρ its density, and V is the particle velocity associated with the pulse. If S increases with increasing amplitude of deformation, the head of the pulse will become steeper as it travels through the medium and it will eventually become a shock front, the gradient of which is limited by dissipative processes, such as internal friction and thermal conductivity. Such compressive shock waves are well known in fluids and in recent years similar shock waves have been produced and studied in blocks of solids1.
Similar content being viewed by others
Article PDF
References
Duvall, G. E., Appl. Mech. Rev., 15, 849 (1962).
Kolsky, H., Stress Waves in Solids (Clarendon Press, Oxford, 1953; Dover reprint, 1964).
Hillier, K. W., Trans. Inst. Rubber Industry, 26, 64 (1950).
Lee, E. H., and Tupper, S. J., Applied Mechanics, 21, 63 (1954).
Mason, P., Proc. Roy. Soc., 273, A, 315 (1963).
Efron, L., and Malvern, L. E., Experimental Mechanics, 255 (1969).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
KOLSKY, H. Production of Tensile Shock Waves in Stretched Natural Rubber. Nature 224, 1301 (1969). https://doi.org/10.1038/2241301a0
Received:
Issue Date:
DOI: https://doi.org/10.1038/2241301a0
This article is cited by
-
Dynamic tensile characterization of pig skin
Acta Mechanica Sinica (2014)
-
Dynamic steady-state analysis of crack propagation in rubber-like solids using an extended finite element method
Computational Mechanics (2012)
-
Numerical analysis of dynamic crack propagation in rubber
International Journal of Fracture (2012)
-
Steady-state crack growth in rubber-like solids
International Journal of Fracture (2011)
-
On hysteretic response and stationary phase fronts in rubber
Continuum Mechanics and Thermodynamics (2010)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.