Visualizing the local optical response to extreme-ultraviolet radiation with a resolution of λ/380

Abstract

Scientists have continually tried to improve the spatial resolution of imaging ever since the invention of the optical microscope in around 1610 by Galileo¹. Recently, a spatial resolution near λ/10 was achieved in a near-field scheme by using surface plasmon polaritons^2,3. However, further improvement in this direction is hindered by the size of metallic nanostructures². Here we show that atom-scale resolution is achievable in the extreme-ultraviolet region by using X-ray parametric down-conversion, which detaches the achievable resolution from the wavelength of the probe light. We visualize three-dimensionally the local optical response of diamond at wavelengths between 103 and 206 Å with a resolution as fine as 0.54 Å. This corresponds to a resolution from λ/190 to λ/380, an order of magnitude better than ever achieved. Although the present study focuses on the relatively high-energy optical regions, our method could be extended into the visible region using advanced X-ray sources^4,5,6,7, and would open a new window into the optical properties of solids.

Main

The optical response is recognized as a powerful tool to investigate materials and as a useful property for widespread application in science and industry. In spite of its importance, our knowledge of the optical property is quite limited. At present, we can only measure the macroscopic optical response, and cannot see how electrons in materials respond to the light due to the limited spatial resolution. In other words, the charge response can be investigated only at the Γ point, the origin in the momentum space⁸. Such a situation contrasts with the case of the magnetic response, which is measurable over the whole Brillouin zone⁹. For example, inelastic neutron scattering indicates fluctuating stripes in high-temperature superconductors^10,11. Microscopic structures, such as stripes and orbital order, are observed commonly in so-called strongly correlated electron systems^10,12. If the optical probe had the atomic resolution to unveil the charge response with large momentum transfer, it could give direct and clear evidence about the charge dynamics of microscopic structures for deeper understanding of the physical properties¹¹.

Our basic idea to realize super-resolution is that the linear optical susceptibility, χ⁽¹⁾(r), is incorporated in the second-order X-ray nonlinear susceptibility, χ⁽²⁾(r). Here, χ⁽¹⁾(r) has a microscopic structure on the atomic scale that determines the local optical response. We consider one of the second-order nonlinear processes¹³, X-ray parametric down-conversion (PDC) into the optical region, where an X-ray pump photon (labelled as p) decays spontaneously into an X-ray signal photon (s) and an optical idler photon (i). The origin of nonlinearity is considered to be the Doppler shift¹⁴, where the induced charge at the idler frequency scatters X-rays at a different frequency (Fig. 1a). We expect that this nonlinear process reveals the structure of induced charge, similar to X-ray structural analysis. Our picture is confirmed by calculation on the basis of early works^14,15 as the following relation for isotropic systems:

Here, χ_Q⁽¹⁾(ω_i) and χ_Q⁽²⁾(ω_p=ω_s+ω_i) are the Qth Fourier coefficients of χ⁽¹⁾(r,ω_i) and χ⁽²⁾(r,ω_p=ω_s+ω_i), respectively, Qis the reciprocal lattice vector, θ_psi is the polarization factor and the other symbols have their ordinary meanings. We note that our interpretation is more general than the early works, which take into account specific electronic states, such as bond charge¹⁴ or valence charge¹⁶, and ignore the core charge. We treat χ_Q⁽²⁾(ω_p=ω_s+ω_i) instead of χ⁽²⁾(r,ω_p=ω_s+ω_i) itself, because we observe X-ray PDC as nonlinear diffraction (Fig. 1b) and determine χ_Q⁽²⁾(ω_p=ω_s+ω_i) experimentally^17,18. The structure of local optical response to the idler light is to be reconstructed by the Fourier synthesis: . Now the diffraction limit is imposed at the X-ray pump wavelength, despite investigating the optical response at the idler frequency.

Figure 1: X-ray PDC and nonlinear diffraction.

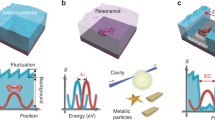
a, Schematic representation of X-ray PDC into the optical region, which is understood as the following inverse process. The optical (idler) wave oscillates electrons in the nonlinear crystal. The X-ray (signal) wave illuminating the nonlinear crystal is scattered at a different frequency because of the Doppler shift. The reflected X-ray (pump) wave has a sum (difference) frequency of the two waves. b, The phase-matching geometry used in this work. The phase-matching condition (momentum conservation) required for X-ray PDC is fulfilled as k_p+Q=k_s+k_i, where k is the wave vector in the nonlinear crystal. Thus, X-ray PDC is observed as a nonlinear diffraction from a grating of χ_Q⁽²⁾(ω_p=ω_s+ω_i). The exact phase matching is realized at a glancing angle slightly larger than the Bragg angle, θ_B. The dashed lines indicate the usual Bragg diffraction. c, The rocking curve measured at an energy of the signal wave, ℏω_s=11.007 keV, for five Q:(1 1 1),(2 2 0),(3 1 1),(2 2 2)and (4 0 0). The idler energy is ℏω_i=100 eV. Note that the rocking curve corresponds to the phase-mismatch dependence as understood from b. Each rocking curve is normalized to the background, which consists of inelastic Compton scattering. The Compton scattering interferes quantum mechanically with X-ray PDC, resulting in the Fano effect. The solid lines indicate fitting with the Fano formula to estimate χ_Q⁽²⁾(ω_p=ω_s+ω_i). The error bars are derived from the square root of row detector counts.

Figure 1c shows a typical set of rocking curves of the nonlinear diffraction measured with synthetic type IIa diamond¹⁹. The photon energies are ℏω_i=100 eV, ℏω_s=11.007 keV and ℏω_p=11.107 keV. The rocking curve was measured with the first five Q for which the Bragg reflection is observable. Three more sets were measured for ℏω_i=60, 80 and 120 eV at the same ℏω_p. The estimation of χ_Q⁽¹⁾(ω_i) is not straightforward due to the characteristic asymmetric peaks of the Fano effect^18,20,21,22. We determined the magnitude of χ_Q⁽²⁾(ω_p=ω_s+ω_i) by analysing the Fano spectra²². Then, |χ_Q⁽²⁾(ω_p=ω_s+ω_i)| was converted to |χ_Q⁽¹⁾(ω_i)| by equation (1) with the polarization factor, θ_psi=sin2θ_B, for the present experimental set-up. Here, θ_B is the Bragg angle for Q at ℏω_p. Note that there is no clear sign of X-ray PDC for Q=(2 2 2) (see Supplementary Information for discussion).

Now let us focus on the linear susceptibility at the idler energy (Fig. 2). To compare it at different photon energies, we normalize |χ_Q⁽¹⁾(ω_i)| to |χ₀⁽¹⁾(ω_i)|. Here, the average linear susceptibility, χ₀⁽¹⁾(ω_i), is calculated from the tabulated refractive index²³. We corrected the local field effect on the macroscopic linear susceptibility using the Lorentz model⁸, although the amount of correction is a few per cent. The linear structure factor, namely the Fourier transform of charge density, of the core (valence) electrons, F_Q^C (F_Q^V), is plotted for comparison. We estimated F_Q^V using the structure factor measured by X-ray powderdiffraction²⁴ and calculated F_Q^C with the XD2006 program²⁵. The |Q| dependence of |χ_Q⁽¹⁾(ω_i)/χ₀⁽¹⁾(ω_i)|shows rapid decay similar to that of |F_Q^V|, indicating that χ⁽¹⁾(r,ω_i) is not localized, but extended. At the same time, we notice a longer tail of |χ_Q⁽¹⁾(ω_i)/χ₀⁽¹⁾(ω_i)| at larger |Q|, which is due to a weak contribution from the localized core electrons. These observations indicate that the bond-charge model¹⁴ is not sufficient to describe X-PDC into the extreme-ultraviolet region. The strong ℏω_i dependence of |χ_Q⁽¹⁾(ω_i)/χ₀⁽¹⁾(ω_i)|, especially for the smaller |Q|, relates to a change in the structure of χ⁽¹⁾(r,ω_i).

Figure 2: Fourier coefficients of linear susceptibility, and the structure factors.

The |Q| dependence of the normalized linear susceptibility, |χ_Q⁽¹⁾(ω_i)/χ₀⁽¹⁾(ω_i)|, is plotted for ℏω_i=60, 80, 100 and 120 eV. The structure factors for the core (F_Q^C, blue bars) and the valence (F_Q^V, orange bars) electrons are shown for comparison. The net-plane spacing, d=2π/|Q|, is used on the abscissa axis in the conventional manner. The error bars represent statistical and fitting uncertainties.

Our key result shown in Fig. 3a–d represents the Fourier-synthesized microscopic structure of linear susceptibility, χ⁽¹⁾(r,ω_i), for the light with the idler energies. The resolution is estimated to be 0.61×2π/|Q_max|=0.54 Å (ref. 1), which is much shorter than the idler wavelengths (corresponding photon energies), ranging from 103 Å (120 eV) to 206 Å (60 eV). The resolution in the unit of λ reaches λ/380 for ℏω_i=60 eV, which is the highest ever achieved. As shown in Fig. 3g, we succeed in reconstructing the three-dimensional structure of χ⁽¹⁾(r,ω_i) with a 0.54 Å resolution. It is the most interesting finding that small spherical regions around each atom respond in phase to the light, whereas disc-shaped regions, which respond in the opposite phase, have a stronger contribution and dominate the optical response. The weak structures in the outer region are artefacts of the Fourier synthesis with a limited value of χ_Q⁽¹⁾(ω_i). Note that χ⁽¹⁾(r,ω_i) is normalized to its negative average level, χ₀⁽¹⁾(ω_i).

Figure 3: Reconstructed microstructures of the optical linear susceptibility and the density distribution of valence and core electrons.

a–d, The 110-plane cuts of the normalized linear susceptibility, χ⁽¹⁾(r,ω_i)/χ₀⁽¹⁾(ω_i), for ℏω_i=60 eV (a), 80 eV (b), 100 eV (c) and 120 eV (d). See the left tick marks of the left colour bar. The contour lines are plotted with an interval of 1.0. The dashed lines indicate the level of χ⁽¹⁾(r,ω_i)/χ₀⁽¹⁾(ω_i)=0. The white bar indicates the resolution of reconstruction, 0.54 Å. e,f, The 110-plane cuts of the valence (e) and the core (f) electron density synthesized from F_Q^C and F_Q^V, respectively. We used 43 Fourier components up to Q=(8 8 0). See the left tick marks of the right colour bar for e and the right tick marks for f. The contour lines are plotted with an interval of 25 e Å⁻³ for e and 1,500 e Å⁻³ for f. The dashed lines indicate the zero level. The white bar indicates the resolution of reconstruction, 0.19 Å. g, Three-dimensional view of χ⁽¹⁾(r,ω_i)/χ₀⁽¹⁾(ω_i) at ℏω_i=60 eV with the 110-plane cut. See the right tick marks of the left colour bar. The red discs and the blue spheres indicate the constant-height surface of χ⁽¹⁾(r,ω_i)/χ₀⁽¹⁾(ω_i)=1.9 (red) and −1.0 (blue), respectively. The blue spheres respond in phase to the light, whereas the red discs in the opposite phase. Note that χ₀⁽¹⁾(ω_i) is negative. Each carbon atom resides at the centre of a blue sphere. The black lines indicate the bonding directions.

An important point to be noted in the Fourier synthesis is the phase problem due to the fact that we measured only the amplitude of χ_Q⁽¹⁾(ω_i). At present, we do not have any established procedure to recover the missing phase, and carry out an exhaustive search. We synthesize χ⁽¹⁾(r,ω_i) using all possible combinations of the phase, and find a structure compatible with physical pictures, such that χ⁽¹⁾(r,ω_i) should be small in the region where the electron density is low and the bonding sites should have a major contribution (see Supplementary Information for details). The number of combinations can be reduced greatly by the following considerations. As we investigate the optical response far from resonances, we regard χ⁽¹⁾(r,ω_i) as real. Then, χ_Q⁽¹⁾(ω_i) is real, because the diamond structure possesses a centre of inversion. We need to determine only the sign of χ_Q⁽¹⁾(ω_i). The symmetry of the diamond structure fixes the relative phase difference among different Q with the same magnitude, for example, χ₁₁₁⁽¹⁾(ω_i)=−χ₁₁₋₁⁽¹⁾(ω_i).

Before we discuss the microscopic structure of χ⁽¹⁾(r,ω_i), we review the macroscopic optical response of diamonds to make clear what we find. For simplicity, we regard the band structure of diamond as a three-level system, which consists of the 1s core level, the valence ‘level’ and the conduction ‘level’. The macroscopic optical response of this three-level system may be described as a sum of two Lorentz oscillators⁵:

Here, n_c (n_v) and ω_c (ω_v) are the number density and the resonance frequency of core (valence) electrons. We ignore the lifetime (damping factor) for simplicity, and assume ℏω_c=289 eV (ref. 22) and ℏω_v=12 eV (ref. 26). It is clear that the valence electron determines χ₀⁽¹⁾(ω) around ℏω=100 eV, because the denominator of the first term is much larger.

According to the macroscopic picture above, it may be thought that the microscopic structure of χ⁽¹⁾(r,ω_i) should be similar to the density distribution of valence electrons. We notice, however, a large difference between them. The valence-electron density (Fig. 3e) has considerable weight at the atomic site, which originates from the 2s orbital, whereas χ⁽¹⁾(r,ω_i) at the atomic site is smaller or has opposite sign to the rest (Fig. 3a–d).

The discrepancy between χ⁽¹⁾(r,ω_i) and the density distribution of valence electron is explained well by taking the contribution from the 1s core electrons into account. The radii of the s orbitals have a quadric dependence on the main quantum number, so the radius of the 1s orbital is four times smaller than that of the 2s orbital, which makes the density of the 1s core electrons 4³ times higher (Fig. 3e,f). Such a high concentration compensates the larger denominator for the 1s core electrons in equation (2). As is clear from equation (2), the 1s core electron responds in phase to the light around 100 eV, whereas the 2s-like state respond in the opposite phase. The 1s core and the 2s-like states, which give comparable but opposite contributions to each other, nearly cancel out at the atomic site.

We make a rough estimate of χ_Q⁽¹⁾(ω_i) by replacing n in equation (2) with F_Q/v_c, where v_c is the volume of the unit cell. The 111 structure factors for the 1s core electrons and the valence electrons are estimated to be F₁₁₁^C=−10.8 and F₁₁₁^V=−7.70, respectively²⁴. We evaluate equation (2) at ℏω=100eV, and find χ₁₁₁⁽¹⁾(100 eV)=1.53×10⁻³ e.s.u., whereas our experimental result is (3.7±0.57)×10⁻³ e.s.u. The agreement between the calculation and our experimental estimation is considered to be satisfactory for the following reason. As for the average susceptibility, we can compare the calculation by equation (2) with that reported. The calculation gives χ₀⁽¹⁾(100 eV)=−3.66×10⁻³ e.s.u., whereas the reported value is −8.31×10⁻³ e.s.u. (ref. 23). The calculation on the basis of equation (2) gives the same orders of magnitude, and has a tendency to underestimate the susceptibility, which is attributed to the model being simpler than the real band structure.

Finally, we consider qualitatively the energy dependence of χ⁽¹⁾(r,ω_i). It may be thought naively that only the magnitude of χ⁽¹⁾(r,ω_i)changes in accordance with χ₀⁽¹⁾(ω_i) and that the shape remains unchanged even when the energy of light changes. We find that the shape of χ⁽¹⁾(r,ω_i) remains basically the same; however, the contrast shows a strong energy dependence. The higher contrast at 120 eV (Fig. 3d) is considered to be due to the stronger resonance effect of 1s core electrons at higher photon energies.

In summary, we have demonstrated that we can make use of the spatial resolution of X-rays, keeping the probing wavelength in the optical region, and can visualize the local optical response to extreme-ultraviolet radiation with atomic resolution. The application of our method in a lower-energy region, such as the visible region, requires a deeper understanding of X-ray PDC and a more sophisticated procedure for the phase determination, because the optical response becomes more complicated than equation (2). We discuss briefly a straightforward application of X-ray PDC to the soft-X-ray region. Recently, soft-X-ray resonant scattering has been recognized as a powerful tool to investigate microscopically the strongly correlated system²⁷. For example, doped holes in high-temperature superconductors are investigated directly using soft-X-ray resonant scattering at the K edge of oxygen²⁸. However, the drawback of using soft X-rays is the limited spatial resolution, 22.8 Å for the oxygen case. Our method should remove this limitation, enlarging the potential of the soft-X-ray probe.