Abstract
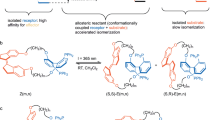
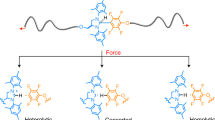
Mechanical stimuli can modify the energy landscape of chemical reactions and enable reaction pathways, offering a synthetic strategy that complements conventional chemistry1,2,3. These mechanochemical mechanisms have been studied extensively in one-dimensional polymers under tensile stress4,5,6,7,8,9 using ring-opening10 and reorganization11, polymer unzipping6,12 and disulfide reduction13,14 as model reactions. In these systems, the pulling force stretches chemical bonds, initiating the reaction. Additionally, it has been shown that forces orthogonal to the chemical bonds can alter the rate of bond dissociation15. However, these bond activation mechanisms have not been possible under isotropic, compressive stress (that is, hydrostatic pressure). Here we show that mechanochemistry through isotropic compression is possible by molecularly engineering structures that can translate macroscopic isotropic stress into molecular-level anisotropic strain. We engineer molecules with mechanically heterogeneous components—a compressible (‘soft’) mechanophore and incompressible (‘hard’) ligands. In these ‘molecular anvils’, isotropic stress leads to relative motions of the rigid ligands, anisotropically deforming the compressible mechanophore and activating bonds. Conversely, rigid ligands in steric contact impede relative motion, blocking reactivity. We combine experiments and computations to demonstrate hydrostatic-pressure-driven redox reactions in metal–organic chalcogenides that incorporate molecular elements that have heterogeneous compressibility16,17,18,19, in which bending of bond angles or shearing of adjacent chains activates the metal–chalcogen bonds, leading to the formation of the elemental metal. These results reveal an unexplored reaction mechanism and suggest possible strategies for high-specificity mechanosynthesis.
This is a preview of subscription content, access via your institution
Access options
Access Nature and 54 other Nature Portfolio journals
Get Nature+, our best-value online-access subscription
$29.99 / 30 days
cancel any time
Subscribe to this journal
Receive 51 print issues and online access
$199.00 per year
only $3.90 per issue
Buy this article
- Purchase on Springer Link
- Instant access to full article PDF
Prices may be subject to local taxes which are calculated during checkout




Similar content being viewed by others
References
Gilman, J. J. Mechanochemistry. Science 274, 65–66 (1996)
Caruso, M. M. et al. Mechanically-induced chemical changes in polymeric materials. Chem. Rev. 109, 5755–5798 (2009)
Hickenboth, C. R. et al. Biasing reaction pathways with mechanical force. Nature 446, 423–427 (2007)
May, P. A. et al. Polymer mechanochemistry: techniques to generate molecular force via elongational flows. Chem. Soc. Rev. 42, 7497–7506 (2013)
Huang, Z. & Boulatov, R. Chemomechanics: chemical kinetics for multiscale phenomena. Chem. Soc. Rev. 40, 2359–2384 (2011)
Diesendruck, C. E. et al. Mechanically triggered heterolytic unzipping of a low-ceiling-temperature polymer. Nat. Chem. 6, 623–628 (2014)
Piermattei, A., Karthikeyan, S. & Sijbesma, R. P. Activating catalysts with mechanical force. Nat. Chem. 1, 133–137 (2009)
Klukovich, H. M. et al. A backbone lever-arm effect enhances polymer mechanochemistry. Nat. Chem. 5, 110–114 (2013)
Chen, Y. et al. Mechanically induced chemiluminescence from polymers incorporating a 1,2-dioxetane unit in the main chain. Nat. Chem. 4, 559–562 (2012)
Davis, D. A. et al. Force-induced activation of covalent bonds in mechanoresponsive polymeric materials. Nature 459, 68–72 (2009)
Wang, J., Kouznetsova, T. B. & Craig, S. L. Single-molecule observation of a mechanically activated cis-to-trans cyclopropane isomerization. J. Am. Chem. Soc. 138, 10410–10412 (2016)
Chen, Z. et al. Mechanochemical unzipping of insulating polyladderene to semiconducting polyacetylene. Science 357, 475–479 (2017)
Li, W. & Gräter, F. Atomistic evidence of how force dynamically regulates thiol/disulfide exchange. J. Am. Chem. Soc. 132, 16790–16795 (2010)
Dopieralski, P. et al. The Janus-faced role of external forces in mechanochemical disulfide bond cleavage. Nat. Chem. 5, 685–691 (2013)
Akbulatov, S. et al. Experimentally realized mechanochemistry distinct from force-accelerated scission of loaded bonds. Science 357, 299–303 (2017)
Yan, H. et al. Hybrid metal–organic chalcogenide nanowires with electrically conductive inorganic core through diamondoid-directed assembly. Nat. Mater. 16, 349 (2017)
Gamba, Z. & Powell, B. M. The condensed phases of carboranes. J. Chem. Phys. 105, 2436 (1996)
Schwertfeger, H., Fokin, A. A. & Schreiner, P. R. Diamonds are a chemist’s best friend: diamondoid chemistry beyond adamantane. Angew. Chem. Int. Ed. 47, 1022–1036 (2008)
Gunawan, M. A. et al. Diamondoids: functionalization and subsequent applications of perfectly defined molecular cage hydrocarbons. New J. Chem. 38, 28–41 (2014)
Castner, D. G., Hinds, K. & Grainger, D. W. X-ray photoelectron spectroscopy sulfur 2p study of organic thiol and disulfide binding interactions with gold surfaces. Langmuir 12, 5083–5086 (1996)
Schneider, S., Dzudza, A., Raudaschl-Sieber, G. & Marks, T. J. Copper(I) tert-butylthiolato clusters as single-source precursors for high-quality chalcocite thin films: precursor chemistry in solution and the solid state. Chem. Mater. 19, 2768–2779 (2007)
Birch, F. Finite elastic strain of cubic crystals. Phys. Rev. 71, 809–824 (1947)
Jang, Y. J. et al. Tree branch-shaped cupric oxide for highly effective photoelectrochemical water reduction. Nanoscale 7, 7624–7631 (2015)
Nowacki, W. Die Krystallstruktur von Adamantan (symm. Tri-cyclo-decan). Helv. Chim. Acta 28, 1233–1242 (1945)
May, P. A. et al. Is molecular weight or degree of polymerization a better descriptor of ultrasound-induced mechanochemical transduction? ACS Macro Lett. 5, 177–180 (2016)
APEX2, https://www.bruker.com/products/x-ray-diffraction-and-elemental-analysis/single-crystal-x-ray-diffraction/overview/sc-xrd-software/apex3.html (Bruker AXS, 2007)
Sheldrick, G. M. et al. Crystal structure refinement with SHELXL. Acta Crystallogr. C. 71, 3–8 (2015)
Sheldrick, G. M. A short history of SHELX. Acta Crystallogr. A 64, 112–122 (2008)
Dolomanov, O. V. et al. OLEX2: a complete structure solution, refinement and analysis program. J. Appl. Cryst. 42, 339–341 (2009)
Mao, H.-K. & Mao, W. L. in Treatise on Geophysics 231–267 (Elsevier, 2007)
Hammersley, A. P. Internal Report, ESRF98HA01T, FIT2D V9.129 Reference Manual V3.1 (European Synchrotron Radiation Facility (ESRF), 1998)
Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996)
Giannozzi, P. et al. QUANTUM ESPRESSO: a modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Matter 21, 395502 (2009)
Macrae, C. F . et al. Mercury: visualization and analysis of crystal structures. J. Appl. Cryst. 39, 453–457 (2006)
Momma, K. & Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Cryst. 44, 1272–1276 (2011)
Dion, M. et al. Van der Waals density functional for general geometries. Phys. Rev. Lett. 92, 246401 (2004)
Lee, K. et al. Higher-accuracy van der Waals density functional. Phys. Rev. B 82, 081101 (2010)
Berland, K. et al. Assessment of two hybrid van der Waals density functionals for covalent and non-covalent binding of molecules. J. Chem. Phys. 146, 234106 (2017)
Acknowledgements
We thank C. Beavers, J. Yan and S. Teat from the Advanced Light Source for help with XRD measurements, and C. Park and D. Popov from the Advanced Photon Source for XAS measurements. This work was supported by the Department of Energy, Office of Basic Energy Sciences, Division of Materials Science and Engineering, under contracts DE-AC02-76SF00515 and DE-FG02-06ER46262. D.P. acknowledges support from Hong Kong Research Grants Council (project number ECS-26305017), the National Natural Science Foundation of China (project number 11774072) and the Alfred P. Sloan Foundation through the Deep Carbon Observatory. D.S.-I. acknowledges support from PAPIIT IA203116/27 and CONACYT FC-2015-2/829. This research used resources of the Advanced Light Source, which is a US Department of Energy (DOE) Office of Science User Facility under contract DE-AC02-05CH11231. This research also used resources of the Advanced Photon Source, a DOE Office of Science User Facility operated for the DOE Office of Science by Argonne National Laboratory under contract DE-AC02-06CH11357. Portions of this work were performed at the Stanford Nano Shared Facilities, supported by the National Science Foundation under award ECCS-1542152. The computational work used resources at the Stanford Research Computing Center, the Research Computing Center at the University of Chicago, and the Deep Carbon Observatory computer cluster.
Author information
Authors and Affiliations
Contributions
H.Y., W.L.M., Z.-X.S. and N.A.M. conceived the idea. H.Y., J.N.H. and D.S.-I. synthesized the crystals and solved their structures. H.Y., F.Y. and Y.L. carried out the high-pressure experiments. H.Y., D.P. and G.G. performed the DFT computations. H.Y. and F.H.L. performed the ex situ characterizations. J.E.P.D., R.M.K.C., B.A.T., A.A.F. and P.R.S. provided the diamondoids and synthesized their derivatives. H.Y. and N.A.M. wrote the paper. All authors contributed to the discussion and revision of the paper.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Additional information
Reviewer Information Nature thanks D. Braga, S. James and L. Yan for their contribution to the peer review of this work.
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Extended data figures and tables
Extended Data Figure 1 Schematic of the molecular anvil and steric blockage scenarios.
a, Relative motions (red arrows) of the rigid ligands under hydrostatic pressure anisotropically deform the mechanophore, leading to reactivity. b, Motion of the ligands is blocked by their steric repulsion, protecting the mechanophore from deformation.
Extended Data Figure 2 Crystal structures of compounds used in this study.
a, Cu-S-M9. b, Ag-S-Ada. c, Ag-S-mDia. Each panel depicts the asymmetric unit (left) and the unit cell (right). Atoms are represented by their thermal ellipsoids at the 50% probability level. Copper, silver, sulfur, carbon and boron are denoted by red, green, yellow, grey and pink respectively. Hydrogen atoms are omitted for clarity. The toluene molecules (depicted in grey) reside in the interstitial spaces between Cu-S-M9 molecules with a 1:1 molar ratio.
Extended Data Figure 3 Unreacted Cu-S-M9.
a, TEM image of uncompressed Cu-S-M9. b, TEM image of Cu-S-M9 after compression to 8 GPa. Scale bars in a and b, 100 nm. c, Zoom-in view of b. Scale bar, 10 nm. No inorganic lattice structures are identified in these samples. d, Representative EDS of unreacted Cu-S-M9. Both Cu and S peaks can be seen. The asterisks mark Ni peaks originating from the TEM grid. The star marks the position of the Si K-edge, an impurity probably introduced during TEM sample preparation.
Extended Data Figure 4 S 2p and Cu 2p X-ray photoelectron spectroscopy of Cu-S-M9.
Red and blue represent uncompressed Cu-S-M9 and sample after compression to 12 GPa, respectively. Discrete dots and solid continuous lines denote experimental data and fitting, respectively. a, The S 2p spectra are fitted to two Voigt peaks representing 2p1/2 and 2p3/2 peaks (dashed lines). The peak position of the uncompressed sample, 162 eV, is characteristic of metal thiolates. The upshift of the binding energy to 163 eV after compression can be attributed to the oxidation of sulfur to form, for example, disulfides. b, The Cu 2p spectra consist of two peaks, namely 2p1/2 at about 936 eV and 2p3/2 at about 954 eV. The absence of satellite peaks excludes the formation of Cu(ii) species. Moreover, the 2p3/2 peak of the uncompressed sample is best fitted by two Voigt peaks (the shoulder peak is denoted by the dashed line and marked by the arrow), characteristic of Cu(i). The 2p3/2 peak of the compressed sample is best fitted with a single Voigt peak, consistent with Cu(0). These features support the conclusion that copper is in the +1 valence state in the pristine sample, and reduced to the zero valence state upon compression to 12 GPa.
Extended Data Figure 5 XRD patterns of the pyrolysis products of Cu-S-M9 and Cu-S-Ada.
Peaks are registered to β-Cu2S (chalcocite, PDF Number 00-026-1116). The pyrolysis product of Cu-S-Ada (red) is more crystallized than that of Cu-S-M9 (blue); however, the two strongest peaks, (110) at 20.75° (d = 1.96 Å) and (103) at 21.85° (d = 1.87 Å) can be clearly seen in both samples. Pyrolysis was carried out at 400 °C in sealed quartz tubes under vacuum. XRD was recorded with a MoKα source (λ = 0.7107 Å).
Extended Data Figure 6 Reversible compression of Cu-S-M9 below 8 GPa.
The dashed line shows ambient XRD calculated from the single-crystal structure. The solid lines are in situ XRD measured at 0.5 GPa (black), 6.1 GPa (red) and back to 0.8 GPa (blue). λ = 0.6199 Å.
Extended Data Figure 7 Experimental and computed unit cell volumes and key structural features of Cu-S-M9 at high pressure.
a, Unit cell volumes determined by experiment and computed by DFT using different exchange-correlation functionals. b–d, S1–S2 distance (b), S1–Cu1–S2 bond angle (c) and average Cu1–S1/Cu1–S2 bond length (d) computed using PBE (red) and vdW-DF (blue) functionals. The unit cell volumes computed by PBE, vdW-DF and vdW-DF2 agree well with experimental data, with less than 5% deviation. The vdW-DF-cx functional gave consistently smaller unit cell volume, probably owing to overestimation of the dispersion interaction. Furthermore, PBE and vdW-DF show the same trends in the key structural changes, that is, decreasing S1–S2 distance and S1–Cu1–S2 bond angle, as well as increasing Cu–S bond length beyond 6 GPa. These results show that the van der Waals interactions do not substantially affect the computed high-pressure structures in our systems.
Extended Data Figure 8 Structural changes of Cu-S-M9 at high pressure.
a, Structure of the Cu-S-M9 molecule. b–d, Changes in S–S distances (b), M9–M9 distances (c) and S–Cu–S bond angles (d) as functions of pressure. All data are extracted from DFT computations.
Extended Data Figure 9 HOMO of Cu-S-M9, uncompressed and compressed.
a, At ambient pressure; b, at 12 GPa. The isosurfaces depict the probability density times the sign of the HOMO wavefunction ψ. Red and blue represent isovalues of ±2 × 10−4 per cubic bohr, respectively. The dashed lines in the 12 GPa structure mark the nodal planes across Cu–S bonds.
Extended Data Figure 10 TEM images of sterically impeded systems.
a, b, Cu-S-Ada before (a) and after (b) 20 GPa compression. Scale bars in a and b, 1 μm. c, Ag-S-Ada after 20 GPa compression. Scale bar, 20 nm. Inset, Fourier transform pattern of c.
Supplementary information
Supplementary Information
This file contains Supplementary Tables S1-S5. (PDF 220 kb)
Rights and permissions
About this article
Cite this article
Yan, H., Yang, F., Pan, D. et al. Sterically controlled mechanochemistry under hydrostatic pressure. Nature 554, 505–510 (2018). https://doi.org/10.1038/nature25765
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1038/nature25765
This article is cited by
-
Dynamical control of nanoscale light-matter interactions in low-dimensional quantum materials
Light: Science & Applications (2024)
-
The many flavours of mechanochemistry and its plausible conceptual underpinnings
Nature Reviews Chemistry (2021)
-
Synthesis and X-ray characterization of 15- and 16-vertex closo-carboranes
Nature Communications (2020)
-
Structure Restoration of Thermally Denatured Collagen by Ultrahigh Pressure Treatment
Food and Bioprocess Technology (2020)
-
Molecules pressured to react
Nature (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.