Abstract
A globally accurate many-body expansion potential energy surface is reported for HCS(X2A′) by fitting a wealth of accurate ab initio energies calculated at the multireference configuration interaction level using aug-cc-pVQZ and aug-cc-pV5Z basis sets via extrapolation to the complete basis set limit. The topographical features of the present potential energy surface are examined in detail and is in good agreement with the raw ab initio results, as well as other theoretical results available in literatures. By utilizing the potential energy surface of HCS(X2A′), the dynamic studies of the C(3P) + SH(X2Π) → H(2S) + CS(X1∑+) reaction has been carried out using quasi-classical trajectory method.
Similar content being viewed by others
Introduction
The HCS radical is a fundamental reaction intermediate in combustion processes1, which plays an important role in molecular formation processes of interstellar clouds2. The transient species HCS and CH2S were identified as a reactive intermediate in hydrogen abstraction reaction of methyl mercaptane (CH2SH) with fluorine atoms by means of photoionization mass spectroscopy3. Kaiser et al. demonstrated that the sulfur-containing species are important in Jupiter’s atmosphere4,5. They carried out experimental and theoretical studies on the reaction C(3Pj) + H2S, which has the following channels,





It should be mentioned that Cristina6 also studied the isomer pair HCS/HSC and related cations using the coupled cluster (CC) method in conjunction with correlation consistent basis sets ranging in size from quadruple to sextuple zeta for all the species to investigate the near-equilibrium potential energy surface (PES). In 1983, Goddard7 firstly predicted the equilibrium geometries of the lowest  and
and  electronic states of HCS using the single-double configuration interaction (SDCI) method with a double-zeta basis. Subsequently, Pope et al.8 performed a theoretical study of fragmentation and rearrangement processes of H2CS and H2CS+ radicals. Moreover, the HCS/HSC and HCS+/HSC+ isomerizations were investigated at the CI level in conjunction with triple-zeta basis sets. Stoecklin et al.9,10,11 investigated two analytic models of the lowest PES of the reaction SH(X2Π) + C(3P) →
electronic states of HCS using the single-double configuration interaction (SDCI) method with a double-zeta basis. Subsequently, Pope et al.8 performed a theoretical study of fragmentation and rearrangement processes of H2CS and H2CS+ radicals. Moreover, the HCS/HSC and HCS+/HSC+ isomerizations were investigated at the CI level in conjunction with triple-zeta basis sets. Stoecklin et al.9,10,11 investigated two analytic models of the lowest PES of the reaction SH(X2Π) + C(3P) →  using the MNDO/CI method, three cols connect the HCS to HSC and ground-state products. Potential energy, electric dipole and transition moment functions were calculated by Senekowitsch et al.12 for the X2A′ and A2A′′ electronic states of the HCS radical at the multiconfiguration self-consistent field (MCSCF) CI level. For the X2A′, the calculated equilibrium geometries are: RCH = 1.083 Å, RCS = 1.573 Å, and αHCS = 131.8°, for the A2A′′, RCH = 1.063 Å, RCS = 1.557 Å, and αHCS = 180°, respectively. They also reported that in linear geometry the two lowest X2A′ and A2A′′ states become degenerate components of the 2Π state and exhibit a strong Renner-Teller coupling effect. HCS, HSC, and the corresponding cationic species were also investigated by Curtiss et al.13, who carried out a theoretical study of the organo-sulfur systems CSHn (n = 0–4) and CSn (n = 0–5). The dissociation and ionization energies, and enthalpies of formation were calculated. Ochsenfeld et al.14 investigated the reaction of atomic carbon with H2S using CC theory. In the same year, Chen et al.15 presented the hyperfine structures of the HCS and isovalent HCO, HSiS and HSiO radicals using the density functional theory (DFT) and multi-reference single and double excitation configuration interaction (MRSDCI) methods. In 2004, Voronin16 reported a global analytical PES corresponding to the lowest adiabatic X2A′ state of HCS using a grid of 1357 energy points calculated at the MRCI level in conjunction with the aug-cc-pVTZ basis set. By employing the PES, the main stationary points of the X2A′ surface were also evaluated. Mitrushchenkov et al.17 performed theoretical studies on HCnS (n = 1–12) radicals in the 2Π electronic ground state using the Hartree Fock (HF), complete active space self-consistent field (CASSCF) and MRCI methods with different basis sets. Habara et al.18,19,20,21 detected microwave transition of the HCS, HSC, H13CS and HS13C radicals in the X2A′ ground electronic state.
using the MNDO/CI method, three cols connect the HCS to HSC and ground-state products. Potential energy, electric dipole and transition moment functions were calculated by Senekowitsch et al.12 for the X2A′ and A2A′′ electronic states of the HCS radical at the multiconfiguration self-consistent field (MCSCF) CI level. For the X2A′, the calculated equilibrium geometries are: RCH = 1.083 Å, RCS = 1.573 Å, and αHCS = 131.8°, for the A2A′′, RCH = 1.063 Å, RCS = 1.557 Å, and αHCS = 180°, respectively. They also reported that in linear geometry the two lowest X2A′ and A2A′′ states become degenerate components of the 2Π state and exhibit a strong Renner-Teller coupling effect. HCS, HSC, and the corresponding cationic species were also investigated by Curtiss et al.13, who carried out a theoretical study of the organo-sulfur systems CSHn (n = 0–4) and CSn (n = 0–5). The dissociation and ionization energies, and enthalpies of formation were calculated. Ochsenfeld et al.14 investigated the reaction of atomic carbon with H2S using CC theory. In the same year, Chen et al.15 presented the hyperfine structures of the HCS and isovalent HCO, HSiS and HSiO radicals using the density functional theory (DFT) and multi-reference single and double excitation configuration interaction (MRSDCI) methods. In 2004, Voronin16 reported a global analytical PES corresponding to the lowest adiabatic X2A′ state of HCS using a grid of 1357 energy points calculated at the MRCI level in conjunction with the aug-cc-pVTZ basis set. By employing the PES, the main stationary points of the X2A′ surface were also evaluated. Mitrushchenkov et al.17 performed theoretical studies on HCnS (n = 1–12) radicals in the 2Π electronic ground state using the Hartree Fock (HF), complete active space self-consistent field (CASSCF) and MRCI methods with different basis sets. Habara et al.18,19,20,21 detected microwave transition of the HCS, HSC, H13CS and HS13C radicals in the X2A′ ground electronic state.
The major goal of the present work is to obtain an accurate global adiabatic PES for the ground state of HCS based on many-body expansion (MBE) scheme. Both aug-cc-pVQZ (AVQZ) and aug-cc-PV5Z (AV5Z) atomic basis sets have been employed. In order to improve the accuracy of PES, such obtained ab initio energies are then extrapolated to the complete basis-set (CBS) limit22,23,24,25,26,27. Based on the adiabatic PES, the reaction dynamics of C + SH reaction were investigated using the quasi-classical trajectory (QCT) method.
Results
Features of HCS(X2A′) potential energy surface
Table 1 shows the characteristics of the CS(X1Σ+), CH(X2Π) and SH(X2Π), including equilibrium geometries, dissociation energies, vibrational frequencies, and spectroscopic constants. Other theoretical22,23,24,25,26,27,28,29,30,31,32 and experimental33 values are also gathered in this table for comparison. It can be found that the present values are in good agreement with results from other literatures. The obtained in the present work equilibrium internuclear distances, vibrational constants, and dissociation energies reproduce well the available experimental and theoretical results. Shown in Fig. 1 are the CS(X1Σ+), CH(X2Π) and SH(X2Π) potential energy curves (PECs). It can be seen from this figure that the modeled PECs accurately mimic the ab initio energies, with the maximum error being smaller than 10 cm−1, which shows smooth and accurate behavior both in short and long regions. In order to evaluate the fitting quality of the present PECs, we calculate the root-mean square derivation (rmsd) using the following equation:

where Vfit(i) and Vab(i) are the i-th energies from fitting and from the ab initio calculation, respectively; N is the number of points employed in the fitting procedure. The values of ΔErmsd are 3.89, 2.03, 4.45 cm−1 for SH, CH, CS, respectively, which show that the fitted PECs are of a high quality.
Figure 2 illustrates the major topographical features of the HCS(X2A′) PES. The salient features of these plots are the most relevant stationary points for the title system. Clearly, the variation of the contours is quite smooth in the whole configuration space. Most notable in Fig. 2(a) is the global minimum (GM) with the bending angle being fixed at its equilibrium ( ), which locates at R1 = 4.596 a0, R2 = 2.953 a0 and R3 = 2.062 a0 (R1 is the SH interatomic separation, R2 the CS interatomic separation, while R3 the CH interatomic separation), and the well depth is −0.3614 Eh relative to the H(2S) + S(3P) + C(3P) asymptote. In this panel, two valleys are distinguishable: the deeper one at the bottom corresponds to the H(2S) + CS(X1Σ+) asymptote, and the valley on the left corresponds to the C(3P) + SH(X2Π) asymptote. Observing the Fig. 2(a), one can find that the H(2S) + CS(X1Σ+) asymptote is bellow the C(3P) + SH(X2Π) asymptote, and it is above the global minimum. A closer observation reveals in this figure that there exists no barrier toward the dissociation of HCS(X2A′) at this configuration into S(3P) + CH(X2Π), and there is a transition state (TS1) connecting the global minimum with the H(2S) + CS(X1Σ+) asymptote. The different collinear configurations are shown in Fig. 2(b–d). In previous works5,8,16,20, one local minimum which denoted as LM1 is reported on the PES of HCS(X2A′). However, four local minima (LM1-LM4) are found on the present PES, with two of them being in linear structures. For convenience of discussion, the properties of all stationary points are collected in Table 2, including the internuclear distances, energies and vibrational frequencies. The global minimum for the HCS ground state from our CBS PES is predicted to be located at R1 = 4.596 a0, R2 = 2.953 a0 and R3 = 2.062 a0, differing 0.044 a0, 0.027 a0, and 0.008 a0 from that of ref. 16, which is based on 1357 grid points with AVTZ basis set. While the internuclear distances of the R1, R2, and R3 differ from the experimental results19 obtained by 0.007 a0, 0.0003 a0, and 0.023 a0, showing a high accuracy. It can be concluded from Table 2 that LM2-LM4 are all in long range, and both LM2 and LM3 correspond to the (H2(S) + CS(X1Σ+) asymptote, while LM4 corresponds to the S(3P) + CH(X2Π) asymptote. Figure 2(b) shows the contour plot of H-S-C linear stretching, in which the van der Waals local minimum (LM3), second-order saddle point (SP7), and transition state (TS7) can be observed. It is interesting to notice that the energy of the H(2S) + CS(X1∑+) asymptote is lower than the C(3P) + SH(X2Π) asymptote energy level, which indicates that the reaction H(2S) + CS(X1∑+) → C(3P) + SH(X2Π) is an endothermic one. The notable features in panel (c) are another van der Waals local minimum (LM4), two second-order saddle points (SP1 and SP2), and there are two transition states (TS6 and TS4). In the last panel, there is a deep well (TS5) about −0.3486 Eh, located at R1 = 4.928 a0, R2 = 2.916 a0 and R3 = 2.021 a0, which can be seen from Table 2. The TS5 and the CH(X2Π) + S(3P) asymptote are connected by a second-order saddle point (SP3).
), which locates at R1 = 4.596 a0, R2 = 2.953 a0 and R3 = 2.062 a0 (R1 is the SH interatomic separation, R2 the CS interatomic separation, while R3 the CH interatomic separation), and the well depth is −0.3614 Eh relative to the H(2S) + S(3P) + C(3P) asymptote. In this panel, two valleys are distinguishable: the deeper one at the bottom corresponds to the H(2S) + CS(X1Σ+) asymptote, and the valley on the left corresponds to the C(3P) + SH(X2Π) asymptote. Observing the Fig. 2(a), one can find that the H(2S) + CS(X1Σ+) asymptote is bellow the C(3P) + SH(X2Π) asymptote, and it is above the global minimum. A closer observation reveals in this figure that there exists no barrier toward the dissociation of HCS(X2A′) at this configuration into S(3P) + CH(X2Π), and there is a transition state (TS1) connecting the global minimum with the H(2S) + CS(X1Σ+) asymptote. The different collinear configurations are shown in Fig. 2(b–d). In previous works5,8,16,20, one local minimum which denoted as LM1 is reported on the PES of HCS(X2A′). However, four local minima (LM1-LM4) are found on the present PES, with two of them being in linear structures. For convenience of discussion, the properties of all stationary points are collected in Table 2, including the internuclear distances, energies and vibrational frequencies. The global minimum for the HCS ground state from our CBS PES is predicted to be located at R1 = 4.596 a0, R2 = 2.953 a0 and R3 = 2.062 a0, differing 0.044 a0, 0.027 a0, and 0.008 a0 from that of ref. 16, which is based on 1357 grid points with AVTZ basis set. While the internuclear distances of the R1, R2, and R3 differ from the experimental results19 obtained by 0.007 a0, 0.0003 a0, and 0.023 a0, showing a high accuracy. It can be concluded from Table 2 that LM2-LM4 are all in long range, and both LM2 and LM3 correspond to the (H2(S) + CS(X1Σ+) asymptote, while LM4 corresponds to the S(3P) + CH(X2Π) asymptote. Figure 2(b) shows the contour plot of H-S-C linear stretching, in which the van der Waals local minimum (LM3), second-order saddle point (SP7), and transition state (TS7) can be observed. It is interesting to notice that the energy of the H(2S) + CS(X1∑+) asymptote is lower than the C(3P) + SH(X2Π) asymptote energy level, which indicates that the reaction H(2S) + CS(X1∑+) → C(3P) + SH(X2Π) is an endothermic one. The notable features in panel (c) are another van der Waals local minimum (LM4), two second-order saddle points (SP1 and SP2), and there are two transition states (TS6 and TS4). In the last panel, there is a deep well (TS5) about −0.3486 Eh, located at R1 = 4.928 a0, R2 = 2.916 a0 and R3 = 2.021 a0, which can be seen from Table 2. The TS5 and the CH(X2Π) + S(3P) asymptote are connected by a second-order saddle point (SP3).
In order to illustrate the other stationary points on the new PES, a contour plot as a function of the C-H bond distance and the  is displayed in Fig. 3, in which the CS bond distance are optimized. The stationary points not appearing in Fig. 2 can be observed in Fig. 3, which are two deep minima (GM and LM1) corresponding to the stable HCS and HSC intermediates and the isomerization transition state (TS2) connecting these two minima is clearly seen from the new PES. The barrier hight of TS2 is calculated to be 21.6 kcal mol−1 above the LM1, which is found to be well consistent with the results of Stoecklin et al.9. The GM well is found to be 37.8 kcal mol−1 bellow the LM1 well. The energy compares well with the result reported by Voronin16. It is interesting to notice the existence of an entrance barrier for the H addition pathway to the CS molecule (or an exit barrier for the HCS/HSC → H + CS dissociation). In another words, the TS1 connects the global minimum (GM) with the CS(X1Σ+) + H(2S) asymptote, while TS3 connects the local minimum (LM1) with the CS(X1Σ+) + H(2S) asymptote. A closer examination shows that the LM1 on the new PES is16.75 kcal mol−1 lower than the CS(X1∑+) + H(2S) asymptote, while Stoecklin et al.10 reported that the LM2 is 5.1 kcal mol−1 above the CS(X1∑+) + H(2S) asymptote. The difference may be due to the different quality of PES. All major topographical features of the analytical potential energy surface function (APES) are probably better viewed in a relaxed triangular plot34 utilizing scaled hyperspherical coordinates (β* = β/Q and γ* = γ/Q), where the Q, β and γ are defined as
is displayed in Fig. 3, in which the CS bond distance are optimized. The stationary points not appearing in Fig. 2 can be observed in Fig. 3, which are two deep minima (GM and LM1) corresponding to the stable HCS and HSC intermediates and the isomerization transition state (TS2) connecting these two minima is clearly seen from the new PES. The barrier hight of TS2 is calculated to be 21.6 kcal mol−1 above the LM1, which is found to be well consistent with the results of Stoecklin et al.9. The GM well is found to be 37.8 kcal mol−1 bellow the LM1 well. The energy compares well with the result reported by Voronin16. It is interesting to notice the existence of an entrance barrier for the H addition pathway to the CS molecule (or an exit barrier for the HCS/HSC → H + CS dissociation). In another words, the TS1 connects the global minimum (GM) with the CS(X1Σ+) + H(2S) asymptote, while TS3 connects the local minimum (LM1) with the CS(X1Σ+) + H(2S) asymptote. A closer examination shows that the LM1 on the new PES is16.75 kcal mol−1 lower than the CS(X1∑+) + H(2S) asymptote, while Stoecklin et al.10 reported that the LM2 is 5.1 kcal mol−1 above the CS(X1∑+) + H(2S) asymptote. The difference may be due to the different quality of PES. All major topographical features of the analytical potential energy surface function (APES) are probably better viewed in a relaxed triangular plot34 utilizing scaled hyperspherical coordinates (β* = β/Q and γ* = γ/Q), where the Q, β and γ are defined as

As shown in Fig. 4, such a plot depicts in a physical way all the stationary points discussed above.
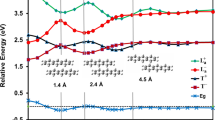
The minimum energy paths (MEPs) in different configurations (at a fixed  angle) are shown in Fig. 5. These MEPs represent the potential energy of HCS as a function of a suitable reaction coordinate defined as
angle) are shown in Fig. 5. These MEPs represent the potential energy of HCS as a function of a suitable reaction coordinate defined as  , where RSH and RCS are the SH and CS internuclear distances, respectively. As shown in Fig. 5, RSH approaches the SH equilibrium distance when the values of the reaction coordinate are in large negative values, whereas large positive values of the reaction coordinate correspond to RCS approaching the CS equilibrium distance. It is shown that the reaction C(3P) + SH(X2Π) → H(2S) + CS(X1∑+) is highly exothermic, with the exothermicity being ∼84.3 kcal mol−1, while Stoecklin et al.10 reported the exoergicity which took into account the zero-point energy is 82.7 kcal mol−1. It can be seen from this figure that the well is becoming shallow from
, where RSH and RCS are the SH and CS internuclear distances, respectively. As shown in Fig. 5, RSH approaches the SH equilibrium distance when the values of the reaction coordinate are in large negative values, whereas large positive values of the reaction coordinate correspond to RCS approaching the CS equilibrium distance. It is shown that the reaction C(3P) + SH(X2Π) → H(2S) + CS(X1∑+) is highly exothermic, with the exothermicity being ∼84.3 kcal mol−1, while Stoecklin et al.10 reported the exoergicity which took into account the zero-point energy is 82.7 kcal mol−1. It can be seen from this figure that the well is becoming shallow from  angle 45° to 180°, and the deepest well is ∼16.2 kcal mol−1 relative to the H(2S) + CS(X1∑+) asymptote with the RSH = 3.483 a0 and RCS = 2.980 a0. Note further that the MEPs on the present PES has a shallow potential barrier in H(2S) + CS(X1∑+) asymptote at
angle 45° to 180°, and the deepest well is ∼16.2 kcal mol−1 relative to the H(2S) + CS(X1∑+) asymptote with the RSH = 3.483 a0 and RCS = 2.980 a0. Note further that the MEPs on the present PES has a shallow potential barrier in H(2S) + CS(X1∑+) asymptote at  angle 90° and 135°. This barrier can be assigned to be TS3 which connects the LM1 with the CS(X1∑+) + H(2S) asymptote, with the barrier hight being calculated to be 3.6 kcal mol−1 higher than the CS(X1∑+) + H(2S) asymptote. However, when the angle becomes smaller, the CS(X1∑+) + H(2S) asymptote and GM are connected by the TS1, which is only 0.25 kcal mol−1 higher than the CS(X1∑+) + H(2S) asymptote.
angle 90° and 135°. This barrier can be assigned to be TS3 which connects the LM1 with the CS(X1∑+) + H(2S) asymptote, with the barrier hight being calculated to be 3.6 kcal mol−1 higher than the CS(X1∑+) + H(2S) asymptote. However, when the angle becomes smaller, the CS(X1∑+) + H(2S) asymptote and GM are connected by the TS1, which is only 0.25 kcal mol−1 higher than the CS(X1∑+) + H(2S) asymptote.
Exploratory dynamic studies
Based on the present HCS(X2A′) PES, we have calculated the integral cross-section (ICS), differential cross-section (DCS) and rate constants of C + SH. Figure 6 presents the QCT ICS as a function of different collision energies (0.05–1.2 eV) for C(3P) + SH(X2Π) (v = 0, 1, 2; j = 0) → H(2S) + CS(X1∑+) reaction. An obvious decline can be seen in Fig. 6 as the collision energy increases, which is ascribed to the deep well and barrierless aspect of the present HCS(X2A′) PES. As the collision energy increases, the ICS curve shows a rapidly decreasing trend from 0.05 eV to 0.2 eV, and relatively slow circumstance between 0.2 eV– 1.2 eV when v = 0. The trend of this curve is very similar to other exothermic reactions35,36,37,38. In order to further investigate the effect of the reactant SH vibrational excitation on this reaction, we plotted the ICSs of C + SH(v, j = 0) reaction for v = 0, 1, 2 in Fig. 6. We can see that the ICSs for the three vibrational states all decrease rapidly as the collision energy increases. As the vibrational quantum number increases, the ICSs for the v = 1, 2 are all higher than that of the ground vibrational state, and the larger the vibrational quantum number, the higher the related ICSs. Thus, it can be concluded that SH vibration enhances the reactivity.
The so obtained ICSs are then used to calculate the rate constant of the title reaction at 300 K, which is 5.12 × 10−13 cm3 molecule−1 s−1. Unfortunately, as far as we know, there is no experimental work reported on the rate constant. Thus, a direct comparison cannot be made between them. While, Voronin et al.16 and Stoeclclin’s et al.11 calculated the rate constant at the same temperature. The results of Voronin16 is 1.745 × 10−13 cm3 molecule−1 s−1. The difference may be due to the different characteristics of the present PES and that of Voronin et al.16.
The DCSs offer an excellent opportunity to study the most familiar vector correlation between the reagent and product relative velocity (k − k0). As shown in Fig. 7, it is clear that the DCS is dominated by scattering in both the forward and backward directions, which is consistent with the complex-forming mechanism for this reaction, and is similar to other exothermic reactions39,40. None of the DCS is completely symmetrical, showing slightly backward bias at all six collision energies. The reason for the results may be that with the energy increasing, and the trap binding becoming small, when the centrifugal potential is approximately equal to the depth of the well, the trend of head to head collision dominant the reaction. So, backward scattering is slightly larger than the forward scattering. The DCSs of different vibrational energy levels with fixed collision energies (0.2 eV, 0.6 eV, 1.0 eV) are also plotted in Fig. 8. We can see that the vibrational excitation DCSs in both forward and backward are lower than that of the ground vibrational state. So we can conclude that the SH vibration inhibits the forward and backward scattering in the same collision energy.
Discussion
We have reported a globally accurate PES for the electronic ground state of HCS based on a wealth of ab initio energies calculated at MRCI(Q)/AVQZ and MRCI(Q)/AV5Z level of theory, which is expected to be realistic over the entire configuration space. Such raw energies are subsequently extrapolated to CBS limit. The properties of the major stationary points, including geometries, energies and vibrational frequencies have been characterized on the current HCS(X2A′) PES, showing good agreement with other theoretical and experimental results. The various topographical features of the current PES give an accurate description over the short and long range interactions. The present PES has been subsequently employed to carry out the QCT calculation of the ICS, DCS and the thermal rate constant for the reaction C(3P) + SH(X2Π) → H(2S) + CS(X1∑+). The present HCS(X2A′) PES is recommended for dynamic studies of C(3P) + SH(X2Π) reaction in more detail,
Methods
Analytical potential energy surface function
The APES for the  can be represented by MBE41, which is as follows:
can be represented by MBE41, which is as follows:

in which the one-body term  is the energy of the separated atoms in their corresponding electronic state, usually
is the energy of the separated atoms in their corresponding electronic state, usually  for all the atoms in their ground states, the two-body terms
for all the atoms in their ground states, the two-body terms  correspond to the diatomics PECs, including the nuclear repulsions, and the three-body term
correspond to the diatomics PECs, including the nuclear repulsions, and the three-body term  takes into account the interactions between three atoms.
takes into account the interactions between three atoms.
For the HCS(X2A′) PES, it follows the dissociation scheme as

Since H(2S), C(3P) and S(3P) are all in their ground states, the values of  can be set to zero, which is similar to other systems42,43,44.
can be set to zero, which is similar to other systems42,43,44.
The terms  involve the
involve the  , CH(X2Π) and SH(X2Π), with their analytical expression being represented by the formalism developed by Aguado and Paniagua45,46, and can be expressed as a sum of two terms corresponding to the short- and long-range potentials,
, CH(X2Π) and SH(X2Π), with their analytical expression being represented by the formalism developed by Aguado and Paniagua45,46, and can be expressed as a sum of two terms corresponding to the short- and long-range potentials,

where

which warrants the diatomic potentials tending to infinite value when RAB → 0, and the long-range potentials which tend to zero as RAB → ∞ takes the following expression

The linear parameters  and the nonlinear parameters βi(i = 1, 2) in Eq. (11) and (12) are obtained by fitting the ab initio energies of the diatoms.
and the nonlinear parameters βi(i = 1, 2) in Eq. (11) and (12) are obtained by fitting the ab initio energies of the diatoms.
The three-body term  of the global potential in Eq. (8) can be written as Mth-order polynomial45,46
of the global potential in Eq. (8) can be written as Mth-order polynomial45,46

where  .
.  and
and  are linear and nonlinear parameters, which are determined in the fitting procedure. The constraints j + k + l ≠ j ≠ k ≠ l and j + k + l ≤ M are employed to warrant that the three-body term becomes zero at all dissociation limits and when at least one of the internuclear distances is zero. M is set to be 9 in the present work, which results in a complete set of parameters to be determined amounts to a total number of 192 for linear coefficients Cjkl and 3 for nonlinear parameters (i.e.,
are linear and nonlinear parameters, which are determined in the fitting procedure. The constraints j + k + l ≠ j ≠ k ≠ l and j + k + l ≤ M are employed to warrant that the three-body term becomes zero at all dissociation limits and when at least one of the internuclear distances is zero. M is set to be 9 in the present work, which results in a complete set of parameters to be determined amounts to a total number of 192 for linear coefficients Cjkl and 3 for nonlinear parameters (i.e.,  ,
,  and
and  ). The root mean squared deviations (rmsd) values of the final PES with respect to all the fitted ab initio energies are gathered in Table 3. As shown in Table 3, a total of 5528 points have been used for the calibration procedure, thus covering a range up to 820 kcal/mol above the HCS global minimum. This table demenstrates that the final PES is able to fit the region of major chemical interest with a high accuracy (rmsd = 0.998 kcal/mol), including the global minimum and transition state for the C + SH dissociation process.
). The root mean squared deviations (rmsd) values of the final PES with respect to all the fitted ab initio energies are gathered in Table 3. As shown in Table 3, a total of 5528 points have been used for the calibration procedure, thus covering a range up to 820 kcal/mol above the HCS global minimum. This table demenstrates that the final PES is able to fit the region of major chemical interest with a high accuracy (rmsd = 0.998 kcal/mol), including the global minimum and transition state for the C + SH dissociation process.
Ab initio electronic structure calculations
The ab initio calculations have been carried out at the MRCI level47,48 of theory with the MOLPRO 2012 package49, including the Davidson correction [MRCI(Q)]50, using the full valence CASSCF51 wave function as the reference. The AVQZ and AV5Z atomic basis sets of Dunning52,53 have been employed. A grid of 5528 ab initio points have been chosen to map the PES over the H − CS region defined by 2.0 ≤ RCS/a0 ≤ 4.5, 0.6 ≤ rH−CS/a0 ≤ 15, and 0.0 ≤ γ/deg ≤ 180, C − SH region by 2.0 ≤ RSH/a0 ≤ 4.0, 0.6 ≤ rC−SH/a0 ≤ 15, and 0.0 ≤ γ/deg ≤ 180, and S − CH region by 1.5 ≤ RCH/a0 ≤ 4.0, 0.6 ≤ rS−CH/a0 ≤ 15, and 0.0 ≤ γ/deg ≤ 180. R, r and γ are the atom-diatom Jacobi coordinates. Cs point group symmetry is employed in the ab initio calculation, which holds two irreducible representations, namely, A′ and A″. For  , seven A′ and two A″ symmetry molecular orbitals (MOs) are determined as the active space, amounting to a total of 338 (207A′ + 131A″) configuration state functions.
, seven A′ and two A″ symmetry molecular orbitals (MOs) are determined as the active space, amounting to a total of 338 (207A′ + 131A″) configuration state functions.
Extrapolation to CBS Limit
Subsequently, the ab initio energies calculated in this way were extrapolated to the CBS limit. The MRCI(Q) energy is treated as usual in split form by writing54

where the subscript X indicates that the ab initio energies are calculated in the AVXZ basis, and the superscript dc and CAS denote the dynamical correlation energy and complete-active space energy, respectively. Note that all extrapolations are carried pointwise, and hence the vector R of the nuclear geometrical coordinates will be omitted for simplicity. X = (Q, 5) is employed in the present work, which is denoted as USTE(Q, 5).
The CAS energies are extrapolated to the CBS limit by utilizing the two-point extrapolation scheme proposed by Karton and Martin55 and validated by Varandas54 in the extrapolation of the CASSCF energies

where α is a predefined constant. Being a two-parameter protocol ( , B), a minimum of two raw energies will be required for the extrapolation. Specifically, Eq. (15) will be calibrated from the CAS/AV(Q, 5)Z energy pairs, using a value of α = 5.34, which has been found to be an optimal value when extrapolating HF energies to the CBS limit.
, B), a minimum of two raw energies will be required for the extrapolation. Specifically, Eq. (15) will be calibrated from the CAS/AV(Q, 5)Z energy pairs, using a value of α = 5.34, which has been found to be an optimal value when extrapolating HF energies to the CBS limit.
The USTE method54,56 has been successfully applied to extrapolate the dc energies, with its formalism been written as

and here,

with A5(0) = 0.0037685459, c = −1.17847713 and α = −3/8 as the universal-type parameters54,56. Thus, Eq. (16) can be reduced into a ( , A3) two-parameter rule, which is actually used for the practical procedure of the extrapolation.
, A3) two-parameter rule, which is actually used for the practical procedure of the extrapolation.
Reaction Dynamics
By employing the present HCS(X2A′) PES, the reaction of C(3P) + SH(X2Π) → H(2S) + CS(X1∑+) is investigated by QCT57,58,59,60,61 calculations.
In present work, the collision energy is chosen to be 0.05–1.2 eV and batches of 100000 trajectories are run for each collision energy. The trajectories are initiated at a distance of 20 Å between the C atom and the SH diatom, and the integration step size is 0.1 fs. The maximal impact parameters bmax are optimized before running the trajectories. The ICS is then calculated as

where Nr is the number of reactive trajectories in a total number of N, and bmax is the maximal value of the impact parameters.
The rate constant k(T) calculated with the ICS and Boltzmann integration over the collision energy is given by

where kB is the Boltzmann constant, E is the collision energy, and f(T) is the electronic degeneracies of reagents and products which can be expressed as ref. 16

The distribution function P(θr), which describes the k-k′ correlation, is expanded in a series of Legendre polynomials57,62,63

where

The coefficients  are called orientation parameter (k is odd) or alignment parameter (k is even).
are called orientation parameter (k is odd) or alignment parameter (k is even).
Additional Information
How to cite this article: Song, Y.-Z. et al. Globally accurate potential energy surface for the ground-state HCS(X2A′) and its use in reaction dynamics. Sci. Rep. 6, 37734; doi: 10.1038/srep37734 (2016).
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
Kaiser, R. I., Sun, W. & Suits, A. G. Crossed beam reaction of atomic carbon C(3Pj) with hydrogen sulfide, H2S(X1A1): Observation of the thioformyl radical, HCS(X2A′). J. Chem. Phys. 106, 5288–5291 (1997).
Lee, H. H., Bettens, R. P. A. & Herbst, E. Fractional abundances of molecules in dense interstellar clouds: A compendium of recent model results. Astron. Astrophys. Suppl. Ser. 119, 111–114 (1996).
Ruscic, B. & Berkowitz, J. Photoionization mass spectrometry of CH2S and HCS. J. Chem. Phys. 98, 2568–2579 (1993).
Kaiser, R. I., Ochsenfeld, C., Head-Gordon, M. & Lee, Y. T. The Formation of HCS and HCSH molecules and their role in the collision of comet Shoemaker-Levy 9 with Jupiter. Science 279, 1181–1184 (1998).
Kaiser, R. I., Ochsenfeld, C., Head-Gordon, M. & Lee, Y. T. Crossed-beam reaction of carbon atoms with sulfur containing molecules. I. Chemical dynamics of thioformyl HCS(X2A′) formation from reaction of C(3Pj) with hydrogen sulfide, H2S(X1A1). J. Chem. Phys. 110, 2391–2403 (1999).
Puzzarini, C. The HCS/HSC and HCS+/HSC+ systems: molecular properties, isomerization, and energetics. J. Chem. Phys. 123, 024313–14 (2005).
Goddard, J. D. The structure of the thioformyl radical, HCS. Chem. Phys. Lett. 102, 224–226 (1983).
Pope, S. A., Hillier, I. H. & Guest, M. F. Rearrangement and fragmentation processes on the potential energy surfaces of thioformaldehyde molecule and cation. J. Ameri. Chem. Soci. 107, 3789–3800 (1985).
Stoecklin, T., Halvick, P. & Rayez, J. Theoretical study of the reaction C(3P) + SH(X2Π). Part 1. Semi-quantitative determination of some parts of the potential energy surfaces. J. Mol. Structure: THEOCHEM 163, 267–283 (1988).
Stoecklin, T., Rayez, J. & Duguay, B. Theoretical study of the reaction C(3P) + SH(X2Π). III. Two analytic models of the lowest potential energy surface. Chem. Phys. 148, 381–397 (1990).
Stoecklin, T., Rayez, J. & Duguay, B. Theoretical study of the reaction C(3P) + SH(X2Π). IV. A quasi-classical trajectory study of the reaction at 300 K. Chem. Phys. 148, 399–409 (1990).
Senekowitsch, J., Carter, S., Rosmus, P. & Werner, H. J. Potential energy and dipole moment functions of the HCS radical. Chem. Phys. 147, 281–292 (1990).
Curtiss, L. A., Nobes, R. H., Pople, J. A. & Radom, L. Theoretical study of the organosulfur systems CSHn(n = 0–4) and : Dissociation energies, ionization energies, and enthalpies of formation. J. Chem. Phys. 97, 6766–6773 (1992).
Ochsenfeld, C., Kaiser, R. I., Lee, Y. T. & Head-Gordon, M. Coupled-cluster ab initio investigation of singlet/triplet CH2S isomers and the reaction of atomic carbon with hydrogen sulfide to HCS/HSC. J. Chem. Phys. 110, 9982–9988 (1999).
Chen, B. Z. & Huang, M. B. Hyperfine structure in HCS and related radicals: a theoretical study. Chem. Phys. Lett. 308, 256–262 (1999).
Voronin, A. Analytical global potential energy surface for the X2A′ state of HCS. Chem. Phys. 297, 49–54 (2004).
Mitrushchenkov, A., Linguerri, R., Rosmus, P. & Maier, J. Alternation of the spin-orbit coupling in the 2Π ground state of HCnS (n = 1−12) radicals. Mol. Phys. 107, 1549–1553 (2009).
Habara, H. et al. Fourier transform millimeter-wave spectroscopy of the HCS radical in the 2A′ ground electronic state. J. Chem. Phys. 108, 8859–8863 (1998).
Habara, H., Yamamoto, S. & Amano, T. Submillimeter-wave spectra of HCS and DCS. J. Chem. Phys. 116, 9232–9238 (2002).
Habara, H. & Yamamoto, S. Microwave spectrum and molecular structure of the HSC radical. J. Chem. Phys. 112, 10905–10911 (2000).
Habara, H. & Yamamoto, S. The 13C hyperfine constants of HCS and HSC studied by microwave spectroscopy. J. Mol. Spectrosc. 219, 30–36 (2003).
Song, Y. Z. & Varandas, A. J. C. Accurate double many-body expansion potential energy surface for ground-state HS2 based on ab initio data extrapolated to the complete basis set limit. J. Phys. Chem. A. 115, 5274–5283 (2011).
Li, Y. Q., Ma, F. C. & Sun, M. T. Accurate ab initio-based adiabatic global potential energy surface for the 22A″ state of NH2 by extrapolation to the complete basis set limit. J. Chem. Phys. 139, 154305-1–7 (2013).
Li, Y. Q. et al. Accurate Double Many-Body Expansion Potential Energy Surface by Extrapolation to the Complete Basis Set Limit and Dynamics Calculations for Ground State of NH2 . J. Comput. Chem. 34, 1686–1696 (2013).
Song, Y. Z. & Varandas, A. J. C. Accurate ab initio double many-body expansion potential energy surface for ground-state H2S by extrapolated to the complete basis set limit. J. Chem. Phys. 130, 134317-1–10 (2009).
Zhang, L. L., Gao, S. B., Meng, Q. T. & Song, Y. Z. Accurate ab initio-based analytical potential energy function for via extrapolation to the complete basis set limit. Chin. Phys. B. 24, 013101-1–7 (2015).
Zhang, L. L., Zhang, J., Meng, Q. T. & Song, Y. Z. Accurate potential energy curve and spectroscopic properties of via extrapolation to the complete basis set limit. Phys. Scri. 90, 035403 (2015).
Peterson, K. A., Mitrushchenkov, A. & Francisco, J. S. A. Theoretical study of the spectroscopic properties of the ground and first excited electronic state of HS2 . Chem. Phys. 346, 34–44 (2008).
Shi, D. H. et al. Accurate analytic potential energy function and spectroscopic study for CH(X2Π) radical using coupled-cluster theory in combination with the correlation-consistent quintuple basis set. J. Mol. Struct.: THEOCHEM 860, 101–105 (2008).
Kalemos, A., Mavridis, A. & Metropoulos, A. An accurate description of the ground and excited states of CH. J. Chem. Phys. 111, 9536–9548 (1999).
Hirata, S. et al. Third-order Douglas-Kroll relativistic coupled-cluster theory through connected single, double, triple, and quadruple substitutions: Applications to diatomic and triatomic hydrides. J. Chem. Phys. 120, 3297–3310 (2004).
Shi, D. H. et al. MRCI study on potential energy curves, spectroscopic parameters and rovibrational energy levels of CS(X1Σ+) J. Mol. Struct.: THEOCHEM. 945, 1–7 (2010).
Molecular spectra and molecular structure constants of diatomic molecules (van nostrand reinhold, new york, 1979). Huber, K. P.; Herzberg, G.
Varandas, A. J. C. A useful triangular plot of triatomic potential energy surfaces. Chem. Phys. Lett. 138, 455–461 (1987).
Lin, S. Y. & Guo, H. Quantum statistical and wave packet studies of insertion reactions of S(1D) with H2, HD, and D2 . J. Chem. Phys. 122, 074304–9 (2005).
Sun, Z. P. et al. Quantum reaction dynamics of the C(1D) + H2(D2) → CH(D) + H(D) on a new potential energy surface. J. Chem. Phys. 139, 014306–6 (2013).
Gao, S. B., Zhang, J., Song, Y. Z. & Meng, Q. T. Cross sections for vibrational inhibition at low collision energies for the reaction . Eur. Phys. J. D. 69, 1–6 (2015).
Gao, S. B., Zhang, L. L., Song, Y. Z. & Meng, Q. T. Coriolis coupling effects in the reaction: A time-dependent wave packet investigation. Chem. Phys. Lett. 651, 233–237 (2016).
Zhao, J. Quasi-classical trajectory study of the O(1D) + HF reaction dynamics on 11A′ potential energy surface. Can. J. Chem. 89, 650–656 (2011).
Zhang, Y. Y. et al. Theoretical prediction of energy dependence for D + BrO→DBr + O reaction: The rate constant and product rotational polarization. Chin. Phys. B. 24, 038201-1–6 (2015).
Varandas, A. J. C. Intermolecular and intramolecular potentials: topographical aspects, calculation, and functional representation via a DMBE expansion method. Adv. Chem. Phys. 74, 255–337 (1988).
Song, Y. Z. et al. Globally accurate ab initio based potential energy surface of H2O+ (X4A″). Chin. Phys. B. 24, 063101-1–8 (2015).
Li, Y. Q., Zhang, P. Y. & Han, K. L. Accurate high level ab initio-based global potential energy surface and dynamics calculations for ground state of . J. Chem. Phys. 142, 124302-1–6 (2015).
Li, Y. Q. et al. Ab initio-based double many-body expansion potential energy surface for the first excited triplet state of the ammonia molecule. J. Chem. Phys. 136, 194705-1–8 (2012).
Aguado, A. & Paniagua, M. A new functional form to obtain analytical potentials of triatomic molecules. J. Chem. Phys. 96, 1265–1275 (1992).
Aguado, A., Tablero, C. & Paniagua, M. Global fit of ab initio potential energy surfaces I. Triatomic systems. Comp. Phys. Comm. 108, 259–266 (1998).
Werner, H. J. & Knowles, P. J. An efficient internally contracted multiconfiguration reference CI method. J. Chem. Phys. 89, 5803–5814 (1988).
Knowles, P. J. & Werner, H.-J. An efficient method for the evaluation of coupling coefficients in configuration interaction calculations. Chem. Phys. Lett. 145, 514–522 (1988).
Werner, H. J. et al. Molpro: a general-purpose quantum chemistry program package. Wires. Comput. Mol. Sci. 2, 242–253 (2012).
Langhoff, S. R. & Davidson, E. R. Configuration interaction calculations on the nitrogen molecule. Int. J. Quantum Chem. 8, 61–72 (1974).
Knowles, P. J. & Werner, H. J. An efficient second order MCSCF method for long configuration expansions. Chem. Phys. Lett 115, 259–267 (1985).
Dunning, T. H. Jr. Gaussian basis sets for use in correlated molecular calculations. I. The atoms boron through neon and hydrogen. J. Chem. Phys. 90, 1007–1023 (1989).
Kendall, R. A., Dunning, T. H. Jr. & Harrison, R. J. Electron affinities of the first-row atoms revisited. Systematic basis sets and wave functions. J. Chem. Phys. 96, 6769–6806 (1992).
Varandas, A. J. C. Extrapolating to one-electron basis-set limit in electronic structure calculations. J. Chem. Phys. 126, 244105–244119 (2007).
Karton, A. & Martin, J. M. L. Comment on “Estimating the Hartree-Fock limit from finite basis set calculations”. Theor. Chem. ACC. 115, 330–333 (2006).
Varandas, A. J. C. Basis-set extrapolation of the correlation energy. J. Chem. Phys. 113, 8880–8887 (2000).
Han, K. L., He, G. Z. & Lou, N. Q. Effect of location of energy barrier on the product alignment of reaction A + BC. J. Chem. Phys. 105, 8699–8704 (1996).
Aoiz, F. J., Brouard, M. & Enriquez, P. A. Product rotational polarization in photon-initiated bimolecular reactions. J. Chem. Phys. 105, 4964–4982 (1996).
Aoiz, F. J., Banares, L. & Herrero, V. J. Recent results from quasiclassical trajectory computations of elementary chemical reactions. J. Chem. Soc., Faraday Trans. 94, 2483–2500 (1998).
Aoiz, F. J., Sáez-Rábanos, V., Martínez-Haya, B. & González-Lezana, T. Quasiclassical determination of reaction probabilities as a function of the total angular momentum. J. Chem. Phys. 123, 094101–14 (2005).
Hu, W. & Schatz, G. C. Theories of reactive scattering. J. Chem. Phys. 125, 132301–15 (2006).
Wang, M. L., Han, K. L. & He, G. Z. Product rotational polarization in the photoinitiated bimolecular reaction A + BC → AB + C on attractive, mixed and repulsive surfaces. J. Chem. Phys. 109, 5446–5454 (1998).
Wang, M. L., Han, K. L. & He, G. Z. Product Rotational Polarization in Photo-initiated Bimolecular Reactions A + BC: Dependence on the Character of the Potential Energy Surface for Different Mass Combinations J. Phys. Chem. A. 102, 10204–10210 (1998).
Acknowledgements
This work was supported by National Natural Science Foundation of China (Grant No 11674198), National Natural Science Foundation of China (Grant No 11304185), Taishan scholar project of Shandong Province, Shandong Provincial Natural Science Foundation (Grant No ZR2014AM022), Shandong Province Higher Educational Science and Technology Program (Grant No J15LJ03), China Postdoctoral Science Foundation (Grant No 2014M561957), and Post-doctoral Innovation Project of Shandong Province (Grant No 201402013).
Author information
Authors and Affiliations
Contributions
Q.T.M. supervised the project, L.L.Z. calculated the ab initio energy points, Y.Z.S. and L.L.Z. fitted the potential energy surface, S.B.G. performed the QCT calculations, Y.Z.S. and L.L.Z.wrote the paper.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Song, YZ., Zhang, LL., Gao, SB. et al. Globally accurate potential energy surface for the ground-state HCS(X2A′) and its use in reaction dynamics. Sci Rep 6, 37734 (2016). https://doi.org/10.1038/srep37734
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep37734
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.





 , starting from −0.3614
, starting from −0.3614  .
.

 angle 45°, 90°, 135°, and 180°.
angle 45°, 90°, 135°, and 180°.

