Abstract
The super-saturated surfactant monolayer collapses with the nanoparticles (NPs) at the water/trichloroethylene (TCE) interface are investigated using molecular dynamics (MD) simulations. The results show that sodium alkyl sulfate (SDS) monolayer collapse is initiated by buckling and followed primarily by budding and the bud encapsulating the NPs and oil molecules. The developed bud detaches from the monolayer into a water phase and forms the swollen micelle emulsion with NPs and oil molecules. We investigate the wavelength of the initial budding and the theoretical description of the budding process. The wavelength of the monolayer increases with bending modulus. The energy barrier of the budding can be easily overcome by thermal fluctuation energy, which indicates that budding process proceeds rapidly.
Similar content being viewed by others
Introduction
The gas/liquid interface is the traditional medium for the assembly of inorganic ions, biomacromolecules, and inorganic nanoparticles. Typically, the langmuir-blodgett (L-B) technique is used to prepare two-dimensional molecular assemblies1,2,3. For example, Rogach and coworkers reported that the CdSe and core/shell CdSe/ZnS nanocrystals self-assembled into large area periodic lateral structures at the gas/liquid interface4. However, the limitations of the self-assembly at the gas-liquid interface emerged, such as the low stabilization, and the 2D structure limitation. Therefore, the self-assembly of nano-material at the liquid/liquid interface is believed to have wide potential in natural and industrial application5. Today, more and more work focuses on fabricating the new nanoscale substances by self-assembly of unit molecules and nanomaterials at the liquid/liquid interface5. For instance, the researchers created CdSe ultra-thin crystal film6, which comprised by 10–50 nm Ag nanoparticles (NPs)7. Recently, Dai et al. have reported the first MD simulation of the in situ self-assembly of NPs and sodium alkyl sulfate (SDS) at a water/trichloroethylene (TCE) interface, highlighting the potential of using the liquid/liquid interface to produce novel nanomaterials8. Despite the self-assembly of nanoparticles at the liquid/liquid interface is receiving ever-increasing attention from both practical and theoretical points of view, the fundaments and principles for the NPs self-assembly are required further explored8.
Recently, the experimenters have done a great deal of work to study the NPs self-assembly on the liquid/liquid interface, the NPs encapsulation on the liquid/liquid interface, and the transportation of NPs across the interface9,10,11. The self-assembly of NPs can be controlled by the size of NPs and the types of ligands in the outer layer of NPs. Because of the different ligands, the NPs can be effectively distributed and assembled on the immiscible solvent interface9,10,11. Dai observed the structures of hydrogen modified diamond-like carbon NPs (HCP) aggregates self-assembled at water-oil interface experimentally11. In the following procedure of self-assembly, the ligands can be cross-linked to generate new types of capsules, well-distributed filter materials, nanoreactors, and sustained release material. Emrick found the gold NPs decorated by the pegylated ligand self-assemble into microcapsules on the Oil/Water interface of the microemulsion12. To increase the stability of the gold NPs-coated capsules, the small molecules were introduced into the oil phase for reaction with the chain-end hydroxyl groups of the pegylated ligand. This NPs self-assembly on the liquid/liquid interface method combines the advantage of the self-assembly on the liquid/liquid interface such as controllable, predictable spatial location, and the special NPs properties such as fluorescent, superconductive, magnetic. Such multi-functional nano-structured capsule which has the packaging and transport properties can be widely used at drug sustained release and delivery13. The NPs encapsulated by monolayer would obtain smaller nano-aggregates14. In order to encapsulate and immunoisolate the cells for treatment of cancer and other illnesses, researchers have worked for a long time to fabricate the capsules with pores between 5 and 20 nm15,16,17,18. The drawbacks of routine approaches are the too broad size distribution of nano-capsule pores, or may require laborious processing for one capsule at one time. Moreover, during the ex-situ filling procedure in appropriate solvents, only the substances sufficiently small to pass the pores can be inserted into the capsule19. Therefore, finding the time-saving one-step method to fabricate microcapsules which have the narrow size distribution and where the substances can be pre-packaged is significant. Edwards found that if salt was introduced into the water phase, the gold NPs capped with stimuli-responsive copolymers would be transferred spontaneously from the water phase to toluene phase across the interface20. The fraction of NPs that transferred across the interface depended on the chemical composition of the capping copolymers and the diameter of NPs. Most of the experiments were limited to study the equilibrium structures, not the dynamic self-assembly process, and could not provide molecularly detailed information of interfacial properties such as the interfacial thickness and so on11. The molecularly detailed information will help us to understand the principles of NPs self-assembly.
In our former simulation of TCE/SDS/Water ternary system, at the high surface coverage (28.4 SDS/Å2), where the interface tension becomes ultra-low, even negative, the interface is then unstable and the monolayer surface is rippled and collapses21. We choose SDS because it is not only one of the most widely used surfactants and has many applications in industry and science, but also plays an important role in a number of the emerging fields, such as packaging design of nanomaterials, and the sustained-release of drug molecules22,23. The collapse transition is initiated by the buckling of monolayers, is followed by budding and detachment of the nanoscale swollen micelle from the monolayer21. The whole process can be divided into five stages corresponding to the bud morphologies, namely, the bending monolayer, the cap-shaped bud, the tubular bud, the bud with a constricted neck, and the swollen micelles. The micellar microemulsion which is receiving ever-increasing attention from both practical and theoretical points of view produced when the blend is stabilized by the thermal fluctuations of its internal interface24,25,26,27,28,29. The TCE swollen micellar microstructure, corresponding to a micellar microemulsion, formed via monolayer collapse transition, has a great potential of ordering nanoparticle and drug delivery as a result of encapsulating nanoparticles into the core of micellar microemulsions and transferring them from one matrix phase to another one. The releasing of drug, nutrients, and nutraceutical with poor water solubility can be controlled by the NPs encapsulated delivery systems30.
The monolayer collapse and NPs encapsulation processes have the dynamic microstructure which is hardly studied in the bulk and constrained state, therefore the mechanism is still very obscure. With the aid of the increase in computational power, MD simulations are a valuable complement to the experiments by providing molecular details. Therefore, the molecular mechanic of monolayer collapse and the NPs encapsulation needed further exploration. In our simulation, the NPs are distributed around the SDS tail to investigate the encapsulation caused by the SDS monolayer collapse.
Method
Our studied liquid/liquid quaternary system is comprised of water and TCE two bulk phases with surfactants and NPs dispersed at their interfaces. The GROMACS 3.3 simulation package31 and GROMOS96 force field32 are used for all our MD simulations. The molecular models, such as HCP, employed in this study are similar to the ones used by Dai et al.8,11, who demonstrated that they are reasonable in reproducing available experimental and simulation data. The SDS molecule is constructed as a hydrocarbon chain of 12 united carbon atoms attached to an SO4 head group with its atoms explicitly modeled. Partial charges on the sulfate head group were adopted from Bruce et al.33,34,35. The initial coordinates for the surfactant analogs and TCE were generated from the small-molecule topology generator PRODRG36. The modified hydrocarbon NPs (mean diameter of 10 Å) were truncated from a diamond-like lattice made of carbon atoms that bonded in non-planar hexagonal structure and, saturated with united CH, CH2, and CH3 atoms. The water was modeled using the single point charge (SPC) model37, with the bond lengths and angles held constant through the use of the SETTLE algorithm15. Bond lengths of surfactants and TCE were constrained using the SHAKE algorithm with a tolerance of 10−4 16.
We started our simulations from the preassembled system consisting of two abutting thick slabs of water and TCE with SDS monolayer and NPs at the two water/TCE interfaces as our former work21. The procedure for preparing an initial configuration was similar to that employed to simulate the liquid/liquid interface of multicomponent systems17,33,34. The SDS monolayers were set up by first placing a certain number of SDS in all-trans configuration in such a way that all headgroups were constrained in a thin slab with X-Y dimensions commensurate with those water/TCE interfaces. The HCP NPs were set up close to the tail groups of the SDS monolayer. Then four monolayer slabs were inserted into the water/TCE system by shifting the positions of two bulk phases upwards and downwards respectively. The corresponding number of sodium ions was randomly placed in the interfacial region by replacing water molecules. Thus, water and TCE slabs were now separated by the SDS and NPs monolayer. It should be noted that at first, the all the molecules, water, SDS, and TCE molecules don’t overlap with each other. The SDS on the two interfaces does not interact with each other due to enough water and TCE between them. After the system was set up, the whole system was subjected to the steepest descent energy minimization with a cutoff of 10 Å for van der Waals and Coulomb forces, then to further density equilibration and thermalization by NPNAT MD runs at an external normal pressure of 1 bar (along the Z axis) at 300 K. The NPNAT ensemble is applied for the simulations at fixed cross-sectional area. In this ensemble, the number of molecules (N), X -Y box dimensions (Lx and Ly), the normal pressure (PN), and the temperature (T) are fixed. As a result, the box size in the normal direction, Lz, fluctuates, in keeping with the condition that its conjugate variable PN is constrained. The temperature was maintained at 300 K using the Berendsen temperature coupling method, and Berendsen bath coupling scheme was used to keep a constant normal pressure of 1 bar18. The cutoff distance for short-range nonbonded interactions (van der Waals and real-space Coulomb) was chosen to be 12 Å and long-range electrostatic forces were computed using the Particle Mesh Ewald approach38,39. A time step of 2 fs was employed. All the simulations were at least 6 ns long.
In order to study the effect of cell length of simulation box, a set of initial structures with different number of SDS per monolayer of NSDS = 675 and 900, and with different number of NPs per monolayer of NNPs = 0, 0, 81 and 144 were created exactly in the same way as above, corresponding to monolayer coverage in the range 28.4 Å2 per SDS (where he monolayer collapse and form microemulsion in our former work21) in cells of Lx × Ly = 120 Å × 120 Å and Lx × Ly = 160 Å × 160 Å. Previous simulations of monolayer collapse show that the size of the simulated system limits both the modes of monolayer collapse and the variety of the bulk (three-dimensional) phase that could be formed25. As a result, four initial structures with and without NPs in different simulation cells were obtained.
The interfacial structures and properties were characterized using the utilities available in GROMACS as well as codes developed by us. The interfacial tension γ is defined as the difference of the normal, PN, and lateral, PL, pressures in the box:

where Lz is the box normal size and PL = (Pxx + Pyy)/2. The factor (1/2) outside the bracket takes into account the fact that there are two interfaces in the system40.
Results and Discussion
NPs encapsulated by SDS monolayer collapse
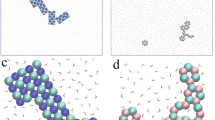
The NPs encapsulated by SDS monolayer at water/TCE interface are discussed in this section. There are 2 buds appeared per monolayer and 3 swollen micelles with NPs encapsulated detached from the monolayer into the water phase, in the Lx × Ly = 120 Å × 120 Å system. An sample of NPs encapsulated by the collapse of SDS monolayer is presented in Fig. 1. For the considered range of 28.4 SDS/Å2, the monolayer is in the “liquid expanded (LE)” phase and unstable. At first, the monolayer increases its interfacial area by the development of curvature (Fig. 1a) and the NPs stay nearby the oil interface. With simulation time increasing, the budding deformations grow in amplitude and form cap-shaped bud (Fig. 1b), and the NPs enter the bud close to the end part of SDS’s tail. The bud proceeds into the water and further grows in amplitude and form tubular bud (Fig. 1c). Yang believes the tubular bud probably arises from the effect of monolayer thickness41. The tubular bud then forms a bud with the neck connected to the monolayer (Fig. 1d). The budding causes some of TCE molecules and NPs close to the interface to be encapsulated into the core of interior shell occupied by the SDS tails. However, SDS molecules are highly deformed at the connection of the bud to the monolayer. Then the neck shrinks its perimeter in order to minimize the energy of the bud-monolayer connection line, which eventually results in the pinching off of the swollen micelles from the monolayer (Fig. 1e). After the swollen micelles detach from the monolayer and diffuse into the bulk of the aqueous phase, the monolayer reforms a flat geometry and stabilizes at the interface. As shown in Table 1, the final interfacial tension after the collapse is very low, compared to the simulation data of 41.5 mNm−1 and the experimental result of 38.9 mNm−1 8, and depends on the SDS monolayer surface coverage after the micelle detachment, which has a great agreement to our former work21. The final system consists of stable SDS monolayers at the water/TCE interface and swollen micelles. Corresponding to the bud morphologies, the whole collapse process which encapsulating the NPs can also be divided into the five stages, which is similar to the collapse process without NPs in our former work21.
The snapshots of SDS monolayer collapse and encapsulate NPs in the Lx × Ly = 120 Å × 120 Å system at high interfacial coverage of 28.4 SDS/Å2.
TCE is shown in green points; S atoms of SDS in yellow; O atoms of SDS in red; and SDS tails, in ochre bonds. NPs are shown in yellow beads. Water molecules (in the middle) are not shown. (a) 40 ps, (b) 400 ps, (c) 1120 ps, (d) 3000 ps, (e) 6000 ps.
The structure of swollen micelle
The interesting features of the swollen micelles structure can be depicted, as shown in Fig. 2. The swollen micelles contain NPs and TCE molecules (Fig. 2a) in the center of their core, which is surrounded by an outer core layer of SDS tails mixed with some TCE, and by the outer corona of SDS head-groups immersed into the external water phase. Nine NPs and 106 TCE molecules are encapsulated into swollen micelle as shown in Fig. 2a. The radius of SDS swollen micelles measured as the radial distribution function g(r) from the sulfur atoms of the head-group to the center of mass of TCE core is 45 Å, which is obviously larger than the reported values of pure SDS micellar radius of 22 Å 42. The radius of NPs core is 20 Å, and the radius of NPs and TCE core is 35 Å (Fig. 2b).
The structure of swollen micelle for the Lx × Ly = 120 Å × 120 Å system with NPs at 28.4 SDS/Å2 coverage, (a) profile of swollen micelle encapsulating TCE molecules and NPs, (b) The radial distribution function g(r) of the center of the swollen micelle and each group of the swollen micelle. The Water, TCE, SDS and NPs are normalized by space and density.
The geometry of the budding
In all the former simulations of Lx × Ly = 80 Å × 80 Å system, only one swollen micelle per monolayer was formed21. Our simulation boxes were enlarged to Lx × Ly = 120 Å × 120 Å and Lx × Ly = 160 Å × 160 Å. We found that in the Lx × Ly = 120 Å × 120 Å system, there are 2 buds appeared per monolayer and 3 swollen micelles detached from the monolayer into the water phase. More interestingly, there are 4 symmetrical buds appeared per monolayer and 6 swollen micelles detached from the monolayer into the water phase in the Lx × Ly = 160 Å × 160 Å system, as shown in Fig. 3a and b.
The similar phenomenon was found by Shinoda in coarse-grained simulation43. Because of the difference of the strength between dipalmitoyl phosphatidylcholine (DPPC) and polyethyleneglycol (PEG) monolayer, the persistence length, i.e., the correlation among the lateral lipids is different. The correlation length is quite large for a DPPC monolayer and very small for PEG monolayer. For DPPC, the buckling of the monolayer was found. However, only one small bud was detected, which were detached from the DPPC monolayer. For PEG, more than nine buds grew almost independently and detached from the monolayer.
We consider the wavelength λ of the bud before detaching from monolayer, defined as the distance from the center of the buds with a max amplitude, as shown in Fig. 3c 42. The wavelength of the Lx × Ly = 160 Å × 160 Å system is 70 Å. This clearly demonstrates that the budding has size constraints. The capillary wave of SDS monolayer before collapse has a certain correlation length and amplitude. The wavelength of the bud is approximate 70 Å. So that, only one bud appears at one monolayer in the Lx × Ly = 80 Å × 80 Å system21, and four buds appear at one monolayer in the Lx × Ly = 160 Å × 160 Å system. The budding of the monolayer is similar to the wrinkling of thin elastic sheets which follows simple scaling laws44. The wavelength of the wrinkles λ ∼ κ−1/4, where κ is the bending modulus. The wavelength is determined by the thicknesses and elastic properties of the film and the soft layer (subphase)45.

where the h is the thickness of the monolayer, and  , Ef is the Young’s modulus of the monolayer, v is the Poisson’s ratio of the monolayer. The thickness of the SDS monolayer is about 15 Å, so that the
, Ef is the Young’s modulus of the monolayer, v is the Poisson’s ratio of the monolayer. The thickness of the SDS monolayer is about 15 Å, so that the  is about 3.48, which can be used to describe the wrinkle phenomenon44.
is about 3.48, which can be used to describe the wrinkle phenomenon44.
Theoretical description of the budding
Based on a mathematical analogy, Bruinsma46 propose a description of the reversible collapse for the formation of surfactant monolayer folds, which is similar to the Griffith Cracks formation of solid plates under stress. For a rectangular, self-adhering elastic sheet of thickness h with a two-dimensional (2D) shear modulus G, an area compressibility modulus K, a bending modulus κ, and self-adhesion energy per unit area W, The shape of a bud obey the following prediction:

where the U(x) is the height of the bud, L is the length of the bud connection to the monolayer, γ is the surface tension, and x is the coordinate along the bud connection, as shown in Fig. 4.
U(0) is the max height of the bud. The bud should have a semielliptical shape according to the formula (3). Γ = U(0)/L is the ratio between the short and long axes of bud. Y is the 2D Young’s modulus, and

The area compressibility modulus of SDS at water-TCE interfaces in the LE phase obtained in our former work is 29.22 mNm−1 21, which is close to the experimental results47.
The elastic force per unit length γ+σ pulling SDS out of the bud should equal the force per unit length W/2 pulling SDS into the bud, whereσ is the contribution to the elastic stress tensor introduced by the bud. For the long-tailed surfactant molecules monolayer, W/2 can be identified as the free energy cost of creating a unit area of hydrocarbon surface, and is in the limited range of 25 ± 4 mNm−1 46. We take the surface energy W/2 of bud formation is approximate 22 mNm−1, just as DPPC and bulk alkanes48. The surface tension γ is approximate 0 mNm−1 near the collapse. Hence, σ must be a constant equal to W/2 − γ. The ratioΓ = U(0/L of SDS bud is approximate 1. Thus we can calculate the shear modulus G (6.8 mNm−1) using the formula (2), which is close to the experimental results 4 mN/m49. we also can obtain the 2D Young’s modulus Y (22 mNm−1) from the formula (4). All the modulus parameters were summarized in Table 2.
Similar to the critical crack size of solid plates, the critical bud length Lcr (above which the bud will grow, otherwise, the bud will shrink), is given by46.

where γc is the line tension at the bilayer-monolayer connection. The γc can be estimated by using the following formula48.

Then the γc is 12 pN in our SDS system according formula (5). The γc of the DPPC/POPG 4:1 monolayer is 48 pN and the γc of the softer DPPC/POPG 1:1 monolayer is 20 pN. Therefore the γc of SDS monolayer, with lower bending modulus, is smaller than the two lipid mixed monolayer (DPPC/POPG), which is reasonable48. Meanwhile, The energy barrier for budding can be obtained according to46.

Using the calculated values, we obtain Lcr = 3.5 Å, and ΔE = 0.5KBT. The energy barriers can be easily overcome by thermal energy, which indicates that budding proceeds spontaneously and rapidly.
Conclusions
In this work, the super-saturated SDS monolayer collapses with the NPs at the water/TCE interface are investigated using MD simulations. The results show that SDS monolayer collapse is initiated by buckling and the formation of nanometer-scale swollen micelles. This process is a potential mode of 3D relaxation of the monolayer at the water/TCE interface. The SDS monolayer collapse transition is followed primarily by budding and the bud encapsulates the NPs and oil molecules. The developed bud detached from the monolayer into a water phase and formed the swollen micelle emulsion with NPs and oil molecules. We investigate the wavelength of the initial budding and the theoretical description of the budding process. The wavelength of the monolayer increases with bending modulus44. The wavelength of the SDS monolayer is about 70 Å. The energy barrier of the budding can be easily overcome by thermal fluctuation energy, which indicates that budding proceeds spontaneously and rapidly. Therefore, this work moves a step forward to precisely predict the wrinkle phenomenon of super-saturated surfactant monolayer collapses with the nanoparticles (NPs) at the water/TCE interface.
Additional Information
How to cite this article: Shi, W. The structure and dynamics of Nano Particles encapsulated by the SDS monolayer collapse at the water/TCE interface. Sci. Rep. 6, 37386; doi: 10.1038/srep37386 (2016).
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
Ulman, A. An Introduction to Ultrathin Organic Films: From Langmuir-Blodgett to Self-Assembly Academic Press, Inc., San Diego (1991).
Akatsuka, K., Haga, M., Ebina, Y., Osada, M., Fukuda, K. and Sasaki, T. Construction of highly ordered lamellar nanostructures through langmuir-blodgett deposition of molecularly thin titania nanosheets tens of micrometers wide and their excellent dielectric properties. ACS Nano 3, 1097–1106 (2009).
Acharya, S., Hill, J. P. & Ariga, K. a. Soft Langmuir–Blodgett technique for hard nanomaterials. Adv. Mater. 21, 2959–2981 (2009).
Lu, N., Chen, X., Molenda, D., Naber, A., Fuchs, H., Talapin, D. V., Weller, H., Muller, J., Lupton, J. M., Feldmann, J., Rogach, A. L. & Chi, L. Lateral patterning of luminescent CdSe nanocrystals by selective dewetting from self-assembled organic templates. Nano Lett. 4, 885–888 (2004).
Rao, C. N. R. et al. Use of the liquid-liquid interface for generating ultrathin nanocrystalline films of metals, chalcogenides, and oxides. J. Colloid Interface Sci. 289, 305–318 (2005).
Kalyanikutty, K. P., Gautam, U. K. & Rao, C. N. R. Ultra-thin crystalline films of CdSe and CuSe formed at the organic-aqueous interface. J. Nanosci. Nanotechnol. 7, 1916–1922 (2007).
Krishnaswamy, R. et al. Interfacial rheology of an ultrathin nanocrystalline film formed at the liquid/liquid interface. Langmuir 23, 3084–3087 (2007).
Luo, M. & Dai, L. L. Molecular dynamics simulations of surfactant and nanoparticle self-assembly at liquid-liquid interfaces. J. Phys.: Condens. Matter 19 (2007).
Skaff, H. et al. Crosslinked capsules of quantum dots by interfacial assembly and ligand crosslinking. Adv. Mater. 17, 2082–2086 (2005).
Lin, Y., Skaff, H., Emrick, T., Dinsmore, A. D. & Russell, T. P. Nanoparticle Assembly and Transport at Liquid-Liquid Interfaces. Science 299, 226–229 (2003).
Dai, L. L., Sharma, R. & Wu, C.-y. Self-Assembled Structure of Nanoparticles at a Liquid-Liquid Interface. Langmuir 21, 2641–2643 (2005).
Glogowski, E., Tangirala, R., He, J. B., Russell, T. P. & Emrick, T. Microcapsules of PEGylated gold nanoparticles prepared by fluid-fluid interfacial assembly. Nano Letters 7, 389–393 (2007).
Joki, T. et al. Continuous release of endostatin from microencapsulated engineered cells for tumor therapy. Nat. Biotechnol. 19, 35–39 (2001).
Jackson, A. M., Myerson, J. W. & Stellacci, F. Spontaneous assembly of subnanometre-ordered domains in the ligand shell of monolayer-protected nanoparticles. Nat. Mater. 3, 330–336 (2004).
Miyamoto, S. & Kollman, P. A. Settle - an Analytical Version Of the Shake and Rattle Algorithm for Rigid Water Models. J. Comp. Chem. 13, 952–962 (1992).
Ryckaert, J.-P., Ciccotti, G. & Berendsen, H. J. C. Numerical integration of the cartesian equations of motion of a system with constraints: molecular dynamics of n-alkanes. J.Comp. Phys. 23, 327–341 (1977).
Schweighofer, K. J., Essmann, U. & Berkowitz, M. Structure and dynamics of water in the presence of charged surfactant monolayers at the water-CCl4 interface. A molecular dynamics study. J. Phys. Chem. B 101, 10775–10780 (1997).
Berendsen, H. J. C., Postma, J. P. M., Gunsteren, W. F. v., DiNola, A. & Haak, J. R. Molecular dynamics with coupling to an external bath. J. Chem. Phys. 81, 3684–3690 (1984).
Boker, A., He, J., Emrick, T. & Russell, T. P. Self-assembly of nanoparticles at interfaces. Soft Matter 3, 1231–1248 (2007).
Edwards, E. W., Chanana, M. & Wang, D. Y. Capping gold nanoparticles with stimuli-responsive polymers to cross water-oil interfaces: In-depth insight to the trans-interfacial activity of nanoparticles. J. Phys. Chem. C 112, 15207–15219 (2008).
Shi, W.-X. & Guo, H.-X. Structure, Interfacial Properties, and Dynamics of the Sodium Alkyl Sulfate Type Surfactant Monolayer at the Water/Trichloroethylene Interface: A Molecular Dynamics Simulation Study. J. Phys. Chem. B 114, 6365–6376 (2010).
Abbott, N. L. & MacKay, R. A. Surfactant applications. Curr. Opin. Colloid Interface Sci. 4, 323–324 (1999).
Garti, N. Microemulsions as microreactors for food applications. Curr. Opin. Colloid Interface Sci. 8, 197–211 (2003).
Sintov, A. C. & Botner, S. Transdermal drug delivery using microemulsion and aqueous systems: Influence of skin storage conditions on the in vitro permeability of diclofenac from aqueous vehicle systems. Int. J. Pharm. 311, 55–62 (2006).
Cilek, A., Celebi, N. & Tirnaksiz, F. Lecithin-based microemulsion of a peptide for oral administration: Preparation, characterization, and physical stability of the formulation. Drug Deliv. 13, 19–24 (2006).
Araya, H., Tomita, M. & Hayashi, M. The novel formulation design of O/W microemulsion for improving the gastrointestinal absorption of poorly water soluble compounds. Int. J. Pharm. 305, 61–74 (2005).
Gupta, S. et al. Designing and testing of an effective oil-in-water microemulsion drug delivery system for in vivo application. Drug Deliv. 12, 267–273 (2005).
Gulsen, D., Li, C. C. & Chauhan, A. Dispersion of DMPC liposomes in contact lenses for ophthalmic drug delivery. Curr. Eye Res. 30, 1071–1080 (2005).
Schwuger, M. J., Stickdorn, K. & Schomacker, R. Microemulsions in technical processes. Chem. Rev. 95, 849–864 (1995).
Acosta, E. Bioavailability of nanoparticles in nutrient and nutraceutical delivery. Curr. Opin. Colloid Interface Sci. 14, 3–15 (2009).
Berendsen, H. J. C., Vanderspoel, D. & Vandrunen, R. Gromacs - A message-passing parallel molecular-dynamics implementation. Comput. Phys. Commun. 91, 43–56 (1995).
Van der Spoel, D. et al. Gromacs: Fast, flexible, and free. J. Comp. Chem. 26, 1701–1718 (2005).
Schweighofer, K. J., Essmann, U. & Berkowitz, M. Simulation of sodium dodecyl sulfate at the water-vapor and water-carbon tetrachloride interfaces at low surface coverage. J. Phys. Chem. B 101, 3793–3799 (1997).
Dominguez, H. Computer Simulations of Surfactant Mixtures at the Liquid/Liquid Interface. J. Phys. Chem. B 106, 5915–5924 (2002).
Bruce, C. D., Berkowitz, M. L., Perera, L. & Forbes, M. D. E. Molecular dynamics simulation of sodium dodecyl sulfate micelle in water: Micellar structural characteristics and counterion distribution. J. Phys. Chem. B 106, 3788–3793 (2002).
Schuttelkopf, A. W. & van Aalten, D. M. F. PRODRG: a tool for high-throughput crystallography of protein-ligand complexes. Acta Crystallogr D 60, 1355–1363 (2004).
Berendsen, H. J. C., Postma, J. P. M., van Gunsteren, W. F. & Hermans, J. In Intermolecular Forces: Proceedings of the Fourteenth Jerusalem Symposium on Quantum Chemistry and Biochemistry Held in Jerusalem, Israel, April 13–16, 1981 (ed Bernard, Pullman ) 331–342 (Springer Netherlands, 1981).
Darden, T., York, D. & Pedersen, L. Particle Mesh Ewald - an N.Log(N) Method for Ewald Sums in Large Systems. J. Chem. Phys. 98, 10089–10092 (1993).
Essmann, U. et al. A Smooth Particle Mesh Ewald Method. J. Chem. Phys. 103, 8577–8593 (1995).
Morrow, M. R., Singh, D., Lu, D. L. & Grant, C. W. M. Glycosphingolipid Acyl Chain Orientational Order in Unsaturated Phosphatidylcholine Bilayers. Biophys. J. 64, 654–664 (1993).
Hong, B., Qiu, F., Zhang, H. & Yang, Y. Budding Dynamics of Individual Domains in Multicomponent Membranes Simulated by N-Varied Dissipative Particle Dynamics. J. Phys. Chem. B 111, 5837–5849 (2007).
Huang, R. & Im, S. H. Dynamics of wrinkle growth and coarsening in stressed thin films. Phys. Rev. E 74, 12 (2006).
Shinoda, W., DeVane, R. & Klein, M. L. Zwitterionic Lipid Assemblies: Molecular Dynamics Studies of Monolayers, Bilayers, and Vesicles Using a New Coarse Grain Force Field. J. Phys. Chem. B 114, 6836–6849 (2010).
Cerda, E. & Mahadevan, L. Geometry and physics of wrinkling. Phys. Rev. Lett. 90, 4 (2003).
Li, B., Huang, S. Q. & Feng, X. Q. Buckling and postbuckling of a compressed thin film bonded on a soft elastic layer: a three-dimensional analysis Arch Appl Mech 80, 175–188 (2010).
Lu, W., Knobler, C. M., Bruinsma, R. F., Twardos, M. & Dennin, M. Folding Langmuir monolayers. Phys Rev Lett 89, 146107 (2002).
Langevin, D. Viscoelasticity of Monolayers. Encyclopedia of Surface and Colloid Science: Second Edition, 6547–6561 (2006).
Baoukina, S., Monticelli, L., Risselada, H. J., Marrink, S. J. & Tieleman, D. P. The molecular mechanism of lipid monolayer collapse. Proc. Natl. Acad. Sci. USA 105, 10803–10808 (2008).
Mackie, A. R., Gunning, A. P., Wilde, P. J. & Morris, V. J. Competitive Displacement of Lactoglobulin from the Air/Water Interface by Sodium Dodecyl Sulfate. Langmuir 16, 8176–8181 (2000).
Acknowledgements
W.X.S. thanks the discussion with Prof. Li Shuzhou and the support from MOE Tier 2 (ACR12/12).
Author information
Authors and Affiliations
Contributions
W.X.S. designed molecular dynamics simulations; W.X.S. performed molecular dynamics simulations; W.X.S. wrote the manuscript. All authors reviewed the manuscript.
Ethics declarations
Competing interests
The author declares no competing financial interests.
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Shi, W. The structure and dynamics of Nano Particles encapsulated by the SDS monolayer collapse at the water/TCE interface. Sci Rep 6, 37386 (2016). https://doi.org/10.1038/srep37386
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep37386
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.