Abstract
Semiconductor nanowires are a promising candidate for next-generation solar cells. However, the optical response of nanowires is, due to diffraction effects, complicated to optimize. Here, we optimize through optical modeling the absorption in a dual-junction nanowire-array solar cell in terms of the Shockley-Quessier detailed balance efficiency limit. We identify efficiency maxima that originate from resonant absorption of photons through the HE11 and the HE12 waveguide modes in the top cell. An efficiency limit above 40% is reached in the band gap optimized Al0.10Ga0.90As/In0.34Ga0.66As system when we allow for different diameter for the top and the bottom nanowire subcell. However, for experiments, equal diameter for the top and the bottom cell might be easier to realize. In this case, we find in our modeling a modest 1–2% drop in the efficiency limit. In the Ga0.51In0.49P/InP system, an efficiency limit of η = 37.3% could be reached. These efficiencies, which include reflection losses and sub-optimal absorption, are well above the 31.0% limit of a perfectly-absorbing, idealized single-junction bulk cell and close to the 42.0% limit of the idealized dual-junction bulk cell. Our results offer guidance in the choice of materials and dimensions for nanowires with potential for high efficiency tandem solar cells.
Similar content being viewed by others
Introduction
The use of III-V nanowires for p-i-n junction solar cells is an emerging avenue for photovoltaics1,2,3,4,5,6. Both single wire4,6,7 and large-area nanowire array5,8,9 devices show promise for next generation solar cells. Already for single nanowire systems, diffraction of light can lead to resonant coupling of light into the nanowire with several absorption peaks as a function of wavelength10,11. Optimization of the geometry of the single-nanowire geometry is necessary to obtain maximum photocurrent12 and open circuit voltage4,12. The resonances can lead to a 20 times stronger absorption per volume semiconductor material in a III-V nanowire as compared to a bulk sample13.
An array of nanowires gives in turn access to large-area devices when higher output power is needed. For such arrays, an efficiency of 13.8% has been demonstrated using InP nanowires with a single p-i-n junction in the axial direction5 and an efficiency of 15.3% has been reached with GaAs nanowires9. However, the use of a single material gives an upper limit for the amount of sun light that can be converted into electrical energy14, due to two reasons. First, the energy of photons with energy below the band gap energy of the semiconductor cannot be utilized since those low-energy photons cannot be absorbed. Second, a large part of the energy of absorbed high-energy photons is wasted due to thermalization. In this thermalization process, the photogenerated electrons and holes relax in energy to their respective band edges.
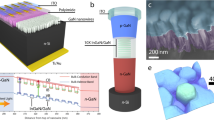
To reach higher efficiencies in solar cells, an avenue is to use multiple semiconductors, epitaxially grown on top of each other15. See Fig. 1 for a system with two different semiconductor materials, where one material is used in the top cell and a different material in the bottom cell. The idea in such a tandem device is to absorb high energy photons in a high band gap top cell. In that top cell, the thermalization loss of the high energy photons is decreased compared to the single junction cell. The lower energy photons continue to the bottom cell where they are absorbed. Due to the lower band gap of the bottom cell than in the single junction cell, more photons are absorbed than in the single junction cell. In this way, the tandem cell can absorb more photons than the single junction cell, while at the same time having reduced thermalization losses. However, in planar cells, the crystal lattice constant between materials in adjacent subcells/layers should be matched to yield high-quality materials without performance limiting dislocations. Such requirements on crystal-lattice matching limit strongly the choice of materials for tandem cells.
Nanowire structures offer a clear benefit for multi-junction solar cells compared with planar cells. Efficient strain relaxation in nanowires allows for the fabrication and combination of dislocation-free, highly lattice-mismatched materials16,17,18,19. Furthermore, III-V semiconductor nanowire arrays can in principle be fabricated on top of a Si substrate19, giving the prospect of using the Si substrate as the bottom cell2,20,21,22,23.
Thus, nanowires offer freedom for the material choice in multi-junction solar cells, making it easy to reach optimum material combinations to match the solar spectrum. Furthermore, the resonant absorption by designing the nanowire geometry holds the prospect of lower material usage than in thin-films13. Therefore, to enable high-efficiency nanowire tandem solar cells, we need to understand the optimum choice of materials for the subcells as well as the optimum nanowire geometry to have the best absorption characteristics for photovoltaics. Already for single junction nanowire-array cells, we know that both the array pitch and the nanowire diameter need to be optimized simultaneously. At the same time, the optimum diameter depends on the band gap of the solar cell, that is, on the material choice8.
Here, we perform optical modeling to calculate and optimize the absorption of light in a dual junction tandem nanowire solar cell (Fig. 1) with the scattering matrix method1,8,13,24,25. This modeling allows us to perform a Shockley-Queisser detailed balance analysis to study and optimize the efficiency potential of the nanowire solar cell as a function of material choice and geometrical design of the nanowires. We show that an efficiency limit above 40% can be reached in the band gap optimized Al0.10Ga0.90As/In0.34Ga0.66As system when we allow for different diameter Dtop and Dbot for the top and the bottom subcell. However, for experiments, the case of Dtop = Dbot might be easier to realize. In this case, we find a 1–2% drop in the efficiency. In the experimentally relevant Ga0.51In0.49P/InP system, an efficiency limit of η = 37.3% is reached for a nanowire length of 13 μm when using equal diameters of Dtop = Dbot = 160 nm and a pitch P = 380 nm (we analyze also the effect of varying nanowire length, with results summarized in Table 1). These efficiencies for nanowire tandem cells are well above the 31.0% limit of an idealized, perfectly absorbing single-junction bulk cell and close to the 42.0% limit of the idealized, band gap optimized dual-junction bulk cell.
(a,d) Optimized Shockley-Queisser detailed balance efficiency as a function of Ltop. Here, values for the HE11 maximum (blue line) and the HE12 maximum (green line) are shown. These resonances show up when the HE11/HE12 waveguide resonance enhances absorption in the top cell for wavelengths close to the bandgap wavelength of the top cell. We show also the maximum efficiency (dashed red line) when we force a single diameter throughout the nanowire (Dbot = Dtop). The dashed black line shows the efficiency limit for the dual-junction cell under the assumption of perfect absorption in both the top and the bottom subcell. (b,e) P (solid line) Dtop (dashed line) and Dbot (dotted line) at the maximum efficiency point. The color of the lines denotes the corresponding maximum as in (a,d), that is, blue for HE11, green for HE12 and red for Dbot = Dtop. (c,f) Similar as (b,e) but for Lbot. The material of the top and the bottom subcell is shown in the title of each subfigure.
Material choice for the top and the bottom cell in a nanowire tandem solar cell
To choose the materials for the top and the bottom nanowire subcell, we perform the well-known Shockley-Queisser detailed balance analysis14 assuming first perfect absorption of above band gap photons in each subcell22,26. This analysis corresponds to the case when each subcell absorbs optimally, without reflection losses. The specific assumption and technical details of the analysis can be found in the Supplementary Information.
Importantly, we assume that cell 1 absorbs all photons of energy above E1, the band gap energy of cell 1. Cell 2 absorbs in turn all photons with energies between E1 and E2, the band gap energy of cell 2. We assume that A1(λ) = 1 for λ < λ1,bg and A1(λ) = 0 otherwise. Here, A1(λ) [A2(λ)] is the absorption spectrum of cell 1 (2), that is, the fraction of incident light of wavelength λ absorbed in cell 1 (2). Similarly, we assume that A2(λ) = 1 for λ1,bg < λ < λ2,bg and A2(λ) = 0 otherwise. Here, λ1,bg = 2πћc/E1 and λ2,bg = 2πћc/E2. In this way, we find the materials that maximize the efficiency limit of the nanowire solar cell when the geometry is designed for optimum absorption (Fig. 2). Note that below, in the section Geometry Design, when considering the effect of the nanowire geometry on the absorption, we model the absorption spectra A1(λ) ≤ 1 and A2(λ) ≤ 1 for each choice of the geometry, which includes sub-optimal absorption and varying reflection losses.
Shockley-Queisser detailed balance efficiency as a function of material band gaps for perfectly absorbing subcells.
The maximum efficiency η = 42.0% shows up at E1 = 1.58 eV and E2 = 0.95 eV for the top and the bottom cell band gap, respectively. Notice that in this analysis for the perfectly absorbing subcells, for E1 < E2 the bottom cell (cell 2) does not absorb any photons. Thus, j2 = 0 and consecutively the current through this current-matched series-connected solar cell is zero, leading to η = 0. The inset shows the efficiency limit for varying top cell band gap for the case of an InP bottom cell, that is, when E2 = 1.34 eV.
We find a maximum efficiency of 42.0% when the band gaps of the top and the bottom cell are E1 = 1.58 eV and E2 = 0.95 eV respectively (Fig. 2). Note that the results in Fig. 2 are in good agreement with previous detailed-balance calculations of multi-junction bulk cells22,26. In our case, for the modeling of the emission to the substrate, we use a refractive index of n = 3.5 to represent the InP substrate. We note that the emission of photons into this high-refractive index substrate has caused a 3% decrease in this maximum efficiency.
To choose the III-V materials for the nanowire subcells, we calculated first the band gap for varying ternary compounds27. After this, we investigated which ternary compounds have tabulated, experimentally determined, reliable refractive index values available for the optics modelling. Among the ternaries for which such refractive index data were readily available, we identified Al0.10Ga0.90As (band gap of 1.55 eV27 and refractive index from ref. [28]) for the top cell and In0.34Ga0.66As (band gap of 0.95 eV27 and refractive index from ref. [29]) for the bottom cell as a good material combination with an efficiency limit of 40.7%.
However, we could imagine that the fabrication of a dual-junction nanowire solar cell could benefit from the knowledge and control of the fabrication of single-junction nanowire solar cells. In this case, the natural candidates are the well-performing InP5 and GaAs9. The band gaps of both these materials work well for the bottom cell (see inset in Fig. 2 for an InP bottom cell and Supplementary Figure S1 for a GaAs bottom cell). However, the surface recombination velocity of unpassivated GaAs can be five orders of magnitude higher than that of unpassivated InP30. Therefore, GaAs nanowires need dedicated surface passivation schemes,9 whereas the requirement on surface passivation is relieved for InP nanowires5. Therefore, we chose to concentrate on an InP bottom cell. Here, a maximum efficiency of 38.6% is found with a top cell band gap energy of 1.86 eV (inset of Fig. 2). We note that Ga0.51In0.49P, for which refractive index data is available31, has a band gap energy of 1.85 eV31, giving an efficiency limit of 38.5% in the tandem configuration with InP. Depending on the surface properties of the GaInP, this GaInP/InP system could perhaps even provide the prospect of high efficiency without dedicated surface passivation schemes. Thus, we study the efficiency limit of both the AlGaAs/InGaAs and the GaInP/InP system.
Geometry Design
After choosing the materials for the top and the bottom cell as described above (the nearly band gap optimized Al0.10Ga0.90As/In0.34Ga0.66As system as well as the technologically relevant Ga0.51In0.49P/InP system), we turn to consider the geometry of the nanowire subcells (Fig. 1). There are five geometry parameters: the length of each subcell (Ltop and Lbot), the diameter of each subcell (Dtop and Dbot) and the pitch (P) of the square array, which need to be optimized with respect to the absorption (A1(λ) and A2(λ)) of light in each subcell.
Different computational methods, such as the finite-element method (FEM)3,32,33, the rigorous coupled wave analysis (RCWA)4,6 and the scattering matrix method1,8,13,24,25, have been used for studying the diffraction and absorption of light in nanostructures through the solution of the Maxwell equations, which give results in good agreement with experiments13. We chose to employ the scattering matrix method to solve the Maxwell equations for normally incident light in order to calculate the absorption spectrum A1(2)(λ) of the nanowire top and bottom cells. We use tabulated refractive index values n(λ) for the Al0.10Ga0.90As28, In0.34Ga0.66As29, Ga0.51In0.49P31 and InP34. We then calculate the Shockley-Queisser detailed balance efficiency (see Supplementary Information Eqs. (S1)–(S6) for technical details).
Note that the optics modeling is done with the nanowires on top of an InP substrate (see Fig. 1). However, absorption of light in the substrate does not contribute to the current or voltage of the solar cell in our analysis. Thus, the substrate functions optically merely to partially reflect the light that reaches the substrate. Therefore, a change to a different substrate, like the less-expensive Si19, with similar n ≈ 3.5 as the InP would give very similar absorption spectra.
We start by considering the case of Al0.10Ga0.90As (band gap of E1 = 1.55 eV) for the top cell and In0.34Ga0.66As (band gap of E2 = 0.95 eV) for the bottom cell, which was found to be a good band gap combination with efficiency limit of 40.7% for perfectly absorbing subcells.
It is known that the nanowire diameter affects strongly the absorption of light1,5,7,8,13,35. Therefore, to study the effect of the nanowire diameter on the absorption in the tandem cell, we fix P = 530 nm, Ltop = 2000 nm and Lbot = 2900 nm [Fig. 3(a)]. As a main feature: the efficiency appears to be a function of just Dtop when Dbot is large enough (typically when Dbot > 250 nm). In this case of large Dbot, two local maxima show up in the efficiency as a function of Dtop. To show these maxima clearly, we set Dbot to a fix value of 470 nm. Here, these two efficiency peaks show up at a top cell diameter of Dtop = 150 nm and Dtop = 345 nm, respectively [Fig. 3(b)].
(a) Efficiency limit as a function of Dtop and Dbot for P = 530 nm, Ltop = 2000 nm and Lbot = 2900 nm. Here, the top cell is of Al0.10Ga0.9As and the bottom cell of In0.34Ga0.66As. (b) Efficiency limit as a function of top cell diameter as extracted from the dashed black line in (a). (c,d) Absorption spectra (red and green lines) for the diameters marked by the vertical lines in (b). Here, the diameter increases in the order of dashed, solid and dashed dotted line. We show also the normalized number of available incident photons as a function of wavelength (blue line). (e,f) Photogenerated current jph1(ph2) in (e) the top cell and (f) the bottom cell, respectively.
To understand the origin of these two efficiency maxima, we study the number of incident photons as a function of wavelength [blue line in Fig. 3(c,d)]. In the region 600 nm to 800 nm, the solar spectrum shows the highest number of incident photons as a function of wavelength. Since we assume that each absorbed photon contributes one charge carrier to the photogenerated current, strong absorption in this wavelength region is very important for j and consequently to the efficiency.
Therefore, we study the absorption spectrum in the top cell as a function of the diameter in the top cell around Dtop = 150 nm and Dtop = 345 nm, respectively, where the two local maxima in η show up. In Fig. 3(c), when the diameter increases from 120 nm to 150 nm, we find an absorption peak in the spectrum and it moves from about 600 nm to 700 nm8,36,37. This peak can be explained as resonant coupling of incident light into the HE11 waveguide mode of the individual nanowires. This resonant coupling leads to enhanced absorption in nanowire arrays36. When Dtop increases further to 180 nm [red dotted line in Fig. 3(b)], the absorption peak has started to vanish since it red-shifts beyond the bandgap wavelength. This shifting and disappearance of the absorption peak leads consequently to a small decrease in the efficiency as Dtop increases from 150 nm to 180 nm.
Similarly, in the case of Dtop = 345 nm we find again an absorption peak at λ ≈ 700 nm [Fig. 3(d)]. This time, the absorption peak originates from the higher order HE12 waveguide mode. This absorption peak has red-shifted beyond the band gap wavelength when Dtop has increased to 375 nm [Fig. 3(d)], leading to a slight decrease in the efficiency. Thus, we find an efficiency maximum for the nanowire tandem solar cell [Fig. 3(a)] when Dtop is optimized to place the HE11 or the HE12 absorption peak just below the band gap wavelength. Very similar results have been reported for the diameter optimization of a single junction InP nanowire solar cell8.
To understand why the efficiency does not noticeably depend on Dbot for Dbot > 250 nm [Fig. 3(a)], we study the photogeneration of charges in the top cell (jph1) and the bottom cell (jph2) [Fig. 3(e,f)]. Since j = j1 = j2 and j1 ≤ jph1 and j2 ≤ jph2 (see Supplementary Information for details), the smaller one of jph1 and jph2 is expected to limit the solar cell efficiency [Fig. 3(a)]. When Dtop < 100 nm, the total current of the tandem cell is strongly limited by jph1. As the diameter of the top cell increases, jph1 can increase to about 20 mA/cm2. However, when the bottom cell diameter is larger than 250 nm, jph2 > 20 mA/cm2. Thus, for Dbot > 250 nm, jph2 > jph1 and the efficiency follows the absorption properties of the current-limiting top cell and therefore depends mainly on Dtop and only very weakly on Dbot.
We note that for the bottom cell, we find a pronounced maximum in jph2 as a function of Dbot for Dbot ≈ 250 nm when Dtop ≈ 0. We assign this maximum in jph2 to the HE11 resonance in the bottom cell. We notice that in Fig. 3(f), that maximum is to a large degree overshadowed for Dtop > 0 by the strong dependence of jph2 on Dtop. When we study the dependence of the efficiency on Dbot for a fixed Dtop (see Supplementary Figure S2), we find that the maximum at Dbot ≈ 250 nm shows up also for Dtop > 0 and broadens with increasing Dtop.
Thus, we have found above two clear local maxima for η, one for Dtop = 150 nm and one for Dtop = 345 nm that originate, respectively, from resonant absorption through the HE11 and HE12 modes in the top cell. However, the results above were derived for a fixed Ltop, Lbot and P. Next, we optimize the efficiency limit for all these five parameters (Dtop, Dbot, Ltop, Lbot and P) simultaneously. To make the optimization numerically feasible, we introduced a numerically efficient iteration process (See Supplementary Information for details). We choose to show the results in Fig. 4 as a function of top cell length Ltop. For tabulated values of the optimized geometry, see Supplementary Information Table S1. For a more complete dependence of the efficiency on the geometrical parameters, see Supplementary Figures S3–S14. Notably, with proper design, an efficiency limit above 40% can be reached by the use of Al0.10Ga0.90As for the top cell and In0.34Ga0.66As for the bottom cell [blue line, when Ltop > 6 μm, in Fig. 4(a)].
In this optimization, we can identify maxima in η to originate from the above discussed HE11 and HE12 resonances in the top cell [Fig. 4(a)]. In the region of Ltop > 600 nm, the HE11 resonance of the top cell leads to a higher efficiency limit than that of the HE12 resonance. These results are in agreement with those for a single junction nanowire array solar cell where the HE11 resonance usually leads to the highest efficiency8. For the dual junction cell here, we call these maxima for brevity the HE11 and HE12 maxima/optima.
For a single junction nanowire cell8, rough values for the optimum diameter were estimated as

Here,  is the real part of the refractive index (at the band gap wavelength) and DHE11(HE12) is the diameter that optimizes the wavelength position of the HE11 and HE12 resonance in order to maximize η. The value for the constant cHE11(12) can be extracted from the work on the single-junction nanowire solar cells8.
is the real part of the refractive index (at the band gap wavelength) and DHE11(HE12) is the diameter that optimizes the wavelength position of the HE11 and HE12 resonance in order to maximize η. The value for the constant cHE11(12) can be extracted from the work on the single-junction nanowire solar cells8.
The diameter for the HE11 (HE12) resonance of the top cell in Fig. 4(b) is Dtop ≈ 150 nm (Dtop ≈ 345 nm) in qualitative agreement with values from Equation (1) [about 169 nm for HE11 and 394 nm for HE12 resonance]. We find that Dbot fluctuates only slightly when Dtop ≈ 150 nm to yield the HE11 maximum (blue dotted line in Fig. 4(b)). In contrast, Dbot fluctuates more at the HE12 maximum (green dotted line in Fig. 4(b)). This fluctuation in Dbot is understood from the fact that for the HE12 maximum at Dtop ≈ 345 nm, the efficiency shows a very broad maximum in Dbot (black dashed line in Fig. 3a and Supplementary Figure S2), which allows for large variations in Dbot when Ltop, Lbot and P are optimized.
Similarly as for the single nanowire case8, we find that the optimum pitch P [solid lines in Fig. 4(b)] tends to increase with increasing nanowire length, that is, with increasing Ltop and Lbot. This behavior can be understood as a competition between increased absorption and increased reflection with decreasing P8. With increasing nanowire length, the absorption increases and we can allow for a larger P to decrease reflection losses.
In our results, we find that Lbot > Ltop [Fig. 4(f)]. However, the efficiency tends to increase as a function of Lbot (see Supplementary Figures S3–S14) and therefore whether we end up in the case of Lbot > Ltop or in the case of Lbot < Ltop depends on how heavily we maximize the efficiency η at the cost of increasing Lbot. We allowed the optimization to stop with respect to Lbot when we reached a value of dη/dLbot < 0.001 μm−1 in our geometry optimization (see Supplementary Information). In this case, for all the considered Ltop, the optimized value for Lbot ended up slightly larger than Ltop.
For fabrication purposes, it could be a benefit to consider Dtop = Dbot, that is, nanowires of a single diameter D throughout (see the red dashed line in Fig. 4a for the resulting efficiency). We found in this case large fluctuations in the optimum value of Lbot when Ltop is increasing (the fluctuation in Lbot could be larger than the value of Ltop). To be able to analyze this case as a function of Ltop, we set an upper limit of Ltop+1000 nm for Lbot.
We find an interesting behavior for the optimized diameter D for these single-diameter nanowires [red dashed line in Fig. 4(b)]. For the smallest considered Ltop of 500 nm, D starts close to the Dtop ≈ DHE11 ≈ 150 nm of the HE11 maximum for the case in which we allow for Dtop ≠ Dbot. When Ltop increases toward the largest considered value of 8000 nm, D increases toward the value of the Dbot ≈ 200 nm which optimizes the HE11 maximum in the Dtop ≠ Dbot case. This behavior can be understood as follows. When Ltop is small, the absorption in the top cell is weak in relative terms and photons also in the short wavelength region can reach the bottom cell due to insufficient absorption in the top cell. As a result, the current and therefore the efficiency, of the solar cell is limited by absorption in the top cell. As a consequence, the optimum D occurs when the absorption in the top cell is optimized for, which happens at D ≈ DHE11. In contrast, when Ltop is large, the absorption in the top cell is instead strong and the performance of the solar cell becomes limited by the current-generation in the bottom cell, which is optimized for D in a similar way as when Dtop ≠ Dbot. Thus, for large Ltop, D goes toward the Dbot that optimizes the HE11 maximum.
Since we find the optimum for D close to the diameters found for the HE11 maximum in the Dtop ≠ Dbot case, we find, not completely surprisingly, values for P close to those of the HE11 case of Dtop ≠ Dbot. As an end result, we find that the efficiency for this case of D = Dtop = Dbot is typically 1 to 2% lower than when we allow for Dtop ≠ Dbot [Fig. 4(a)].
We have also studied the efficiency of the InP based Ga0.51In0.49P/InP nanowire tandem system [Fig. 4(d–f)], with maximum efficiency of 38.5% for perfectly absorbing subcells, which should be set in relation to the limit of 42.0% for the idealized, perfectly absorbing, band gap optimized dual-junction bulk cell. Also for this material choice we reach an efficiency within 2% of this maximum, with Ltop > 6 μm and Lbot > 7 μm, when we allow for Dtop ≠ Dbot. Also here, an additional drop by about 1% occurs with the constraint Dtop = Dbot. With Ga0.51In0.49P and InP as the material and Dtop = Dbot, we reach η = 35.5% when Ltop = 2000 nm and Lbot = 3000 nm, considerably higher than the maximum 31.0% possible in the single junction bulk solar cell case. To aid the reader, we show in Table 1 the values extracted from Fig. 4 for this case of Dtop = Dbot (for the HE11 and HE12 maximum, we refer the reader to Supplementary Information Table S1).
Conclusion
We performed electromagnetic modeling to investigate theoretically the absorption properties of a dual junction nanowire array solar cell. We used then the Shockley-Queisser efficiency limit as a metric for optimizing the materials and geometry of the nanowires. The optimized geometries are presented in Fig. 4, Table 1 and Supplementary Information Table S1. The drop in efficiency limit when moving away from such an optimized geometry is presented in Supplementary Information Figures S3–S14. These results present a guideline for choosing a nanowire geometry that has promise for optimized absorption in a dual-junction nanowire array solar cell. In this way, our results can be used as a starting point for theoretical studies on the optimization of the electrical properties of dual-junction nanowire array solar cells. Our results can also guide in the choice of materials and dimensions for the fabrication of nanowires aimed for tandem solar cells.
Additional Information
How to cite this article: Chen, Y. et al. Design for strong absorption in a nanowire array tandem solar cell. Sci. Rep. 6, 32349; doi: 10.1038/srep32349 (2016).
References
Anttu, N. Shockley-queisser detailed balance efficiency limit for nanowire solar cells. ACS Photonics 2, 446–453 (2015).
Bu, S. et al. Optical and electrical simulations of two-junction III-V nanowires on Si solar cell. Appl. Phys. Lett. 102, 031106 (2013).
Kupec, J., Stoop, R. L. & Witzigmann, B. Light absorption and emission in nanowire array solar cells. Opt. Express 18, 27589–27605 (2010).
Sandhu, S., Yu, Z. & Fan, S. Detailed balance analysis and enhancement of open-circuit voltage in single-nanowire solar cells. Nano Lett. 14, 1011–1015 (2014).
Wallentin, J. et al. InP nanowire array solar cells achieving 13.8% efficiency by exceeding the ray optics limit. Science 339, 1057–1060 (2013).
Sandhu, S., Yu, Z. & Fan, S. Detailed balance analysis of nanophotonic solar cells. Opt. Express 21, 1209–1217 (2013).
Nowzari, A. et al. A comparative study of absorption in vertically and laterally oriented InP core-shell nanowire photovoltaic devices. Nano Lett. 15, 1809–1814 (2015).
Anttu, N. & Xu, H. Q. Efficient light management in vertical nanowire arrays for photovoltaics. Opt. Express 21, A558–A575 (2013).
Åberg, I. et al. A GaAs nanowire array solar cell with 15.3% efficiency at 1 sun. IEEE J. Photovolt. 6, 185–190 (2016).
Cao, L. et al. Engineering light absorption in semiconductor nanowire devices. Nat. Mater. 8, 643–647 (2009).
Hosseinnia, A. & Anttu, N. Absorption through a coupled optical resonance in a horizontal InP nanowire array. Photon. Res. 3, 125–128 (2015).
Krogstrup, P. et al. Single-nanowire solar cells beyond the shockley-queisser limit. Nat. Photonics 7, 306–310 (2013).
Anttu, N. et al. Optical far-field method with subwavelength accuracy for the determination of nanostructure dimensions in large-area samples. Nano Lett. 13, 2662–2667 (2013).
Shockley, W. & Queisser, H. J. Detailed balance limit of efficiency of p-n junction solar cells. J. Appl. Phys. 32, 510 (1961).
Devos, A. Detailed balance limit of the efficiency of tandem solar-cells. J. Phys. D. 13, 839–846 (1980).
Caroff, P. et al. Insb heterostructure nanowires: MOVPE growth under extreme lattice mismatch. Nanotechnology 20, 495606 (2009).
Bjork, M. T. et al. One-dimensional steeplechase for electrons realized. Nano Lett. 2, 87–89 (2002).
Gudiksen, M. S., Lauhon, L. J., Wang, J., Smith, D. C. & Lieber, C. M. Growth of nanowire superlattice structures for nanoscale photonics and electronics. Nature 415, 617–620 (2002).
Mårtensson, T. et al. Epitaxial III-V nanowires on silicon. Nano Lett. 4, 1987–1990 (2004).
Hu, Y., Li, M., He, J. J. & LaPierre, R. R. Current matching and efficiency optimization in a two-junction nanowire-on-silicon solar cell. Nanotechnology 24, 065402 (2013).
LaPierre, R. R. Theoretical conversion efficiency of a two-junction III-V nanowire on Si solar cell. J. Appl. Phys. 110, 014310 (2011).
Huang, N. F., Lin, C. X. & Povinelli, M. L. Limiting efficiencies of tandem solar cells consisting of III-V nanowire arrays on silicon. J. Appl. Phys. 112, 064321 (2012).
Benali, A. et al. Optical simulation of multijunction solar cells based on III-V nanowires on silicon. Energy Procedia 60, 109–115 (2014).
Anttu, N. et al. Crystal phase-dependent nanophotonic resonances in InAs nanowire arrays. Nano Lett. 14, 5650–5655 (2014).
Anttu, N. & Xu, H. Q. Scattering matrix method for optical excitation of surface plasmons in metal films with periodic arrays of subwavelength holes. Phys. Rev. B 83, 165431 (2011).
Strandberg, R. Detailed balance analysis of area de-coupled double tandem photovoltaic modules. Appl. Phys. Lett. 106, 033902 (2015).
Vurgaftman, I., Meyer, J. & Ram-Mohan, L. Band parameters for III-V compound semiconductors and their alloys. J. Appl. Phys. 89, 5815–5875 (2001).
Aspnes, D. E., Kelso, S. M., Logan, R. A. & Bhat, R. Optical properties of AlxGa1−xAs. J. Appl. Phys. 60, 754 (1986).
Kim, T. J. et al. Dielectric functions of InxGa1−xAs alloys. Phys. Rev. B 68, 115323 (2003).
Joyce, H. J. et al. Ultralow surface recombination velocity in InP nanowires probed by terahertz spectroscopy. Nano Letters 12, 5325–5330 (2012).
Levinshtein, M., Rumyantsev, S. & Shur, M. Handbook series on semiconductor parameters Vol. 2: Ternary And Quaternary III-V Compounds (World Scientific, 1996).
Wang, S., Yan, X., Zhang, X., Li, J. & Ren, X. Axially connected nanowire core-shell p-n junctions: A composite structure for high-efficiency solar cells. Nanoscale Res. Lett. 10, 22 (2015).
Kupec, J. & Witzigmann, B. Dispersion, wave propagation and efficiency analysis of nanowire solar cells. Opt. Express 17, 10399–10410 (2009).
Glembocki, O. J. & Piller, H. “Indium phosphide (InP)” In Handbook of optical constants of solids (ed E. D. Palik ) (Academic Press, Burlington) pp. 503–516 1997.
Fan, Z. et al. Ordered arrays of dual-diameter nanopillars for maximized optical absorption. Nano Lett. 10, 3823–3827 (2010).
Wang, B. & Leu, P. W. Tunable and selective resonant absorption in vertical nanowires. Opt. Lett. 37, 3756–3758 (2012).
Aghaeipour, M. et al. Tunable absorption resonances in the ultraviolet for InP nanowire arrays. Opt. Express 22, 29204–29212 (2014).
Acknowledgements
This work was performed within NanoLund and received funding from the People Programme (Marie Curie Actions) of the European Union’s Seventh Framework Programme (FP7-People-2013-ITN) under REA grant agreement No. 608153, PhD4Energy and the European Union’s Horizon 2020 research and innovation programme under grant agreement No. 641023, NanoTandem. This article reflects only the author’s view and the Funding Agency is not responsible for any use that may be made of the information it contains.
Author information
Authors and Affiliations
Contributions
Y.C. performed the simulations, analyzed and interpreted the results and wrote the paper. M.E.P. and N.A. initiated and supervised the study and interpreted the results. All authors contributed to writing and editing the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Chen, Y., Pistol, ME. & Anttu, N. Design for strong absorption in a nanowire array tandem solar cell. Sci Rep 6, 32349 (2016). https://doi.org/10.1038/srep32349
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep32349
This article is cited by
-
InP/GaInP nanowire tunnel diodes
Nano Research (2018)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.