Abstract
Polycrystalline (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) samples were synthesized using solid-state reaction and characterized by X-ray diffraction (XRD). The effect of Zn doping upon saturation magnetization (MS) indicates that the Zn favors to occupying Cu(II) square pyramidal crystallographic site. The AC susceptibility (χ′ac) was measured at various temperatures (χ′ac–T) and magnetic field strengths (χ′ac–H). The Zn doping concentration is found to affect greatly the M-T and χ′ac-T. The skyrmion phase has been inferred from the χ′ac-H data and then indicated within the H-T phase diagrams for various Zn doping concentrations. The striking and unexpected observation is that the skyrmion phase region becomes split upon Zn doping concentration. Interestingly, second conical boundary accompanied by second skyrmion phase was also observed from dχ′ac/dH vs. H curves. Atomic site disorder created by the chemical doping modulates the delicate magnetic interactions via change in the Dzyaloshinskii-Moriya (DM) vector of distorted Cu(II) square pyramidal, thereby splitting of skyrmion phase might occur. These findings illustrate the potential of using chemical and atomic modification for tuning the temperature and field dependence of skyrmion phase of Cu2OSeO3.
Similar content being viewed by others
Introduction
Ever since the discovery of high-Tc superconductivity1, copper oxide materials have garnered significant attention due to exotic physical properties such as charge order stripes2, electronic phase separation3, giant magnetoresistance4 and multiferroics5. This family of materials produce complex and rich phase diagrams because of the strong interplay between their crystal lattices and the spin and orbital degrees of freedoms4. The prime research focus is to address the underlying mechanism behind the physical insight and to establish the technological relevance of these materials.
Recently, a peculiar magnetic state called as “skyrmion or A-phase” has been stabilized in noncentrosymmetric B20 chiral magnetic systems, such as MnSi, FeSi and FeGe6,7,8. Magnetic skyrmion is a topologically stable particle-like spin configuration where spins mold into a vortex-like ordering. More recently, the recognition of skyrmion in insulating spin-1/2 Cu2OSeO39 was reported. It has further triggered the intensive research activity because skyrmion motion can be controlled by external electric fields instead of Joule heating currents, thus making it a good candidate for ultra-low power spintronic devices9,10. The skyrmion lattice can be probed with several experimental methods, such as Lorentz transmission electron microscopy11, magnetic force microscopy12, spin-polarized scanning tunneling microscopy13 and small angle neutron scattering14. The signature of skyrmion lattice can also be detected by relatively simple methods such as magnetic χ′ac15, heat capacity16, topological Hall effect17 and electrical polarization18,19,20.
From the crystallographic view, the common point to both high-Tc superconductor YBa2Cu3O7 and skyrmion Cu2OSeO3 systems is the presence of two different Cu sites. It indicates the microscopic picture of these novel phenomena are hidden in the local structures of two Cu ions and its complex magnetic interactions between Cu(I)/Cu(II) ions. In YBa2Cu3O7, Cu ions are located on two different sites i.e. Cu(I)O chains and Cu(II)O2 planes. The superconductivity mainly originates from the electron transport across the Cu(II)O2 planes21. In Cu2OSeO3, the two Cu2+ ions occupy trigonal bipyramidal (Cu(I)) and square pyramidal (Cu(II)) of oxygen ligands, with the ratio of 1:322. Neutron scattering, μSR and NMR experiments have established the ferrimagnetism of Cu spin with three up (Cu(II)) and one down (Cu(I)) spin alignment22,23,24. However, a close inspection of the magnetic interactions and the crystal lattice of Cu2+ spins reveal the presence of more complex superexchange interactions between the Cu-O-Cu bridges25,26. Interestingly, unexpected two distinct coupled skyrmion sublattices, which arise from the two different magnetic active orbitals i.e. Cu(I) and Cu(II), have been identified in Cu2OSeO3 system using the orbital sensitive resonant X-ray scattering27. It indicates that the site-specific chemical (Cu(I)/Cu(II)) doping might help to gain further insights of this novel skyrmion phase. Following the similar study in high-Tc YBa2Cu3O721, we explore the chemical doping to shed the light on the microscopic origin of these two skyrmion sublattices. The primary goals of this work are to find out what is the role of Cu(I) and Cu(II) sites in the formation of skyrmion phase? What are the effects of nonmagnetic Zn doping on the skyrmion lattice ? Consequently, what are the electronic and magnetic nature of skyrmion phase in Cu2OSeO3?
Results and Discussion
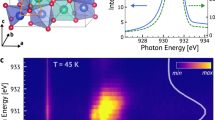
Figure 1(a) shows the X-ray diffraction pattern of Cu2OSeO3 sample. The pattern showed the cubic crystal structure and refined with P213 space group using GSAS Rietveld program. The obtained lattice parameter (a = 8.9219(1) Å) consistent with the previous report indicates the good quality of the sample22. The local structures trigonal bipyramidal and square pyramidal (represented in Fig. 1(b)) determined by the Rietveld method are highly distorted similarly to previous report22. To check the Zn occupation in Cu2OSeO3 crystal, we have performed the room temperature extended X-ray absorption fine structure (EXAFS). EXAFS is highly sensitive technique to probe local crystal structure such as coordination number of ligand and interatomic distance of metal ion to ligand atoms28. The EXAFS spectrum analysis was carried out in Athena software. The moduli of their Fourier transforms |F(R)| are presented in Fig. 1(c,d). As seen from Fig. 1(c), the spectrum for Cu2OSeO3 at Cu K-edge shows the prominent peak around 1.85 Å followed by the small hump at 2.35 Å. To understand the EXAFS spectrum we have compared the EXAFS spectrum with Fig. 1(b). The primary difference between these two structures of Fig. 1(b) is the axial bond length that is longer for the square pyramidal structure. By comparing EXAFS spectrum with the bond lengths of Fig. 1(b), the small hump can be assigned to the axial bond length of Cu(II)-O4 square pyramidal. Fig. 1(d) depicts the |F(R)| spectra for (Cu0.94Zn0.06)2OSeO3 at Cu and Zn K-edges. As shown in the figure the Zn K-edge spectrum qualitatively identical to the Cu K-edge (low radial distance peak might come from other sources such as atomic XAFS and/or multielectron excitations28). As expected Zn spectrum shifted to high radial distances, this indicates the enhancement of bond length due to the changes in the covalent bond formation upon Zn doping. Most importantly, Zn spectrum exhibits the Zn-O4 bond peak even for small doping concentration (6% of Zn) which qualitatively indicates the Zn certainly occupy the Cu(II) site. On the other hand, the low Zn doping concentration hampers the quantitative estimation of Zn at Cu(II) site.
(a) Rietveld refinement of X-ray pattern of Cu2OSeO3 sample. (b) Trigonal bipyramidal and square pyramidal sites of Cu2OSeO3 derived from Rietveld analysis. Fourier transforms moduli radial distribution functions of EXAFS spectra (c) Cu K-edge for Cu2OSeO3. (d) Cu and Zn K-edge for (Cu0.94Zn0.06)2OSeO3 respectively. Arrows near small hump in Fig. 1 (c,d) indicate Cu(II)-O4 and Zn-O4 bond lengths respectively.
Figure 2 depicts the isothermal M-H loops at T = 5 K with the field up to 5 T for (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2). The saturation magnetization MS at 5 T is observed to be 0.519 μB/Cu2+ for x = 0, a close match with the theoretical predicted (0.5 μB/Cu2+) ferrimagnetic alignment of Cu spins9. For the doping case, the nonmagnetic Zn2+ ion might occupy two possible crystallographic (Cu(I)/Cu(II)) sites. If non-magnetic Zn replaces any one of three ferromagnetically aligned Cu(II) sites that decrease the overall magnetization of the unit cell (0.25 μB/Cu2+). On the other hand, Zn placed at Cu(I) site enhances the overall magnetization as well as ferromagnetic strength (0.75 μB/Cu2+). To estimate the probable Zn site occupation we have simulated the MS values for both the case of Cu(I)/Cu(II)) sites. The inset also plots the theoretically predicted values that would be expected for each of doping sites. The measured values line up well with theoretical expectation for the Cu(II) sites, which reveals that the nonmagnetic Zn2+ ions favor occupying the Cu(II) site.
(a) M vs. H curves of (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) series at T = 5 K; Inset shows the MS vs. Zn doping concentration. Dashed lines indicate the theoretically predicted occupation probabilities of Zn at (Cu(I) or Cu(II) crystallographic positions respectively. The experimental data matches the Cu(II) site occupation. (b) The graphical representation of resultant magnetic moment for (I) Cu2OSeO3 (II) Zn at Cu(I) site and (III) Zn at Cu(II) site respectively.
Figure 3 shows the M-T and χ′ac–T curves for (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2). For x = 0, the M-T and χ′ac–T curves are in good agreement with those of measured in single crystal Cu2OSeO329. Moreover, both M-T and χ′ac–T are changed correspondingly and systematically with Zn doping concentration x. Just below ferrimagnetic transition TC ~ 58 K, a clear peak appeared at 56 K shown in χ′ac–T for x = 0 is the hallmark signature of skyrmion phase30. It is noted that the peak is lowered in temperature and becomes fainter with increasing x. On the other hand, a second smaller but notable peak is developed as x ≥ 0.02 and shifted towards lower temperature with increasing x. These results clearly hint the possible formation of second skyrmion phase when x ≥ 0.02.
AC susceptibility is known to be a sensitive technique for revealing coexisting phases in complex magnetic materials, including skyrmion system15,25. The H dependent χ′ac curves at 50–58 K are shown in Fig. 4(a) for Cu2OSeO3. Below the vicinity of peak temperature ~56 K as shown Fig. 3b, the evolution of peak anomalies is noticed in the intermediate field region 100 ≤ H ≤ 400 Oe in χ′ac vs. H curves (Fig. 4(a)). It confirms the growth of skyrmion phase15,25 as T ≤ 56 K. For low temperatures, the peak anomalies suppress in the χ′ac vs. H curves which indicates the skyrmion phase is almost disappeared for T < 52 K. To demonstrate the typical way of extracting the skyrmion phase boundaries, the χ′ac vs. H curves at selected temperatures T1 = 57 K, T2 = 56 K, T3 = 55 K, T4 = 53 K and T5 = 51 K are shown in Fig. 4(b). The H-T phase diagram with skyrmion zone marked in red for Cu2OSeO3 is successfully constructed and displayed in Fig. 4(c). The evolution of different magnetic phase zones, i.e. helical, conical and skyrmion boundaries are in good agreement with previously published results14.
(a) χ′ac vs. H plot at temperatures 50–57 K. (b) Selected temperature curves from (a), but offset each other for easy comparison. The notations of HA1 and HA2 indicate skyrmion phase boundaries while HC1 and HC2 indicate conical phase boundaries respectively. The values of HA1 and HA2 are determined by the peaks while HC1 and HC2 are the inflection points in the first derivative of χ′ac vs. H curves. (c) H vs. T phase diagram for Cu2OSeO3, where the skyrmion phase zone is marked in red.
To investigate the influence of Zn doping on the skyrmion phase of Cu2OSeO3, the χ′ac vs. H for (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) are performed for a broad range of T. The representative results of x = 0.1 are shown in the Fig. 5(a). The characteristic features of the χ′ac vs. H curves for x = 0.1 at 51 K ≤ T ≤ 53 K are comparable to that of Cu2OSeO3 at 52 K ≤ T ≤ 56 K (shown in Fig. 4(a)), that the signature of skyrmion phase is noticed with two peaks. With decreasing temperature to 48 K < T < 51 K, the two peaks become smeared. However, as the temperature is lowered to 47 K ≤ T ≤ 48 K, the signature of skyrmion peaks reappeared for H between 80 and 210 Oe. This unexpected observation of second skyrmion phase is a quite novel phenomenon and never been reported in the Cu2OSeO3 system. Along with second skyrmion signature, χ′ac displays second inflection point in the dχ′ac/dH vs. H (see supplementary material) curves. It might indicate the appearance of the second conical boundary in the phase diagram accompanied with the second skyrmion phase. However, further experimental verification needed to confirm these signatures. It is important to emphasize that, similar atomic doping effect in a metallic skyrmion systems such as Mn1−xFexSi and Mn1−xCoxSi31 lead to Quantum phase transitions with a suppressed of helical magnetic and skyrmion phases. Contradictory, the present study indicates the atomic disorder strongly influence the ground state magnetic properties of the Cu2OSeO3 system that lead to more complex magnetic behavior with the generation of additional novel phases in the H-T phase diagram. The multiple inflection points in dχ′ac/dH vs. H curves are systematically changes with the Zn doping concentration in a selected temperature window, which are displayed in the supplementary material. Following the same plotting procedure as mentioned in Fig. 4(b,c), the Fig. 5(b) is successfully constructed from Fig. 5(a).
Applying the same method as described in Figs 4 and 5, the H-T phase diagrams derived from χ′ac-H data at selected temperatures for each of 8 samples (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) are established and shown in Fig. 6, where the boundaries of conical, helical and skyrmion phases are plotted (with various colors) approximately using limited and discrete data points. The surprising finding is that the single skyrmion phase at x = 0 is splitted into two well-defined small branches in two different temperature regions and about the same magnetic field as x ≥ 0.02. Moreover, the second skyrmion phase is hosted by the second conical phase boundary. Both branches of skyrmion phase are systematically shifted towards low temperature side with x. The opening of temperature gap between two branches of skyrmion phase is larger for higher Zn doping concentration. The second skyrmion phase and its associated conical phase boundaries are firmly decoupled with that of the initial skyrmion phase; this can be clearly visible for the doping concentration x ≥ 0.1. Meanwhile, the high-temperature branch becomes harder to extract from the data as x > 0.15. In fact, the trends of splitting, suppression and decreasing in temperature of skyrmion phases found in Fig. 6 are consistent with those observed in Fig. 3(b).
The splitting of skyrmion phase for Zn doping Cu2OSeO3 is a novel and interesting phenomenon. Similar to high-Tc superconducting materials, it might be associated with the two crystallographic sites of Cu ions and their complex magnetic interactions. From the magnetic point of view, Cu2OSeO3 exhibits a quite complex behavior with several magnetic interactions between Cu(I)/Cu(II) via oxygen bridging25. In the unit cell of Cu2OSeO3 structure, the 4 Cu(I) ions are placed in the undistorted trigonal bipyramidal, whereas 12 Cu(II) ions are distributed among the distorted square pyramidal sites. According to Goodenough-Kramer (G-K) rules, a negative superexchange interaction (<J) corresponds to the orbital overlap angle of Cu ions close to 90° and it goes to positive (>J) if it deviates from 90° 32. A close examination of Cu2OSeO3 using an AC susceptibility technique exposed the antiferromagnetic (AFM) ordering at 59 K followed by ferromagnetic (FM) ordering at 58 K25. The complicated behaviour originates from the unequal strength of three nearest neighbors (NN) AFM interaction of Cu(I)-Cu(II) and three NN FM interaction of Cu(II)-Cu(II) ions25. The crystallography studies in Fig. 1 along with magnetization studies in Fig. 2 suggest that the Cu(II) is the preferable site for Zn doping. Replacing of nonmagnetic Zn2+ for Cu2+ site enhances the Coulombic repulsion of electronic orbital that leads to the decrease in TC. Moreover, the presence of nonmagnetic dopant along with the weak perturbation for the overlaps of electronic orbital can show a significant impact on the complex magnetic exchange interactions between Cu(I)/Cu(II) ions. In general, the helical ground state originates from the competition between Heisenberg superexchange and DM interactions of Cu ions9. The strength of DM interaction depends on the relative change of g-factor from the free electron g-value, i.e. DM ∝ (Δg/g)J, where J is the exchange interaction term33. Symmetry calculation analysis using Raman modes by Gnezdilov et al. indicates the change of DM strength is more significant for the distorted Cu(II) square pyramidal33. The disorder effect is further amplified by doping the nonmagnetic Zn that strongly modulates the DM interaction strength via the change of radial vector and the canting angle between the adjacent spin pairs. Consequently, it manipulates the complex magnetic interactions between Cu(I)/Cu(II) ions.
Similar to resonant X-ray scattering studies on Cu2OSeO3, these two skyrmion phases can be possibly associated with the two Cu sublattices27. Chemical doping might alters the modulation vector of Moirelike skyrmion phase of pure Cu2OSeO3. These results reminiscent the recent observation of unexpected coupled skyrmion sublattices27 and theoretical prediction of novel half skyrmion state in Cu2OSeO3 system34. However, it needs more experimental verifications whether the second skyrmion and its accompanied conical phase are originated from decoupling of coupled Cu skyrmion sublattices or it is related to new kind of spin skyrmion structure. A detailed reciprocal space map using the neutron scattering and scanning tunneling microscope studies are particularly required to shed light on this complex skyrmion behavior. Our findings open up a new pathway for further experimental and theoretical research to elucidate the exotic quantum topological skyrmion phases using chemical doping.
In summary, we have successfully synthesized and well characterized the high quality polycrystalline (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) samples. Zn doped skyrmion exhibits the complex and rich phase diagram. The significant findings are: (1) The dopant Zn is favored to occupy the Cu(II) square pyramid crystallographic site. (2) The M-T and χ′ac-T are changed dramatically with the increase of Zn doping concentration. (3) The skyrmion phase shown in H-T phase diagram of Cu2OSeO3 is split with Zn doping as demonstrated in detailed H and T dependent χ′ac data. (4) Second conical boundary extracted from the dχ′ac/dH vs. H curves accompanied by the second skyrmion phase. All these results suggest a interesting novel scenario and this unexpected observed appearance of second skyrmion and its conical boundary might be related to the way that the Zn doping manipulates the DM vector of the distorted Cu(II)O5 square pyramid through the influence of delicate magnetic interactions. These results point to a new direction of tuning the skyrmion lattice in Cu2OSeO3 by assorted chemical and atomic modification.
Methods
In this study, polycrystalline samples of (Cu1−xZnx)2OSeO3 (0 ≤ x ≤ 0.2) were prepared by solid-state reaction method. Nominal mixtures of high purity CuO, ZnO and SeO2 powders were pressed into pellets. The pellets were sealed in an evacuated quartz tube and heated to a temperature range of 520 °C to 600 °C for 72 h, then slowly cooled over several hours to room temperature. This process was repeated at least twice with intermediate grinding. X-ray diffraction patterns show good quality of samples, with only a minor impurity phases appearing when x ≥ 0.06. Homogeneity of Zn distribution was analyzed with energy dispersive X-ray analysis, which indicated a uniform distribution of Zn throughout the sample (the supplementary material). Temperature and field dependent DC magnetization and AC susceptibility measurements were performed by a SQUID magnetometer (MPMS-XL7, Quantum Design). EXAFS K-edge experiments were carried out in transmission mode for Cu and fluorescence mode for Zn respectively at the 17C beamline in the National Synchrotron Radiation Research Center (NSRRC), Hsinchu, Taiwan.
Additional Information
How to cite this article: Wu, H. C. et al. Unexpected observation of splitting of skyrmion phase in Zn doped Cu2OSeO3. Sci. Rep. 5, 13579; doi: 10.1038/srep13579 (2015).
References
Wu, M. K. et al. Superconductivity at 93 K in a new mixed-phase Y-Ba-Cu-O compound system at ambient pressure. Phys. Rev. Lett. 58, 908–910 (1987).
Mook, H. A., Dai, P. & Doğan, F. Charge and Spin Structure in YBa2Cu3O6.35 . Phys. Rev. Lett. 88, 097004 (2002).
Matsuda, M. et al. Electronic phase separation in lightly doped La2−xSrxCuO4 . Phys. Rev. B 65, 134515 (2002).
Kastner, M. A., Birgeneau, R. J., Shirane, G. & Endoh, Y. Magnetic, transport and optical properties of monolayer copper oxides. Rev. Mod. Phys. 70, 897–928 (1998).
Kimura, T., Sekio, Y., Nakamura, H., Siegrist, T. & Ramirez, A. P. Cupric oxide as an induced-multiferroic with high-TC . Nat. Mater. 7, 291–294 (2008).
Mühlbauer, S. et al. Skyrmion Lattice in a Chiral Magnet. Science 323, 915–919 (2009).
Nagaosa, N. & Tokura, Y. Topological properties and dynamics of magnetic skyrmions. Nat. Nanotech 8, 899–911 (2013).
Huang, S. X. & Chien, C. L. Extended Skyrmion Phase in Epitaxial FeGe(111) Thin Films. Phys.Rev. Lett. 108, 267201 (2012).
Seki, S., Yu, X. Z., Ishiwata, S. & Tokura, Y. Observation of Skyrmions in a Multiferroic Material. Science 336, 198–201 (2012).
Felser, C. Skyrmions. Angew. Chem. Int. Ed. 52, 1631–1634 (2013).
Yu, X. Z. et al. Real-space observation of a two-dimensional skyrmion crystal. Nature 465, 901–904 (2010).
Milde, P. et al. Unwinding of a Skyrmion Lattice by Magnetic Monopoles. Science 340, 1076–1080 (2013).
Kirsten von, B., André, K., Oswald, P. & Roland, W. Interface-induced chiral domain walls, spin spirals and skyrmions revealed by spin-polarized scanning tunneling microscopy. J. Phys.: Conden. Matt 26, 394002 (2014).
Adams, T. et al. Long-Wavelength Helimagnetic Order and Skyrmion Lattice Phase in Cu2OSeO3 . Phys. Rev. Lett. 108, 237204 (2012).
Bauer, A. & Pfleiderer, C. Magnetic phase diagram of MnSi inferred from magnetization and ac susceptibility. Phys. Rev. B 85, 214418 (2012).
Bauer, A., Garst, M. & Pfleiderer, C. Specific Heat of the Skyrmion Lattice Phase and Field-Induced Tricritical Point in MnSi. Phys. Rev. Lett. 110, 177207 (2013).
Neubauer, A. et al. Topological Hall Effect in the A Phase of MnSi. Phys. Rev. Lett. 102, 186602 (2009).
Belesi, M. et al. Magnetoelectric effects in single crystals of the cubic ferrimagnetic helimagnet Cu2OSeO3 . Phys. Rev. B 85, 224413 (2012).
Omrani, A. A. et al. Exploration of the helimagnetic and skyrmion lattice phase diagram in Cu2OSeO3 using magnetoelectric susceptibility. Phys. Rev. B 89, 064406 (2014).
Chu, P. et al. Real-space anisotropic dielectric response in a multiferroic skyrmion lattice. Sci. Rep. 5, 8318 (2015).
Xiao, G. et al. High-temperature superconductivity in tetragonal perovskite structures: Is oxygen-vacancy order important? Phys. Rev. Lett. 60, 1446–1449 (1988).
Bos, J.-W. G., Colin, C. V. & Palstra, T. T. M. Magnetoelectric coupling in the cubic ferrimagnet Cu2OSeO3 . Phys. Rev. B 78, 094416 (2008).
Maisuradze, A. et al. μSR investigation of magnetism and magnetoelectric coupling in Cu2OSeO3 . Phys. Rev. B 84, 064433 (2011).
Belesi, M. et al. Ferrimagnetism of the magnetoelectric compound Cu2OSeO3 probed by 77Se NMR. Phys. Rev. B 82, 094422 (2010).
Živković, I., Pajić, D., Ivek, T. & Berger, H. Two-step transition in a magnetoelectric ferrimagnet Cu2OSeO3 . Phys. Rev. B 85, 224402 (2012).
Yang, J. H. et al. Strong Dzyaloshinskii-Moriya Interaction and Origin of Ferroelectricity in Cu2OSeO3 . Phys. Rev. Lett. 109, 107203 (2012).
Langner, M. C. et al. Coupled Skyrmion Sublattices in Cu2OSeO3 . Phys. Rev. Lett. 112, 167202 (2014).
Teo, B. K. EXAFS: basic principles and data analysis. (Springer-Verlag, 1986).
Huang, C. L. et al. Observation of a second metastable spin-ordered state in ferrimagnet Cu2OSeO3 . Phys. Rev. B 83, 052402 (2011).
Zivkovic, I., White, J. S., Ronnow, H. M., Prsa, K. & Berger, H. Critical scaling in the cubic helimagnet Cu2OSeO3 . Phys. Rev. B 89, 060401(R) (2014).
Bauer, A. et al. Quantum phase transitions in single-crystal Mn1−xFexSi and Mn1−xCoxSi: Crystal growth, magnetization, ac susceptibility and specific heat. Phys. Rev. B 82, 064404 (2010).
Goodenough, J. B. Magnetism and the chemical bond. (Interscience Publishers, 1963).
Gnezdilov, V. P. et al. Magnetoelectricity in the ferrimagnetic Cu2OSeO3: symmetry analysis and Raman scattering study. Low. Temp. Phys. 36, 550–557 (2010).
Janson, O. et al. The quantum nature of skyrmions and half-skyrmions in Cu2OSeO3 . Nature Communications 5, 5376 (2014).
Acknowledgements
This work was supported by the Ministry of Science and Technology, Taiwan, under Grant No. MOST: 103-2112-M-110-010-MY3.
Author information
Authors and Affiliations
Contributions
H.D.Y. supervised the project. K.D.C. and H.C.W. have equal contribution to this work for the characterization and analysis of the experimental data. H.D.Y. and H.B. initiated the original idea. T.Y.W. and T.Y.C. involved in sample synthesis and characterization. K.D.C., H.D.Y. and H.C.W. wrote the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Rights and permissions
This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/
About this article
Cite this article
Wu, H., Wei, T., Chandrasekhar, K. et al. Unexpected observation of splitting of skyrmion phase in Zn doped Cu2OSeO3. Sci Rep 5, 13579 (2015). https://doi.org/10.1038/srep13579
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep13579
This article is cited by
-
Tuning the Disconnected Magnetic Phase by Regulating the Lattice Distortion in FeSi1−xGex Alloys
Journal of Electronic Materials (2023)
-
Multiple low-temperature skyrmionic states in a bulk chiral magnet
npj Quantum Materials (2019)
-
Magnon spectrum of the helimagnetic insulator Cu2OSeO3
Nature Communications (2016)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.