Abstract
Graphene charge carriers behave as massless Dirac fermions, opening the exciting possibility to observe long-range virtual tunneling of electrons in a solid. In granular metals, electron hops arising from series of virtual transitions are predicted to yield observable currents at low-enough temperatures, but to date experimental evidence is lacking. We report on electron transport in granular graphene films self-assembled by hydrogenation of suspended graphene. While the log-conductance shows a characteristic T−1/2 temperature dependence, cooling the samples below 10 K drives a triple crossover: a slope break in log-conductance, simultaneous to a substantial increase in magneto-conductance and onset of large mesoscopic conductance fluctuations. These phenomena are signatures of virtual transitions of electrons between distant localized states and conductance statistics reveal that the high crossover-temperature is due to the Dirac nature of granular graphene charge carriers.
Similar content being viewed by others
Introduction
Short-lived particles allowed by Heisenberg's uncertainty principle are called “virtual”, but the effects they induce are very real. They lead to vacuum fluctuations and mediate fundamental forces1, explain the Lamb shift of atomic levels2, the Casimir effect3 and possible Hawking radiations4. Though unobservable, virtual particles are key ingredients of modern quantum electrodynamics. Considerable research efforts have thus been devoted to measuring the most direct consequences of their existence, such as virtual-to-real photon conversion during dynamic Casimir effect5. Quantum dot nanostructures are excellent test beds too, as electron tunneling through virtual states6,7,8 generates background currents observable below ~100 mK9. Interestingly, higher-order currents between distant localized states are predicted to arise from multiple transitions to virtual states in macroscopic granular metals10,11,12, but this phenomenon coined multiple elastic co-tunneling (MEC) has not been observed yet. From this perspective, graphene is a particularly promising material, in which virtual excitations of charges play a special role. They are theoretically predicted to induce Dirac fermions' jittery motion called zitterbewegung13 and give a minimum conductivity to pristine graphene. In granular form, graphene dots' linear density of states (DOS)14 means that high-energy virtual states should contribute significantly to elastic co-tunneling currents despite shorter life-times, unlike ordinary granular metals (GM). This not only makes granular graphene the ideal platform for the first observation of MEC, but also provides a rare opportunity to measure long-range effects mediated by high-energy virtual states.
Results
Recently, chemical functionalization of graphene15 was reported to be a viable method to produce granular graphene16, due to the tendency of adatoms to form electrically insulating clusters. At high enough adatoms concentrations, such clusters merge into percolative pathways, effectively partitioning the graphene sheet into weakly-coupled graphene dot arrays, or GM. To conveniently fabricate graphene GMs, we exposed suspended CVD graphene sheets17 to hydrogen plasma18,19, thereby allowing adsorption of sufficiently high concentrations of hydrogen atoms on both sides of the graphene scaffold. We then stamped as-produced doubly-hydrogenated graphene (DHG) films on 90 nm-SiO2 chips. Au/Cr contacts in two- and four-probe geometries were then fabricated. Typical devices are shown in inset of figure 1(a). To observe the charge-neutrality point (CNP) at gate voltages Vg ~ 0 V, the samples were then vacuum-dried20 in-situ at 10−6 Torr for a day before cooling below 0°C. We then measured electron transport in 8 devices from room temperature down to 2.4 K. Figure 1(a) shows the room-temperature resistance R in units of resistance quantum h/e2, against charge-carrier density n for a typical sample (D0). The R(n) curve has a characteristic graphene-like shape, but is broad and R(n) ≫ 1 throughout the whole range of measured densities, indicating strong localization.
(a) Sheet resistance against charge density n at room temperature (Device D0). Inset shows an optical picture of typical devices. Scale bar: 10 μm. (b) Constant-conductance Vsd2 vs T2 domains extracted from conductance G against Vsd curves at 2.3 K and all temperatures between 3 K and 20 K in steps of 1 K (inset). The solid lines of the main figure are linear fits of slope ~ 1.4 × 10−4 V2K−2 to G = 1 nS (circles), G = 2 nS (triangles), G = 4 nS (squares), G = 10 nS (diamonds) and G = 20 nS (stars) domains. These domains correspond to traces represented as green dashed lines in inset. (c) Raman spectrum (device D1). The data (black) are fitted by a five-peak line-shape (red), sum of Breit-Wigner-Fano peaks: violet (D), blue (G), cyan (D′), green (2D) and orange (D + G). The inset is a zoom on the G and D′ peaks.
Next, we measure the conductance G for different bias voltages Vsd and temperatures T to extract the typical localization length ξ of our samples. The inset of figure 1(b) shows G against Vsd at different temperatures T between 2.3 K and 20 K for device D1. For consistency, all subsequent data shown in main text correspond to the same device D1. Data taken for other devices are reported in Supplementary Material, section A. We observe that above Vsd ~ 0.1 V, G increases with Vsd while below, G is bias-independent, indicating an Ohmic behavior. Crucially, G(Vsd, T) data can be used to extract ξ without assuming any particular transport mechanism. Since an electron hopping against the source-drain electric field E = Vsd/L over a distance d increases its energy by an amount eEd, it was shown that charge carriers experience an effective temperature  where L = 4 μm is the channel length and α ≈ 0.67 is a constant21. Importantly, Teff uniquely determines G, which implies that constant-conductance domains of (Vsd2, T2) - space are straight lines of slope −(αeξ/kBL)2. Figure 1(b) shows such domains extracted from G(Vsd,T) data at 1, 2, 4, 10 and 20 nS. As expected, they are well-fitted by straight lines of slope ~ −1.4 × 10−4 V2K−2, giving ξ ≈ 45 nm.
where L = 4 μm is the channel length and α ≈ 0.67 is a constant21. Importantly, Teff uniquely determines G, which implies that constant-conductance domains of (Vsd2, T2) - space are straight lines of slope −(αeξ/kBL)2. Figure 1(b) shows such domains extracted from G(Vsd,T) data at 1, 2, 4, 10 and 20 nS. As expected, they are well-fitted by straight lines of slope ~ −1.4 × 10−4 V2K−2, giving ξ ≈ 45 nm.
We now show that our DHG samples have a GM structure by complementing our electric transport measurements with Raman spectroscopy data, presented in figure 1(c). The spectrum shown in figure 1(c) exhibits a prominent D peak as well as broad 2D and D + D′ peaks. The D peak partially overlaps with the G peak. The D′ peak at 1614 cm−1 almost completely merges with the G peak at ~ 1588 cm−1 but can still be resolved as shown by the inset of figure 1(c). These are characteristics of strongly sp3-hybridized graphene22. The D-peak of sp3-hybridized graphene probes the distribution of sp3-bonds on the graphene lattice23. More precisely, the ratio ID/IG of integrated intensities of the D and G peaks is related to the typical adatom cluster size rs and the mean distance LD between nearest cluster centers by the following formula24,25:

where CA and CS are constants, rA = rS + δ and δ is the average distance laser-excited electrons travel before recombining with holes. Experimentally, it was shown that CA ~ 4, CS ~ 0.9 and δ ~ 2 nm for a laser of 2.4 eV26,27. By fitting Fano line-shapes28 to the Raman spectrum peaks, we calculate the peaks integrated intensities and ID/IG ~ 1.8. This value is clearly inconsistent with a random distribution of isolated adatoms, which would yield LD ~ δ ≪ ξ. Conversely, hydrogen adatoms form clusters of typical radius rs29. Simple geometric considerations discussed in section B of Supplementary Material impose that LD, rs and ξ typically follow the relation  . Since ξ ≈ 45 nm, we find rs ~ 30 nm by solving F(rs, LD) ≈ 1.8, leading to LD ≈ 2 rs. In other words, clusters tend to merge, isolating graphene dots of size ξ and our DHG samples have a GM structure. This agrees with previous studies on graphene quantum dots of size ~ ξ30 yielding comparable Raman spectra due to edge scattering26,31.
. Since ξ ≈ 45 nm, we find rs ~ 30 nm by solving F(rs, LD) ≈ 1.8, leading to LD ≈ 2 rs. In other words, clusters tend to merge, isolating graphene dots of size ξ and our DHG samples have a GM structure. This agrees with previous studies on graphene quantum dots of size ~ ξ30 yielding comparable Raman spectra due to edge scattering26,31.
Next, we focus on identifying the dominant charge transport mechanisms by analyzing the temperature-dependence of the conductance G(T). More precisely, one expects G to follow a G = G0exp(−(T0/T)γ) law characteristic of hopping transport, where γ and T0 depend on the exact hopping mechanism32. We thus measured G around CNP for different T between 2.4 K and 300 K. G(T) is systematically measured in the low-bias Ohmic regime, where both electric-field-driven electron hopping and Joule heating are negligible. As shown in section C of Supplementary Material, we observed a reduced activation energy33 β = dlnG/dlnT linear in lnG with slope ~−1/2 both for lnG < −21.5 and lnG > −19. Therefore, we plotted G against T−1/2 in figure 2(a). Strikingly,  between 300 K and ~12 K and between ~8 K and 2.4 K, but with a much smaller slope. A distinct slope break is thus identified around Tcross = 10 K. Graphene being atomically thin, this phenomenon certainly does not reflect a decrease in effective sample dimensionality from three to two dimensions34. Besides, our measured samples have a channel width W ≈ 6–7 μm systematically larger than the length L ≤ 5 μm to avoid any possible 2D to 1D crossover upon lowering the temperature. A G0 exp(−(T0/T)γ) fit both above and below Tcross respectively gives γ = 0.56 ± 0.04 and γ = 0.495 ± 0.05. In these regimes, G(T) is thus neither of the Arrhenius type (γ = 1) nor of the Mott's 2D variable-range hopping (VRH) type (γ = 1/3). However, γ = 1/2 suggests an Efros-Shklovskii (ES) VRH behavior, the 1D Mott VRH being excluded due to the geometry of our devices. Such behavior contrasts with the result of several earlier studies, in particular18, performed on strongly localized hydrogenated graphene samples, where the conduction is attributed to 2D Mott VRH. However, these results were obtained for less hydrogenated graphene samples, fabricated by exposing a single graphene face to hydrogen plasma. Conversely, a γ = 1/2 behavior was already reported in heavily oxidized graphene films16 with a GM structure. However, the presence of both a gamma-1/2 behavior and a slope break as observed in figure 2(a) around 10 K has never been observed before, to the best of our knowledge. While a crossover between two γ = 1/2 regimes with different slopes is not expected in standard VRH theories32, it is predicted for granular systems10, each regime reflecting a distinct transport mechanism illustrated in figure 2(b): multiple inelastic co-tunneling (MIC) at high temperatures and multiple elastic co-tunneling (MEC) at low temperatures. During MIC, multiple electrons simultaneously tunnel from the Fermi sea of a grain to an excited state of a neighboring grain, along a string of grains ultimately left in an excited state, thus requiring a finite temperature or electric field. During MEC, charge carriers tunnel between distant grains by transiting to intermediate virtual states, without absorbing or emitting phonons. To verify that our G(T) data result from a MIC-to-MEC crossover, we fit a function of the form G(T) = GMIC(T) + GMEC(T), where
between 300 K and ~12 K and between ~8 K and 2.4 K, but with a much smaller slope. A distinct slope break is thus identified around Tcross = 10 K. Graphene being atomically thin, this phenomenon certainly does not reflect a decrease in effective sample dimensionality from three to two dimensions34. Besides, our measured samples have a channel width W ≈ 6–7 μm systematically larger than the length L ≤ 5 μm to avoid any possible 2D to 1D crossover upon lowering the temperature. A G0 exp(−(T0/T)γ) fit both above and below Tcross respectively gives γ = 0.56 ± 0.04 and γ = 0.495 ± 0.05. In these regimes, G(T) is thus neither of the Arrhenius type (γ = 1) nor of the Mott's 2D variable-range hopping (VRH) type (γ = 1/3). However, γ = 1/2 suggests an Efros-Shklovskii (ES) VRH behavior, the 1D Mott VRH being excluded due to the geometry of our devices. Such behavior contrasts with the result of several earlier studies, in particular18, performed on strongly localized hydrogenated graphene samples, where the conduction is attributed to 2D Mott VRH. However, these results were obtained for less hydrogenated graphene samples, fabricated by exposing a single graphene face to hydrogen plasma. Conversely, a γ = 1/2 behavior was already reported in heavily oxidized graphene films16 with a GM structure. However, the presence of both a gamma-1/2 behavior and a slope break as observed in figure 2(a) around 10 K has never been observed before, to the best of our knowledge. While a crossover between two γ = 1/2 regimes with different slopes is not expected in standard VRH theories32, it is predicted for granular systems10, each regime reflecting a distinct transport mechanism illustrated in figure 2(b): multiple inelastic co-tunneling (MIC) at high temperatures and multiple elastic co-tunneling (MEC) at low temperatures. During MIC, multiple electrons simultaneously tunnel from the Fermi sea of a grain to an excited state of a neighboring grain, along a string of grains ultimately left in an excited state, thus requiring a finite temperature or electric field. During MEC, charge carriers tunnel between distant grains by transiting to intermediate virtual states, without absorbing or emitting phonons. To verify that our G(T) data result from a MIC-to-MEC crossover, we fit a function of the form G(T) = GMIC(T) + GMEC(T), where  and
and  are MIC and MEC conductance terms. The best fit is obtained for Gin ≈ 6 μS, Tin ≈ 700 K, Gel ≈ 1.5 nS and Tel ≈ 6 K. We now show that these values match theoretical expectations for co-tunneling in granular graphene. We start with Gin, which is the total conductance in the T ≫ Tin limit and is related to the nearest-grain tunneling conductance g by g ~ LGin/W, where W ≈ 7 μm is the sample width. This leads to g ~ 0.1 ≪ 1 in units of e2/h, which is a characteristic of insulating GM. The inter-dot tunneling conductance g should also be compared to the intra-dot conductance gdot35. Since before hydrogenation, our graphene samples have a typical mobility μ ≈ 5000 cm2/V.s, the intra-dot mean-free path is
are MIC and MEC conductance terms. The best fit is obtained for Gin ≈ 6 μS, Tin ≈ 700 K, Gel ≈ 1.5 nS and Tel ≈ 6 K. We now show that these values match theoretical expectations for co-tunneling in granular graphene. We start with Gin, which is the total conductance in the T ≫ Tin limit and is related to the nearest-grain tunneling conductance g by g ~ LGin/W, where W ≈ 7 μm is the sample width. This leads to g ~ 0.1 ≪ 1 in units of e2/h, which is a characteristic of insulating GM. The inter-dot tunneling conductance g should also be compared to the intra-dot conductance gdot35. Since before hydrogenation, our graphene samples have a typical mobility μ ≈ 5000 cm2/V.s, the intra-dot mean-free path is  where
where  . A graphene grain of size ~ξ with at least one Dirac fermion, corresponding to an areal density of ~5 × 1010/cm2, thus has l > 13 nm and a Thouless energy36
. A graphene grain of size ~ξ with at least one Dirac fermion, corresponding to an areal density of ~5 × 1010/cm2, thus has l > 13 nm and a Thouless energy36  larger than ~10 meV. Therefore, ETh typically exceeds the dots mean energy level spacing37
larger than ~10 meV. Therefore, ETh typically exceeds the dots mean energy level spacing37  , so that
, so that  is at least a few e2/h. gdot ≫ g is thus satisfied throughout the experimentally relevant range of densities. This is again perfectly consistent with an insulating granular metal behavior and contrasts sharply with the case of homogeneously disordered systems10, where
is at least a few e2/h. gdot ≫ g is thus satisfied throughout the experimentally relevant range of densities. This is again perfectly consistent with an insulating granular metal behavior and contrasts sharply with the case of homogeneously disordered systems10, where  . Next, we notice that Tin ≈ 700 K is significantly larger than the charging energy of a grain
. Next, we notice that Tin ≈ 700 K is significantly larger than the charging energy of a grain  , where ε ≈ 3.5 is the dielectric constant of the inter-granular medium16. This agrees with MIC theory10 which predicts Tin = χinTc, where χin is a logarithmically T-dependent coefficient. As T ≪ Tc,
, where ε ≈ 3.5 is the dielectric constant of the inter-granular medium16. This agrees with MIC theory10 which predicts Tin = χinTc, where χin is a logarithmically T-dependent coefficient. As T ≪ Tc,  where
where  is the rate of inelastic tunneling between two neighboring grains12, giving χin ≈ 8 at T = 10–20 K and hence Tin, theory ≈ 800 K, close to the experimental value. We finally focus on Tel, whose value extracted from figure 2(a) is one order of magnitude smaller than Tc. This remains true at all Vg despite fluctuations and Tel averages to θel ≈ 10 K. More details on the statistics of Tel are reported in Supplementary Material, section D. Theoretically,
is the rate of inelastic tunneling between two neighboring grains12, giving χin ≈ 8 at T = 10–20 K and hence Tin, theory ≈ 800 K, close to the experimental value. We finally focus on Tel, whose value extracted from figure 2(a) is one order of magnitude smaller than Tc. This remains true at all Vg despite fluctuations and Tel averages to θel ≈ 10 K. More details on the statistics of Tel are reported in Supplementary Material, section D. Theoretically,  , where p is the probability of virtual transition to a neighboring grain10,11. For conventional two-dimensional GMs, the DOS in each grain is constant and the level spacing is small compared to the charging energy, leading to10
, where p is the probability of virtual transition to a neighboring grain10,11. For conventional two-dimensional GMs, the DOS in each grain is constant and the level spacing is small compared to the charging energy, leading to10  and Tel of the order of few Tc. Therefore, Schrödinger fermions cannot account for the observed Tel ≪ Tc behavior. Unlike MIC for which only states within kBTeff around the Fermi level contribute to G, virtual transitions to high-energy states contribute to the MEC conductance and the band structure plays a key role. In the Dirac fermions case, the short life-time
and Tel of the order of few Tc. Therefore, Schrödinger fermions cannot account for the observed Tel ≪ Tc behavior. Unlike MIC for which only states within kBTeff around the Fermi level contribute to G, virtual transitions to high-energy states contribute to the MEC conductance and the band structure plays a key role. In the Dirac fermions case, the short life-time  of high-energy fermions is compensated by a DOS which increases linearly with energy E, making the contribution of high-energy virtual states significant. It remains true for chaotic dots with edges of random shape14,37. Following refs. 11 and 38, we calculated in Supplementary Material (section E) a lower-bound for the virtual transition probability of graphene's Dirac Fermions:
of high-energy fermions is compensated by a DOS which increases linearly with energy E, making the contribution of high-energy virtual states significant. It remains true for chaotic dots with edges of random shape14,37. Following refs. 11 and 38, we calculated in Supplementary Material (section E) a lower-bound for the virtual transition probability of graphene's Dirac Fermions:  , where Γ is the energy bandwidth. This is in accord with experimental statistics on pel shown in inset of figure 2(a) and implies that pin ~ pel just above Tcross, between 20 K and 40 K, thus providing strong evidence for MIC-to-MEC crossover.
, where Γ is the energy bandwidth. This is in accord with experimental statistics on pel shown in inset of figure 2(a) and implies that pin ~ pel just above Tcross, between 20 K and 40 K, thus providing strong evidence for MIC-to-MEC crossover.
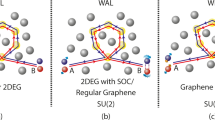
(a) Low-bias G vs T−1/2 at Vg = 1.5 V. The violet line is a fit accounting for inelastic and elastic co-tunneling mechanisms as described in main text. The blue (resp. red) dashed line corresponds to the best power law fit for lnG vs T−1/2 below 6 K (resp. above 10 K). Inset shows a histogram for pel extracted from slopes of lnG(T) vs T−1/2 at different Vg, shown in Supplementary Material, section D. The red solid line is a guide to the eyes and the dashed green line is the lower-bound for pel calculated in Supplementary Material, section E. (b) Cartoon representation of hydrogenated graphene sheets (bottom), MEC (centre) and MIC (top). Hydrogen clusters partition hydrogenated graphene into disconnected metallic graphene dots. Hydrogen concentration is encoded by shades of green, from white (hydrogen-poor) to green (hydrogen-rich). Hopping from initial to final localized state (grey) occurs by two possible mechanisms described in main text: MIC (top) and MEC (centre). Cones represent graphene's energy spectrum within individual dots circled by dashed lines in bottom panel. Discrete energy levels due to confinement are marked by red and blue circles. Red (blue) balls represent electrons (holes). Arrows correspond to transitions due to absorption of phonons (top) or virtual transitions (bottom).
A powerful way to gain further insight is to analyze the variations of G with Vg and Vsd, as each transport mechanism leaves its own mesoscopic fluctuations footprints38,39,40,41. Figure 3(a) shows G measured at 3 K for −7.5 V ≤ Vg ≤ 7.5 V and 0 ≤ Vsd ≤ 200 mV and exhibits vertical stripes of width ΔVg ~ 200 mV, corresponding to peaks and valleys in conductance reproduced at all measured Vsd. This is highlighted by figure 3(b), which shows four different traces extracted from figure 3(a) at Vsd = 10 mV, 80 mV, 120 mV and 175 mV. While overall the conductance increases with Vsd, the different fixed-Vsd G(Vg) curves show reproducible peaks and valleys. To analyze these fluctuations quantitatively, we systematically extracted the standard deviation of log-conductance σlnG at fixed source-drain bias from figure 3(a) and three other data sets shown in Supplementary Material, section F. Figure 4 shows σlnG plotted against Teff from 3 K up to 80 K, in double-log scale. Two different regimes can be clearly distinguished. Below Teff = 10 K, σlnG is weakly temperature-dependent while above 10 K, σlnG decreases rapidly with Teff. Quite remarkably, these two regimes coincide with the two distinct G(T) regimes observed in figure 2(a). We start by analyzing the high-Teff regime, where σlnG is very well described by the power-law  . This behavior is clearly incompatible with Mott or ES VRH as such phenomena would lead to
. This behavior is clearly incompatible with Mott or ES VRH as such phenomena would lead to  with a < 141. We now compare the observed fluctuations above 10 K to expected MIC-induced fluctuations. From a hopping percolation viewpoint42, the fluctuations in log-conductance σLnG are related to the standard deviation s of log-conductance at the scale of a hopping distance rhop, by
with a < 141. We now compare the observed fluctuations above 10 K to expected MIC-induced fluctuations. From a hopping percolation viewpoint42, the fluctuations in log-conductance σLnG are related to the standard deviation s of log-conductance at the scale of a hopping distance rhop, by  where
where  ,
,  and ν ≈ 4/3 is the critical exponent in two dimensions. Since MIC is a fundamentally phase-incoherent process involving a different charge carrier for each intermediate transition (see figure 2(b)), MIC-induced fluctuations in conductance do not originate from quantum interferences between electron wave functions. It must rather arise from fluctuations in inter-dot conductances accompanying changes in percolation network as gate voltage is tuned. From this perspective, we find - based on probabilistic arguments developed in Supplementary Material, section G1 and G3 - that
and ν ≈ 4/3 is the critical exponent in two dimensions. Since MIC is a fundamentally phase-incoherent process involving a different charge carrier for each intermediate transition (see figure 2(b)), MIC-induced fluctuations in conductance do not originate from quantum interferences between electron wave functions. It must rather arise from fluctuations in inter-dot conductances accompanying changes in percolation network as gate voltage is tuned. From this perspective, we find - based on probabilistic arguments developed in Supplementary Material, section G1 and G3 - that  where10
where10  and Nth is the number of energy levels accessible in a neighboring grain by emitting or absorbing a phonon. For a 2D GM with parabolic-band grains,
and Nth is the number of energy levels accessible in a neighboring grain by emitting or absorbing a phonon. For a 2D GM with parabolic-band grains,  and hence
and hence  . This corresponds to
. This corresponds to  , which does not satisfactorily fit the data. However, in graphene grains, energy levels are not evenly spaced. The nth energy level from neutrality point has an expected value of energy
, which does not satisfactorily fit the data. However, in graphene grains, energy levels are not evenly spaced. The nth energy level from neutrality point has an expected value of energy  where
where  . Therefore,
. Therefore,  and
and  (see Supplementary Material, section G2). This yields
(see Supplementary Material, section G2). This yields  , which agrees very well with the data. In other words, the unusually strong Teff – dependence of σlnG above 10 K can only be understood in terms of inelastic co-tunneling of Dirac fermions.
, which agrees very well with the data. In other words, the unusually strong Teff – dependence of σlnG above 10 K can only be understood in terms of inelastic co-tunneling of Dirac fermions.
(a) Conductance as a function of Vg and Vsd at 3 K. The linear color scale corresponds to the measured conductance in nS. (b) Conductance against Vg traces at 3 K extracted from fig. 3(a): at Vsd = 10 mV (blue), Vsd = 80 mV (green), Vsd = 120 mV (orange) and Vsd = 175 mV (red). The vertical black dashed curves are guides to the eyes highlighting the reproducibility of the peaks in conductance across different voltage biases.
Standard deviation σlnG of the log-conductance as a function of Teff.
Data points were extracted from 4 distinct data sets - DS1, DS2, DS3, DS4, presented in Supplementary Material, section F - measured in sample D1. DS3 corresponds to fig. 3(a). Blue circles (DS1) were extracted from G vs Vsd curves taken at 3 K for different Vg. Green triangles (DS2) and orange diamonds (DS3) were extracted from G vs Vg, Vsd plots. Red squares (DS4) correspond to G vs Vg curves taken at 6 K and fixed source-drain current. Dashed and solid lines correspond to theoretically predicted power-laws for different transport mechanisms: Dirac fermions (DF) MIC (black), Schrödinger fermions (SF) MIC (violet), ES (green) and Mott VRH (pink), bottleneck-limited MEC (red). Inset shows the high-field MC at Vg = 0 V between 2 K and 40 K. Data points are shown in black. The solid red line is a guide to the eyes and the dashed green line indicates Tcross.
We now discuss the sub-Tcross regime, where σlnG ~ 0.5 is weakly T-dependent, clearly ruling out MIC as dominant transport mechanism below 10 K. This is perfectly consistent with figure 2(a) which indicates a crossover to MEC below 10 K. Since MEC is a phase-preserving process, it is tempting to assign this behavior to quantum interferences between distinct phase-coherent charge-carrier paths43,44, a phenomenon known to produce almost Teff-independent conductance fluctuations of large magnitude. This view is supported by magneto-transport experiments carried out at 2.4 K for −500 mV ≤ Vsd ≤ 500 mV. The inset of figure 4 shows the relative magneto-conductance MC = G(8T)/G(0T) against Teff from 2.4 K up to 40 K. MC is almost constant and close to 1 above Tcross where MIC dominates, reflecting its phase-incoherent nature, whereas MC rapidly increases to ~2 below Tcross, a manifestation of quantum interferences11,44,45 attributable to MEC. Moreover, figure 4 shows that the sub-Tcross data are well-described by  with η ~ 0.16 or less, suggesting conduction is limited by a strongly resistive portion of the granular graphene film of size ~ rhop, or “bottleneck”. Such a situation systematically occurs in granular media at sufficiently low Teff when σLnG approaches unity41,46, which is the case around Tcross. Since quantum interferences within a bottleneck must give the main contribution to σlnG, the observed
with η ~ 0.16 or less, suggesting conduction is limited by a strongly resistive portion of the granular graphene film of size ~ rhop, or “bottleneck”. Such a situation systematically occurs in granular media at sufficiently low Teff when σLnG approaches unity41,46, which is the case around Tcross. Since quantum interferences within a bottleneck must give the main contribution to σlnG, the observed  plausibly approximates the
plausibly approximates the  law for systems of size close to the phase-coherence length47,48,49. In summary, both the significant increase in magneto-conductance and weak temperature-dependence of σlnG below 10 K indicate the appearance of quantum interferences. The presence of such strong interferences only below Tcross is consistent with the existence of a crossover from phase-incoherent MIC above 10 K to MEC below 10 K.
law for systems of size close to the phase-coherence length47,48,49. In summary, both the significant increase in magneto-conductance and weak temperature-dependence of σlnG below 10 K indicate the appearance of quantum interferences. The presence of such strong interferences only below Tcross is consistent with the existence of a crossover from phase-incoherent MIC above 10 K to MEC below 10 K.
Discussion
Our results show that doubly-hydrogenated graphene is not amorphous but has a granular structure instead. This finding is consistent with the tendency of hydrogen atoms adsorbed on graphene to phase-separate50 and form electrically insulating clusters. This observation is particularly interesting as graphane phases – or fully hydrogenated graphene regions – are predicted to be high-temperature superconductors51. From this perspective, hydrogenation of suspended graphene sheets appears as a viable route towards the synthesis of novel granular superconductors10,52. The granularity of our doubly-hydrogenated graphene samples is reflected by the presence of Dirac fermions in this material, which can exist in graphene grains or dots, but not in amorphous media. Both the large crossover temperature from inelastic to elastic co-tunneling and the temperature dependence of the log-conductance fluctuations with gate voltage are signatures of the presence of Dirac fermions in our samples.
In conclusion, we observed multiple elastic co-tunneling for the first time in a granular metal. In our granular graphene samples, both multiple inelastic and elastic co-tunneling mechanisms showed signatures of Dirac fermions. The presence of large high-order elastic co-tunneling currents in granular graphene establishes granular Dirac materials as ideal platforms for the study of vacuum fluctuations and quantum noise53.
Methods
Device fabrication
CVD graphene was grown on copper foil using the technique described in ref. 17. The copper foil was etched away using an ammonium persulfate solution and transferred on a Quantifoil S7/2 square mesh holey carbon TEM grids, following a polymer-free transfer technique similar to ref. 54. Our freestanding graphene sheets were subsequently exposed to hydrogen plasma following the technique used in ref. 19. The resulting samples were deposited on 90 nm-SiO2 chips immediately after plasma exposure, by gently stamping the TEM-grid-supported graphene sheet onto the substrate. This procedure generally left a large number of 7 × 7 μm2 square-shaped hydrogenated graphene flakes on SiO2, corresponding to the TEM grid mesh geometry. Devices were then fabricated by standard electron-beam lithography technique followed by thermal evaporation of Au/Cr electrodes.
Electron transport measurement
After fabrication, samples were loaded in a variable temperature insert coupled to a 9 T superconducting magnet. A pressure of ~10−6 Torr was maintained during the experiments. Electron transport measurements were carried out with a Keithley 6517B Electrometer/High-Resistance Meter. To eliminate possible DC noise, we used the following procedure: for each bias Vsd, the source-drain current Isd was measured 10 times at +Vsd within ~1 s, then 10 times at –Vsd within ~1 s. The resulting noise-filtered current was then systematically calculated as  , where
, where  corresponds to the 10-point average. Vsd was sourced by Keithley 6517B or Keithley 6430. Keithley 6430 was systematically used to source the gate voltage. When needed, a fixed Isd was sourced with a Keithley 6221, while Vsd was measured with Keithley 6517 B.
corresponds to the 10-point average. Vsd was sourced by Keithley 6517B or Keithley 6430. Keithley 6430 was systematically used to source the gate voltage. When needed, a fixed Isd was sourced with a Keithley 6221, while Vsd was measured with Keithley 6517 B.
References
Peskin, M. E. & Schroeder, D. V. An Introduction to Quantum Field Theory. (Addison-Wesley Publishing Company, Reading, 1995).
Scully, M. O. & Zubairy, M. S. Quantum Optics. (Cambridge University Press, Cambridge, 1997).
Rodriguez, A. W., Capasso, F. & Johnson, S. G. The Casimir effect in microstructured geometries. Nature Photon. 5, 211–221 (2011).
Hawking, S. W. Black hole explosions? Nature 248, 30–31 (1974).
Wilson, C. M. et al. Observation of the dynamical Casimir effect in a superconducting circuit. Nature 479, 376–379 (2011).
Averin, D. & Nazarov, Y. Virtual electron diffusion during quantum tunneling of the electric charge. Phys. Rev. Lett. 65, 2446–2449 (1990).
Fujisawa, T. Spontaneous Emission Spectrum in Double Quantum Dot Devices. Science 282, 932–935 (1998).
De Franceschi, S. et al. Electron Cotunneling in a Semiconductor Quantum Dot. Phys. Rev. Lett. 86, 878–881 (2001).
Gustavsson, S. et al. Detecting single-electron tunneling involving virtual processes in real time. Phys. Rev. B 78, 155309 (2008).
Beloborodov, I., Lopatin, A., Vinokur, V. & Efetov, K. Granular electronic systems. Rev. Mod. Phys. 79, 469–518 (2007).
Feigel'man, M. V. & Ioselevich, A. S. Variable range cotunneling and conductivity of a granular metal. JETP Lett. 81, 341–347 (2005).
Beloborodov, I., Lopatin, A. & Vinokur, V. Coulomb effects and hopping transport in granular metals. Phys. Rev. B 72, 125121 (2005).
Katsnelson, M. I. Zitterbewegung, chirality and minimal conductivity in graphene. EPJ B 51, 157–160 (2006).
Berry, M. V. & Mondragon, R. J. Neutrino Billiards: Time-Reversal Symmetry-Breaking Without Magnetic Fields. Proc. R. Soc. A 412, 53–74 (1987).
Balakrishnan, J., Koon, G. K. W., Jaiswal, M., Castro Neto, A. H. & Özyilmaz, B. B. Colossal enhancement of spin-orbit coupling in weakly hydrogenated graphene. Nature Phys. 9, 284–287 (2013).
Joung, D., Zhai, L. & Khondaker, S. I. Coulomb blockade and hopping conduction in graphene quantum dots array. Phys. Rev. B 83, 115323 (2011).
Bae, S. et al. Roll-to-roll production of 30-inch graphene films for transparent electrodes. Nature Nanotech. 5, 574–578 (2010).
Elias, D. C. et al. Control of Graphene's Properties by Reversible Hydrogenation: Evidence for Graphane. Science 323, 610–613 (2009).
Jaiswal, M. et al. Controlled Hydrogenation of Graphene Sheets and Nanoribbons. ACS Nano 5, 888–896 (2011).
Matis, B. R. et al. Surface Doping and Band Gap Tunability in Hydrogenated Graphene. ACS Nano 6, 17–22 (2012).
Marianer, S. & Shklovskii, B. Effective temperature of hopping electrons in a strong electric field. Physical Review B 46, 13100–13103 (1992).
Nair, R. R. et al. Fluorographene: a two-dimensional counterpart of Teflon. Small 6, 2877–2884 (2010).
Ferrari, A. C. Raman spectroscopy of graphene and graphite: Disorder, electron–phonon coupling, doping and nonadiabatic effects. Solid State Commun. 143, 47–57 (2007).
Lucchese, M. M. et al. Quantifying ion-induced defects and Raman relaxation length in graphene. Carbon 48, 1592–1597 (2010).
Martins Ferreira, E. H. et al. Evolution of the Raman spectra from single-, few- and many-layer graphene with increasing disorder. Phys. Rev. B 82, 125429 (2010).
Ryu, S., Maultzsch, J., Han, M., Kim, P. & Brus, L. E. Raman spectroscopy of lithographically patterned graphene nanoribbons. ACS Nano 5, 4123–4130 (2011).
Eckmann, A. et al. Probing the nature of defects in graphene by Raman spectroscopy. Nano Lett. 12, 3925–3930 (2012).
Saito, R., Hofmann, M., Dresselhaus, G., Jorio, A. & Dresselhaus, M. S. Raman spectroscopy of graphene and carbon nanotubes. Adv. Phys. 60, 413–550 (2011).
Rappoport, T. G., Uchoa, B. & Castro Neto, A. H. Magnetism and magnetotransport in disordered graphene. Phys. Rev. B 80, 245408 (2009).
Kim, S. et al. Size-dependence of Raman scattering from graphene quantum dots: Interplay between shape and thickness. Appl. Phys. Lett. 102, 053108 (2013).
Casiraghi, C. et al. Raman Spectroscopy of Graphene Edges. Nano Lett. 9, 1433–1441 (2009).
Shklovskii, B. I. & Efros, A. L. Electronic Properties of Doped Semiconductors. (Springer-Verlag, Berlin, New York, 1984).
Liu, G. & Soonpaa, H. Electric-field and temperature effects in a two-dimensional system with strong localization. Phys. Rev. B 48, 5682–5684 (1993).
Tran, T. et al. Sequential tunneling and inelastic cotunneling in nanoparticle arrays. Phys. Rev. B 78, 075437 (2008).
Tran, T. et al. Multiple Cotunneling in Large Quantum Dot Arrays. Phys. Rev. Lett. 95, 076806 (2005).
Edwards, J. T. & Thouless, D. J. Numerical studies of localization in disordered systems. J. Phys. C: Solid State Phys. 5, 807–820 (1972).
Ponomarenko, L. A. et al. Chaotic Dirac billiard in graphene quantum dots. Science 320, 356–358 (2008).
Aleiner, I. L. & Glazman, L. I. Mesoscopic Fluctuations of Elastic Cotunneling. Phys. Rev. Lett. 77, 2057–2060 (1996).
Milliken, F. & Ovadyahu, Z. Observation of conductance fluctuations in large In2O3-x films. Phys. Rev. Lett. 65, 911–914 (1990).
Cronenwett, S. M., Patel, S. R., Marcus, C. M., Campman, K. & Gossard, A. C. Mesoscopic Fluctuations of Elastic Cotunneling in Coulomb Blockaded Quantum Dots. Phys. Rev. Lett. 79, 2312–2315 (1997).
Raikh, M. & Ruzin, I. Size effect in the longitudinal hopping conduction of a narrow two-dimensional channel. Phys. Rev. B 42, 11203–11207 (1990).
Strelniker, Y., Havlin, S., Berkovits, R. & Frydman, A. Resistance distribution in the hopping percolation model. Phys. Rev. E 72, 016121 (2005).
Beloborodov, I., Lopatin, A. & Vinokur, V. Universal description of granular metals at low temperatures: Granular Fermi liquid. Phys. Rev. B 70, 205120 (2004).
Nguyen, V. L., Spivak, B. Z. & Shklovskii, B. I. Hopping-conductivity fluctuations in small samples. JETP Lett. 43, 44–47 (1986).
Zhao, H. L., Spivak, B. Z., Gelfand, M. P. & Feng, S. Negative magnetoresistance in variable-range-hopping conduction. Phys. Rev. B 44, 10760–10767 (1991).
Strelniker, Y., Berkovits, R., Frydman, A. & Havlin, S. Percolation transition in a two-dimensional system of Ni granular ferromagnets. Phys. Rev. E 69, 065105 (2004).
Somoza, A., Ortuño, M. & Prior, J. Universal Distribution Functions in Two-Dimensional Localized Systems. Phys. Rev. Lett. 99, 116602 (2007).
Prior, J., Somoza, A. & Ortuño, M. Conductance fluctuations and single-parameter scaling in two-dimensional disordered systems. Phys. Rev. B 72, 024206 (2005).
Choe, D. H. & Chang, K. J. Effect of dimensionality on the localization behavior in hydrogenated graphene systems. Nano Lett. 12, 5175–5180 (2012).
Rakhmanov, A. L., Rozhkov, A. V., Sboychakov, A. O. & Nori, F. Phase separation of hydrogen atoms adsorbed on graphene and the smoothness of the graphene-graphane interface. Phys. Rev. B 85, 035408 (2012).
Savini, G., Ferrari, A. C. & Giustino, F. First-Principles Prediction of Doped Graphane as a High-Temperature Electron-Phonon Superconductor. Phys. Rev. Lett. 105, 037002 (2010).
Allain, A., Han, Z. & Bouchiat, V. Electrical control of the superconducting-to-insulating transition in graphene-metal hybrids. Nature Mater. 11, 590–594 (2012).
Clerk, A. A., Devoret, M. H., Girvin, S. M., Marquardt, F. & Schoelkopf, R. J. Introduction to quantum noise, measurement and amplification. Rev. Mod. Phys. 82, 1155–1208 (2010).
Regan, W. et al. A direct transfer of layer-area graphene. Appl. Phys. Lett. 96, 113102 (2010).
Acknowledgements
We thank A.H. Castro Neto, V. Pereira, M.A. Cazalilla and J. Martin for helpful discussions. This work was supported by the Singapore Millennium Foundation-NUS Research Horizons award (R-144-001-271-592; R-144-001-271-646) and NUS-YIA (R144-000-283-101).
Author information
Authors and Affiliations
Contributions
A.P. and M.J. designed and performed the experiments. A.P. and M.J. analyzed the data. A.P. wrote the paper. B.-H.H. and J.-H.A. prepared graphene by chemical vapor deposition. Y.W. and K.P.L. hydrogenated graphene. All authors discussed the data and reviewed the manuscript. B.Ö. devised and supervised the project.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Supplementary Information
Ozyilmaz - Supplementary Material - Multiple Virtual Tunneling of Dirac fermions in Granular Graphene
Rights and permissions
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. To view a copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/
About this article
Cite this article
Pachoud, A., Jaiswal, M., Wang, Y. et al. Multiple Virtual Tunneling of Dirac Fermions in Granular Graphene. Sci Rep 3, 3404 (2013). https://doi.org/10.1038/srep03404
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep03404
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.