Abstract
The control of complex oxide heterostructures at atomic level generates a rich spectrum of exotic properties and unexpected states at the interface between two separately prepared materials. The frustration of magnetization and conductivity of manganite perovskite at surface/interface which is inimical to their device applications, could also flourish in tailored functionalities in return. Here we prove that the exchange bias (EB) effect can unexpectedly emerge in a (La,Sr)MnO3 (LSMO) “single” film when large compressive stress imposed through a lattice mismatched substrate. The intrinsic EB behavior is directly demonstrated to be originating from the exchange coupling between ferromagnetic LSMO and an unprecedented LaSrMnO4-based spin glass, formed under a large interfacial strain and subsequent self-assembly. The present results not only provide a strategy for producing a new class of delicately functional interface by strain engineering, but also shed promising light on fabricating the EB part of spintronic devices in a single step.
Similar content being viewed by others
Introduction
Metal and semiconductor heterostructures have led to prominent advances in consumer electronics over the last decades1,2,3. Efforts are increasingly shifting to artificially constructed heterointerfaces between different complex oxides, accompanied by the observation of numerous fascinating phenomena, high-mobility conductivity4,5, exchange bias6,7 and ferroelectricity enhancement8,9, etc. Among these oxide systems, the technological importance of magnetic tunnel junctions10,11 and spin injectors12 as potential applications of magnetically active mixed valence manganites, have continuously triggered extensive research.
The coupling of several degrees of freedom (such as spin, charge, lattice and orbital) enriches the physical properties of mixed valence perovskite manganite13. Moreover, electrostatic and strain-related effects promote the electronic and structural reconstructions at interfaces among dissimilar oxides. Manganite films display very different properties, strongly affected by substrates and film thicknesses14,15,16. The existence of an interfacial layer (often referred to as the dead layer), which exhibits an insulating and non-ferromagnetic behavior, has been suggested to be responsible for the competitions between variable phases. The origin of this interfacial layer has been vigorously pursued and several mechanisms are proposed: electronic and/or chemical phase separation16,17,18, eg orbital reconstruction and delocalization19,20 and a Mn3+ enrichment region21, whereas a conclusive mechanism remains under debate. A long-term challenge for this rather contradictory situation is that the subtle variation at the interface makes its fingerprint hard to be directly detected. On the other hand, this interfacial layer is thought to be inimical to the device application11,22. Large amount of works are pursuing the reduction of dead layer through various ways like reducing the substrate/film lattice mismatch and changing interfacial chemical stoichiometry23,24. However, these attempts are so far incapable to completely eliminate the unfavorable structures18. Hence it is meaningful to go to the opposite direction, exploring a way to enlarge the interfacial reconstruction and benefit from it with tailored functionalities in return.
Specifically, the orbital reorganization results in an antiferromagnetic (AFM) layer in contrast to its ferromagnetic (FM) counterpart according to x-ray magnetic circular/linear dichroism (XMCD/XLD) experiments16,19,21. In-plane compressive or tensile epitaxial strain favours the 3z2-r2 (C-type AFM) or the x2-y2 (A-type AFM) orbital ordering, respectively16,19. The EB effect, discovered 60 years ago25 and now widely used in spintronic devices2, is commonly observed in FM/AFM bilayers and its nature is still exploited6,7,26. It is then quite natural to expect an intrinsic exchange bias (EB) effect at the self-assembled FM/AFM interface, which is anticipated to enhance the spin polarization at FM/AFM interface and fabricate EB part of spintronic devices in a single step22. Although the AFM layer in manganite is depending on the MnO6 octahedral distortion which could be tuned by the strain engineering27, the intrinsic EB effect observed in AFM-based manganites is still a question of interest lacking experimental insight and in-depth investigation28,29,30,31.
In this context, to enlarge the compressive strain, LaSrAlO4 (001) (LSAO; a = 3.756 Å) substrates with a comparatively small lattice were intentionally employed to grow 45 unit cell (u.c.) La2/3Sr1/3 MnO3 (LSMO; a = 3.870 Å for the bulk) films. The interfacial reconstruction of structure and composition is directly observed, resulting in two magnetic sublattices and intrinsic exchange bias in manganite. The structure evolution and property variation of different thickness LSMO also provides the growth dynamics for LSMO grown under large compressive strain.
Results
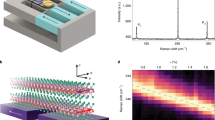
A typical atomic resolution aberration-corrected high-angle annular dark field scanning transmission electron microscope (HAADF-STEM) image with its analysis is presented in Fig. 1. Remarkably, instead of a uniform film, the LSMO is divided into three sublattices in the order of increasing the distance from the film/substrate interface: (1) LaAlO3-like (LAO-like), (2) LaSrMnO4 and (3) LSMO. The boundaries and coherence (highlighted by colored spheres) of these three phases are clearly recognizable in the HAADF-STEM image. A magnified view of their schematic counterparts is illustrated in Fig. 1a. According to the electron energy-loss spectroscopy (EELS) (d = 0.8 and 2.0 nm) and energy dispersive x-ray spectroscopy (EDX) with a spatial accuracy of one lattice site, it is surprisingly found that the film/substrate interface is a La and Al (diffused from the substrate during the 700°C growth) enrichment region (Supplementary Fig. S1a), in contrast to the lacking of Mn and depletion of Sr ascribed to the large compressive strain [the size of Sr (Mn) is much larger than that of La (Al)], exhibiting a chemical concentration far away from LSMO. A combination of concentration characterization and lattice feature with the thickness of 6 u.c., reveals the formation of LaAlO3-like (LAO) layer just above the interface. It directly bears the strain from the substrate serving as a buffer layer.
Direct observation of self-assembled structures in LSMO films.
(a), Sketch of LAO-like, LaSrMnO4 and LSMO phases and their interfaces. (b), Typical HAADF-STEM image of the interfacial area. Interfaces between (1) LAO-like, (2) LaSrMnO4 and (3) LSMO are marked by white dashed lines. The cations are highlighted by colored circles. (c), EELS Mn L2,3 absorption edge for the region shown in the yellow frame of (b). The distance (d) in EELS is the space of the probing place and substrate surface and the data are the average results of ±1 u.c. range. Elemental mappings by EDX measurement for the area of HAADF-STEM image (d) are shown in (e), La (red), (f), Sr (green), (g), Mn (blue) and (h), Al (cyan). The white scale bars in (d–h) represent a length of 5 nm.
The penetration of Sr and shortage of La (Fig. 1e,f ) produce a K2NiF4 lattice in area (2) on the top of the LAO-like buffer. Taken together, concentration of this area and the LAO-like layer keeps a chemical equilibrium of LSMO. A closer inspection of the EELS results reveals that the Mn-L2,3 spectrum in this area (d = 3.2 nm) shifts to the low-energy comparing with those of the upper region, indicating that the valence of Mn is mainly in +3 (Ref. 32). The coexistence of K2NiF4 lattice in conjunction with enriched Sr and Mn3+ demonstrate that a cell of AFM LaSrMnO4 dominates in this area, followed by the growth of normal LSMO in area (3). This observation is corroborated by the inspection of O-EELS (Supplementary Fig. S1b) and surface sensitive XLD spectroscopy (Supplementary Fig. S2). In LaSrMnO4, Mn ions at the corner of crystal cell share the B-site of LAO and LSMO, while La and Sr ions locate at its A-site, with c-axis of 13.09 Å. It is also reasonable to form self-assembled three phases in LSMO from the viewpoint of large lattice misfit between LSMO/LSAO, producing a lattice gradient of  along the growth direction and the relaxation of large strain. Conversely, LSMO grown on SrTiO3 substrate (the most used substrate for LSMO growth, a = 3.904 Å) with a much smaller mismatch and a different sign of strain, exhibits a homogeneous film without obvious phase separation (Supplementary Fig. S3). A lattice distortion but without apparent self-assembly is observed in the LSMO film grown on LaAlO3 (a = 3.789 Å), which coincides with its moderate strain between the case on LaSrAlO4 and SrTiO3 (Supplementary Fig. S4), suggesting that the film/substrate interface could be tuned by strain. It is noteworthy that the LAO-like and LaSrMnO4 phases constitute the dead layer of present LSMO film. In particular, magnetic frustration in the present interface is associated with a reconstruction of microstructure and composition, which is essentially different from the dead layer proposed previously17,18,19,20, thus providing a new clue as to how to grow fascinating novel structures with tailored functionalities by the intentional interface design.
along the growth direction and the relaxation of large strain. Conversely, LSMO grown on SrTiO3 substrate (the most used substrate for LSMO growth, a = 3.904 Å) with a much smaller mismatch and a different sign of strain, exhibits a homogeneous film without obvious phase separation (Supplementary Fig. S3). A lattice distortion but without apparent self-assembly is observed in the LSMO film grown on LaAlO3 (a = 3.789 Å), which coincides with its moderate strain between the case on LaSrAlO4 and SrTiO3 (Supplementary Fig. S4), suggesting that the film/substrate interface could be tuned by strain. It is noteworthy that the LAO-like and LaSrMnO4 phases constitute the dead layer of present LSMO film. In particular, magnetic frustration in the present interface is associated with a reconstruction of microstructure and composition, which is essentially different from the dead layer proposed previously17,18,19,20, thus providing a new clue as to how to grow fascinating novel structures with tailored functionalities by the intentional interface design.
Such unprecedentedly self-assembled structures have a profound influence on magnetic properties. Corresponding magnetic loops measured at 5 K are shown in Fig. 2a. One of the loops (solid sphere) was measured after field-cooling from room temperature in a +20 kOe field to 5 K. The most eminent feature observed here is the shift of the loop along the magnetic field axis towards negative fields (Fig. 2a). In contrast, on cooling in the presence of a −20 kOe field, the loop is biased in the positive direction. This observation confirms the presence of intrinsic EB in a nominally “single” LSMO film at low temperatures with a bias field HEB of 246 Oe, defined as the absolute offset of the loops along the field axis. These measurements are arbitrary from several subsequent runs with different growth that all confirm the reproducible EB behavior. The absence of biasing effect in LSMO grown on (001) SrTiO3 (Supplementary Fig. S5) suggests the key role of considerable strain and layered structures for the exchange coupling. The blocking temperature (TB) above which the symmetric magnetic loops are recovered independently of the cooling process, is of great importance for studying the strong coupling in LSMO. The dependence of the EB field on temperature is depicted in Supplementary Fig. S6 and summarized in Fig. 2b. The results of coercivity HC are also shown for a comparison. The EB field decreases with increasing the temperature, finally vanishing at around TB = 110 K. Also, the enhancement of HEB with the decrease of temperature indicates that the possible lateral phase separated LSMO, reduced in lower temperatures, should not be in charge of the EB effect observed33,34,35. This TB is self-consistent with the Néel temperature TN of LaSrMnO4 of ~110K14 and sets a temperature limit for the onset of the pinning activity for the AFM-based phase in LSMO. In general, G-type AFM LaSrMnO4 could not contribute to the EB behavior because of its compensated spins arrangement36,37, but recently spin canting37 and the Dzyaloshinskii-Moriya interaction38 are proposed to drive the exchange bias at FM/G-AFM interface. On the other hand, the monotonous decay of HC with temperature is different to some other known EB systems which have a peak at TB29. It means that AFM-based phase in our case is not in a stable equilibrium and the thermal effect strongly weakens its coupling with FM7,36.
Intrinsic exchange bias in a LSMO “single” film.
(a), M–H loops of the 45 u.c. sample measured at 5 K after field cooling from room temperature in +20 kOe (solid spheres) and −20 kOe (open circles). For clarity, only the data between −4.5 and 4.5 kOe are shown in the figures, while the actual measurements were carried out between −20 and 20 kOe. (b), Temperature dependence of HEB and HC for the sample. The solid lines through the data points are guides to the eye.
We now turn towards the unstable spin state in the AFM and the origin of EB effect. Hysteresis loops were measured at T = 5 K, with various cooling fields (Hcool = 0.02, 0.2, 1, 2, 5, 20, 50 and 70 kOe) (Fig. 3a). HEB as a function of Hcool is subsequently summarized in the inset of Fig. 3a. We note that HEB increases rapidly to 293 Oe as Hcool = 2 kOe and then decreases monotonously to 142 Oe after cooling in a field of 70 kOe. Such a decrease of HEB under a large cooling field could be explained by the fact that the exchange coupling is reduced by the large Zeeman coupling36,39. This behavior normally occurs at a compensated AFM/FM interface associated with the interfacial canted AFM moments, which would be frozen at low temperatures37,39,40,41. The frozen state, most likely exists at LaSrMnO4/LSMO interface in our system, is reaffirmed by the field-cooling (FC) and zero-field-cooling (ZFC) magnetization versus temperature (M–T) curves measured under various magnetic fields of 50, 100, 200, 300 and 500 Oe (Fig. 3b). There are two prominent features in the data: a peak in the ZFC M–T curves (Tpeak) and a bifurcation between the ZFC and FC curves below the irreversibility temperature Tirr. These phenomena are quite characteristic for M–T curves involving spin glass40. Two characteristic temperatures (Tpeak and Tirr) are very close especially with a lower measurement field, while both the Tpeak and Tirr are reduced when the measurement field is enhanced, suggesting that the frozen state is clearly suppressed by a strong field. As presented in the inset of Fig. 3b, the dependence of Tirr on the field follows the Almeida-Thouless line40:

where TF is the zero-field spin glass freezing temperature and ΔJ parameters the width of the distribution of the exchange interaction. The linear fit of the Almeida-Thouless line bolsters the existence of the spin glass behavior in LSMO with a freezing temperature TF ≈ 147 K.
Demonstration of the LSMO/spin glass interface responsible for the exchange bias.
(a), M-H loops measured at 5 K after field cooling from room temperature in different magnetic fields of 0.02, 0.2, 1, 2, 5, 20, 50 and 70 kOe. HEB is shown as a function of the cooling field in the inset. (b), M-T curves measured under various fields (H = 50, 100, 200, 300 and 500 Oe). The solid and dashed lines are the FC and ZFC data, respectively. The corresponding plot of H2/3 vs Tirr and linear fitting to Eq. (1) are shown in the inset. (c), Real χ' and imaginary χ″ ac magnetic susceptibility components at different frequencies as a function of temperature. The arrow indicates the shift of the maximum with increasing frequency and temperature.
To further investigate the dynamics of spin glass in LSMO, ac susceptibility measurements were carried out in the range of 5–300 K with a field of 10 Oe and different frequencies (0.1–500 Hz), as presented in Fig. 3c. Apparently, frequencies dependent maxima are clearly recognizable for both the real (χ') and the imaginary (χ″) components of the magnetic susceptibility. Such maxima are intimately correlated to the frozen of moments with decreasing temperature40,42. As the frequency of the field is ramped up, the maxima become weaker and shift slightly to higher temperatures, which is due to the elongation of action time induced frozen delay42. The presence of spin glass as a result of magnetic frustration can be linked to the competition between the AFM super-exchange and the FM double-exchange interactions at the interface of LaSrMnO4/LSMO41. We then conclude that the canted AFM moments associated with spin glass behavior is responsible for the EB effect.
Measurements performed over a range of LSMO thicknesses offer further insight into the EB effect and growth dynamics of self-assembled structures. A series of LSMO with different thicknesses on LSAO were then prepared. The EB behavior as a function of LSMO thickness is shown in Fig. 4a and its inset. The increase of film thickness to 45 u.c. boosts the HEB to the maximum of 246 Oe and then HEB is reduced to 30 Oe for 150 u.c as expected, ascribed to the thick FM layer in the EB system29. Particularly, there is no resolvable exchange bias in fully strained 7 u.c. LSMO, indicating the absence of AFM phase in such ultrathin films. We confirm this speculation by a HAADF-STEM image and corresponding EDX results (Supplementary Fig. S7). Explanations may include that there is no lattice relaxation and space for phase separation in ultrathin films with several monolayers. At the initial growth stage (7 u.c.), the lattice volume of LSMO (53.4 Å3) is much smaller than that of its bulk (57.9 Å3), indicating the existence of a large compressive strain (Supplementary Fig. S8). Consequently, both the magnetism and conductivity are seriously suppressed, leading to a nonmetallic and weak-ferromagnetic LSMO with a low Curie temperature (TC) of ~122 K, as shown in Fig. 4b and c. When the LSMO thickness increases, the lattice volume approaches to the value of the bulk, causing the monotonous enhancement of both TC and conductivity. The 45 u.c. LSMO sample shows a transition from a nonmetallic to metallic state considering the tendency of the temperature dependent resistivity. Nevertheless the thick samples, e.g., 150 u.c., show a much lower resistivity with a typical metallic state and TC ≈ 310 K.
Magnetic and electric properties for LSMO samples with various thicknesses.
(a), Normalized M-H curves measured at 5 K after field cooling from room temperature in 20 kOe. M-T and R-T curves for different LSMO thicknesses (7, 30, 45, 60 and 150 u.c.) are presented in (b) and (c), respectively. For clarity, the moment in M-T curves are multiplied by a coefficient as shown.
A comparison among the microstructures and physical properties of samples with different thicknesses, especially for the samples from 7 u.c. to 45 u.c., provides the growth dynamics for LSMO grown under large compressive strain as follows: initially a highly strained LSMO film is grown on LSAO showing a non-equilibrium state. Subsequently, both Mn and Sr are squeezed out by the interfacial strain and the position of Mn is compensated by the diffusion of Al from the substrate, forming the LAO-like structure, above which AFM LaSrMnO4 with one crystal cell emerges and then it contacts with the FM LSMO with the formation of FM/spin glass interface. Both LAO-like and LaSrMnO4 phases serve as buffer layers for the growth of normal LSMO and most of the strain is released by these self-assembled structures. Differently, such interface engineering could not be observed in the LSMO deposited on SrTiO3 (001) substrates due to the small film/substrate mismatch, whose strain energy could be released by a simple lattice distortion16. Correspondingly, we could not find any EB behavior for the LSMO with various thicknesses grown on the SrTiO3 substrates (Supplementary Fig. S9), while for the films grow on LaAlO3 (001) substrates the HEB are in between (SrTiO3 and LSAO).
Discussion
The structural reconstruction of LSMO displays an enlarged image of interfacial state of manganite perovskite film, which might provide a new and in-depth understanding of the magnetic and electric frustration at the interface. The strain engineering design results in the formation of antiferromagnetic LaSrMnO4 and ferromagnetic LSMO interface through self-assembly in a nominally “single” LSMO film. The self-assembled structures with tailored functionalities that are inaccessible in uniform films include but not limited to the manganites. Hence this self-assembly and corresponding growth dynamics provide a conceptually novel vision for interface engineering on the growth of complex oxide films. The direct observation of antiferromagnetic phase near the interface and the intrinsic exchange bias derived from the coupling between LSMO/spin glass would advance the insight into the contradictory situation of the interface of LSMO. It paves a new way for investigating the dead layer and more studies are needed to fully understand the self-assembly and the exchange bias observed in manganite films. Besides its fundamental significance, the creation of a well-defined exchange bias system by self-assembly demonstrates the abroad ability for the fabrication of the biasing components of spintronics in a simplified way.
Methods
Sample preparation
All of the samples were grown using pulsed layer deposition (PLD) from a stoichiometric La2/3Sr1/3MnO3 target by applying a KrF excimer laser at a rate of 1.16 nm/min. During growth, the substrates (LaSrAlO4, SrTiO3 and LaAlO3) were held at 700°C and in an oxygen background pressure of 200 mTorr. The growth was monitored in situ by RHEED (reflection high-energy electron diffraction) analysis allowing precise control of the thickness at the unit cell scale and accurate characterization of the growth dynamics (Supplementary Fig. S10a). After the growth, the samples were slowly cooled to room temperature in 300 Torr of oxygen at a rate of ~5°C/min to improve the oxidation level.
Sample characterization
Microstructures of cross-sectional samples were investigated by aberration-corrected HAADF-STEM in a Titan™ G2 80–200 with ChemiSTEM™ FEI with the resolution of 160 pm and 80 pm for image and probe corrector, respectively. EDX as well as EELS were performed with atomic resolution in order to probe the atomic structure of the interfaces and to check chemical interdiffusion. A Quantum Design superconducting quantum interference device (SQUID) measurement system (MPMS-7) was used to measure the magnetic properties in the temperature range 5–350 K with the magnetic field (H) applied in-plane along the (100) direction of the substrate. All the M–H (magnetization versus magnetic field) and the field-cooling M–T (magnetization versus temperature) measurements in this work without special statement were carried out after cooling down to 5 K in a magnetic field of 20 kOe and 2 kOe, respectively. Conductivity was determined in a Van der Pauw four-probe configuration by the ET9000 electric transport measurement system.
References
Hwang, H. Y. et al. Emergent phenomena at oxide interfaces. Nature Mater. 11, 103–113 (2012).
Chappert, C., Fert, A. & Nguyen Van Dau, F. The emergence of spin electronics in data storage. Nature Mater. 6, 813–823 (2007).
Hu, J. M., Li, Z., Chen, L. Q. & Nan, C. W. High-density magnetoresistive random access memory operating at ultralow voltage at room temperature. Nature Commun. 2, 553 (2011).
Ohtomo, A. & Hwang, H. Y. A high-mobility electron gas at the LaAlO3/SrTiO3 heterointerface. Nature 427, 423–426 (2004).
Herranz, G., Sánchez, F., Dix, N., Scigaj, M. & Fontcuberta, J. High mobility conduction at (110) and (111) LaAlO3/SrTiO3 interfaces. Sci. Rep. 2, 758 (2012).
Wu, S. M. et al. Reversible electric control of exchange bias in a multiferroic field-effect device. Nature Mater. 9, 756–761 (2010).
Gibert, M. et al. Exchange bias in LaNiO3-LaMnO3 superlattices. Nature Mater. 11, 195–198 (2012).
Choi, K. J. et al. Enhancement of ferroelectricity in strained BaTiO3 thin films. Science 306, 1005–1009 (2004).
Rogdakis, K. et al. Tunable ferroelectricity in artificial tri-layer superlattices comprised of non-ferroic components. Nature Commun. 3, 1064 (2012).
Bibes, M., Villegas, J. E. & Barthélémy, A. Ultrathin oxide films and interfaces for electronics and spintronics. Adv. Phys. 60, 5–84 (2011).
Yamada, H. et al. Engineered interface of magnetic oxides. Science 305, 646–648 (2004).
Barraud, C. et al. Unravelling the role of the interface for spin injection into organic semiconductors. Nature Phys. 6, 615–620 (2010).
Dagotto, E. Complexity in strongly correlated electronic systems. Science 309, 257 (2005).
Coey, J. M. D., Viret, M. & Molnár, S. von Mixed-valence manganites. Adv. Phys. 48, 167–293 (1999).
Pesquera, D. et al. Surface symmetry-breaking and strain effects on orbital occupancy in transition metal perovskite epitaxial films. Nature Commun. 3, 1189–1195 (2012).
Huijben, M. et al. Critical thickness and orbital ordering in ultrathin La0.7Sr0.3MnO3 films. Phys. Rev. B 78, 094413 (2008).
Dagotto, E., Hotta, T. & Moreo, A. Colossal magnetoresistant materials: the key role of phase separation. Phys. Rep. 344, 1–153 (2001).
Kourkoutis, L. F., Song, J. H., Hwang, H. Y. & Muller, D. A. Microscopic origins for stabilizing room-temperature ferromagnetism in ultrathin manganite layers. Proc. Natl. Acad. Sci. U.S.A. 107, 11682–11685 (2010).
Tebano, A. et al. Evidence of orbital reconstruction at interfaces in ultrathin La0.67Sr0.33MnO3 films. Phys. Rev. Lett. 100, 137401 (2008).
Lepetit, M. B., Mercey, B. & Simon, C. Interface effects in perovskite thin films. Phys. Rev. Lett. 108, 087202 (2012).
Lee, J.–S. et al. Hidden magnetic configuration in epitaxial La1-xSrxMnO3 films. Phys. Rev. Lett. 105, 257204 (2010).
Freeland, J. W. et al. Full bulk spin polarization and intrinsic tunnel barriers at the surface of layered manganites. Nature Mater. 4, 62–67 (2005).
Sun, J. Z., Abraham, D. W., Rao, R. A. & Eom, C. B. Thickness-dependent magnetotransport in ultrathin manganite films. Appl. Phys. Lett. 74, 3017 (1999).
Peng, R. et al. The origin of the dead-layer at the La0.67Sr0.33MnO3/SrTiO3 interface and dead-layer reduction via interfacial engineering. arXiv:1301.4822 (2013).
Meiklejohn, W. H. & Bean, C. P. New magnetic anisotropy. Phys. Rev. 102, 1413–1414 (1956).
Wang, Y. Y. et al. Room-temperature perpendicular exchange coupling and tunneling anisotropic magnetoresistance in an antiferromagnet-based tunnel junction. Phys. Rev. Lett. 109, 137201 (2012).
MacManus-Driscoll, J. L. et al. Strain control and spontaneous phase ordering in vertical nanocomposite heteroepitaxial thin films. Nature Mater. 7, 314–320 (2008).
Niebieskikwiat, D. & Salamon, M. B. Intrinsic interface exchange coupling of ferromagnetic nanodomains in a charge ordered manganite. Phy. Rev. B 72, 174422 (2005).
Karmakar, S. et al. Evidence of intrinsic exchange bias and its origin in spin-glass-like disordered L0.5Sr0.5MnO3 manganites (L = Y, Y0.5Sm0.5 and Y0.5La0.5). Phy. Rev. B 77, 144409 (2008).
Giri, S. K., Poddar, A. & Nath, T. K. Evidence of exchange bias effect and surface spin glass ordering in electron doped Sm0.09Ca0.91MnO3 nanomanganites. J. Appl. Phys. 112, 113903 (2012).
Xie, B. T., Zhao, Y. G. & Xiong, C. M. Capacitance characteristics of phase separated La0.5Ca0.5MnO3/Nb–SrTiO3 p-n junction. Appl. Phys. Lett. 93, 072112 (2008).
Samet, L. et al. EELS study of interfaces in magnetoresistive LSMO/STO/LSMO tunnel junctions. Eur. Phys. J. B 34, 179–192 (2003).
Becker, T. et al. Intrinsic inhomogeneities in manganite thin films investigated with scanning tunneling spectroscopy. Phys. Rev. Lett. 89, 237203 (2002).
Tebano, A. et al. Strain-induced phase separation in La0.7Sr0.3MnO3 thin films. Phys. Rev. B 74, 245116 (2006).
Dagotto, E. Nanoscale Phase Separation and Colossal Magnetoresistance (Springer-Verlag, Berlin, 2003).
Kiwi, M. Exchange bias theory. J. Magn. Magn. Mater. 234, 584–595 (2001).
Yu, P. et al. Interface ferromagnetism and orbital reconstruction in BiFeO3-La0.7Sr0.3MnO3 heterostructures. Phys. Rev. Lett. 105, 027201 (2010).
Dong, S. et al. Exchange bias driven by the Dzyaloshinskii-Moriya interaction and ferroelectric polarization at G-type antiferromagnetic perovskite interfaces. Phys. Rev. Lett. 103, 127201 (2009).
Bianco, L. Del et al. Field-cooling dependence of exchange bias in a granular system of Fe nanoparticles embedded in an Fe oxide matrix. Phys. Rev. B 70, 052401 (2004).
Binder, K. & Young, A. P. Spin glasses: Experimental facts, theoretical concepts and open questions. Rev. Mod. Phys. 58, 801–976 (1986).
Ding, J. F. et al. Interfacial spin glass state and exchange bias in manganite bilayers with competing magnetic orders. Phys. Rev. B 87, 054428 (2013).
Alonso, J. et al. Crossover from superspin glass to superferromagnet in FexAg100-x nanostructured thin films (20 ≤ x ≤ 50). Phys. Rev. B 82, 054406 (2010).
Acknowledgements
The authors are grateful to Prof. Z. Zhang, Z. J. Shen, J. H. Hong of Zhejiang University for the assistance of TEM characterization and to Prof. G. A. Gehring and Prof. P. Yu for fruitful discussions and critical reading of manuscript. The authors also acknowledge Beamline BL08U in Shanghai Synchrotron Radiation Facility (SSRF) for XLD measurements. This work was supported by the National Natural Science Foundation of China (Grant Nos. 51322101, 51202125 and 51231004), National Basic Research Program of China (Grant No. 2010CB832905) and National Hi-tech (R&D) project of China (Grant no. 2012AA03A706).
Author information
Authors and Affiliations
Contributions
B.C. and G.Y.W. prepared the samples. B.C. and C.S. carried out the measurements. C.S. and F.P. conceived and directed the project. F.Z. and H.J.M. provided advice on the experiments. All authors participated in discussing the data and writing the manuscript.
Ethics declarations
Competing interests
The authors declare no competing financial interests.
Electronic supplementary material
Supplementary Information
SREP-13-02632-Supplemantary information
Rights and permissions
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. To view a copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/
About this article
Cite this article
Cui, B., Song, C., Wang, G. et al. Strain engineering induced interfacial self-assembly and intrinsic exchange bias in a manganite perovskite film. Sci Rep 3, 2542 (2013). https://doi.org/10.1038/srep02542
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/srep02542
This article is cited by
-
Influence of substrate-induced strain on exchange bias effect in YSMO/LSMO heterostructures
Bulletin of Materials Science (2023)
-
Interfacial engineering manipulation of magnetic anisotropy evolution via orbital reconstruction in low-dimensional manganite superlattices
Science China Materials (2022)
-
Intrinsic exchange bias effect in strain-engineered single antiferromagnetic LaMnO3 films
Science China Materials (2019)
-
Atomic-scale engineering of ferroelectric-ferromagnetic interfaces of epitaxial perovskite films for functional properties
Scientific Reports (2017)
-
The Exchange Bias of LaMnO3/LaNiO3 Superlattices Grown along Different Orientations
Scientific Reports (2017)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.