Skip to main content

Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.

  • Perspective
  • Published:

Controlling the interaction of ultracold alkaline-earth atoms

Nature Reviews Physics volume 2, pages 213–220 (2020)Cite this article

Subjects

A Publisher Correction to this article was published on 26 May 2020

This article has been updated

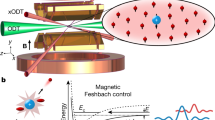
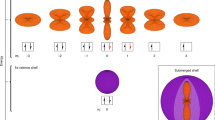
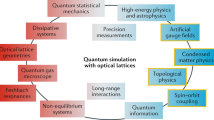
Abstract

Ultracold alkaline-earth atoms are used in precision measurements and quantum simulation. Because of their unique atomic structure, they could enable the study of problems in quantum many-body systems, such as the simulation of synthetic gauge fields, Kondo and SU(N) physics. But to fully exploit this potential, the capability to tune the interatomic interaction to the strongly interacting regime is needed. Several theoretical proposals and experimental demonstrations have shown that both the spin-independent and spin-exchange interaction can be tuned to resonance in the alkaline-earth atoms. In this Perspective, we review these advances and discuss the opportunities brought by these interaction-control tools for future quantum simulation studies.

This is a preview of subscription content, access via your institution

Access options

Buy this article

  • Purchase on Springer Link
  • Instant access to full article PDF
Buy now

Prices may be subject to local taxes which are calculated during checkout

Fig. 1: Schematic illustration of single-atom energy level and interaction potential.
Fig. 2: Theoretical proposal and experimental demonstration of orbital Feshbach resonance in 173Yb atoms.
Fig. 3: Theoretical proposal and experimental demonstration of controlling the spin-exchange interaction.

Similar content being viewed by others

Change history

References

  1. Bloch, I., Dalibard, J. & Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885–964 (2008).

    ADS  Google Scholar 

  2. Bloch, I., Dalibard, J. & Nascimbène, S. Quantum simulations with ultracold quantum gases. Nat. Phys. 8, 267–276 (2012).

    Google Scholar 

  3. Gross, C. & Bloch, I. Quantum simulations with ultracold atoms in optical lattices. Science 357, 995–1001 (2017).

    ADS  Google Scholar 

  4. Goldman, N., Budich, J. C. & Zoller, P. Topological quantum matter with ultracold gases in optical lattices. Nat. Phys. 12, 639–645 (2016).

    Google Scholar 

  5. Takasu, Y. et al. Spin-singlet Bose–Einstein condensation of two-electron atoms. Phys. Rev. Lett. 91, 040404 (2003).

    ADS  Google Scholar 

  6. Fukuhara, T., Takasu, Y., Kumakura, M. & Takahashi, Y. Degenerate Fermi gases of ytterbium. Phys. Rev. Lett. 98, 030401 (2007).

    ADS  Google Scholar 

  7. Kraft, S., Vogt, F., Appel, O., Riehle, F. & Sterr, U. Bose–Einstein condensation of alkaline earth atoms: 40Ca. Phys. Rev. Lett. 103, 130401 (2009).

    ADS  Google Scholar 

  8. Stellmer, S., Tey, M. K., Huang, B., Grimm, R. & Schreck, F. Bose–Einstein condensation of strontium. Phys. Rev. Lett. 103, 200401 (2009).

    ADS  Google Scholar 

  9. Martinez de Escobar, Y. N. et al. Bose–Einstein condensation of Sr 84. Phys. Rev. Lett. 103, 200402 (2009).

    Google Scholar 

  10. DeSalvo, B. J., Yan, M., Mickelson, P. G., Martinez de Escobar, Y. N. & Killian, T. C. Degenerate Fermi gas of Sr 87. Phys. Rev. Lett. 105, 030402 (2010).

    ADS  Google Scholar 

  11. Cazalilla, M. A. & Rey, A. M. Ultracold Fermi gases with emergent SU(N) symmetry. Rep. Prog. Phys. 77, 124401 (2014).

    ADS  Google Scholar 

  12. Daley, A. J. Quantum computing and quantum simulation with group-II atoms. Quantum Inf. Process. 10, 865–884 (2011).

    Google Scholar 

  13. Blagoev, K. B. & Komarovskii, V. A. Lifetimes of levels of neutral and singly ionized lanthanide atoms. At. Data Nucl. Data Tables 56, 1–40 (1994).

    ADS  Google Scholar 

  14. Bloom, B. J. et al. An optical lattice clock with accuracy and stability at the 10−18 level. Nature 506, 71–75 (2014).

    ADS  Google Scholar 

  15. Ludlow, A. D., Boyd, M. M., Ye, J., Peik, E. & Schmidt, P. O. Optical atomic clocks. Rev. Mod. Phys. 87, 637–701 (2015).

    ADS  Google Scholar 

  16. Ushijima, I., Takamoto, M., Das, M., Ohkubo, T. & Katori, H. Cryogenic optical lattice clocks. Nat. Photonics 9, 185–189 (2015).

    ADS  Google Scholar 

  17. Nicholson, T. L. et al. Systematic evaluation of an atomic clock at 2 \(\times \) 10−18 total uncertainty. Nat. Commun. 6, 6896 (2015).

    ADS  Google Scholar 

  18. Schioppo, M. et al. Ultra-stable optical clock with two cold-atom ensembles. Nat. Photonics 11, 48–52 (2017).

    ADS  Google Scholar 

  19. Campbell, S. L. et al. A Fermi-degenerate three-dimensional optical lattice clock. Science 358, 90–94 (2017).

    ADS  Google Scholar 

  20. Marti, G. E. et al. Imaging optical frequencies with 100 \(\mu \)Hz precision and 1.1 \(\mu \)m resolution. Phys. Rev. Lett. 120, 103201 (2018).

    ADS  Google Scholar 

  21. Wall, M. L. et al. Synthetic spin–orbit coupling in an optical lattice clock. Phys. Rev. Lett. 116, 035301 (2016).

    ADS  Google Scholar 

  22. Livi, L. F. et al. Synthetic dimensions and spin–orbit coupling with an optical clock transition. Phys. Rev. Lett. 117, 220401 (2016).

    ADS  Google Scholar 

  23. Kolkowitz, S. et al. Spin–orbit-coupled fermions in an optical lattice clock. Nature 542, 66–70 (2017).

    ADS  Google Scholar 

  24. Bromley, S. L. et al. Dynamics of interacting fermions under spin–orbit coupling in an optical lattice clock. Nat. Phys. 14, 399–404 (2018).

    Google Scholar 

  25. Goldman, N., Juzeliunas, G., Öhberg, P. & Speilman, I. B. Light-induced gauge fields for ultracold atoms. Rep. Prog. Phys. 77, 126401 (2014).

    ADS  Google Scholar 

  26. Zhai, H. Degenerate quantum gases with spin–orbit coupling: a review. Rep. Prog. Phys. 78, 026001 (2015).

    ADS  MathSciNet  Google Scholar 

  27. Boyd, M. M. et al. Optical atomic coherence at the 1-second time scale. Science 314, 1430–1433 (2006).

    ADS  Google Scholar 

  28. Cazalilla, M. A., Ho, A. F. & Ueda, M. Ultracold gases of ytterbium: ferromagnetism and Mott states in an SU(6) Fermi system. New J. Phys. 11, 103033 (2009).

    ADS  Google Scholar 

  29. Gorshkov, A. V. et al. Two-orbital SU(N) magnetism with ultracold alkaline-earth atoms. Nat. Phys. 6, 289–295 (2010).

    Google Scholar 

  30. Taie, S. et al. Realization of a SU(2) \(\times \) SU(6) system of Fermions in a cold atomic gas. Phys. Rev. Lett. 105, 190401 (2010).

    ADS  Google Scholar 

  31. Stellmer, S., Grimm, R. & Schreck, F. Detection and manipulation of nuclear spin states in fermionic strontium. Phys. Rev. A 84, 043611 (2011).

    ADS  Google Scholar 

  32. Taie, S., Yamazaki, R., Sugawa, S. & Takahashi, Y. An SU(6) Mott insulator of an atomic Fermi gas realized by large-spin Pomeranchuk cooling. Nat. Phys. 8, 825–830 (2012).

    Google Scholar 

  33. Zhang, X. et al. Spectroscopic observation of SU(N)-symmetric interactions in Sr orbital magnetism. Science 345, 1467–1473 (2014).

    ADS  Google Scholar 

  34. Pagano, G. et al. A one-dimensional liquid of fermions with tunable spin. Nat. Phys. 10, 198–201 (2014).

    Google Scholar 

  35. Hofrichter, C. et al. Direct probing of the Mott crossover in the SU(N) Fermi–Hubbard Model. Phys. Rev. X 6, 021030 (2016).

    Google Scholar 

  36. Bishof, M. et al. Resolved atomic interaction sidebands in an optical clock transition. Phys. Rev. Lett. 106, 250801 (2011).

    ADS  Google Scholar 

  37. Martin, M. J. et al. A quantum many-body spin system in an optical lattice clock. Science 341, 632–636 (2013).

    ADS  MathSciNet  MATH  Google Scholar 

  38. Clivati, C. et al. Measuring absolute frequencies beyond the GPS limit via long-haul optical frequency dissemination. Optics Express 24, 11865–11875 (2016).

    ADS  Google Scholar 

  39. Franchi, L. et al. State-dependent interactions in ultracold 174Yb probed by optical clock spectroscopy. N. J. Phys. 19, 103037 (2017).

    Google Scholar 

  40. Goban, A. et al. Emergence of multi-body interactions in a fermionic lattice clock. Nature 563, 369–373 (2018).

    ADS  Google Scholar 

  41. Köhler, T., Góral, K. & Julienne, P. S. Production of cold molecules via magnetically tunable Feshbach resonances. Rev. Mod. Phys. 78, 1311–1361 (2006).

    ADS  Google Scholar 

  42. Chin, C., Grimm, R., Julienne, P. S. & Tiesinga, E. Feshbach resonances in ultracold gases. Rev. Mod. Phys. 82, 1225–1285 (2010).

    ADS  Google Scholar 

  43. Giorgini, S., Pitaevskii, L. P. & Stringari, S. Theory of ultracold atomic Fermi gases. Rev. Mod. Phys. 80, 1215–1274 (2008).

    ADS  Google Scholar 

  44. Strinati, G. C., Pieri, P., Roepke, G., Schuck, P. & Urban, M. The BCS–BEC crossover: from ultra-cold Fermi gases to nuclear systems. Phys. Rep. 738, 1–76 (2018).

    ADS  MathSciNet  MATH  Google Scholar 

  45. Olshanii, M. Atomic scattering in the presence of an external confinement and a gas of impenetrable bosons. Phys. Rev. Lett. 81, 938 (1998).

    ADS  Google Scholar 

  46. Zhang, R., Cheng, Y., Zhai, H. & Zhang, P. Orbital Feshbach resonance in alkali-earth atoms. Phys. Rev. Lett. 115, 135301 (2015).

    ADS  Google Scholar 

  47. Höfer, M. et al. Observation of an orbital interaction-induced Feshbach resonance in 173Yb. Phys. Rev. Lett. 115, 265302 (2015).

    ADS  Google Scholar 

  48. Pagano, G. et al. Strongly interacting gas of two-electron fermions at an orbital Feshbach resonance. Phys. Rev. Lett. 115, 265301 (2015).

    ADS  Google Scholar 

  49. Oppong, N. D. et al. Observation of coherent multiorbital polarons in a two-dimensional Fermi gas. Phys. Rev. Lett. 122, 193604 (2019).

    ADS  Google Scholar 

  50. Zhang, R. et al. Kondo effect in alkaline-earth-metal atomic gases with confinement-induced resonances. Phys. Rev. A 93, 043601 (2016).

    ADS  Google Scholar 

  51. Cheng, Y., Zhang, R., Zhang, P. & Zhai, H. Enhancing Kondo coupling in alkaline-earth-metal atomic gases with confinement-induced resonances in mixed dimensions. Phys. Rev. A 96, 063605 (2017).

    ADS  Google Scholar 

  52. Riegger, L. et al. Localized magnetic moments with tunable spin exchange in a gas of ultracold fermions. Phys. Rev. Lett. 120, 143601 (2018).

    ADS  Google Scholar 

  53. Boyd, M. M. et al. Nuclear spin effects in optical lattice clocks. Phys. Rev. A 76, 022510 (2007).

    ADS  Google Scholar 

  54. Cheng, Y., Zhang, R. & Zhang, P. Quantum defect theory for the orbital Feshbach resonance. Phys. Rev. A 95, 013624 (2017).

    ADS  Google Scholar 

  55. Regal, C. A., Ticknor, C., Bohn, J. L. & Jin, D. S. Creation of ultracold molecules from a Fermi gas of atoms. Nature 424, 47–59 (2003).

    ADS  Google Scholar 

  56. O’Hara, K. M., Hemmer, S. L., Gehm, M. E., Granade, S. R. & Thomas, J. E. Observation of a strongly interacting degenerate Fermi gas of atoms. Science 298, 2179–2182 (2002).

    ADS  Google Scholar 

  57. Scazza, F. et al. Observation of two-orbital spin-exchange interactions with ultracold SU(N)-symmetric fermions. Nat. Phys. 10, 779–784 (2014). Corrigendum Nat. Phys. 11, 514 (2015).

    Google Scholar 

  58. Cappellini, G. et al. Direct observation of coherent interorbital spin-exchange dynamics. Phys. Rev. Lett. 113, 120402 (2014). Erratum Phys. Rev. Lett. 114, 239903 (2015).

    ADS  Google Scholar 

  59. Barber, Z. W. et al. Optical lattice induced light shifts in an Yb atomic clock. Phys. Rev. Lett. 100, 103002 (2008).

    ADS  Google Scholar 

  60. Dzuba, V. A. & Derevianko, A. Dynamic polarizabilities and related properties of clock states of the ytterbium atom. J. Phys. B 43, 074011 (2010).

    ADS  Google Scholar 

  61. Hewson, A. C. The Kondo Problem to Heavy Fermions (Cambridge Univ. Press, 1993).

  62. Zhang, R. & Zhang, P. Control of spin-exchange interaction between alkali-earth-metal atoms via confinement-induced resonances in a quasi-(1.0)-dimensional system. Phys. Rev. A 98, 043627 (2018).

    ADS  Google Scholar 

  63. Zhang, R. & Zhang, P. Tight-binding Kondo model and spin-exchange collision rate of alkaline-earth atoms in a mixed-dimensional optical lattice. Phys. Rev. A 101, 013636 (2020).

    ADS  Google Scholar 

  64. Ji, Q., Zhang, R., Zhang, X. & Zhang, W. Confinement-induced resonance of alkaline-earth-metal-like atoms in anisotropic quasi-one-dimensional traps. Phys. Rev. A 98, 053613 (2018).

    ADS  Google Scholar 

  65. Xu, J. et al. Reaching a Fermi-superfluid state near an orbital Feshbach resonance. Phys. Rev. A 94, 033609 (2016).

    ADS  Google Scholar 

  66. Iskin, M. Two-band superfluidity and intrinsic Josephson effect in alkaline-earth-metal Fermi gases across an orbital Feshbach resonance. Phys. Rev. A 94, 011604 (2016).

    ADS  Google Scholar 

  67. Iskin, M. Trapped 173Yb Fermi gas across an orbital Feshbach resonance. Phys. Rev. A 95, 013618 (2017).

    ADS  Google Scholar 

  68. Mondal, S., Inotani, D. & Ohashi, Y. Photoemission spectrum in the BCS–BEC crossover regime of a rare-earth Fermi gas with an orbital Feshbach resonance. J. Phys. Soc. Jpn 87, 094301 (2018).

    ADS  Google Scholar 

  69. Mondal, S., Inotani, D. & Ohashi, Y. Single-particle excitations and strong coupling effects in the BCS–BEC crossover regime of a rare-earth Fermi gas with an orbital Feshbach resonance. J. Phys. Soc. Jpn 87, 084302 (2018).

    ADS  Google Scholar 

  70. Mondal, S., Inotani, D. & Ohashi, Y. Closed-channel contribution in the BCS–BEC crossover regime of an ultracold Fermi gas with an orbital Feshbach resonance. J. Phys. Conf. Ser. 969, 012017 (2018).

    Google Scholar 

  71. Klimin, S. N., Tempere, J. & Milošević, M. V. Diversified vortex phase diagram for a rotating trapped two-band Fermi gas in the BCS–BEC crossover. New J. Phys. 20, 025010 (2018).

    ADS  Google Scholar 

  72. Laird, E. K., Shi, Z.-Y., Parish, M. M. & Levinsen, J. Frustrated orbital Feshbach resonances in a Fermi gas. Phys. Rev. A 101, 022707 (2020).

    ADS  Google Scholar 

  73. He, L., Wang, J., Peng, S.-G., Liu, X.-J. & Hu, H. Strongly correlated Fermi superfluid near an orbital Feshbach resonance: stability, equation of state, and Leggett mode. Phys. Rev. A 94, 043624 (2016).

    ADS  Google Scholar 

  74. Zhang, Y.-C., Ding, S. & Zhang, S. Collective modes in a two-band superfluid of ultracold alkaline-earth-metal atoms close to an orbital Feshbach resonance. Phys. Rev. A 95, 041603 (2017).

    ADS  Google Scholar 

  75. Zhang, H., Badshah, F., Basit, A. & Ge, G.-Q. Fermi gas of orbital Feshbach resonance in synthetic 1D.1 dimensional optical lattice. Laser Phys. Lett. 15, 115501 (2018).

    ADS  Google Scholar 

  76. Deng, T.-S., Zhang, W. & Yi, W. Tuning Feshbach resonances in cold atomic gases with interchannel coupling. Phys. Rev. A 96, 050701(R) (2017).

    ADS  Google Scholar 

  77. Wang, S., Pan, J.-S., Cui, X., Zhang, W. & Yi, W. Topological Fulde–Ferrell states in alkaline-earth-metal-like atoms near an orbital Feshbach resonance. Phys. Rev. A 95, 043634 (2017).

    ADS  Google Scholar 

  78. Zhou, X., Pan, J.-S., Yi, W., Chen, G. & Jia, S. Interaction-induced exotic vortex states in an optical lattice clock with spin–orbit coupling. Phys. Rev. A 96, 023627 (2017).

    ADS  Google Scholar 

  79. Zhou, X. et al. Symmetry-protected topological states for interacting fermions in alkaline-earth-like atoms. Phys. Rev. Lett. 119, 185701 (2017).

    ADS  Google Scholar 

  80. Zou, P., He, L., Liu, X.-J. & Hu, H. Strongly interacting Sarma superfluid near orbital Feshbach resonances. Phys. Rev. A 97, 043616 (2018).

    ADS  Google Scholar 

  81. Cappellini, G. et al. Coherent manipulation of orbital Feshbach molecules of two-electron atoms. Phys. Rev. X 9, 011028 (2019).

    Google Scholar 

  82. Qi, X. L. & Zhang, S. C. Topological insulators and superconductors. Rev. Mod. Phys. 83, 1057–1110 (2011).

    ADS  Google Scholar 

  83. Cui, X., Lian, B., Ho, T.-L., Lev, B. L. & Zhai, H. Synthetic gauge field with highly magnetic lanthanide atoms. Phys. Rev. A 88, 011601(R) (2013).

    ADS  Google Scholar 

  84. Burdick, N. Q., Tang, Y. & Lev, B. L. Long-lived spin–orbit-coupled degenerate dipolar Fermi gas. Phys. Rev. X 6, 031022 (2016).

    Google Scholar 

  85. Mancini, M. et al. Observation of chiral edge states with neutral fermions in synthetic Hall ribbons. Science 349, 1510–1513 (2015).

    ADS  MathSciNet  MATH  Google Scholar 

  86. Song, S. et al. Spin–orbit-coupled two-electron Fermi gases of ytterbium atoms. Phys. Rev. A 94, 061604(R) (2016).

    ADS  Google Scholar 

  87. Song, S. et al. Observation of symmetry-protected topological band with ultracold fermions. Sci. Adv. 4, 4748 (2018).

    ADS  Google Scholar 

  88. Song, S. et al. Observation of nodal-line semimetal with ultracold fermions in an optical lattice. Nat. Phys. 15, 911–916 (2019).

    Google Scholar 

  89. Chevy, F. Universal phase diagram of a strongly interacting Fermi gas with unbalanced spin populations. Phys. Rev. A 74, 063628 (2006).

    ADS  Google Scholar 

  90. Cui, X. & Zhai, H. Stability of a fully magnetized ferromagnetic state in repulsively interacting ultracold Fermi gases. Phys. Rev. A 81, 041602(R) (2010).

    ADS  Google Scholar 

  91. Massignan, P., Zaccanti, M. & Bruun, G. M. Polarons, dressed molecules, and itinerant ferromagnetism in ultracold Fermi gases. Rep. Prog. Phys. 77, 034401 (2014).

    ADS  Google Scholar 

  92. Chen, J.-G., Deng, T.-S., Yi, W. & Zhang, W. Polarons and molecules in a Fermi gas with orbital Feshbach resonance. Phys. Rev. A 94, 053627 (2016).

    ADS  Google Scholar 

  93. Xu, J. & Qi, R. Polaronic and dressed molecular states in orbital Feshbach resonances. Eur. Phys. J. D 72, 65 (2018).

    ADS  Google Scholar 

  94. Chen, J.-G., Shi, Y.-R., Zhang, X. & Zhang, W. Polarons in alkaline-earth-like atoms with multiple background Fermi surfaces. Front. Phys. 13, 136702 (2018).

    ADS  Google Scholar 

  95. Deng, T.-S. et al. Repulsive polarons in alkaline-earth-metal-like atoms across an orbital Feshbach resonance. Phys. Rev. A 97, 013635 (2018).

    ADS  Google Scholar 

  96. Falco, G. M., Duine, R. A. & Stoof, H. T. C. Molecular Kondo resonance in atomic Fermi gases. Phys. Rev. Lett. 92, 140402 (2004).

    ADS  Google Scholar 

  97. Duan, L.-M. Controlling ultracold atoms in multi-band optical lattices for simulation of Kondo physics. Europhys. Lett. 67, 721–727 (2004).

    ADS  Google Scholar 

  98. Foss-Feig, M., Hermele, M., Gurarie, V. & Rey, A. M. Heavy fermions in an optical lattice. Phys. Rev. A 82, 053624 (2010).

    ADS  Google Scholar 

  99. Foss-Feig, M., Hermele, M. & Rey, A. M. Probing the Kondo lattice model with alkaline-earth-metal atoms. Phys. Rev. A 81, 051603(R) (2010).

    ADS  Google Scholar 

  100. Carmi, A., Oreg, Y. & Berkooz, M. Realization of the SU(N) Kondo effect in a strong magnetic field. Phys. Rev. Lett. 106, 106401 (2011).

    ADS  Google Scholar 

  101. Bauer, J., Salomon, C. & Demler, E. Realizing a Kondo-correlated state with ultracold atoms. Phys. Rev. Lett. 111, 215304 (2013).

    ADS  Google Scholar 

  102. Nishida, Y. SU(3) orbital Kondo effect with ultracold atoms. Phys. Rev. Lett. 111, 135301 (2013).

    ADS  Google Scholar 

  103. Nakagawa, M. & Kawakami, N. Laser-induced Kondo effect in ultracold alkaline-earth fermions. Phys. Rev. Lett. 115, 165303 (2015).

    ADS  Google Scholar 

  104. Isaev, L. & Rey, A. M. Heavy-Fermion valence-bond liquids in ultracold atoms: cooperation of the Kondo effect and geometric frustration. Phys. Rev. Lett. 115, 165302 (2015).

    ADS  Google Scholar 

  105. Kuzmenko, I., Kuzmenko, T., Avishai, Y. & Kikoin, K. Model for overscreened Kondo effect in ultracold Fermi gas. Phys. Rev. B 91, 165131 (2015).

    ADS  Google Scholar 

  106. Isaev, L., Schachenmayer, J. & Rey, A. M. Spin-orbit-coupled correlated metal phase in Kondo lattices: an implementation with alkaline-earth atoms. Phys. Rev. Lett. 117, 135302 (2016).

    ADS  Google Scholar 

  107. Kuzmenko, I., Kuzmenko, T., Avishai, Y. & Jo, G.-B. Coqblin–Schrieffer model for an ultracold gas of ytterbium atoms with metastable state. Phys. Rev. B 93, 115143 (2016).

    ADS  Google Scholar 

  108. Kuzmenko, I., Kuzmenko, T., Avishai, Y. & Jo, G.-B. Multipolar Kondo effect in a \({}^{1}{S}_{0}{-}^{3}{P}_{0}\) mixture of \({}^{1}{S}_{0}{-}^{3}{P}_{0}\)Yb atoms. Phys. Rev. B 97, 075124 (2018).

    ADS  Google Scholar 

  109. Nakagawa, M., Kawakami, N. & Ueda, M. Non-Hermitian Kondo effect in ultracold alkaline-earth atoms. Phys. Rev. Lett. 121, 203001 (2018).

    ADS  Google Scholar 

  110. Kanász-Nagy, M. et al. Exploring the anisotropic Kondo model in and out of equilibrium with alkaline-earth atoms. Phys. Rev. B 97, 155156 (2018).

    ADS  Google Scholar 

  111. Ashida, Y., Shi, T., Banuls, M. C., Cirac, J. I. & Demler, E. Solving quantum impurity problems in and out of equilibrium with the variational approach. Phys. Rev. Lett. 121, 026805 (2018).

    ADS  Google Scholar 

  112. Wenz, A. N. et al. From few to many: observing the formation of a Fermi sea one atom at a time. Science 342, 457–460 (2013).

    ADS  Google Scholar 

  113. Bergeman, T., Moore, M. G. & Olshanii, M. Atom–atom scattering under cylindrical harmonic confinement: numerical and analytic studies of the confinement induced resonance. Phys. Rev. Lett. 91, 163201 (2003).

    ADS  Google Scholar 

Download references

Acknowledgements

This work is supported by the Ministery of Science and Technology (grant nos. 2018YFA0307601 (R.Z.), 2018YFA0306502 (P.Z.), 2016YFA0301600 (H.Z.)) and the National Natural Science Foundation of China (grant nos. 11804268 (R.Z.), 11434011 (P.Z.), 11674393 (P.Z.) and 11734010 (H.Z.)). P.Z. is also supported by the Research Funds of Renmin University of China (Grant No. 16XNLQ03). H.Z. is also supported by the Beijing Outstanding Young Scholar Program.

Author information

Authors and Affiliations

  1. School of Science, Xi’an Jiaotong University, Xi’an, China

    Ren Zhang

  2. Institute for Advanced Study, Tsinghua University, Beijing, China

    Yanting Cheng & Hui Zhai

  3. Department of Physics, Renmin University of China, Beijing, China

    Peng Zhang

Authors
  1. Ren Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yanting Cheng
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Peng Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Hui Zhai
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

The authors contributed equally to all aspects of the article.

Corresponding author

Correspondence to Hui Zhai.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Peer review information

Nature Reviews Physics thanks the anonymous reviewers for their contribution to the peer review of this work.

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, R., Cheng, Y., Zhang, P. et al. Controlling the interaction of ultracold alkaline-earth atoms. Nat Rev Phys 2, 213–220 (2020). https://doi.org/10.1038/s42254-020-0157-9

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1038/s42254-020-0157-9

This article is cited by

Search

Advanced search

Quick links

Nature Briefing

Sign up for the Nature Briefing newsletter — what matters in science, free to your inbox daily.

Get the most important science stories of the day, free in your inbox. Sign up for Nature Briefing