Introduction

Thermodynamic relations are often written as inequalities. A notable example is the Clausius inequality that lower bounds a change in entropy between two equilibrium states by the absorbed heat divided by the temperature at which that heat is absorbed, ΔS ≥ Q/T1. Its importance stems from the fact that physical, chemical or biological transformations may be divided into three distinct categories depending on the sign of the inequality: nonequilibrium (ΔS > Q/T), equilibrium (ΔS = Q/T) and impossible (ΔS < Q/T) transformations. Such inequalities thus make a qualitative assertion about the possible direction of a thermodynamic process and allows one to predict its evolution. However, while the sign of thermodynamic inequalities is clear for initial and final equilibrium states, it usually depends on them, when initial or final states are nonequilibrium states: in some instances, a generalized Clausius inequality of the form ΔS ≥ g(ρi, ρf), where g(ρi, ρf) is a function of initial state ρi and final state ρf, holds, whereas in other cases, a reversed generalized Clausius inequality of the form ΔS ≤ g(ρi, ρf) applies2,3,4.

Understanding thermodynamic behavior at small scales and determining the correct thermodynamic inequalities in these systems is of fundamental and practical interest5,6. The theory of thermodynamics was originally developed for macroscopic systems (such as a gas contained in a cylinder) which inherently interact with an environment large enough that it can be considered as a heat bath (the air around the cylinder, for instance)1. The effect of such a heat reservoir is to thermalize any nonequilibrium state to an equilibrium Gibbs state. In the past decades, this framework has been successfully extended to nonequilibrium microscopic systems in contact with a heat bath5,6. While the usual laws of thermodynamics still hold on average in this case, they have been generalized along single trajectories to account for nonnegligible thermal fluctuations7,8,9. Stochastic thermodynamics has enabled the theoretical and experimental study of the energetics of small systems, from colloidal particles to enzyme and molecular motors7,8,9. This framework has further revealed the importance of information-theoretic quantities, such as information entropy and relative entropy, to characterize the thermodynamics of nonequilibrium irreversible processes7,8,9.

On the other hand, with the emergence of quantum technologies, many laboratories around the world have been investigating nanoscopic systems that are highly isolated from their surroundings10,11. Contrary to the assumptions of standard thermodynamics, nonequilibrium states do not necessarily thermalize in such a situation due to the lack of an external heat bath12,13,14. Non-thermalization occurs in a broad class of classical and quantum systems, including nonlinear interacting systems, such as discrete breathers15 and Fermi–Pasta–Ulam-type systems16, collective monopole modes in noninteracting ideal gases17 as well as strongly coupled integrable many-body systems with long-lived transient states12,13,14. Another prominent example is provided by laser cooling of atoms which plays an essential role in the study of new states of matter and high-resolution spectroscopy18,19. Most laser cooling schemes indeed only induce thermalization of the momentum degrees of freedom18,19. In dense atomic samples, frequent atomic collisions redistribute the energy and establish thermal equilibrium. However, in dilute gases with rare interparticle collisions, the absence of a heat reservoir leads to far from equilibrium states that do not thermalize on their own. This lack of equilibration has profound consequences for the sign of thermodynamic inequalities. A generalized Clausius inequality has been shown to generally hold for nonequilibrium states that are driven by a heat bath to a thermal state2,3,4. By contrast, for initially isolated equilibrium states that dissipatively evolve into a nonthermal state, the generalized Clausius inequality has been predicted to be reversed2,3,4. While this generalized Clausius and its reverse agree for equilibrium processes, the two inequalities prognosticate exact opposite possible nonequilibrium transformations.

We here report the experimental study of both inequalities, and the associated relative entropies, using an initially isolated ultracold atomic sample of few noninteracting Cesium (Cs) atoms in a crossed optical dipole trap18,19. In doing so, we extend far-from-equilibrium experiments usually performed with single microscopic systems, such as colloidal particles7,8,9, to a nanoscopic few-atom system. The difficulty in this situation is to experimentally specify the phase-space distributions of the system that are needed to evaluate the relative entropy. In addition, while most nonequilibrium experiments on closed systems have so far focused on mechanical quenches12,13,14, we consider thermal perturbations. In our setup, axial and radial trap directions are only weakly coupled, making the problem essentially one-dimensional. We prepare an equilibrium Gibbs state of the atomic system by applying a sufficiently long optical molasses pulse that thermalizes position and momentum degrees of freedom18,19. We further employ pulses of degenerate Raman sideband cooling (DRSC)20,21,22 to drive the atomic sample out of equilibrium by only thermalizing momentum coordinates. We measure the position distribution of the atoms by means of position resolved in-situ fluorescence imaging23,24. In combination with numerical Monte-Carlo simulations of the three-dimensional trapping potential, we are able to determine the axial phase-space distribution of the system, from which we evaluate temperature, energy, entropy and relative entropy. We confirm the validity of the generalized Clausius inequality for the thermalization induced by an optical molasses pulse and present the first experimental observation of the reversed generalized Clausius inequality for the nonequilibrium transformation generated by a degenerate Raman pulse.

Results

We begin by considering two thermodynamic processes (Fig. 1a): the first transformation, produced by a degenerate Raman pulse in the experiment, brings an initial equilibrium state ρ1 at temperature T1 to a nonequilibrium state ρ2, whereas the second transformation, triggered by an optical molasses pulse, connects the nonequilibrium state ρ2 to a thermal Gibbs state ρ3 at temperature T3. The variation ΔS23 = S3 − S2 of the (information) entropy, \({S}_{i}=-{k}_{{{{{{{{\rm{B}}}}}}}}}\int\,dzd{v}_{z}\,{\rho }_{i}(z,{v}_{z})\ln {\rho }_{i}(z,{v}_{z})\), where ρi(z, vz) is the (axial) phase-space density of the system and kB the Boltzmann constant, during the second process satisfies25,26,27,28,

$${{\Delta }}{S}_{{{{{{{{\rm{23}}}}}}}}}-\frac{{U}_{{{{{{{{\rm{23}}}}}}}}}}{{T}_{{{{{{{{\rm{3}}}}}}}}}}=D({\rho }_{{{{{{{{\rm{2}}}}}}}}}| | {\rho }_{{{{{{{{\rm{3}}}}}}}}})\ge 0,$$
(1)

where U23 = ∫dzdvz (ρ3 − ρ2)H is the average energy absorbed by the system and \(H(z,{v}_{z})={m}_{{{{{{{{\rm{Cs}}}}}}}}}{v}_{z}^{2}/2+{U}_{z}(z)\) its Hamiltonian. The energy U23 reduces to the heat Q23 exchanged with the environment, when no work is performed on the system, as in our experiment; as usual, we associate work with the energy change induced by a time-dependent trapping potential or an external (possibly time-dependent) nonconservative force5,6,7,8,9. The entropy production is given by the relative entropy between initial and final phase-space distributions, \(D({\rho }_{{{{{{{{\rm{2}}}}}}}}}| | {\rho }_{{{{{{{{\rm{3}}}}}}}}})={k}_{{{{{{{{\rm{B}}}}}}}}}\int\,dzd{v}_{z}\,{\rho }_{2}\ln ({\rho }_{2}/{\rho }_{3})\)29. Since the latter quantity is nonnegative, Eq. (1) implies the usual Clausius inequality. Formula (1) is thus a nonequilibrium, information-theoretic extension of that inequality. On the other hand, the (information) entropy difference ΔS12 = S2 − S1 during the first transformation is2,3,4,

$${{\Delta }}{S}_{{{{{{{{\rm{12}}}}}}}}}-\frac{{U}_{{{{{{{{\rm{12}}}}}}}}}}{{T}_{{{{{{{{\rm{1}}}}}}}}}}=-D({\rho }_{{{{{{{{\rm{2}}}}}}}}}| | {\rho }_{{{{{{{{\rm{1}}}}}}}}})\le 0.$$
(2)

This is an expression of a reversed generalized Clausius inequality that shows that the (information) entropy change is here upper bounded by the ratio of mean energy and temperature. In this case, the system may be regarded as interacting with a nonequilibrium bath: for nonequilibrium passive baths, the energy exchanged is simply the heat Q12, while there is an additional work contribution for nonpassive baths30,31.

Fig. 1: Experimental setup.
figure 1

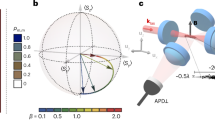
a Schematic of the two thermodynamic processes examined: (i) transition from the equilibrium state ρ1 to the nonequilibrium state ρ2 induced by a degenerate Raman sideband cooling (DRSC) pulse and (ii) transformation from the nonequilibrium state ρ2 to the thermal state ρ3 generated by an optical molasses pulse. b Characterization of the dipole trap potential V(x, y, z) used to store the Cesium (Cs) atoms. The red lines indicate the different directions of the potential cuts shown in the inset: the full potential (blue lines) is there compared to a separable potential approximation (red dashed lines) along the x-, y- and z-direction, as well as a diagonal cut indicated by its length l. c Typical fluorescence images of the few Cs atom samples showing single Cs atoms as bright spots. By extracting the atomic positions along the z-axis from the images and binning them in a histogram, the atomic density distribution is measured. d For every state of the experimental protocol, the position distribution is measured at four evolution times, t = 0, 1.45, 2.90 and 4.35 ms (blue bars). By combining the measured dynamics with a model (solid lines), the full axial phase-space distribution can be determined (insets).

Full size image

In our experiment, we initialize the system by trapping up to 12 Cs atoms in a magneto-optical trap and then transfer them into a crossed optical dipole trap. At typical temperatures of about 10 μK, the atomic collision rate of 113 Hz for the maximum number of 12 atoms is smaller than the inverse evolution time observed in the experiment. As a result, the system is effectively noninteracting. The crossed optical dipole trap at a wavelength λ = 1064 nm is formed by a horizontal trap beam (at a beam waist of 21 μm and power of 1 W) and a second vertical beam (with waist of 165 μm and power of 8 W). Figure 1b shows cuts through the total potential, V(x, y, z) = Vx(x) + Vy(y) + Vz(z), created by these Gaussian laser beams (including the contribution of gravity). The potential is strongly anharmonic but separable (Fig. 1b). Atomic motions along radial and axial directions are hence decoupled (Methods), and the problem is essentially one-dimensional.

We first prepare the sample in a thermal state ρ1 at temperature T1 by applying a (tunable) optical molasses onto the atoms (Methods). By varying the detuning of the molasses cooling laser, the temperature T1 can be controlled between 16 μK and 21 μK. We then drive the system to a nonequilibrium state ρ2 with the help of a degenerate Raman pulse (Methods). To that end, the atoms in the crossed dipole trap are pinned during the cooling pulse by a three dimensional optical lattice created by the DRSC laser beams, leaving their position distribution unchanged. At the same time, the cooling effect of the DRSC redistributes the atomic velocities, leading to a Maxwell distribution of the (axial) velocity component \({\tilde{f}}_{M}({v}_{z})\propto \exp \left(-{m}_{{{{{{{{\rm{Cs}}}}}}}}}{v}_{z}^{2}/2{k}_{{{{{{{{\rm{B}}}}}}}}}{T}_{{{{{{{{\rm{R}}}}}}}}}\right)\) characterized by an effective Raman temperature TR. Here, \({\tilde{f}}_{i}({v}_{z},t)\) generically denotes the momentum projection of the phase-space distribution ρi(z, vz, t). We finally thermalize the atomic cloud to a Gibbs state ρ3 at temperature T3 by applying a second pulse of optical molasses light.

We next measure the time evolution of these states by extracting the (axial) z-positions of every single Cs atom from fluorescence images (Fig. 1c). For that purpose, a one-dimensional optical lattice is superimposed to the crossed dipole trap potential along the z-direction in order to freeze the atomic density distribution after a given time t. This allows us to access the time-dependent distributions fi(z, t), the position projection of the phase-space density ρi(z, vz, t), for the three states as shown in Fig. 1d. The position distributions for the two equilibrium states ρ1 and ρ3 do not vary in time, as expected. By contrast, the density-evolution of the nonequilibrium state ρ2 features a breathing dynamics, visible as a contraction of the distribution induced by the Raman pulse and the free phase-space evolution of the atomic cloud.

We determine the temperature T1 of the thermal state ρ1 from the measured position distributions by comparing them to the expected (axial) Boltzmann distribution \({f}_{{{{{{{{\rm{B}}}}}}}}}(z)\propto \exp \left(-{V}_{z}(z)/{k}_{{{{{{{{\rm{B}}}}}}}}}{T}_{1}\right)\) for various values of the parameter T1 in a χ2-analysis. Averaging over the temperatures from all four measured times, t = 0, 1.45, 2.90 and 4.35 ms, we find T1 = 15.6 ± 3.8 μK. We similarly obtain T3 = 22.7 ± 6.0 μK for the thermal state ρ3.

The characterization of the nonequilibrium state ρ2 proceeds as follows. Right after the DRSC pulse at t = 0, the velocity distribution is a Maxwellian at temperature TR, while the position distribution is still a Boltzmannian at temperature T1. Since TR ≠ T1, both f(z, t) and \(\tilde{f}({v}_{z},t)\) will evolve in time. Noting that the Raman temperature is the only free parameter of this nonequilibrium dynamics, TR can be extracted from the measured position distributions by again employing a χ2-analysis as before: we get TR = 4.4 ± 3.3 μK. A further consequence of the randomization effect of a Raman pulse is that the phase-space distribution factorizes, \({\rho }_{2}(z,{v}_{z},t=0)={f}_{B}(z){\tilde{f}}_{M}({v}_{z})\), directly after such a pulse. This factorization property also holds for a thermal state. We can thus determine the full axial phase-space distribution of all the three states, immediately after each cooling pulse, from the subsequently measured evolution of the respective position distributions.

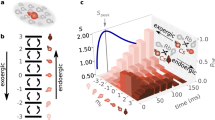
We now evaluate all the thermodynamic quantities needed to test the two generalized Clausius inequalities (1) and (2), such as energy, entropy and entropy production, from the phase-space densities ρi (i = 1, 2, 3). Figure 2a shows the validation of the reversed generalized Clausius inequality (2) for the first thermodynamic transformation that links the equilibrium state ρ1 to the nonequilibrium state ρ2 for three different values of the temperature T1, corresponding to three laser detunings (red, orange and yellow points); the inaccessible region is indicated by the gray area. According to the generalized Clausius inequality (1), these three processes are impossible, and hence not experimentally observable. Figure 2b exhibits both sides of the reversed generalized Clausius equality (2), as a function of time, for the three temperature values. The equality, including the nonequilibrium entropy production (red, orange and yellow crosses), is found to be obeyed for all the measurement points. Remarkably, all the thermodynamic quantities are independent of time, even though the nonlinear microscopic dynamics, as represented by the integrand of the relative entropy, \({{{{{{{\mathcal{D}}}}}}}}({\rho }_{1},{\rho }_{2})={\rho }_{1}\ln \left({\rho }_{1}/{\rho }_{2}\right)={{{{{{{\mathcal{D}}}}}}}}(z,{v}_{z})\), exhibit complex whorl structures (Fig. 2c) induced by the anharmonicity of the trap32. This follows from the (quasi) Liouvillian evolution of the isolated atomic system.

Fig. 2: Generalized Clausius inequalities.
figure 2

a Verification of the reversed generalized Clausius inequality (2) for the transition from ρ1 to ρ2. The red, orange and yellow points correspond to molasses detunings of Δfcool = −82 MHz, −62 MHz and −42 MHz, respectively. b Time evolution of all thermodynamic quantities in the reversed Clausius equality; the solid gray line indicates the analytical result (3). The small drift is due to the normalization of the phase-space density which is truncated to the experimentally accessible position range. c Complex whorl dynamics emerging in the phase-space integrand \({{{{{{{\mathcal{D}}}}}}}}={\rho }_{1}\ln \left({\rho }_{1}/{\rho }_{2}\right)\) of the relative entropy D for Δfcool = −82 MHz. d–f Analogous results to a–c for the generalized Clausius inequality (1). Error bars show statistical uncertainty of  ± 1σ standard deviation.

Full size image

Since the position marginals fi(z) before and after the Raman pulse are the same, we may additionally use the additivity property of the relative entropy for independent distributions29 to derive an analytical expression for the nonequilibrium entropy production at t = 0,

$$D({\rho }_{2}(0)| | {\rho }_{1})\,=\,D({\tilde{f}}_{2}(0)| | {\tilde{f}}_{1})\,=\,\frac{{k}_{{{{{{{{\rm{B}}}}}}}}}}{2}\,\left[\ln \left(\frac{{T}_{1}}{{T}_{{{{{{{{\rm{R}}}}}}}}}}\right)+\frac{{T}_{{{{{{{{\rm{R}}}}}}}}}}{{T}_{1}}-1\right].$$
(3)

Equation (3) follows from the Gaussian form of the Maxwell velocity distribution29 and only depends on the initial temperature T1 and the Raman temperature TR. This analytical value is indicated by the horizontal line in Fig. 2b and shows excellent agreement.

We have repeated the same analysis for the second thermodynamic process that brings the nonequilibrium state ρ2 to the thermal state ρ3 (Fig. 2d–f), for three different temperatures T3 (blue, teal and green points). The generalized Clausius inequality (1) holds for this thermalization process, as expected.

We finally examine the linear response approximation of the entropy production, which enables a simpler description of a nonequilibrium process6. Close to equilibrium, when ρi = ρj + dρij, the relative entropy D(ρi∣∣ρj) can be Taylor expanded as33,34,

$$D({\rho }_{i}| | {\rho }_{j})\simeq {k}_{{{{{{{{\rm{B}}}}}}}}}\int\,{dzdv}_{z}\frac{{(d{\rho }_{ij})}^{2}}{{\rho }_{j}}={k}_{{{{{{{{\rm{B}}}}}}}}}\frac{{U}_{ij}^{2}}{{\sigma }_{j}^{2}},$$
(4)

where \({\sigma }_{j}^{2}=\int\,{dzdv}_{z}{(H-\langle H\rangle )}^{2}{\rho }_{j}\) is the energy variance. This approximation, which expresses the entropy production as the distance between the mean energies in units of the fluctuations, is shown (triangles) for both processes in Fig. 3. It is markedly different from the exact values (crosses), indicating that the two transformations operate far from equilibrium.

Fig. 3: Linear response regime.
figure 3

a–c Relative entropy D (crosses) and linear response (4) (Y symbol) for the reversed generalized Clausius inequality. d–f Same quantities for the generalized Clausius equality. The linear approximation deviates significantly from the exact value, indicating that the experiment is performed far from the linear-response regime.

Full size image

Discussion

We have presented a detailed experimental study of two different generalized Clausius inequalities for the information entropy change using a closed sample of ultracold Cesium atoms. To this end, we have evaluated typical thermodynamic quantities, such as energy, entropy and relative entropy, from the determined phase-space density, for two nonequilibrium laser cooling protocols. We have confirmed the generalized Clausius inequality for the process ending with a thermal state and the reversed generalized Clausius inequality for the transformation beginning with a thermal state. Remarkably, the reversed inequality predicts exact opposite possible nonequilibrium transformations, highlighting the fact that the direction of a thermodynamic process is determined by its initial conditions35,36. While the Clausius inequality is ubiquitous in macroscopic systems that quickly thermalize, the reversed generalized Clausius inequality, which neither depends on the number of particles nor on their interactions, is expected to be the rule for systems, classical and quantum, that do not thermalize on their own in the absence of a heat bath12,13,14,15,16,17. Our findings provide unique insight into thermodynamic inequalities based on information-theoretic quantities, and the associated direction of nonequilibrium transformations, in nanoscopic systems not coupled to a heat reservoir. The ability of our experiment to reconstruct phase-space distributions is currently limited to few-particle systems. Exploring the thermodynamic consequences of the reversed generalized Clausius formula in more complex nonequilibrium systems would therefore be of interest in future studies.

Methods

Laser cooling techniques

For the optical molasses cooling of single Cs atoms, we employ the three-dimensional molasses laser setup which is also used for the creation of the magneto-optical trap18. There, three pairs of counter-propagating laser beams are employed for both, cooling and repumping light. The total cooling light intensity is Icool = 7.3 mW/cm2 = 2.7Isat and the detuning of cooling light to the \(F=4\to {F}^{{\prime} }=5\) hyperfine transition of the Cs D2 line37 is varied between Δfcool = −82 MHz = −15.7ΓD2 and Δfcool = −42 MHz = −8.0ΓD2. The repumping light at a total intensity of Irep = 2.5 mW/cm2 = 0.9Isat is on resonance to the \(F=3\to {F}^{{\prime} }=4\) hyperfine transition. When applying the optical molasses cooling to Cs atoms stored in the optical dipole trap, the atoms are cooled and at the same time move in the trapping potential. This creates a thermal-phase space distribution, if the duration of the molasses pulse is sufficiently long compared to the inverse trap frequency. For the molasses pulses employed here with several 100 ms duration, the state created after the cooling pulse is hence a thermal Gibbs state.

This is in contrast to the state created by the degenerate Raman sideband cooling (DRSC) technique. The DRSC scheme employed in our experiment closely follows ref. 21. An illustration of our setup can be found in ref. 38. A set of four DRSC lattice laser beams creates a three-dimensional optical lattice potential which is superposed to the dipole trap potential. At a typical detuning of δ44 = −6 MHz = −1.1ΓD2 to the \(\left\vert F=4\right\rangle \to \left\vert {F}^{{\prime} }=4\right\rangle\)-transition and an intensity of 0.48 W/cm2, the trap depth of this optical lattice for atoms in the \(\left\vert F=4\right\rangle\)-manifold is around kB × 40 μK with trap frequencies in the range of 10–100 kHz. The cooling effect of the DRSC is based on the successive reduction of the vibrational state \(\left\vert n\right\rangle\) in this lattice site. This is achieved by adjusting the magnetic field such that neighboring levels \(\vert F,{m}_{f}\rangle \vert n\rangle\) and \(\vert F,{m}_{f}+1\rangle \vert n-1\rangle\) of hyperfine and vibrational state become degenerate and hence can be coupled by a Raman transition with two photons from the DRSC lattice laser. An additional DRSC pump beam driving σ+-transitions at a detuning of δ32 = 12 MHz = 2.3ΓD2 from the \(\left\vert F=3\right\rangle \to \left\vert {F}^{{\prime} }=2\right\rangle\)-transition dissipates the vibrational energy. As a result, for the duration of the DRSC pulse, the Cs atoms are confined in the lattice sites created by the DRSC lattice lasers and cannot move in the optical dipole trap. Therefore, after the DRSC pulse, the atomic velocity distribution is given by the temperature TR which induced by the DRSC, but the position distribution in the optical dipole trap is unchanged and hence corresponds to a different temperature.

Potential separation

The anharmonic trapping potential in the experiment can be well described by a separable potential, \(\tilde{V}(x,y,z)\approx {V}_{x}(x)+{V}_{y}(y)+{V}_{z}(z)\). This approximation is constructed by sampling the full potential along the three principal axes x, y, and z, yielding the potential cuts Ui(i). Figure 1b of the main text shows the potential cuts Vi(i) and quantifies the validity of this approximation along the xyz-diagonal, where deviations are expected to be most significant. The relative deviation \({{{\Delta }}}_{{{{{{{{\rm{rel}}}}}}}}}=[\tilde{V}(x,y,z)-V(x,y,z)]/V(x,y,z)\) between the separable approximation and the full potential is below 10% for atoms with energies below kB × 100 μK. This indicates that for typical atomic energies of kB × 10 μK the separable approximation \(\tilde{V}\) is not only qualitatively but also quantitatively a good approximation of the full potential V. As a result, the atomic motion along the radial and axial directions are decoupled, thereby facilitating an independent treatment of the observed axial direction.