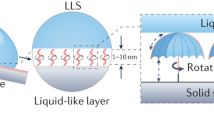
Abstract
Recently, there has been much progress in the design and application of oil-repellent superoleophobic surfaces. Polyzwitterionic brush surfaces are of particular interest, because of their ability to repel oil under water, even in the absence of micro-/nanostructures. The origin of this underwater superoleophobicity is attributed to the presence of a stable water film beneath the oil droplet, but this had not been demonstrated experimentally. Here, using optical interferometric techniques, we show that an oil droplet effectively hydroplanes over a water film, whose thickness is between one hundred and hundreds of nanometres. In addition, using a custom-built droplet force apparatus, we find the friction and adhesion forces to be in the nN range for millimetric-sized droplets. These forces are much lower than for other classes of well-known liquid-repellent surfaces, including the lotus-leaf effect and lubricant-infused surfaces, where the typical force is on the order of μN.
Similar content being viewed by others
Introduction
There is a growing interest in developing superoleophobic surfaces for various applications, including oil-repellent coatings and oil–water separation membranes1. Most superoleophobic surfaces (both in air and under water) require careful design of micro-/nanostructures to trap an air/water layer and hence confine oil droplet contact to the topmost tips of the structures2,3,4. Damage to the structures greatly impairs the oil repellency performance of these surfaces, as the air/water layer is no longer stable and the oil droplet becomes highly pinned. This is analogous to the wetting transitions from Cassie–Baxter to Wenzel states in lotus-leaf effect superhydrophobic surfaces5,6.
In contrast, polyzwitterionic brush surfaces are able to repel oil under water even in the absence of micro-/nanostructures7,8. This has been attributed to the ability of polyzwitterionic brushes to swell under water and retain hydration shells around their charged moieties. This stabilizes a water film between the oil droplet and the underlying substrate, and hence results in extreme oil repellency. Incidentally, it is thought that the presence of a water film on cartilage surfaces (stabilized by zwitterionic phospholipids) is similarly responsible for the ultra-low friction in skeletal joints9,10.
Despite recent progress, there is little direct experimental evidence for the presence of the water film between the oil droplet and the substrate, and most previous discussions implicitly assume the presence of such a hydration layer7,8,11,12. The thickness of this water film—should it exist—is not known and its relation to oil repellency remains poorly understood. Furthermore, the effect of such a water film on adhesion and friction of oil droplets has not been investigated. Here, using reflection interference contrast micrscopy (RICM), we are able to directly visualize and quantify the thickness of the water film between an oil droplet and a glass substrate grafted with poly(sulfobetaine methacrylate) (PSBMA) brushes. In addition, using a custom-built instrumentation, which we name the droplet force apparatus (DFA), we found the friction and adhesion forces of the oil droplet Fadh/fric to be in the nN range. Our results can be largely explained by viscous dissipation in the experimentally observed water film. Finally, we propose and experimentally verify simple scaling relations for Fadh/fric.
Results
Polymer brush growth
The PSBMA brushes were grown on glass or silicon wafer using surface-initiated atom transfer radical polymerization (SI-ATRP)13,14. The brush thickness, as measured with ellipsometry and atomic force microscopy (AFM), initially increased linearly as a function of the polymerization time, but then reached a plateau after about an hour to a maximum thickness hdry = 34 ± 4 nm (Fig. 1a). When immersed under water, the brush swells by about 50% to hwet = 51 ± 5 nm. The relatively small swelling ratio is consistent with densely grafted chains in the brush state13,15. See Supplementary Figs. 1–3 and Supplementary Notes 1–3 for contact angle measurements, chemical, and AFM characterizations of the brushes.
Polyzwitterionic brushes and water film stability. a Dry and wet thicknesses of the poly(sulfobetaine methacrylate) (PSBMA) brushes hdry,wet measured using ellipsometry and atomic force microscopy (AFM) for different polymerization times. b–d The presence of a stable hydration layer beneath the oil droplet can be confirmed using reflection interference contrast microscopy. e Sideview image of the oil droplet. f–h In the absence of a stable water film, solid–oil contact can clearly be seen. i Sideview image of the oil droplet. Scale bars are 50 μm for c and g, 10 μm for d and h, and 0.5 mm for e and i. The droplets are held in place by a capillary tube
Stability and thickness of water film
The presence or absence of a water film can be confirmed using RICM16,17. Here we shone monochromatic light (wavelength λ = 561 nm) from below and captured the reflection off the droplet’s base using a camera (Fig. 1b). The presence of a water film results in thin-film interference and, in particular, dark and bright fringes as light reflected off the various interfaces (oil–water, water–brush, and brush–glass) interfere destructively and constructively with one another.
For a glass surface grafted with 30 nm-thick PSBMA brushes, the presence of interference fringes at the edge of the droplet’s base (Fig. 1c, d) indicates the presence of a continuous water film. As there is no contact between the droplet and the underlying substrate, the droplet’s base is free from pinning and hence appears smooth and circular (Fig. 1c). In contrast, for 6 nm-thick brushes, the water film is not stable and the droplet–solid contact area can clearly be seen (Fig. 1g); in place of interference fringes at the edge, there is instead an irregularly shaped outline due to three-phase contact-line pinning (Fig. 1h).
We note that the static contact angles of the oil droplet can be indistinguishable (effectively 180°) for both stable and unstable states (Fig. 1e, i). Recently, several groups have established the difficulty of accurately measuring contact angles close to 180°18,19. Small uncertainty in the baseline of the droplet, even at single pixel level, leads to large error in contact angle values (>10°), highlighting the challenge in using conventional contact angle measurements to characterize the wetting properties of surfaces18,19,20,21
Experimentally, we found that the water film becomes unstable for brushes with thicknesses hdry < 5 nm and hwet < 9 nm (shaded grey, Fig. 1a). This is likely because for short polymer chains, the wetting properties of the surface are dominated by the presence of non-hydrophilic bromine atoms from the polymerization initiator. X-ray photoelectron spectroscopy characterization of the surface is consistent with this view (Supplementary Fig. 2).
The presence of a stable water film has a profound impact on the oil-repellent performance of the PSBMA brushes. The measured adhesion and friction forces Fadh/fric for a millimetric-sized oil droplet are on the order of tens of nN for a stable hydration state, but increase to several μN for an unstable hydration state. In comparison, Fadh/fric is on the order of μN for similarly sized water droplets on superhydrophobic surfaces exhibiting the lotus-leaf effect20,22,23.
For a stationary droplet, the water film thickness hwater can be determined using dual-wavelength confocal RICM16,17. We raster scanned the surface simultaneously with two focused beams of monochromatic lights with wavelengths λ = 458 and 561 nm, and captured the reflected light through the pinhole of a confocal microscope (Fig. 2a). From the reflection intensities of the two wavelengths, the water film profile hwater can be deduced unambiguously using classical Fresnel theory (Fig. 2b). After waiting for 20 min, we find that the equilibrium hwater is about 200 nm, much thicker than the swollen brush film thickness hwet (Fig. 2c).
Results of the confocal reflection interference contrast microscopy (RICM) experiments. a Water film visualized using RICM at wavelengths λ = 405 and 561 nm at different times. Scale bar is 100 μm. b Normalized reflection light intensities across the centre of the droplet’s base at t = 1 min for λ = 405 and 561 nm (solid blue and green lines, respectively). Dashed lines are the best-fit lines using the theory of Fresnel reflection. c Water film profiles at different times. The equilibrium film thickness hwater is about 200 nm
Alternatively, hwater can be measured by shining white light and analyzing the reflected light using a spectrometer, i.e., spectroscopic reflectometry (Fig. 3a). Similar equilibrium film thicknesses (between 100 and 200 nm) are obtained using this method (Fig. 3b). Details of the two techniques (spectroscopic reflectometry and RICM) can be found in our previous work17 and also in Supplementary Figs. 4–8 and Supplementary Notes 3 and 4. See also discussion in the ‘Methods’ section.
Water film thickness hwater for a moving oil droplet. a hwater measured using spectroscopic reflectometry. b Thin-film interference of the water film results in different colours observed under the droplet. hwater can be determined by fitting the reflectivity measurements from the spectrometer (measured areas are indicated by blue circles) with the theory of Fresnel reflection. Dashed lines are the best-fit curves. Scale bars are 100 μm. c Non-dimensionalized quantities hwater/R against Ca = ηU/γ obtained for silicone and fluorinated oils. Dashed line is the prediction from Landau–Levich–Derjaguin theory. Errors for thickness measurements are about 10 nm and are smaller than the marker sizes
The relatively thick water film is stabilized by repulsive electric double-layer force. In water, both oil and the PSBMA surface acquire negative surface charges, with reported zeta potentials of −40 mV and −35 mV, respectively12,25. In the absence of added electrolytes, the electrostatic forces between the charged interfaces generate a repulsive pressure given by
where εo and ε = 80 are the vacuum and relative permittivities, kB is the Boltzmann constant, T is the absolute temperature, z is the valency of the counterions, and e is the elementary charge26. P(h) is balanced by the capillary pressure γ/R, which predicts an equilibrium h of about 100 nm, consistent with the experimentally observed hwater. We also note that hwater is reduced to 10 nm with added salt (0.1 M NaCl), as the surface charges are screened by the dissolved ions (see Supplementary Fig. 8 and Supplementary Note 5).
Experimentally, we found that hwater increases with the droplet’s speed U. For example, hwater for the same droplet increases from 130 to 330 nm when moving at U = 40 μm s−1 (Fig. 3b), and reaches more than 1 μm for U > 1 mm s−1 (Supplementary Fig. 5d). For silicone and fluorinated oils moving at U = 0.1–2.0 mm s−1, hwater is well described by the Landau–Levich–Derjaguin (LLD) scaling
where R is the droplet’s radius, Ca = ηU/γ is the capillary number, η is the water viscosity, and γ = 40–50 mN m−1 is the oil–water interfacial tensions (Fig. 3c)17,27,28,29. This is analogous to liquid films entrained during the dip-coating process.
It is known that water remains fluid even when confined to subnanometre films30. Thus, for hwater > 100 nm, water acts as a lubricating film, and we expect friction and adhesion forces Ffric,adh to be minimal and be dominated by viscous dissipation in the water film. Moreover, Ffric,adh should be relatively insensitive to details of the brush layer, such as its thickness hwet and grafting density. We show this explicitly by measuring Ffric,adh using a custom-built force sensor, which we name the DFA.
Adhesion and friction forces
DFA is an improvement to an experimental setup reported in our previous works17,20. By building the whole setup on an active-damped optical table and inside an enclosure to minimize draught, we are able to greatly reduce the environmental noise and measure forces as small as tens of nN, making it one of the most sensitive force sensors for wetting applications22,31. In contrast, our previous setup has a sensitivity of more than 100 nN17. At the same time, the film dynamics at the droplet’s base can be visualized by either RICM (Fig. 1b) or spectroscopic reflectometry (Fig. 3) making the combined setup an extremely powerful scientific apparatus for the direct and simultaneous measurement of contact areas, thicknesses, and forces for liquid droplets interacting with various interfaces. Details of the setup can be found in Supplementary Figs. 4–8 and Supplementary Note 4.
Figure 4a is a schematic of how Ffric was measured using the DFA for different speeds U. The droplet was attached to an acrylic capillary tube with inner and outer diameters of 0.288 and 0.360 mm, respectively; as the surface was moving at controlled U, the force acting on the droplet was inferred from the tube’s deflection Δx, as F = kΔx, where k = 2–20 mN m−1 is the flexular spring constant, which we determine individually for each tube. With a high-resolution camera and auto-correlation algorithm, it is possible to detect Δx of a few μm, which translates to a force resolution of about 10 nN (Supplementary Figs. 6 and 7).
Friction force Ffric acting on the droplet. a Ffric measured using the droplet force apparatus (DFA). b Force–time curves for droplets (2R = 2.3 mm) for stable and unstable water films (blue and red lines, respectively). The surfaces started moving with U = 0.7 mm s−1 at time t = 8 s and stopped at t = 25 s. Oscillation of F when the motor was stopped are transients due to sudden deceleration akin to a dampled harmonic oscillator. Interferograms are for the droplets’ base at time points A, B, and C. Scale bar is 50 μm. c Plots of non-dimensionalized force F/2rγ vs. capillary number Ca. Each point represents a single force measurement. The uncertainties in deflection detected by the auto-correlation algorithm are either smaller than the point size or represented by the error bars
Figure 4b shows the force–time curves for silicone oil droplets (2R = 2.3 mm) moving at U = 0.7 mm s−1. When a stable water film is present, the force required to jumpstart the motion Fs = 230 nN is close to that required to maintain the motion Fk = 150 nN (Supplementary Movie 1). This is reminiscent of the static and kinetic friction forces between two solid surfaces20,32. For a droplet hydroplaning on a water film that follows the LLD scaling, it is known that the friction force due to viscous dissipation is given by
where 2r is the size of the droplet’s base, which depends on the applied normal force FN = (2γ/R)πr2 17,33,34 (see Supplementary Fig. 9 and Supplementary Note 6 for derivation). For silicone and fluorinated oils (empty and filled markers in Fig. 4c, respectively) of 2R = 3.3–4.7 mm and 2r = 0.25–0.93 mm, as long as the water film is stable, Fk is well described by Eq. (3) (dashed line in Fig. 4c with a prefactor of 12) and is independent of hwet = 9–45 nm.
We can also define the effective coefficient of friction μf = Ffric/FN ~ (R/r)Ca2/3. For millimetric droplets moving at U = 1–1000 μm s−1, this translates to μf = 0.01–0.1. In comparison, μf can be as low as 0.001 for solid–solid friction between cartilage surfaces9,10. Nonetheless, Ffric is remarkably low. At the lowest speed U = 10 μm s−1, Ffric,min = 25 nN, much lower than that for lotus-leaf effect surfaces (typically μN for speeds ranging from μm s−1 to mm s−1)8,20.
In contrast, for unstable water film, Fs = 1.9 μN is much larger than Fk = 150 nN. In this case, Fs is the force required to force wet the water film (Supplementary Movie 2) and its magnitude (~μN) is comparable to Ffric in lotus-leaf effect surfaces. Once the droplets moves, the water film is dynamically stabilized at a thickness given by the LLD scaling (Eq. 2) and Fk is once again given by Eq. (3). Experimentally, we found that Fs is independent of the applied U (solid line in Fig. 4c), similar to the friction behaviour of a water droplet on superhydrophobic lotus-leaf effect surfaces8,20.
The adhesion force Fadh can similarly be measured using the DFA, with the surface positioned vertically (Fig. 5a). In a typical experiment, the surface first approached the droplet from afar up to a loading force Fload (A to B in Fig. 5b), before retracting at a controlled speed U (B to C). The adhesion force Fadh is the maximum force just before the droplet detaches (Supplementary Movies 3 and 4). For a droplet of 2R = 2.0 mm and U = 50 μm s−1, Fadh = 85 nN and 4.6 μN for stable and unstable water film, respectively (Fig. 5b). For the latter case, we occasionally measured a snap-in force Fsnap as the water film dewetted and the oil droplet contacted the surface; there is contact-line pinning and the droplet’s base is jagged and distorted. After the droplet detached, a small microdroplet was sometimes left on the surface. In contrast, for stable water film, Fsnap was never observed, the droplet’s base was always smooth and circular in shape, and there was no microdroplet left behind (see RICM images at time point C in Fig. 5b).
Adhesion force Fadh acting on the droplet. a Fadh measured using the droplet force apparatus (DFA). b Force–time curves for droplets (2R = 2.0 mm) for stable and unstable water films (blue and red lines, respectively). The surface was approaching the droplet (A to B) and retracting from it (B to C) at a speed U = 0.05 mm s−1. Interferograms are for the droplets’ base at time points A, B, and C. Scale bar is 50 μm. c Plots of non-dimensionalized force Fadh/2Rγ vs. capillary number Ca. Each point represents single force measurement. The uncertainties in deflection detected by the auto-correlation algorithm are either smaller than the point size or represented by the error bars
For a stable water film, Fadh is once again dominated by viscous forces. At the point of droplet detachment, using scaling arguments, we expect hwater ~ RCa1/2 and r ~ RCa1/4 (cf. Eq. 2)35,36 (see Supplementary Fig. 9 and Supplementary Note 7 for derivation). Hence, Fadh should scale as
However, we found that the experimental data is best explained with an exponent of 0.6 (rather than 0.5) and a prefactor of 4 (dashed line in Fig. 5c). To account for this discrepancy requires solving the Navier–Stokes equation fully, but the physical rationale for Eq. (4) is nevertheless correct: Fadh is viscous in origin and is therefore insensitive to the details of the polymer brush layer, e.g., its thickness hwet = 9–45 nm. Experimentally, we also found that Fadh is independent of Fload (Supplementary Fig. 10). At the lowest velocity U = 2 μm s−1, we obtained Fadh,min = 20 nN for a millimetric droplet. In comparison, when the water film is unstable, Fadh is much larger (>1 μN) and is independent of U = 4–2000 μm s−1 and Ca = 8 × 10−8–4 × 10−5 (solid line in Fig. 5c).
Finally, we note that the friction and adhesion forces measured by the DFA are primarily due to contact-line pinning (for unstable water film) or viscous flow (for stable film) at the droplet’s base, and not due to flow around the droplet or any capillary force acting on the cantilever. We confirmed this by repeating the friction force measurement with the droplet’s base far from the surface (a couple of millimetres away), in which case no measurable cantilever deflection was detected. See also discussion in Supplementary Figs. 6 and 7, and Supplementary Note 4.
Discussion
We would like to point out that the polyzwitterionic brush surfaces are qualitatively different from other classes of liquid-repellent surfaces, notably the lotus-leaf effect surfaces6,34 and the Nepenthes pitcher-plant inspired lubricated surfaces37,38. For example, for polyzwitterionic brush surfaces Ffric,adh varies nonlinearly with U (∝U2/3 and U0.6, respectively), whereas for lotus-leaf effect surfaces Ffric,adh is relatively insensitive to U ~ mm s−1 or less20,22,34,39. Table 1 summarizes the functional forms of Ffric,adh for three surface classes, as reported here and elsewhere in the literature17,20,23,33,34. The values of |Ffric,adh| are for droplets of size 2R = 1–4 mm and speeds U = 0.01–2 mm s−1, and as discussed previously, |Ffric,adh| is much lower for polyzwitterionic brushes as compared with lotus-leaf effect and lubricated surfaces.
In this work (and our previous work20), we have presented the friction and adhesion force data in their non-dimensionalized forms: Ffric/2rγ or Fadh/2Rγ vs. Ca. Figures 4c and 5c can be thought of as universal phase diagrams for liquid repellency; Ffric and Fadh data for different classes of liquid-repellent surfaces and experimental conditions (different oils, droplet sizes, and speeds) can be plotted on the same graph for direct comparison. The non-dimensional quantity Ffric/2rγ is also numerically equivalent to the contact angle hystereses Δcosθ that are typically reported in the literature (Furmidge’s relation)40. This, again, allows for easy comparison with literature data. For example, most lotus-leaf effect surfaces have Δcos θ = 0.05–0.10, much higher than the values reported here where Ffric/2rγ = 0.001–0.0120.
In summary, we have clarified the origin of underwater oil repellency for polyzwitterionic brush surfaces, in particular the role played by hydration lubrication. We were able to measure the thickness of the water film beneath the oil droplet with nanometric accuracy, as well as the friction and adhesion forces with nN resolutions using a custom-built DFA. Just as the surface force apparatus has revolutionized the study of surface forces, we believe our DFA setup will help resolve many of the outstanding questions in wetting science. Finally, although we have confined our discussion to oil-repellent properties of polyzwitterionic brushes, many of the ideas and techniques outlined here are relevant to other oil-repellent surfaces (e.g., polycationic/anionic brushes and hydrogels), and more generally to other classes of liquid-repellent surfaces. The insights generated here are pertinent to the rational design of anti-fouling materials not just for liquids but also for biological contaminants.
Methods
Chemicals and materials
The (3-trimethoxysilyl)-propyl 2-bromo-2-methylpropionate (Br-initiator, 95%) was purchased from Gelest, Inc. SBMA ([2-(Methacryloyloxy)ethyl]dimethyl-(3-sulfopropyl) ammonium hydroxide, ≥96%), copper(I) bromide (CuBr, ≥98%), and 2,2-′bipyridine (bipy, ≥99%) were purchased from Sigma-Aldrich. Methanol was purchased from J.T. Baker. All chemicals were used as received.
Silicone oil (Polyphenyl-methylsiloxane, viscosity ~100 mPa s−1, density 1.06 g ml−1) and fluorinated oil (viscosity ~15 mPa s−1, density 1.93 g mL−1, Fluorinert FC-70) were purchased from Sigma-Aldrich. The water–oil interfacial tensions are 40 and 50 mN m−1 for silicone and fluorinated oils, respectively, as measured using the pendant drop method.
Deionized (DI) water with a resistivity of 18.2 MΩ cm−1 was obtained from a Milli-Q water purification system (Millipore, Bedford, MA, USA).
Sample preparation
The polymer brush surfaces are prepared using SI-ATRP using a protocol adapted from Azzaroni et al.13.
The surfaces (glass or silicon wafer) were first rinsed extensively with DI water and then ethanol, before drying under a nitrogen stream. The dried surfaces were then subjected to oxygen plasma surface cleaning at 150 W for 120 s. Br initiator was vapour deposited onto the cleaned surfaces. In a typical procedure, the cleaned surfaces were heated in a vacuum oven at 75 °C with the Br initiator (100 μL) overnight. The silanized surfaces were then cleaned (by rinsing with anhydrous toluene, ethanol, and water, sequentially) and then dried under a nitrogen stream. The dried silanized surfaces were then heated in an oven at 110 °C for 20 min.
For the polymer brush growth, the solvent solution (4:1 methanol:water, 50 mL) was first deoxygenated by bubbling with nitrogen for at least 30 min. SBMA (53.70 mmol, 15.0 g) was dissolved in 40 mL of the solvent solution to form the monomer solution, whereas bipy (1.344 mmol, 209.88 mg) and CuBr (0.5375 mmol, 77.11 mg) were dissolved in the remaining 10 mL of the solvent solution to form the catalytic solution. Both solutions were then stirred, while continuously being bubbled with nitrogen. After about 10 min, the catalytic solution was added to the monomer solution and was allowed to stir for another 2 min under nitrogen protection. The reaction mixture was then transferred to the reaction vessel containing the silanized surfaces. The reaction was performed under nitrogen protection. The reaction time was varied to achieve various brush heights. Upon completion of the polymer brush growth, the surfaces were rinsed with copious amounts of warm DI water (60 °C) and dried under a nitrogen stream.
Determining brush thickness
The dry and wet thicknesses of the polymer brush layer on a silicon wafer can be determined using either ellipsometry or AFM measurements. Both techniques give similar results. For brush layer on glass, the thickness can only be determined using AFM measurement, as the refractive index contrast between the brush layer and glass is too small for accurate ellipsometry measurement.
Spectroscopic ellipsometry measurement was performed using a commercial ellipsometer (VASE from J.A. Woollam Co., Inc.) at an incident angle of 70°. For ellipsometry measurement in water, the surface is placed in a custom-built quartz chamber with side windows at 70° angle. The index of refraction of the polymer brush layer was fitted using a Cauchy model (dry and wet). For swollen brush layer, the index of refraction can alternatively be modelled using the Bruggeman effective medium approximation. Both models (Cauchy and Brugemann) give similar results. At least three measurements across each sample were taken.
For AFM measurement, a small section of the brush layer was first scratched using a tweezer to reveal the underlying glass/silicon substrate; the surface was then rinsed with copious amount of water and dried under nitrogen gas to remove any detached brushes. The dry and wet thicknesses of the brush layer can then be measured using AFM by probing the scratched section. Detailed topography of the brushes was obtained using the Quantitative Imaging mode (JPK Instruments), which minimizes the distortion due to lateral and compressive forces41,42. This is particularly important for brushes under water, which become soft when swollen (see Supplementary Fig. 3 for details).
Determining water film thickness
The thickness of the water film can be determined using either microscopic reflection spectroscopy or dual-wavelength confocal RICM. See Supplementary Figs. 4, 5 and 8.
For confocal RICM, we raster scanned the surface simultaneously with two focused beams of monochromatic lights with wavelengths λ = 458 and 561 nm, and captured the reflected light through the pinhole of a confocal microscope; as a result, only reflected light from the focal plane, i.e., the interface of interest, was able to reach the photomultiplier tube of the microscope. This is crucial, because the weak refractive index contrast leads to a weak reflection signal that can be overwhelmed by stray light. In the presence of a thin water film, the light reflected off the various interfaces (glass–brush, brush–water, and water–oil) will interfere with one another constructively or destructively and result in bright or dark fringes, respectively. From the reflection intensities of the two wavelengths, the water film profile hwater can be deduced unambiguously using classical Fresnel theory24.
For microscopic reflection spectroscopy, white light is used instead of monochromatic lights. The light reflected off the various interfaces will again interfere with one another and its intensities (at various wavelengths) were then analyzed using a spectrometer. As with confocal RICM, the local film thickness hwater can be deduced using classical Fresnel theory. See Supplementary Fig. 4 for the optical path of the setup.
In both methods, the reflection intensities were fitted to classical Fresnel theory using Levenberg–Marquart algorithm with hwater as the only fitting parameter.
The refractive indices of the various materials (water, polymer brush, glass, and oil), including their optical dispersions, as well as the the polymer brush thickness hwet were measured using an ellipsometer and inputted directly into the Fresnel theory. The accuracy of hwater is therefore limited by the uncertainty of Δhwet, which is about 5 nm, much smaller than hwater > 100 nm.
Data availability
The authors declare that the data supporting the findings of this study are available from the corresponding author upon reasonable request.
References
Yong, J., Chen, F., Yang, Q., Huo, J. & Hou, X. Superoleophobic surfaces. Chem. Soc. Rev. 46, 4168–4217 (2017).
Tuteja, A. et al. Designing superoleophobic surfaces. Science 318, 1618–1622 (2007).
Liu, T. & Kim, C.-J. Turning a surface superrepellent even to completely wetting liquids. Science 346, 1096–1100 (2014).
Liu, M., Wang, S., Wei, Z., Song, Y. & Jiang, L. Bioinspired design of a superoleophobic and low adhesive water/solid interface. Adv. Mater. 21, 665–669 (2009).
de Gennes, P.-G., Brochard-Wyart, F. & Quéré, D. Capillarity and wetting phenomena: drops, bubbles, pearls, waves (Springer, 2004).
Quéré, D. Wetting and roughness. Annu. Rev. Mater. Res. 38, 71–99 (2008).
Kobayashi, M. et al. Wettability and antifouling behavior on the surfaces of superhydrophilic polymer brushes. Langmuir 28, 7212–7222 (2012).
Liu, M., Wang, S. & Jiang, L. Nature-inspired superwettability systems. Nat. Rev. Mater. 2, 17036 (2017).
Jahn, S., Seror, J. & Klein, J. Lubrication of articular cartilage. Annu. Rev. Biomed. Eng. 18, 235–258 (2016).
Zhulina, E. B. & Rubinstein, M. Lubrication by polyelectrolyte brushes. Macromolecules 47, 5825–5838 (2014).
Huang, K.-T., Yeh, S.-B. & Huang, C.-J. Surface modification for superhydrophilicity and underwater superoleophobicity: applications in antifog, underwater self-cleaning, and oil–water separation. ACS Appl. Mater. Interfaces 7, 21021–21029 (2015).
Shi, C. et al. Long-range hydrophilic attraction between water and polyelectrolyte surfaces in oil. Angew. Chem. Int. Ed. 55, 15017–15021 (2016).
Azzaroni, O., Brown, A. A. & Huck, W. T. S. UCST wetting transitions of polyzwitterionic brushes driven by self-association. Angew. Chem. Int. Ed. 45, 1770–1774 (2006).
Zoppe, J. O. et al. Surface-initiated controlled radical polymerization: state-of-the-art, opportunities, and challenges in surface and interface engineering with polymer brushes. Chem. Rev. 117, 1105–1318 (2017).
Cheng, N., Brown, A. A., Azzaroni, O. & Huck, W. T. S. Thickness-dependent properties of polyzwitterionic brushes. Macromolecules 41, 6317–6321 (2008).
Limozin, L. & Sengupta, K. Quantitative reflection interference contrast microscopy (RICM) in soft matter and cell adhesion. ChemPhysChem 10, 2752–2768 (2009).
Daniel, D., Timonen, J. V. I., Li, R., Velling, S. J. & Aizenberg, J. Oleoplaning droplets on lubricated surfaces. Nat. Phys. 13, 1020 (2017).
Srinivasan, S., McKinley, G. H. & Cohen, R. E. Assessing the accuracy of contact angle measurements for sessile drops on liquid-repellent surfaces. Langmuir 27, 13582–13589 (2011).
Liu, K., Vuckovac, M., Latikka, M., Huhtamäki, T. & Ras, R. H. A. Improving surface-wetting characterization. Science 363, 1147–1148 (2019).
Daniel, D. et al. Origins of extreme liquid repellency on structured, flat, and lubricated hydrophobic surfaces. Phys. Rev. Lett. 120, 244503 (2018).
Huhtamäki, T., Tian, X., Korhonen, J. T. & Ras, R. H. A. Surface-wetting characterization using contact-angle measurements. Nat. Protoc. 13, 1521 (2018).
Liimatainen, V. et al. Mapping microscale wetting variations on biological and synthetic water-repellent surfaces. Nat. Commun. 8, 1798 (2017).
Reyssat, M. & Quéré, D. Contact angle hysteresis generated by strong dilute defects. J. Phys. Chem. B 113, 3906–3909 (2009).
Born, M. & Wolf, E. Principles of optics: electromagnetic theory of propagation, interference and diffraction of light (Elsevier, 2013).
Guo, S. et al. Surface charge control for zwitterionic polymer brushes: tailoring surface properties to antifouling applications. J. Coll. Interface Sci. 452, 43–53 (2015).
Israelachvili, J. N. Intermolecular and surface forces (Academic Press, 2011) Chap. 14.
Landau, L. & Levich, V. Dragging of a liquid by a moving plate. Acta Physicochim. USSR 17, 42–54 (1942).
Derjaguin, B. Thickness of liquid layer adhering to walls of vessels on their emptying and the theory of photo-and motion-picture film coating. Dokl. Acad. Sci. USSR 39, 13–16 (1943).
Bretherton, F. P. The motion of long bubbles in tubes. J. Fluid Mech. 10, 166–188 (1961).
Raviv, U., Perkin, S., Laurat, P. & Klein, J. Fluidity of water confined down to subnanometer films. Langmuir 20, 5322–5332 (2004).
Pilat, D. W. et al. Dynamic measurement of the force required to move a liquid drop on a solid surface. Langmuir 28, 16812–16820 (2012).
Gao, N. et al. How drops start sliding over solid surfaces. Nat. Phys. 14, 191 (2018).
Keiser, A., Keiser, L., Clanet, C. & Quéré, D. Drop friction on liquid-infused materials. Soft Matter 13, 6981–6987 (2017).
Reyssat, M., Richard, D., Clanet, C. & Quéré, D. Dynamical superhydrophobicity. Faraday Discuss. 146, 19–33 (2010).
Bouwhuis, W. et al. Maximal air bubble entrainment at liquid-drop impact. Phys. Rev. Lett. 109, 264501 (2012).
Klaseboer, E., Manica, R. & Chan, D. Y. C. Universal behavior of the initial stage of drop impact. Phys. Rev. Lett. 113, 194501 (2014).
Lafuma, A. & Quéré, D. Slippery pre-suffused surfaces. Europhys. Lett. 96, 56001 (2011).
Wong, T.-S. et al. Bioinspired self-repairing slippery surfaces with pressure-stable omniphobicity. Nature 477, 443–447 (2011).
Mouterde, T., Raux, P. S., Clanet, C. & Quéré, D. Superhydrophobic frictions. Proc. Natl Acad. Sci. USA 116, 8220–8223 (2019).
Furmidge, G. C. L. Studies at phase interfaces. i. the sliding of liquid drops on solid surfaces and a theory for spray retention. J. Colloid Sci. 17, 309–324 (1962).
Rodriguez-Emmenegger, C., Janel, S., de los Santos Pereira, A., Bruns, M. & Lafont, F. Quantifying bacterial adhesion on antifouling polymer brushes via single-cell force spectroscopy. Polym. Chem. 6, 5740–5751 (2015).
Chopinet, L., Formosa, C., Rols, M. P., Duval, R. E. & Dague, E. Imaging living cells surface and quantifying its properties at high resolution using AFM in QI mode. Micron 48, 26–33 (2013).
Acknowledgements
We thank R.E. Cohen and P.V. Braun for fruitful discussions, and K. Serrano for advice on polymer brush synthesis. We are grateful to the Agency for Science, Technology and Research (A*STAR) for providing financial support under the PHAROS Advanced Surfaces Programme (grant number 1523700101, project number IMRE/16-2P1203).
Author information
Authors and Affiliations
Contributions
D.D. and N.T. conceived and planned the experiments. D.D., A.Y.T.C., L.C.H.M., R.L., X.Q.K. and X.Z. executed the experimental work. D.D. analysed the experimental results and performed the scaling analysis for the friction and adhesion forces. D.D. and N.T. wrote the manuscript. All authors reviewed the manuscript.
Corresponding authors
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Daniel, D., Chia, A.Y.T., Moh, L.C.H. et al. Hydration lubrication of polyzwitterionic brushes leads to nearly friction- and adhesion-free droplet motion. Commun Phys 2, 105 (2019). https://doi.org/10.1038/s42005-019-0205-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1038/s42005-019-0205-x
This article is cited by
-
New insights and novel perspectives in bileaflet mechanical heart valve prostheses thromboresistance
Journal of Cardiothoracic Surgery (2024)
-
Probing surface wetting across multiple force, length and time scales
Communications Physics (2023)
-
Water droplet friction and rolling dynamics on superhydrophobic surfaces
Communications Materials (2020)
Comments
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.